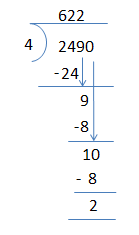Marksparks, Author at Answer Key for Math

Primary Mathematics Workbook 4A Common Core Edition Solutions Chapter 2 The Four Operations Of Whole Numbers Exercises 2.1

March 17, 2023March 15, 2023 by Marksparks

Primary Mathematics Chapter 2 The Four Operations Of Whole Numbers

Page 40 Exercise 2.1 Problem 1

To find – The sum of

7 4 2 3
+ 6 5 2
_________

Place the digits under respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place values

7 4 2 3
+ 6 5 2
___________

Adding the digits in each place value

Th H T O
7 4 2 3
+ 6 5 2
___________
8 0 7 5

Hence, the sum of ⇒ 7 4 2 3 + 6 5 2 = 8075.

Page 40 Exercise 2.1 Problem 2

To find – The sum of

2 3 5 7
+ 6 5 2
__________

Place the digits under respective place values.

Add the digits in each place value taking the carryover, if any, to the next column to the left, and add it along with the digit in that place value.

Continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place values,

Th H T O
2 3 5 7
+ 6 5 2
___________

Adding the digits in each place value

Th H T O
2 3 5 7
+ 6 5 2
_____________
3 0 0 9

Hence, the sum of ⇒ 2 3 5 7 + 6 5 2 = 3009.

Page 41 Exercise 2.1 Problem 3

Given: Some numbers.

To find – The difference between the given numbers.

The difference between two numbers is nothing but the subtraction of the smallest number from the largest number.

Follow the steps given below.

Complete the subtractions in the first row.

The first difference (subtraction) is given by

5 6 7 8
1 2 3 4
−
____________
4 4 4 4

The second difference (subtraction) is given by

8 4 3 2

− 9 7 6
_________
7 4 5 6

The third difference (subtraction) is given by

5 1 2 3
1 2 3 4
−
_________
3 8 8 9

Complete the subtractions in the second row.

The first difference (subtraction) is given by

3 0 0 0
2 8 7 4
−
__________
1 2 6

The second difference (subtraction) is given by

9 0 0 1
− 1 2 8
__________
8 8 7 3

The third difference (subtraction) is given by

6 0 6 0
3 6 8 7
−
__________
2 3 7 3

Complete the subtractions in the third row.

The first difference (subtraction) is given by

5 2 6 2
4 9 0 8
−
__________
3 5 4

The second difference (subtraction) is given by

1 0 0 6
− 8 9 7
___________
1 0 9

The third difference (subtraction) is given by

9 0 2 2
1 1 3 4
−
__________
7 8 8 8

Complete the subtractions in the fourth row.

The first difference (subtraction) is given by

4 2 0 0
1 5 3 2
−
_________
2 6 6 8

The second difference (subtraction) is given by

1 9 8 2
− 9 9 9
_________
9 8 3

The third difference (subtraction) is given by

7 2 5 8

6 1 8 9
_
__________
1 0 6 9

The complete difference for each expression is given below.

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 41 Exercise 2.1 Problem 3, table

Page 41 Exercise 2.1 Problem 4

Given: Some numbers in a box.

To find – The name of the city which is a landmark of Golden Bridge.

Referring to Exercise 2.1 above table it is obtained that

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 41 Exercise 2.1 Problem 4, table 1

Observe that, the first given number 109 corresponds to the letter “S” in the above table.

The second given number 1069 corresponds to the letter “A “ in the above table.

The third given number 3889 corresponds to the letter “N “ in the above table, and so on…

Finally, in this way, all the letters will form the word “SAN FRANCISCO” as shown in the table below.

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 41 Exercise 2.1 Problem 4, table 3

The city that is the landmark of Golden Bridge is “SAN FRANCISCO” which is represented by the following table.

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 41 Exercise 2.1 Problem 4, table 2

Page 42 Exercise 2.2 Problem 1

To find – The sum of

8 + 2

28 + 2

328 + 2

6,328 + 2

Place the digits under respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place values for each sum

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 42 Exercise 2.2 Problem 1 Digit 1

Adding the digits in each place value

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 42 Exercise 2.2 Problem 1 Digit 2

Hence, the addition is ⇒ 8 + 2 = 10 28 + 2 = 30 328 + 2 = 330, and 6,328 + 2 = 6330.

Page 42 Exercise 2.2 Problem 2

To find – The sum of

4 + 6

54 + 6

254 + 6

3,254 + 6

Place the digits under respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place values for each sum

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 42 Exercise 2.2 Problem 2 Digit 1

Adding the digits in each place value,

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 42 Exercise 2.2 Problem 2 Digit 2

Hence, the addition is ⇒ 4 + 6 = 10 , 54 + 6 = 60, 254 + 6 = 260, and 3,254 + 6 = 3260.

Page 42 Exercise 2.2 Problem 3

To find – The sum of 2,457 + 4

Place the digits under the respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place values for each sum

Th H T O
2 4 5 7
+ 4
____________

Adding the digits in each place value

Th H T O
2 4 5 7
+ 4
_________
2 4 6 1

Hence, the addition is ⇒ 2,457 + 4 = 2461.

Page 42 Exercise 2.2 Problem 4

Given: 2,839 + 6

We have to find the sum of these numbers.

Place the digits under respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place value for each sum

Th H T O
2 8 3 9
+ 6
___________

Adding the digits in each place value and taking carry over

Th H T O
2 8 3 9
+ 6
____________
2 8 4 5

Hence, the addition will be ⇒ 2,839 + 6 = 2845.

Page 43 Exercise 2.2 Problem 5

To subtract

10 − 6

40 − 6

250 − 6

5,230 − 6

Place the digits under respective place values.

Beginning with the ones, we go on subtracting place value wise borrowing if necessary, from the next place value to the left.

Referring to the question and placing digits under respective place value for each sum

TO TO HTO ThHTO
10 40 250 5230
−6 −6 −6 −6
_________________________

Subtracting the digits in each place value

TO TO HTO ThHTO
10 40 250 5230
−6 −6 −6 −6
______________________
4 34 244 5224

Hence, the result of subtraction is ⇒ 10 − 6 = 4, 40 − 6 = 34, 250 − 6 = 244, and 5,230 − 6 = 5224.

Page 43 Exercise 2.2 Problem 6

To subtract

10 − 9

100 − 9

1,000 − 9

2,000 − 9

Place the digits under respective place values.

Beginning with the ones, we go on subtracting place value wise, borrowing if necessary, from the next place value to the left.

Referring to the question and placing digits under respective place value for each sum

TO HTO ThHTO ThHTO
10 100 1000 2000
−9 −9 −9 −9
_____________________________

Subtracting the digits in each place value

TO HTO ThHTO ThHTO
10 100 1000 2000
−9 −9 −9 −9
______________________________
1 91 991 1991

Hence, the result of subtraction is ⇒ 10 − 9 = 1, 100 − 9 = 91, 1,000 − 9 = 991 and, 2,000 − 9 = 1991.

Page 43 Exercise 2.2 Problem 7

To subtract – 2,570 − 68.

Place the digits under respective place value.

Beginning with the ones, we go on subtracting place value wise, borrowing if necessary, from the next place value to the left.

Referring to the question and placing digits under respective place value for each sum

Th H T O
2 5 7 0
−6 8
___________

Subtracting the digits in each place value

Th H T O
2 5 7 0
−6 8
_______________
2 5 0 2

Hence, the result of subtraction is 2,570 − 68 = 2,502.

Page 43 Exercise 2.2 Problem 8

To subtract – 3,410 − 9.

Place the digits under respective place value.

Beginning with the ones, we go on subtracting place value wise, borrowing if necessary, from the next place value to the left.

Referring to the question and placing digits under respective place value for each sum

Th H T O
3 4 1 0
−9
____________

Subtracting the digits in each place value

Th H T O
3 4 1 0
−9
____________
3 4 0 1

Hence, the result of subtraction is 3,410 − 9 = 3,401.

Page 44 Exercise 2.3 Problem 1

To add

1,582 + 9

1,582 + 99

1,582+999

Place the digits under respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place value for each sum
Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 44 Exercise 2.3 Problem 1 Digit 1

Subtracting the digits in each place value
Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 44 Exercise 2.3 Problem 1 Digit 2

Hence, the result of addition is ⇒ 1,582 + 9 =1,591, 1,582 + 99 = 1,681 and 1,582 + 999 = 2,581.

Page 44 Exercise 2.3 Problem 2

To add

2,756+8

2,756+98

2,756+998

Place the digits under respective place values.

Add the digits in each place value, and continue this process till we add the digits in all the place values.

Referring to the question and placing digits under respective place value for each sum

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 44 Exercise 2.3 Problem 2 Digit 1

Subtracting the digits in each place value

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.1- 2.7 , Page 44 Exercise 2.3 Problem 2 Digit 2

Hence, the result of addition is ⇒ 2,756 + 8 = 2,764, 2,756 + 98 = 2,854 and 2,756 + 998 = 3,754.

Page 44 Exercise 2.3 Problem 3

Given: 1963 + 98

To find: Add or subtract. By using mathematical operation we can add it or solve it.

First, we perform operation that is given

Adding 1963 and 98 ⇒ 1963 + 98 = 2061

Addition is 1963 + 98 = 2061

Page 44 Exercise 2.3 Problem 4

Given: 7981 − 99

To find – Subtract.By using given operation we can solve it.

Subtracting 99 into 7981 ⇒ 7981 − 99 = 7882

Subtraction is 7981 − 99 = 7882

Page 44 Exercise 2.3 Problem 5

Given: 9405 − 998

To find – Subtract

We can write, 9405 − 998 = 8407 (Subtracting)

Subtraction is 9405− 998 = 8407

Page 44 Exercise 2.3 Problem 6

Given: 3824+997

To find – Find addition.

By using mathematical operation, we can write

3824 + 997 = 4821 (Adding 3824 and 997)

Addition is 3824 + 997 = 4821

Page 44 Exercise 2.3 Problem 7

Given: 6448 + 199

To find – Solve addition.

First, we write, 6448 + 199 = 6647 (Adding 6448 and 199, and simplify)

The addition is 6448 + 199 = 6647

Page 44 Exercise 2.3 Problem 8

Given: 5832 − 598

To find – Subtract the given number.

By using mathematical operation we can solve it.

5832−598 = 5234 (Subtracting 598 from 5832, and simplify)

Subtraction is 5832−598 = 5234

Page 45 Exercise 2.3 Problem 9

Given: 2100 − 80

To find – Subtract.

By using mathematical operation we can solve it.

2100 − 80 = 2020 (Subtracting 80from 2100, and simplify)

Subtraction is 2100 − 80 = 2020

Page 45 Exercise 2.3 Problem 10

Given: 2700 − 98

To find – Subtract 2700 and 98

By using mathematical operation we can solve it.

2700 − 98 = 2602 (Subtracting 98from 2700, and simplify)

Subtraction is 2700 − 98 = 2602

Page 45 Exercise 2.3 Problem 11

Given: 3200 − 54

To find – Subtract the given numbers.

By using mathematical operation we can solve it.

3200−54 = 3146 (Subtracting 54 from 3200, and simplify)

Subtraction is 3200 − 54 = 3146

Page 45 Exercise 2.3 Problem 12

Given: 3900−99

To find – SubtractBy using mathematical operation

3900 − 99 = 3801 (Subtracting 99 from 3900, and simplify)

Subtraction is 3900 − 99 = 3801

Page 45 Exercise 2.3 Problem 13

Given: 4200 − 98

To find – SubtractWe have

4200 − 98 = 4102 (Subtracting 98 from 4200, and simplify)

Subtraction is 4200 − 98 = 4102

Page 45 Exercise 2.3 Problem 14

Given: 3000 − 400

To find – Subtract 400 from 3000

First, we can write

3000−400=2600(Subtracting and simplify)

Subtraction is 3000 − 400 = 2600

Page 45 Exercise 2.3 Problem 15

Given: 3000 − 750

To find – Subtract

We can solve it by using the operation,

3000 − 750 = 2250 (Subtracting 750 from 3000 and simplify)

Subtraction is 3000 − 750 = 2250

Page 45 Exercise 2.3 Problem 16

Given: 3000−530

To find – Subtract

By using mathematical operation we can solve it

3000−530 = 2470 (Subtracting 530from 3000, and simplify)

Subtraction is 3000−530 = 2470

Page 45 Exercise 2.3 Problem 17

Given: 3000−999

To find – Subtract

We have, 3000−999 = 2001 (Subtracting 999 from 3000 , and simplify)

Subtraction is 3000 − 999 = 2001

Page 45 Exercise 2.3 Problem 18

Given: 4000−998

To find – Subtract

We have, 4000 − 998 = 3002 (Subtracting 998 from 4000 , and simplify)

Subtraction is 4000 − 998 = 3002

Page 46 Exercise 2.4 Problem 1

Given: 3890+14

To find – Add 3890 and 14

By using mathematical operation (addition) we can solve it.

We have

3890+14 = 3904 (Add 3890 and 14, and simplify)

Addition is 3890+14 = 3904

Page 46 Exercise 2.4 Problem 2

Given: 3598+42

To find – Add 3598 and 42

By solving mathematical operation we can solve it

3598 + 42 = 3640 (Add 3598 and 42, and simplify)

Addition is 3598 + 42 = 3640

Page 46 Exercise 2.4 Problem 3

Given: 4264 + 38

To find – Add 4264 and 38

We first write given operation and then solve

4264 + 38 = 4302 (Add 4264 and 38, and simplify)

Addition is 4264 + 38 = 4302

Page 46 Exercise 2.4 Problem 4

Given: 2700 + 324

To find – Add 2700 + 324

By using addition we can solve it

2700 + 324 = 3024 (Add 2700 and 324, and simplify)

Addition is 2700 + 324 = 3024

Page 46 Exercise 2.4 Problem 5

Given: 2997+203

To find – Add 2997+203

By using addition we can solve it

2997 + 203 = 3200 (Add 2997 and 203, and simplify)

Addition is 2997 + 203 = 3200

Page 46 Exercise 2.4 Problem 6

Given: 2998 + 275

To find – Add 2998 and 275

By using addition we can solve it

2998 + 275 = 3273 (Add 2998 and 275, and simplify)

Addition is 2998275 = 3273

Page 47 Exercise 2.4 Problem 7

Given: 2543 + 68 =_____

To find – Write the missing numbers.

By using mathematical operations we can solve it

2543 + 60 = 2603

2603 + 8 = 2611

First, we add +60 into 2543 and then we add +8 into 2603

Answer is ⇒ 2543+ 60 = 2603, 2603 + 8 = 2611, 2543 + 68 = 2611

Page 47 Exercise 2.4 Problem 8

Given: 3276 + 2040 = _______

To find – Write the missing numbers.

First, we add 2000 and then we add 40 into 5276

3276 + 2000 = 5276

5276 + 40 = 5316

3276 + 2040 = 5316

Missing numbers are ⇒ 3276 + 2000 = 5276, 5276 + 40 = 5316, 3276 + 2040 = 5316

Page 47 Exercise 2.4 Problem 9

Given: 3524 − 630 = ______

To find – Write the missing number.

By using mathematical operations we can solve.

First, we subtract 600 and then subtract 30 from 3524

3524−600 = 2924

2924−30 = 2894

3524−630 = 2894

Missing numbers are ⇒ 3524 − 600 = 2924, 2924 − 30 = 2894, 3524 − 630 = 2894

Page 47 Exercise 2.4 Problem 10

Given: 4261−2500=?

To find – Write missing numbers.

By using mathematical operations we can solve it.

$4261 \stackrel{-2000}{\longrightarrow} 2261 \stackrel{-500}{\longrightarrow} 1761$

4261- 2500 = 1761

The missing numbers are ⇒ $4261 \stackrel{-2000}{\longrightarrow} 2261 \stackrel{-500}{\longrightarrow} 1761$, $4261-2500$= 1761

Page 47 Exercise 2.4 Problem 11

Given: 3423 − 607

To find – Subtract 607 from 3423

Subtracting 607 from 3423 we get, 3423−607 = 2816

The subtraction is 2816.

Page 47 Exercise 2.4 Problem 12

Given: 5697 − 2700

To find – Subtract 2700 from 5697

Subtracting 2700 from 5697 we get, 5697−2700=2997

Subtraction is 2997.

Page 48 Exercise 2.5 Problem 1

Given: 319 + 589

To find – Round each number to the nearest hundred then estimate it.

First, we round each number to the nearest hundred then we solve it.

319 + 589
↓
300 + 600 = 900

The estimate value of expression is 900.

Page 48 Exercise 2.5 Problem 2

Given: 782 − 509

To find – Round the number to nearest hundred then estimate it.

First, we write round the number to nearest hundred then solve it.

780 − 509
↓ ↓
800 − 500 = 300

The estimate value of expression is 300.

Page 48 Exercise 2.5 Problem 3

Given: 792 + 204

To find – Round the number to nearest hundred then estimate expression.

First, we round the number to nearest hundred then solve.

792 + 204
↓ ↓
800 + 200 = 1000

The estimate value of expression is 1000.

Page 48 Exercise 2.5 Problem 4

Given: 903 − 288

To find – Round the number to nearest hundred then estimate expression.

First, we round the number to nearest hundred then solve.

903 − 288
↓ ↓
900 − 300 = 600

The estimate value of expression is 600.

Page 48 Exercise 2.5 Problem 5

Given: 612 + 589

To find – Round the number to nearest hundred then estimate expression.

First, we round the number to nearest hundred then solve.

612 + 589
↓ ↓
600 + 600 = 1200

The estimate value of expression is 1200.

Page 48 Exercise 2.5 Problem 6

Given: 892−328

To find – Round the number to nearest hundred then estimate expression.

First, we round the number to nearest hundred then estimate expression.

892 − 328
↓ ↓
900 − 300 = 600

The estimate value of expression is 600.

Page 48 Exercise 2.5 Problem 7

Given: 1798 + 416

To find – Round the number to nearest hundred then estimate it.

First, we round the number to nearest hundred then solve.

1798 + 416
↓ ↓
1800 + 400 = 2200

The estimate value of expression is 2200.

Page 48 Exercise 2.5 Problem 8

Given: 2304−996

To find – Round the number to nearest hundred then estimate value of expression.

First, we round the number to nearest hundred then solve.

2304 − 996
↓ ↓
2300 − 1000 = 1300

The estimate value of expression is 1300.

Page 49 Exercise 2.5 Problem 9

Given: 296 + 109 + 394

To find – Round the number to nearest hundred then solve.

First, we round the number to nearest hundred then solve it.

296 + 109 + 394
↓ ↓ ↓
300 + 100 + 400 = 800

The estimate value of expression is 800.

Page 49 Exercise 2.5 Problem 10

Given: 704 − 196 − 312

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

704 − 196 − 312
↓ ↓ ↓
700 − 200 − 300 = 200

The estimate value of expression is 200.

Page 49 Exercise 2.5 Problem 11

Given: 998 − 194 + 97

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

998 − 194 + 97
↓ ↓ ↓
1000 − 200 + 100 = 900

The estimate value of expression is 900.

Page 49 Exercise 2.5 Problem 12

Given: 499 + 301 − 29

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

499 + 301 − 29
↓ ↓ ↓
500 + 300 − 30 = 770

The estimate value of expression is 770.

Page 49 Exercise 2.5 Problem 13

Given: 1992 − 67 + 489

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

2000 − 70 + 500 = 2430

The estimate value of expression is 2430.

Page 49 Exercise 2.5 Problem 14

Given: 2409 + 593 − 708

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

2400 + 600 − 700 = 2300

The estimate value of expression is 2300.

Page 49 Exercise 2.5 Problem 15

Given: 1109 − 98 + 392

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

1100 − 100 + 400 = 1410

The estimate value of expression is 1410.

Page 49 Exercise 2.5 Problem 16

Given: 3012 + 62 + 402

To find – Round the number to nearest hundred then solve it.

First, we round the number to nearest hundred then solve it.

3010 + 70 + 400 = 3480

The estimate value of expression is 3480.

Page 50 Exercise 2.6 Problem 1

Given: 4670

European stamps and 698 African stamps more than European stamps.

To find – Total stamps altogether.

First, we write given stamps and then we add all stamps.

European − Stamps  → African − stamps  → Total−stamps
4670 + 4670 + 698 =

4670 + 5368 = 10038

Sarah have 10038 total stamps.

Page 50 Exercise 2.6 Problem 2

Given: I Drove 325 miles on Saturday.

To find – How many miles did she travels on two days?

First, we add 49 miles to 325 miles for Sunday drove then we calculate for both days drove.

325miles + 49miles = 374miles on Sunday.

For two days travel is:

325miles + 374miles = 699miles

Mrs. Charles did travels on both days are 699 miles.

Page 51 Exercise 2.6 Problem 3

Given: Cost $3225 for Piano.

To find – How much more money does she need?

First, we add total money then we subtract total money from the Piano cost.

Total money, $1950 + $625 = $2575

Now she needs money more

$3225 − $2575 = $650

Mrs. Gina need $650 more money.

Page 51 Exercise 2.6 Problem 4

Given: Earned $2365 in January, $2740 in February.

To find – How much did he saved?

First, we calculate both months earned money then we subtract spend money from earned money.

Earned in January = $2365

Earned in February ($2365+$375) = $2740

Total = $2365 + $2740

= $5105

He spent $4250, Now we calculate saving $5105 − $4250=$855, Mr. Mudley save $855.

Page 52 Exercise 2.7 Problem 1

We are given to estimate and multiply for 82 × 6.

So, the nearest round figure for 82 is 80.

So, the estimation will be 80 × 6 = 480.

Therefore, the estimated product is 80 × 6 = 480.

Page 52 Exercise 2.7 Problem 2

We are given to estimate and multiply for 79 × 6.

So, the nearest round figure for 79 is 80.

So, the estimation will be 80 × 7 = 560.

Therefore, the estimated product is 80 × 7 = 560.

Page 52 Exercise 2.7 Problem 3

We are given to estimate and multiply for 83 × 8.

So, the nearest round figure for 83 is 80.

So, the estimation will be 80 × 8 = 640.

Therefore, the estimated product is 80 × 8 = 640

Page 52 Exercise 2.7 Problem 4

We are given to estimate and multiply for 96 × 9.

So, the nearest round figure for 96 is 100.

So, the estimation will be 100 × 9 = 900.

Therefore, the estimated product is 100 × 9 = 900.

Page 53 Exercise 2.7 Problem 5

We are given to estimate and multiply for 213 × 5.

So, the nearest round figure for 213 is 200.

So, the estimation will be 200 × 5 = 1000.

Therefore, the estimated product is 200 × 5 = 1000.

Page 53 Exercise 2.7 Problem 6

We are given to estimate and multiply for 497 × 4.

So, the nearest round figure for 497 is 500.

So, the estimation will be 500 × 4 = 2000.

Therefore, the estimated product is 500 × 4 = 2000.

Page 53 Exercise 2.7 Problem 7

We are given to estimate and multiply for 706 × 8.

So, the nearest round figure for 706 is 700.

So, the estimation will be 700 × 8 = 5600.

Therefore, the estimated product is 700 × 8= 5600.

Page 53 Exercise 2.7 Problem 8

We are given to estimate and multiply for 898 × 7.

So, the nearest round figure for 898 is 900.

So, the estimation will be 900 × 7 = 6300.

Therefore, the estimated product is 900 × 7 = 6300.

Page 54 Exercise 2.7 Problem 9

We are given to multiply the given numbers:

By multiplying, we get

34 × 4 = 136

47 × 5 = 235

228 × 3 = 684

219 × 6 = 1314

357 × 7 = 2499

285 × 9 = 2565

356 × 8 = 2848

489 × 9 = 4401

Therefore, by multiplying the given numbers we get the below answers.

34 × 4 = 136

47 × 5 = 235

228 × 3 = 684

219 × 6 = 1314

357 × 7 = 2499

285 × 9 = 2565

356 × 8 = 2848

489 × 9 = 4401

Primary Mathematics Workbook 4A Common Core Edition Solutions Chapter 2 The Four Operations Of Whole Numbers Exercises 2.8

March 17, 2023March 15, 2023 by Marksparks

Primary Mathematics Chapter 2 The Four Operations Of Whole Numbers

Page 55 Exercise 2.8 Problem 1

We are given to estimate and multiply for 1893 × 4.

So, the nearest round figure for 1893 is 2000.

So, the estimation will be 2000 × 4 = 8000.

Therefore, the estimated product is 2000 × 4 = 8000.

Page 55 Exercise 2.8 Problem 2

We are given to estimate and multiply for 4036×7.

So, the nearest round figure for 4036 is 4000

So, the estimation will be 4000×7=28000

Therefore, the estimated product is 4000×7=28000.

Page 55 Exercise 2.8 Problem 3

We are given to estimate and multiply for 5987×8.

So, the nearest round figure for 6000 is 5987.

So, the estimation will be 4000 × 7 = 28000.

Therefore, the estimated product is 6000 × 8 = 48000.

Page 55 Exercise 2.8 Problem 4

We are given to estimate and multiply for 8195 × 9.

So, the nearest round figure for 8000 is 8195.

So, the estimation will be 8000 × 9 = 72000.

Therefore, the estimated product is 8000 × 9 = 72000.

Page 56 Exercise 2.9 Problem 1

We are given that the hotel received 1320 stems of flowers each month and it is also given that the hotel received for 6 months.

We have to find the total number of stems of flowers did manager received.

Which is the product of a number of stems received per month and a number of months.

= 1320 × 6

= 7920

Therefore, the total number of flowers that the hotel received altogether is 7920.

Page 56 Exercise 2.9 Problem 2

We are given that a bottle contains red beads and white beads.

The number of red beads is three times the number of white beads.

If there are 1875 red beads then we have to find white beads.

Which is three times of red beads.

= 1875 × 3

= 5625

Therefore, the total number of white beads are 5625.

Page 57 Exercise 2.9 Problem 3

We are given that Mrs Wiley earned $2350 in January.

She earned $500 in February.

In March, she earned twice as much as in February.

We have to find the amount earned by Wiley in March.

Money earned in February is = $2350 + $500 = $2850.

Hence money earned in March is = 2 × $2850 = $5700.

Therefore, the money earned by Wiley in March is $5700.

Page 58 Exercise 2.9 Problem 4

It is given David bought 2 computers at $3569 each.

He had $2907 left with the money.

We are asked how much money he have at first.

The total money that he have at first is the sum of cost of the computers and the remaining amount.

Cost of two computers is = 2 × 3569

= $7138

The total money that he have at first is = 7138 + 2907

= $10045

Therefore, the total money that he have at first is $10045.

Page 58 Exercise 2.9 Problem 5

It is given that 5 people shared a sum of money.

2 of them received Others received

We are asked to find the sum of the money.

Sum of the money is the sum of the two people’s money and the other’s people money.

Two people’s money is = 2 × 4356 = 8712.

Other people’s money is = 3 × 3807 = 11421.

Therefore, total money is = 8712 + 11421 = 20133.

Therefore, the sum of money is $20133.

Page 59 Exercise 2.10 Problem 1

We are given to estimate and then divide for the given numbers.

Given: 292 ÷ 4

We have to round of the large number to the nearest second number’s multiple for estimating.

By rounding off, 292 will become 280.

= 280 ÷ 4 = 70

Therefore, by estimating and dividing the given number, we get 70.

Page 59 Exercise 2.10 Problem 2

We are given to estimate and then divide for the given numbers.

Given: 378 ÷ 6

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 378 will become 360.

= 360 ÷ 6 = 60

Therefore, by estimating and dividing the given number, we get 70.

Page 59 Exercise 2.10 Problem 3

We are given to estimate and then divide for the given numbers.

Given: 651 ÷ 7

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 651 will become 630.

= 630 ÷ 7 = 90

Therefore, by estimating and dividing the given number, we get 90.

Page 59 Exercise 2.10 Problem 4

We are given to estimate and then divide for the given numbers.

Given: 801 ÷ 9

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 801 will become 810.

= 810 ÷ 9 = 90

Therefore, by estimating and dividing the given number, we get 90.

Page 60 Exercise 2.10 Problem 5

We are asked to fill the empty cells in the given table using the answers.

Let us find the quotients and remainders.

After that let us fill the table with the correct divisors.

Let us solve the divisions

For 68 ÷ 4, the remainder is 0 and the quotient is 17.

For 96 ÷ 8 remainder is 0 and the quotient is 12.

For 98 ÷ 7 remainder is 0 and the quotient is 14.

For 635 ÷ 5 remainder is 0 and the quotient is 127.

For 963 ÷ 9 remainder is 0 and the quotient is 107.

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.8- 2.16, Page 60 Exercise 2.10 Problem 5 table 1

Therefore the filled table is

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercises 2.8- 2.16, Page 60 Exercise 2.10 Problem 5 table 2

Page 61 Exercise 2.11 Problem 1

We are given to estimate and then divide for the given numbers.

Given: 2475÷5

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 2475 will become 2500.

= 2500 ÷ 5 = 500

Therefore, by estimating and dividing the given number, we get 500

Page 61 Exercise 2.11 Problem 2

We are given to estimate and then divide for the given numbers.

Given: 3594÷6

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 3594 will become 3600.

= 3600 ÷ 6 = 600

Therefore, by estimating and dividing the given number, we get 600.

Page 61 Exercise 2.11 Problem 3

We are given to estimate and then divide for the given numbers.

Given: 4214 ÷ 7

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 4214 will become 4200.

= 4200 ÷ 7 = 600

Therefore, by estimating and dividing the given number, we get 600.

Page 61 Exercise 2.11 Problem 4

We are given to estimate and then divide for the given numbers.

Given: 6480 ÷ 9

We have to round of the large number to nearest second number’s multiple for estimating.

By rounding off, 6490 will become 6300.

= 6300 ÷ 9 = 700

Therefore, by estimating and dividing the given number, we get 700.

Page 62 Exercise 2.11 Problem 5

We are given to multiple or divide the given numbers.

Multiplication:

4023 × 3 = 12069

2370 × 5 = 11850

3208 × 9 = 28872

7248 × 6 = 43488

Division:

5208 ÷ 4 = 1302

9207 ÷ 8 = 3069

1936 ÷ 8 = 242

2520 ÷ 10 = 252

Therefore, by doing multiplication and division, we get

Multiplication:

4023 × 3 = 12069

2370 × 5 = 11850

3208 × 9 = 28872

7248 × 6 = 43488

Division:

5208 ÷ 4 = 1302

9207 ÷ 8 = 3069

1936 ÷ 8 = 242

2520 ÷ 10 = 252

Page 63 Exercise 2.11 Problem 6

We are given to replace letter n with a number to make equation true.

Given: n × 6 = 6474

To find – The value of n .

Divide both sides by 6, and we get

⇒ $\frac{n \times 6}{6}$ = $\frac{6474}{6}$

⇒ n = 1079

Therefore, value of n is 1079.

Page 63 Exercise 2.11 Problem 7

We are given to replace letter n with a number to make equation true.

Given: n × 7 = 8904.

To find – The value of n .

Divide both sides by 7, and we get

⇒ $\frac{n \times 7}{7}$= $\frac{8904}{7}$

⇒ n = 1272

Therefore, value of n is 1272.

Page 63 Exercise 2.11 Problem 8

We are given to replace letter n with a number to make equation true.

Given: n × 5 = 3290.

To find – The value of n.

Divide both sides by 5, and we get

⇒ $\frac{n \times 5}{5}$= $\frac{3290}{5}$

⇒ n = 658

Therefore, the value of n is 658.

Page 63 Exercise 2.11 Problem 9

We are given to replace letter n with a number to make equation true.

Given: n ÷ 2 = 3845.

To find – The value of n.

Multiply both sides by 2, and we get

$\frac{n \times 2}{2}$= 3845 × 2

⇒ n= 7690

Therefore, the value of n is 7690.

Page 63 Exercise 2.11 Problem 10

We are given to replace letter n with a number to make equation true.

Given: n ÷ 3 = 4095.

To find – The value of n.

Multiply both sides by 3, and we get

$\frac{n \times 3}{3}$ = 4095 × 3

⇒ n = 12285

Therefore, the value of n is 12285.

Page 64 Exercise 2.12 Problem 1

It is given that a baker made meat pies 4

Times the number of vegetable pies.

It is given that there are 4864 meat pies.

We are asked to find how many meat pies are made more than vegetable pies.

For that we have to do number of meat pies – number of vegetable pies.

Let us consider x for vegetable pies.

Hence, from the question

4x = 4864

Or x = $\frac{4864}{4}$= 1216

Number of meat pies made more than vegetable pies are

⇒ 4864 − 1216

⇒ 3648

Therefore, 3648 meat pies are made more than vegetable pies.

Page 64 Exercise 2.12 Problem 2

It is given that Margo sold three times as many pears as apples in a week.

She sold 3456 apples.

We are asked to find the number of pears did she sell.

Let us consider number of pears as x.

From the question

3x = 3456

Or x= $\frac{3456}{3}$

x = 1152

Therefore, 1152 pears are sold.

Page 65 Exercise 2.12 Problem 3

It is given sue, Pat and Jerry shared $387 equally among themselves.

Sue gave $42 and Pat gave $28.

We are asked to find the money did Pat have in the end.

It is given that $387 is shared equally which means

= $\frac{387}{3}$

= 129

Therefore, everyone have $129 with them at start.

Now, Sue gave Pat $42 means

= $129+$42

= 172

And now pat sent $28 back.

= $172 − $28 = 144

Therefore, P at have $144 at the end.

Page 65 Exercise 2.12 Problem 4

It is given that a sum of 1040 was divided into 8 equal parts.

It is given that Alice got 4 parts and bob got 1 part and remaining are shared equally to the other 5 members,

By dividing 8 parts, we get

= $\frac{1040}{8}$

= 130

From the question we can say that Bob gets 130.

The remaining are 3 × 130 = 390.

Now Gloria have $\frac{390}{5}$

= 78

The amount that shared between them is 78 per each.

The number that bob exceeded than Gloria is

= 130 − 78

= 52

Therefore, Bob has 52 more than Gloria.

Page 67 Exercise 2.13 Problem 1

We are given to find the multiplications for the given numbers.

For up:

B − 21 × 13 = 273.

D − 17 × 39 = 663

F − 37 × 24 = 888.

G − 83 × 79 = 6557.

For down:

A − 28 × 31 = 868.

B − 53 × 45 = 2385.

C − 59 × 63 = 3717.

E − 49 × 14 = 686.

Therefore the products of the given numbers will be

For up:

B − 21 × 13 = 273.

D − 17 × 39 = 663

F − 37 × 24 = 888.

G − 83 × 79 = 6557.

For down:

A − 28 × 31 = 868.

B − 53 × 45 = 2385.

C − 59 × 63 = 3717.

E − 49 × 14 = 686.

Page 69 Exercise 2.14 Problem 1

We are given to estimate and multiply for 52 × 39.

So, the nearest round figure for 52 and 39 is 50 and 40 respectively.

So, the estimation will be 50 × 40=2000.

Therefore, the estimated product is 50 × 40 = 2000.

Page 69 Exercise 2.14 Problem 2

We are given to estimate and multiply for 78 × 33.

So, the nearest round figure for 78 and 33 is 80 and 30 respectively.

So, the estimation will be 80 × 30 = 2400.

Therefore, the estimated product is 80 × 30 = 2400.

Page 69 Exercise 2.14 Problem 3

We are given to estimate and multiply for 29 × 87.

So, the nearest round figure for 29 and 87 is 30 and 90 respectively.

So, the estimation will be 30 × 90 = 2700.

Therefore, the estimated product is 30 × 90 = 2700

Page 69 Exercise 2.14 Problem 4

We are given to estimate and multiply for 92 × 71.

So, the nearest round figure for 92 and 71 is 90 and 70 respectively.

So, the estimation will be 90 × 70 = 6300.

Therefore, the estimated product is 90 × 70 = 6300.

Page 70 Exercise 2.14 Problem 5

We are given to estimate and multiply for 218 × 37.

So, the nearest round figure for 218 and 37 is 200 and 40 respectively.

So, the estimation will be 200 × 40 = 8000.

Therefore, the estimated product is 200 × 40 = 8000.

Page 70 Exercise 2.14 Problem 6

We are given to estimate and multiply for 483×59.

So, the nearest round figure for 483 and 59 is 500 and 60 respectively.

So, the estimation will be 500 × 60 = 30000.

Therefore, the estimated product is 500 × 60 = 30000.

Page 70 Exercise 2.14 Problem 7

We are given to estimate and multiply for 372 × 64.

So, the nearest round figure for 372 and 64 is 400 and 60 respectively.

So, the estimation will be 400 × 60 = 24000.

Therefore, the estimated product is 400 × 60 = 24000.

Page 70 Exercise 2.14 Problem 8

We are given to estimate and multiply for 648 × 78.

So, the nearest round figure for 648 and 78 is 600 and 80 respectively.

So, the estimation will be 600 × 80 = 48000.

Therefore, the estimated product is 600 × 80 = 48000.

Page 71 Exercise 2.15 Problem 1

We are asked to find the value of 36 × 9.

Which means 9 times of 36.

So, now let us find 10 times of 36 and then we can subtract 36 from it.

= 36 × 10 − 36

= 360 − 36

= 324

Therefore, the product of 36 × 9 is 324.

Page 71 Exercise 2.15 Problem 2

We are asked to find the value of 58 × 99.

Which means 99 times of 58.

So, now let us find 100 times of 58 and then we can subtract 58 from it.

= 58 × 100 − 58

= 5800 − 58

= 5742

Therefore, the product of 58 × 99 is 5742.

Page 71 Exercise 2.15 Problem 3

We are asked to find the value of 69×99

Which means 99 times of 69.

So, now let us find 100 times of 69 and then we can subtract 69 from it.

= 69 × 100 − 69

= 6900 − 69

= 6831

Therefore, the product of 69 × 99 is 6831.

Page 71 Exercise 2.15 Problem 4

We are asked to find the value of 87×99.

Which means 99 times of 87.

So, now let us find 100 times of 87 and then we can subtract 87 from it.

= 87 × 100 − 87

= 8700 − 87

= 8613

Therefore, the product of 87 × 99 is 8631.

Page 71 Exercise 2.15 Problem 5

We are asked to find the value of 68 × 50.

Which means 68 times of 50.

Now let us make 50 as 100 by rewriting 68 as 34×2.

We get

= 34 × 2 × 50

= 34 × 100

= 3400

Therefore, the product of 68 × 50 is 3400.

Page 71 Exercise 2.15 Problem 6

We are asked to find the value of 32 × 25.

Which means 32 times of 25.

Now let us make 25 as 100 by rewriting 32 as 4 × 8.

We get

= 8 × 4 × 25

= 8 × 100

= 800

Therefore, the product of 32 × 25 is 800.

Page 71 Exercise 2.15 Problem 7

We are asked to find the value of 84 × 25.

Which means 84 times of 25.

Now let us make 25 as 100 by rewriting 84 as 4 × 21.

We get

= 21 × 4 × 25

= 21 × 100

= 2100

Therefore, the product of 84 × 25 is 2100.

Page 71 Exercise 2.15 Problem 8

We are asked to find the value of 25 × 56 .

Which means 56 times of 25.

Now let us make 25 as 100 by rewriting 84 as 4 × 14.

We get

= 14 × 4 × 25

= 14 × 100

= 1400

Therefore, the product of 25 × 56 is 1400.

Page 72 Exercise 2. 15 Problem 9

We are asked to answer for the given multiplications.

Across:

118 × 23 = 2714

249 × 31 = 7719

329 × 18 = 5922

167 × 17 = 2839

138 × 11 = 1518

249 × 25 = 6225

Down:

895 × 31 = 27745

676 × 62 = 7719

346 × 28 = 9688

406 × 53 = 21518

119 × 29 = 3451

135 × 65 = 8775

Therefore, the product of the given numbers is:

Across:

118 × 23 = 2714

249 × 31 = 7719

329 × 18 = 5922

167 × 17 = 2839

138 × 11 = 1518

249 × 25 = 6225

Down:

895 × 31 = 27745

676 × 62 = 7719

346 × 28 = 9688

406 × 53 = 21518

119 × 29 = 3451

135 × 65 = 8775

Page 73 Exercise 2.16 Problem 1

It is given that Natalie made 14 jars of butter biscuits and 16 jars of jam biscuits.

And it is also given that they are 48 biscuits in each jar.

We have to find the total number of biscuits she made which is equal to product of number of jars and number of biscuits.

The total number of jars is

14 + 16 = 30

Therefore, the total no of biscuits are 30 × 48 = 1440.

Therefore, Natalie made 1440 biscuits altogether.

Page 73 Exercise 2.16 Problem 2

It is given that William bought 12 packets of yoghurt.

Each pack contains 465ml of yoghurt. He used 2500ml of yoghurt.

We have to find the remaining yoghurt.

The remaining yoghurt is equal to subtraction of total yoghurt and used yoghurt.

Total yoghurt is

= 12 × 465

= 5580

Therefore, the remaining is

= 5580 − 2000

= 3580ml

Therefore, 3580ml of yoghurt is left after usage.

Primary Mathematics Workbook 4A Common Core Edition Solutions Chapter 2 The Four Operations Of Whole Numbers Exercise 2.17

March 17, 2023March 15, 2023 by Marksparks

Primary Mathematics Chapter 2 The Four Operations Of Whole Numbers

Page 74 Exercise 2.17 Problem 1

Given: Holly’s savings = 312

Leigh’s savings = 998

To find – How much Holly saved more than Leigh.

Here, we have the subtracted Leigh’s savings from Holly’s savings to find how much more was saved by Holly

Amount saved = Holly’s savings – Leigh’s savings

⇒ 10312 − 7998

⇒ 2314

Holly saved more than Leigh.

Page 74 Exercise 2.17 Problem 2

Given: To find the quotient and remainder when 2490 is divided by 4.

Let’s divide 2490 by 4

Primary Mathematics Workbook 4A Common Core Edition Chapter 2 The Four operations Of Whole Numbers Exercise 2.17 Page 74 Exercise 2.17 Problem 2

Quotient = 622

Remainder = 2

When 2490 is divided by 4

Quotient = 622

Remainder = 2

Page 74 Exercise 2.17 Problem 3

Given: 1548,397,621

First round off the numbers to its nearest hundred.

Then, add all the numbers.

Rounding off 1548 to its nearest hundred = 1500

Rounding off 397 to its nearest hundred = 400

Rounding off 621 to its nearest hundred = 600

Now, add all the rounded numbers.

⇒ 1500 + 400 + 600

⇒ 2500

Required estimate = 2500

Page 74 Exercise 2.17 Problem 4

Given: 459,24

First, find the product of the given numbers

Then, round it off to the nearest ten.

Product of 459,24:

⇒ 459 × 24

⇒ 11016

Now, rounding off to the nearest ten.

⇒ 11020

Required estimate ⇒ 11020

Page 74 Exercise 2.17 Problem 5

Given: To subtract 238 from the product of 23 and 80

First, find the product of 23 and 80

Then subtract 238 from the product.

Product of 23 and 80 :

⇒ 23 × 80

⇒ 1840

Now, subtract 238 from the product of 23 and 80.

⇒ 1840−238

⇒ 1602

Required answer = 1602

Page 75 Exercise 2.17 Problem 6

Given: Divide the sum of 352 and 698 by 5

First, find the sum of 352 and 698

Then divide by 5

Sum of 352 and 698

⇒ 352 + 698

⇒ 1050

Now, divide by 5

⇒ $\frac{1050}{5}$

⇒ 210

Required answer = 210

Page 75 Exercise 2.17 Problem 7

Given: 4345 + 998 ◯ 5345 − 98

To fill – The circle with either <,> or =.

First, find the left-hand side (LHS) of the circle, and then find the right-hand side (RHS) of the circle.

If LHS is greater than RHS, use >

If LHS is smaller than RHS, use <

If LHS is equal than RHS, use =

LHS:

⇒ 4345 + 998

⇒ 5343

RHS:

⇒ 5345 − 98

⇒ 5247

Here, LHS is greater than RHS. 5343 is greater than 5247

Therefore, 5343>5247

The required solution is 4345+998>5345−98

Page 75 Exercise 2.17 Problem 8

Given: (600 × 8) + (5 × 8) + (3 × 8) ◯ 654 × 8

To fill – The circle with either <,> or =.

First, find the left-hand side (LHS) of the circle, and then find the right-hand side (RHS) of the circle.

If LHS is greater than RHS, use >

If LHS is smaller than RHS, use <

If LHS is equal than RHS, use =

LHS:

First, multiply the terms in the bracket and then add.

⇒ (600 × 8) + (5 × 8) + ( 3 × 8)

⇒ (4800) + (5 × 8) + (3 × 8)

⇒ (4800) + (40) + (3 × 8)

⇒ (4800) + (40) + (24)

⇒ 4864

RHS:

⇒ 654 × 8

⇒ 5232

Here, LHS is smaller than RHS.

4864 is smaller than 5232

Therefore, 4864<5232

The required solution is (600 × 8) + (5 × 8) + (3 × 8)< 654 × 8

Page 75 Exercise 2.17 Problem 9

Given: 7191 ÷ 9 ◯ 5994 ÷ 6

To fill – The circle with either <,> or =.

First, find the left-hand side (LHS) of the circle, and then find the right-hand side (RHS) of the circle.

If LHS is greater than RHS, use >

If LHS is smaller than RHS, use <

If LHS is equal than RHS, use =

LHS:

⇒ $\frac{7191}{9}$

⇒ 799

RHS:

⇒ 5994 ÷ 6

⇒ 999

Here, LHS is smaller than RHS.

799 is smaller than 999

Therefore, 799<999

The required solution is 7191 ÷ 9 < 5994 ÷ 6

Page 75 Exercise 2.17 Problem 10

Given: 605 × 40 ◯ 505 × 30

To fill – The circle with either <,> or =.

First, find the left-hand side (LHS) of the circle, and then find the right-hand side (RHS) of the circle.

If LHS is greater than RHS, use >

If LHS is smaller than RHS, use <

If LHS is equal than RHS, use =

LHS:

⇒ 605 × 40

⇒ 24200

RHS:

⇒ 505 × 30

⇒ 15150

Here, LHS is greater than RHS.

24200 is greater than 15150

Therefore, 24200>15150

The required solution is 605 × 40 > 505 × 30

Page 75 Exercise 2.17 Problem 11

Given: 1000−750 + 480 ÷ 3 =

To find – The value of the given expression.

By BODMAS, first, divide the expression.

Then add and subtract.

First, divide the expression.

⇒ 1000 − 750 + 480 ÷ 3

⇒ 1000−750 + 160

Now add all the same sign terms.

⇒ 1160 − 750

⇒ 410

The required solution is 1000 − 750 + 480 ÷ 3 = 410

Page 75 Exercise 2.17 Problem 12

Given: 9000−(6000−1430)

To find – The value of the given expression.

By BODMAS, first, subtract the expression in the bracket.

Then add and subtract.

First, subtract the expression in the bracket.

⇒ 9000 − (6000 − 1430)

⇒ 9000 − (4570)

Now subtract the terms.

⇒ 9000 − 4570

⇒ 4430

The required solution is 9000 −(6000 −1430) = 4430

Page 75 Exercise 2.17 Problem 13

Given: 1475−(18×21)

To find – The value of the given expression.

By BODMAS, first, multiply the expression inside brackets.

Then subtract.

First, multiply the expression inside brackets.

⇒ 1475 − (18 × 21)

⇒ 1475 − (378)

Now subtract the terms.

⇒ 1475 − 378

⇒ 1097

The required solution is 1475 − (18 × 21) = 1097

Page 75 Exercise 2.17 Problem 14

Given: 40 + 13 × (12 + 6)=

To find – The value of the given expression.

By BODMAS, first, add the expression in the brackets.

Then multiply and add.

First, add the expression in the brackets.

⇒ 40 + 13 × (12 + 6)

⇒ 40 + 13 × (18)

Now multiply the last two terms.

⇒ 40 + 234

⇒ 274

Therefore,40 + 13 × (12 + 6) = 274

Page 75 Exercise 2.17 Problem 15

Given: 30 × (40 + 50)

To match the correct expression.

Here, 30 is multiplied with both 40and50

The above expression can be rewritten as ([30×40]+[30×50])

Therefore, the correct match is the price of forty 30-cent red pencils and fifty 30-cent blue pencils.

(Here ‘and’ indicate addition)

Hence,30×(40+50): The price of forty 30-cent red pencils and fifty 30-cent blue pencils.

Page 75 Exercise 2.17 Problem 16

Given: 30 + 40 × 50

To match the correct expression.

Here, 40 is multiplied 50 and add 30

The above expression can be rewritten as ([1×30]+[40×50])

Therefore, the correct match is the price of one 30-cent pencils and fifty 40-cent erasers. (Here ‘and’ indicates addition)

30+40×50: The price of one 30-cent pencil and fifty 40-cent erasers.

Page 75 Exercise 2.17 Problem 17

Given: 30 × 40 + 50

To match the correct expression.

Here, 30 is multiplied with 40 and then 50 is added.

The above expression can be rewritten as ([30×40]+[50×1])

Therefore, the correct match is the price of forty 30-cent red pencils and a 50-cent eraser. (Here ‘and’ indicate addition)

30+40×50: The price of forty 30-cent red pencils and a 50-cent eraser.

Page 76 Exercise 2.17 Problem 18

Given: Lucas made 1192 muffins. He wants to put them in bags that can hold 6 muffins each.

To find – The least number of bags he needs.

Here, we have to find how 1192 muffins can be divided into 6 muffins in each bag.

Here, divide 1192 by 6

⇒ $\frac{1192}{6}$

⇒ 198.67

So, from the above calculation, we can conclude that

Least number of bags needed =198

Therefore, the least number of bags needed = 198

Page 76 Exercise 2.17 Problem 19

Given: A computer costs $1857.

It costs 3 times as much as a printer.

To find – How much the computer and the printer cost altogether.

Let the cost of the printer be x

Here, the cost of the computer $1857 is 3 times the cost of the printer.

∴ 1857 = 3x

Dividing both sides by 3

x = $\frac{1857}{3}$

x = 619

Therefore, the cost of the printer is $619

Page 77 Exercise 2.17 Problem 20

Given: Nicole and Tasha have 2000 stickers altogether. Nicole has 600 more stickers than Tasha.

To find – Number of stickers Nicole has.

Let x be the number of stickers Nicole has.

Let y be the number of stickers Tasha has.

Nicole has 600 more stickers than Tasha.

Therefore, the number of Nicole stickers = 600+ number of Tasha stickers.

⇒ x = 600 + y

Nicole and Tasha have 2000 stickers altogether.

Number of Nicole’s stickers + Number of Tasha’s stickers = 2000

x + y = 2000

Substitute x = 600 + y in the above equation.

600 + y + y = 2000

600 + 2y = 2000

2y = 2000 − 600

2y = 1400

y = 700

Therefore, the number of stickers Nicole have x = 600 + 700 = 1300

Therefore, the number of stickers Nicole have are = 1300

Page 77 Exercise 2.17 Problem 21

Given: 2500 people took part in a cross-country race.

The number of adults was 4 times the number of children. There were 1200 men.

To find – The number of women.

Let x be the number of adults.

Let y be the number of children

2500 people took part in a cross-country race. Therefore, number of adults + number of children = 2500

x + y = 2500……. (1)

The number of adults was 4 times the number of children. ⇒ x = 4y ……….(2)

Substitute 2 in (1)

x + y = 2500

4y + y = 2500

5y = 2500

y = 500

Therefore, number of adults ⇒ x = 4y = 4(500) = 2000

Number of men +number of women=number of adults

⇒ 1200 + Number of women = 2000

⇒ Number of women = 2000−1200

⇒ Number of women = 800

Therefore, the number of women are = 800

Page 78 Exercise 2.17 Problem 22

Given: the cost of a stereo set and a television set was shared equally among 4 people.

The television set cost $1980

The stereo set cost $1200 more than the television set.

To find – How much each person should pay.

Let the cost of the stereo set be x

The television set cost = $1980

The stereo set cost $1200 more than the television set.

Therefore, cost of stereo set = $1200 + $1980

⇒ x = 1200 + 1980

⇒ x = 3180

The total cost of stereo set and television:

⇒ 1980 + 3180

⇒ 5160

The cost of a stereo set and a television set was shared equally among 4 people.

∴ Amount each person should pay =$\frac{5160}{4}$

= 1290

Therefore the amount each person should pay is = $1290

Page 78 Exercise 2.17 Problem 23

Given: Jared bought a table and 12 chairs for $2400

Each chair cost $165

Let the cost of a table be x

Let the cost of a chair be y

To find – The cost of a table.

Jared bought a table and 12 chairs for $ 2400.

⇒ x + 12y = 2400

Each chair cost

Therefore, y = 165

⇒ x + 12(165) = 2400

⇒ x + 1980 = 2400

⇒ x = 2400−1980

⇒ x = 420

Therefore, the cost of a table is $ 420

Page 79 Exercise 2.17 Problem 24

Given: A shopkeeper has 50 boxes of apples. There were 24 apples in each box.

He sold all the apples for $1.

To find – How much money he received.

A shopkeeper has 50 boxes of apples.

There were 24 apples in each box.

Therefore, the total number of apples = 50 × 24 = 1200

He sold the apples for $1.

Therefore, the money he received = $1200

Therefore, the money he received is = $1200

Page 79 Exercise 2.17 Problem 25

Given: A greengrocer had 25 crates of grapefruit.

There were 36 grapefruit in each crate.

She threw away 28 rotten grapefruit and sold 786 of the rest.

To find – The number of grapefruit left.

First, find the total number of grapefruits.

Then subtract the number of rotten grapefruit and sold fruit from the total to find the number of grapefruit left.

A greengrocer had 25 crates of grapefruit.

There were 36 grapefruit in each crate.

Total number of grapefruit = 25 × 36 = 900

She threw away 28 rotten grapefruit and sold 786 of the rest.

⇒ 900 − 28 − 786

⇒ 900 − 814

⇒ 86

Therefore, the number of grapefruit left is = 86

Page 80 Exercise 2.17 Problem 26

Given: There are 14 blocks of apartments in an estate.

There are 25 floors in each block of apartments. There are 4 apartments on each floor.

To find – The number of apartments altogether.

Using the given information, the total number of apartments is equal to

⇒ 14 × 25 × 4

= 1400

Therefore, the total number of apartments = 1400

Primary Mathematics Workbook 4A Common Core Edition Solutions Chapter 1 Whole Numbers Exercises 1.13

March 24, 2023March 14, 2023 by Marksparks

Primary Mathematics Chapter 1 Whole Numbers

Page 35 Exercise 1.13 Problem 1

Given: 14 thousands 6 tens.

To write: The sentence in the standard form.

Use the strategy of BODMAS to simplify the thousands and tens separately.

Then add both tens and thousands with standard form.

Solve the sentence using parentheses wherever necessary.

Given sentence is written as

14 thousands = 14 × 1000

= 14000

6 tens = 6 × 10 = 60

Further adding both the solutions

14 thousands + 6 tens = 14000 + 60

= 14 × 1000 + 6 × 10

= (14 × 1000) + (6 × 10)

= 14060

Therefore the standard form is 14060.

Hence, the standard form of 14 thousands and 6 tens is 14060.

Page 35 Exercise 1.13 Problem 2

Given: 32 thousands 5 hundreds 2 tens.

To write: The sentence in the standard form.

Use the strategy of BODMAS to simplify the thousands, hundreds, and tens separately.

Then add tens, hundreds, and thousands with standard form.

Solve the sentence using parentheses where ever necessary.

Given sentence is written as

32 thousands = 32 × 1000

= 32000

5 hundreds = 5 × 100

= 500

2 tens = 2 × 10

= 20

Further adding the standard forms

32 thousands + 5 hundreds + 2 tens = 32000 + 500 + 20

= 32 × 1000 + 5 × 100 + 2 × 10

=(32×1000) + (5 × 100) + (2 × 10)

= 32520

Therefore the standard form is 32520.

Hence, the standard form of 32 thousands, 5 hundreds, and 2 tens is 32520.

Page 35 Exercise 1.13 Problem 3

Given: 4 hundred thousands 6 tens 9 ones

To write: The sentence in the standard form.

Use the strategy of BODMAS to simplify the hundred thousands, ones, and tens separately

Then add tens, hundred thousands, and ones with standard form.

Solve the sentence Using parentheses wherever necessary.

Given sentence is written as

4 hundred thousands = 4 × 100 × 1000

9 ones = 9 × 1

6 tens = 6 × 10

Further adding the standard forms

4 hundred thousands+6 tens+9 ones=4×100×1000+6×10+9×1

=(4 × 100 × 1000) + (6 × 10) + (9 × 1)

Therefore the standard form is (4 × 100 × 1000) + (6 × 10) + (9 × 1)

Hence, the standard form of 4 hundred thousands , 6 tens, and 9 ones is (4 × 100 × 1000) + (6 × 10) + (9 × 1)

Page 35 Exercise 1.13 Problem 4

Given: 55 thousands 3 hundred 82

To write: The sentence in the standard form.

Use the strategy of BODMAS to simplify the hundred , thousands, ones separately

Then add tens, hundred, thousands, and ones with standard form.

Solve the sentence Using parentheses wherever necessary.

Given sentence is written as

55 thousands = 55 × 1000

3 hundred = 3 × 100

Eighty two = 82

Further adding the standard forms

55 thousands + 3 hundred + 82 = 55 × 1000 + 3 × 100 + 82

= (55 × 1000) + (3 × 100) + 82

Therefore the standard form is (55 × 1000) + (3 ×1 00) + 82

Hence, the standard form of 55 thousands 3 hundred and 82 is (55 × 1000) + (3 × 100) + 82

Page 35 Exercise 1.13 Problem 5

Given: 2 hundred thousands Twelve

To write: The sentence in the standard form.

Use the strategy of BODMAS to simplify the hundred thousand and twelve separately.

Then add twelve, hundred thousand with standard form.

Solve the sentence Using parentheses wherever necessary.

Given sentence is written as

2 hundred thousands = 2 × 100 × 1000

Twelve = 12

Further adding the standard forms

2 hundred thousands+twelve = 2 × 100 × 1000 + 12

= (2 × 100 × 1000) + 12

Therefore the standard form is (2 × 100 × 1000) + 12

Hence, the standard form of 2 hundred thousands twelve is (2 × 100 × 1000) + 12

Page 35 Exercise 1.13 Problem 6

Given: 12, 025

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses where ever necessary.

Expand the given number

12025 = 12000 + 25

= (12 × 1000) + 25

Further writing the number in expanded sentence forms as words

12025 = (12 × 1000) + 25

= Twelve thousand twenty-five

Therefore the expanded form is twelve thousand twenty-five

Hence, the expanded form of 12025 in words is twelve thousand twenty-five

Page 35 Exercise 1.13 Problem 7

Given:  500,006

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses where ever necessary.

Expand the given number

500,006 = 500000 + 6

= (5 × 100 × 1000) + 6

Further writing the number in expanded sentence forms as words

500,006 = (5 × 100 × 1000) + 6

= Five hundred thousand six

Therefore the expanded form is five hundred thousand six

Hence, the expanded form of 500,006 in words is five hundred thousand six

Page 35 Exercise 1.13 Problem 8

Given: 34,120.

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately.

Then write the number in the sentence form as words in expanded form.

Solve the sentence using parentheses wherever necessary.

Expand the given number

34120 = 30000 + 4000 + 100 + 20

= 3 × 10,000 + 4 × 1000 + 1 × 100 + 2 × 10

Further writing the number in expanded sentence forms as words

34120 = 3 × 10,000 + 4 × 1000 + 1 × 100 + 2 × 10

= Thirty four thousand one hundred twenty

Therefore the expanded form is thirty-four thousand one hundred twenty

Hence, the expanded form of 34120 is 3 × 10,000 + 4 × 1000 + 1 × 100 + 2 × 10. In words it will bethirty four thousand one hundred twenty.

Page 36 Exercise 1.14 Problem 1

Given: 385,270

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses wherever necessary.

Check for the digit to fill the blank.

Expand the given number

385,270 = 300,000 + 85000 + 200 + 70

= 3 × 100 × 1000 + 85 × 1000 + 2 × 100 + 70

= (3 × 100 × 1000) + (8 × 10 × 000) + (5 × 1000) + (2 × 100) + 70

Further writing the number in expanded sentence forms as words

385,270 = (3 × 100 × 1000) + (8 × 10 × 000) + (5 × 1000) + (2 × 100) + 70

= Three hundred thousand eight-ten thousands five thousand two hundreds seventy
Referring to the expanded form the digit 8 in 385,270 stands for (8×10×000) that is eight-ten thousands

Hence, the digit 8 in 385,270 stands for (8×10×000) that is eight-ten thousands

Page 36 Exercise 1.14 Problem 2

Given: 396,048

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses wherever necessary.

Check for the digit to fill the blank.

Expand the given number

396,048 = 300,000 + 96000 + 40 + 8

= 3 × 100 × 1000 + 96 × 1000 + 40 + 8

= (3 × 100 × 1000) + (96 × 1000) + ( 4 × 10) + 8

Further writing the number in expanded sentence forms as words

396,048 = (3 × 100 × 1000) + (96 × 1000) + (4 × 10) + 8

= Three hundred thousands ninety-six thousands four tens eight

Referring to the expanded form the digit 3 in 396,048 stands for (3 × 100 × 000) that is three hundred thousands

Hence, the digit 3 in 396,048 stands for (3 × 100 × 000) that is three hundred thousands

Page 36 Exercise 1.14 Problem 3

Given: 98,406

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses wherever necessary.

Check for the digit to fill the blank.

Expand the given number

98,406 = 90000 + 8000 + 400 + 6

= 9 × 10 × 1000 + 8 × 1000 + 400 + 6

= (9 × 10 × 1000) + (8 × 1000) + (4 × 100) + 6

Further writing the number in expanded sentence forms as words

98,406=(9 × 10 × 1000) + (8 × 1000) + (4 × 100) + 6

= Nine ten thousands eight thousands four hundreds six

Referring to the expanded form of 98,406 the blank is 8000 or (8 × 1000)

Hence, the missing number of the number 98,406 is 8000 or (8 × 1000)

Page 36 Exercise 1.14 Problem 4

Given: 10,501

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses wherever necessary.

Check for the digit to fill the blank.

Expand the given number

10,501= 10000 + 500 + 1

= 10 × 1000 + 5 × 100 + 1

= (10 × 1000) + (5 × 100) + 1

Further writing the number in expanded sentence forms as words

10,501= (10 × 1000) + (5 × 100) + 1

= 10,501

= Ten thousands five hundreds and one

Referring to the expanded form the blank is 10,501

Hence, the missing number is 10,501

Page 36 Exercise 1.14 Problem 5

Given: 67,014

To write: The sentence in the expanded form.

Use the strategy of BODMAS to expand the hundreds thousands tens and ones separately

Then write the number in the sentence form as words in expanded form

Solve the sentence Using parentheses wherever necessary.

Check for the digit to fill the blank.

Expand the given number

67014 = 67000 + 14

= 67 × 1000 + 14

Further writing the number in expanded sentence forms as words

67014 = (67 × 1000) + 14

= Sixty seven thousands fourteen

Referring to the expanded form of 67014 the blank is 67000 or (67 × 1000)

Hence, the missing number of the number 67014 is 67000 or (67 × 1000)

Page 36 Exercise 1.14 Problem 6

Given: 10,000 more than 46,952 is _____.

Question is to fill the blank with the number which is 10,000 more than 46,952

So, by adding 10,000 to 46,952 will give the required number.

⇒ 46,952 + 10,000 = 56,952

Hence the required number is obtained.

Therefore, it is found that 10,000 more than 46,952 is 56,952.

Page 36 Exercise 1.14 Problem 7

Given:100,000 less than 999,998 is____.

Question is to fill the blank with the number which is 100,000 lesser than 999,998

So, finding difference between 999,998 and 100,000 will give the required number.

⇒ 999,998−100,000 = 899,998

Hence the required number is obtained.

Therefore, it is found that 100,000 less than 999,998 is 899,998.

Page 36 Exercise 1.14 Problem 8

Given: 79,049 is ____ less than 80,049

Question is to fill the blank with the number whose difference from 80,049 gives 79,049

So, finding difference will give

80,049−x = 79,049

x = 80,049 − 79,049

x = 1000

Hence the required number is obtained.

Therefore, it is found that 79,049 is 1000 less than 80,049.

Page 36 Exercise 1.14 Problem 9

Given: 300,561 is _____more than 290,561

Question is to fill in the blank with the number whose addition to 290,561 will make it 300,561

So, adding x with 290,561 will give 300,561

290,561 + x = 300,561

x = 300,561 − 290,561

x = 10,000

Hence the required number is obtained.

Therefore, it is found that 300,561 is 10,000 more than 290,561.

Page 36 Exercise 1.14 Problem 10

Given: A number pattern _____ ; 48,615 ; 58,615 ; ______ ; 78,615

Question is to fill the blanks according to the pattern.

Identify the sequence that which mathematical operation is carried out through out the pattern and accordingly solve it.

The difference between the third number and second number can be found by 58,615 − 48,615 = 10,000

This difference must be the common factor in this sequence.

So, the sequence is designed in a manner that the succeeding number will be 10,000 more than the preceding number.

So, the first term will be 10,000 Less than the second term.

⇒ 48,615−10,000 = 38,615

And the fourth term will be 10,000 more than the third.

⇒ 58,615 + 10,000 = 68,615

Then, the pattern will be, 38,615; 48,615; 58,615; 68,615; 78,615

Therefore, the given number pattern is completed as 38,615; 48,615; 58,615;68,615; 78,615.

Page 36 Exercise 1.14 Problem 11

Given: A number pattern 103,840 ; 102,840 ; 101,840; _____ ; _______ ; _______

Question is to fill the blanks according to the pattern.

Identify the sequence that which mathematical operation is carried out through out the pattern and accordingly solve it.

The difference between the first number and second number can be found by

⇒ 103,840−102,840 = 1000

Similarly, the difference between the first number and second number can be found by

102,840 − 101,840 = 1000

So, the common difference is 1000

So, the sequence is designed in a manner that the succeeding number will be 1000 less than the preceding number.

So, the fourth term will be less than the third term.

101,840 − 1000 = 100,840

The third term will be less than the fourth term.

100,840 − 1000 = 99,840

And, the fourth term will be less than the sixth term.

99,840 − 1000 = 98,840

Then, the pattern will be, 103,840;102,840;101,840; 100,840;99,840; 98,840

Therefore, the given number pattern is completed as 103,840;102,840;101,840; 100,840; 99,840; 98,840.

Page 36 Exercise 1.14 Problem 12

Use the strategy of BODMAS wherever necessary.

Check LHS = RHS

Solve LHS and RHS by expanding them to thousands, hundreds, tens, and ones to identify the inequality.

Compare LHS and RHS to find the answer/solution.

Expand the numbers to identify the inequality

LHS = 85928

= 80000 + 5000 + 900 + 20 + 8

RHS = 85892

= 80000 + 5000 + 800 + 90 + 2

Further comparing LHS = RHS

Clearly, the hundreds place of 85928 is greater than 85892

So, 85928>85892

Hence, by comparing the both the numbers we conclude that 85928>85892

Page 36 Exercise 1.14 Problem 13

Use the strategy of BODMAS wherever necessary.

Check LHS = RHS

Solve LHS and RHS by expanding them to thousands, hundreds, tens, and ones to identify the inequality.

Compare LHS and RHS to find the answer/solution.

Expand the numbers to identify the inequality

LHS = 630,109

= 600,000 + 30,000 + 100 + 9

RHS = 603,901

= 600,000 + 3000 + 900 + 1

Further comparing LHS = RHS

Clearly, the thousands place of 630,109 is greater than 603,901

So, 630,109>603,901

Hence, by comparing the both the numbers we conclude that 630,109>603,901

Page 36 Exercise 1.14 Problem 14

Given data: 4569 − 999____3569 + 999

To write: <,> or = in each blank.

Calculate the L.H.S (left-hand side) of the blank as shown below

∴ 4569−999 = 3570

Again calculate the R.H.S (right-hand side) of the blank as shown below

∴ 3569 + 999 = 4568

It can be clearly observed that the term on R.H.S is greater than the term on the L.H.S and therefore the sign to be used is <.

Thus, it can be clearly concluded that 4569 − 999 < 3569 + 999.

Page 36 Exercise 1.14 Problem 15

Given data: 54100_____ 541 × 1000

To write: <,> more = in each blank.

Calculate the L.H.S (left-hand side) if necessary, of the blank as shown below

∴ 54100

Again calculate the R.H.S (right-hand side) of the blank as shown below

∴ 541 × 1000 = 541000

It can be clearly observed that the term on R.H.S is greater than the term on the L.H.S and therefore the sign to be used is <.

Thus, it can be clearly concluded that 54100<541 × 1000.

Page 36 Exercise 1.14 Problem 16

Given data: 42000 ÷ 7 ____ 3600 ÷ 6

To write: <,> or = in each blank.

Calculate the L.H.S (left-hand side) if necessary, of the blank as shown below

∴ 42000 ÷ 7 = 6000

Again calculate the R.H.S (right-hand side) of the blank as shown below

∴ 3600 ÷ 600

It can be clearly observed that the term on L.H.S is greater than the term on the R.H.S and therefore the sign to be used is >.

Thus, it can be clearly concluded that 42000 ÷ 7 > 3600 ÷ 6.

Page 36 Exercise 1.14 Problem 17

Given data: 50000 × 7 ____ 400000

To write:<,> or = in each blank.

Calculate the L.H.S (left-hand side) if necessary, of the blank as shown below

∴ 50000×7=350000

Again calculate the R.H.S (right-hand side) if necessary, of the blank as shown below

∴ 400000

It can be clearly observed that the term on R.H.S is greater than the term on the L.H.S and therefore the sign to be used is <.

Thus, it can be clearly concluded that 50000 × 7 < 400000.

Page 36 Exercise 1.14 Problem 18

Given data: 23000 + 40000 ___ 87000−24000

To write: <,> or, =, in each blank.

Calculate the L.H.S (left-hand side) if necessary, of the blank as shown below

∴ 23000 + 40000 = 63000

Again calculate the R.H.S (right-hand side) if necessary, of the blank as shown below

∴ 87000 − 24000 = 63000

It can be clearly observed that the term on L.H.S is equal to the term on the R.H.S and therefore the sign to be used is =.

Thus, it can be clearly concluded that 23000 + 40000 = 87000 − 24000.

Page 37 Exercise 1.15 Problem 1

Given data: $460000 + a = 480000$

To find – The missing number represented by each a.

Solve the equation as shown below

∴ 460000 + a = 480000

a = 480000 − 460000

a = 20000

Thus, it can be clearly concluded that the missing number represented by a, in the equation 460000 + a = 480000 is, 20000.

Page 37 Exercise 1.15 Problem 2

Given data: 48000 ÷ a = 6000

To find – The missing number represented by each a.

Solve the equation as shown below

∴ 48000 ÷ a = 6000

48000 ÷ 6000 = a

a = 8

Thus, it can be clearly concluded that the missing number represented by a, in the equation 48000 ÷ a = 6000 is, 8.

Page 37 Exercise 1.15 Problem 3

Given data: 48000 − a = 6000

To find – The missing number represented by each a.

Solve the equation as shown below

∴ 48000 − a = 6000

48000 − 6000 = a

a = 42000

Thus, it can be clearly concluded that the missing number represented by a, in the equation 48000 − a = 6000 is, 42000

Page 37 Exercise 1.15 Problem 4

Given data: 4000 × a = 32000

To find – The missing number represented by each a.

Solve the equation as shown below

∴ 4000 × a = 32000

a = $\frac{32000}{4000}$

a = 8

Thus, it can be clearly concluded that the missing number represented by a, in the equation 4000 × a = 32000 is, 8.

Page 37 Exercise 1.15 Problem 5

Given data: 22906,28609,82096,28069,8209

To arrange: The numbers in increasing order.

Observe that 8209 is the whole number which is the lowest in value and now on arranging it with increasing values, the following can be obtained as shown below

∴ 8209,226906,28069,28609,82096

Thus, it can be clearly concluded that the numbers, 22906,28609,82096,28069,and 8209 can be arranged in increasing order as 8209,22906,28069,28609,and 82096.

Page 37 Exercise 1.15 Problem 6

Given data: 435260,296870,503140,463540

To arrange: The numbers in increasing order.

Observe that 296870 is the whole number which is the lowest in value and now on arranging it with increasing values, the following can be obtained as shown below

∴ 296870,435260,463540,503140

Thus, it can be clearly concluded that the numbers 435260,296870,503140,and 463540 can be arranged in increasing order as 296870,435260,463540,and 503140.

Primary Mathematics Workbook 4A Common Core Edition Chapter 1 Whole Numbers Exercise 1.13 - 1.17 Page 38, Exercise 1.16 ,Problem 1

Page 37 Exercise 1.15 Problem 7

Given data: Some figures are given.

To fill – In Figure 4.

Observe that the number of circles in the first figure is 4, then 9, and then 16, and therefore, in the fourth figure it again increased to 25

Thus, in the fourth figure, one line of circles will increase like in the fourth line of figure 3 with 2 more circles in it.

Therefore, the fourth figure can be filled in as shown below

Thus, it can be clearly concluded that figure 4 can be filled in as shown below

Primary Mathematics Workbook 4A Common Core Edition Chapter 1 Whole Numbers Exercise 1.13 - 1.17 Page 38, Exercise 1.16 , Problem 1.

Page 38 Exercise 1.16 Problem 1

Given data: Some figures are given.

To find – The pattern that we notice in the number of circles.

Observe that the number of circles in the first figure is 4, then 9 and then 16 and therefore, in the fourth figure it again increased to 25

Thus, the difference in the number of circles is increasing by 2 after being 4 and 9 in the first and second figures respectively.

Thus, there is an increasing pattern with the number of circles in the n^thfigure being (n+1)².

Thus, it can be clearly concluded that the pattern that we notice in the number of circles is an increasing pattern with the number of circles in the n^th figure being (n+1)².

Page 38 Exercise 1.16 Problem 2

Given data: The digits are 0,1,9,and 5,8.

To find – The greatest 5 -digit number that can be formed using all of the given five digits.

Observe that digit greatest in value, that is, 9, and now arrange the digits in order of decreasing values to obtain as shown below

Therefore,98510

Thus, it can be clearly concluded that the greatest 5 -digit number that can be formed using all of the given five digits that are, 0,1,9,5, and 8 is, 98510.

Page 38 Exercise 1.16 Problem 3

Given data: The multiples of 8.

To find – The first 5 multiples of 8.

Observe that the first five multiples of 8 are including 8 itself and as shown below

Therefore 8,16,24,32 and 40

Thus, it can be clearly concluded that the first 5 multiples of 8 are 8,16,24,32, and 40.

Page 38 Exercise 1.16 Problem 4

Given data: The common multiples of 4 and 5.

To find – The first2 common multiples of 4 and 5.

Observe that the first two common multiples of 4 and 5 can be calculated as shown below

Therefore

L.C.M = 2.2.5

= 20

[where L.C.M represents the lowest common multiple]

And also, 20.2 = 40

Thus, it can be clearly concluded that the first 2 common multiples of 4 and 5 are 20,and 40.

Page 38 Exercise 1.16 Problem 5

Given data: The given number is 4598.

To find – If 6 is a factor of 4598.

Observe that on dividing 4598 by 6, the following is obtained as shown below

Therefore $\frac{4598}{6}$ = 766.334

Which shows that 6 is not a factor of 4598.

Thus, it can be clearly concluded that 6 is not a factor of 4598.

Page 38 Exercise 1.16 Problem 6

Given data: The given number is 54.

To find – All the factors of 54.

Observe that the various numbers which can be divided by 54 are obtained as shown below

Therefore; 1,2,3,6,9,18,27,54,−1,−2,−3,−6,−9,−18,−27,−54 are all factors of 54.

Thus, it can be clearly concluded that all the factors of 54 are 1,2,3,6,9,18,27,54,−1,−2,−3,−6,−9,−18,−27,−54.

Page 38 Exercise 1.16 Problem 7

Given data: The given numbers are 4,6,8, and 12.

To find – The common factor of 18 and 36 from these numbers.

Observe that the various numbers which can be divided by 18 and 36 are obtained as shown below

Therefore; 1,2,3,6,9,18,−1,−2,−3,−6,−9,−18 are all factors of 18.

1,2,3,4,6,9,12,18,36,−1,−2,−3,−4,−6,−9,−12,−18,−36 are all factors of 36.

Now, observe that the common factors of 18 and 36 are 1,2,3,6,9,18,−1,−2,−3,−6,−9,−18, and out of the given four numbers it is 6

Thus, it can be clearly concluded that the common factor of 18 and 36 from the numbers, 4,6,8, and 12 is 6.

Page 39 Exercise 1.17 Problem 1

Given data: The given numbers are 25 and 30.

To find – A prime number between 25 and 30.

Observe that a prime number between 25 and 30 is 29 as it is divisible by 1 and itself only.

Thus, it can be clearly concluded that a prime number between 25 and 30 is 29.

Page 39 Exercise 1.17 Problem 2

Given data: 5 × 24 = 5 × 6 × n

To find – The missing factor represented by each n.

Solve the equation as shown below

∴ 5×24 = 5 × 6 × n

$\frac{5×24}{5×6}$ = n

n = 4

Thus, it can be clearly concluded that the missing factor represented by n, in the equation 5 × 24 = 5 × 6 × n is, 4.

Page 39 Exercise 1.17 Problem 3

Given data: 2 × 180 = 4 × n

To find – The missing factor represented by each n.

Solve the equation as shown below

∴ 2 × 180 = 4 × n

$\frac{2×180}{4}$ = n

n = 90

Thus, it can be clearly concluded that the missing factor represented by n, in the equation 2 × 180 = 4 × n is, 90

Page 39 Exercise 1.17 Problem 4

Given data: 26 × 55 = 26 × n × 5

To find – The missing factor represented by each n.

Solve the equation as shown below

∴ 26 × 55 = 26 × n × 5

$\frac{26×55}{26×5}$ = n

n = 11

Thus, it can be clearly concluded that the missing factor represented by n, in the equation 26×55 = 26×n×5, is 11.

Page 39 Exercise 1.17 Problem 5

Given data: 15 × 250 = 15 × 5 × n

To find – The missing factor represented by each n.

Solve the equation as shown below

∴ 15 × 250 = 15 × 5 × n

$\frac{15×250}{15×5}$ = n

n = 50

Thus, it can be clearly concluded that the missing factor represented by n, in equation 15 × 250 = 15 × 5 × n is, 50.

Page 39 Exercise 1.17 Problem 6

Given data: 18 × 35 = 9 × 2 × n × 7

To find – The missing factor represented by each n.

Solve the equation as shown below

∴ 18 × 35 = 9 × 2 × n × 7

$\frac{18×35}{9×2×7}$ = n

n = 5

Thus, it can be clearly concluded that the missing factor represented by n, in the equation 18 × 35 = 9 × 2 × n × 7 is, 5.

Page 39 Exercise 1.17 Problem 7

Given data: The given equation is 67−(100−52) = ____

To solve: The following equation that is given.

Solve the equation using the BODMAS (Bracket, Of, Division Multiplication Addition and Subtraction) rule as shown below

∴ 67−(100−52) = 67 − 100 + 52

= 67−48

= 19

Thus, it can be clearly concluded that the given equation that is, 67−(100−52) =_____can be solved as 67−(100−52) = 19.

Page 39 Exercise 1.17 Problem 8

Given data: The given equation is (84−32) ÷ 4 =_____.

To solve: The following equation that is given.

Solve the equation using the BODMAS (Bracket, Of, Division Multiplication Addition and Subtraction) rule as shown below

∴ (84−32) ÷ 4 = 52 ÷ 4

= 13

Thus, it can be clearly concluded that the given equation that is, (84−32) ÷ 4 =____can be solved as (84−32) ÷ 4 = 13.

Page 39 Exercise 1.17 Problem 9

Given data: The given equation is 72 ÷6 + 18 ÷ 3 =____.

To solve: The following equation that is given.

Solve the equation using the BODMAS (Bracket, Of, Division Multiplication Addition and Subtraction) rule as shown below

∴ 72 ÷ 6 + 18 ÷ 3 = 12 + 6

= 18

Thus, it can be clearly concluded that the given equation that is, 72 ÷ 6 + 18 ÷ 3=_____can be solved as 72 ÷ 6 + 18 ÷ 3 = 18.

Page 39 Exercise 1.17 Problem 10

Given data: The given equation is 47−28 ÷ 7 × 8 =____.

To solve: The following equation that is given.

Solve the equation using the BODMAS (Bracket, Of, Division Multiplication Addition and Subtraction) rule as shown below

∴ 47−28 ÷ 7 × 8 = 47 − 4 × 8

= 47−32

= 15

Thus, it can be clearly concluded that the given equation that is, 47 − 28 ÷ 7 × 8=____ can be solved as 47−28 ÷ 7 × 8 = 15.

Page 39 Exercise 1.17 Problem 11

Given data: (37−15) × (5 + 3)______ 22 × 9

To write: >,< or = in each blank.

Calculate the L.H.S (left-hand side) of the blank using BODMAS as shown below

∴ (37−15) × (5 + 3) = 22 × 8

= 176

Again calculate the R.H.S (right-hand side) of the blank using BODMAS as shown below

∴ 22 × 9 = 198

It can be clearly observed that the term on R.H.S is greater than the term on the L.H.S and therefore the sign to be used is <.

Thus, it can be clearly concluded that (37−15) × (5 + 3) < 22 × 9.

Page 39 Exercise 1.17 Problem 12

Given data: 40 ÷ (12−4) + 2_____ 40 ÷ 10

To write: >,< or = in each blank.

Calculate the L.H.S (left-hand side) of the blank using BODMAS as shown below

∴ 40 ÷ (12−4) + 2 = 40 ÷ 8 + 2

= 5 + 2

= 7

Again calculate the R.H.S (right-hand side) of the blank using BODMAS as shown below

∴ 40 ÷ 10 = 4

It can be clearly observed that the term on R.H.S is less than the term on the L.H.S and therefore the sign to be used is >.

Thus, it can be clearly concluded that 40 ÷ (12 − 4) + 2 > 40 ÷ 10.

Page 39 Exercise 1.17 Problem 13

Given data: 16 + 2×_____= 20

To fill: In the blank to make the equation true.

Let the blank or the missing value be represented by x

Solve the equation as shown below

Therefore

16 + 2×__ = 20

20−16 = 2x

$\frac{4}{2}$ = x

2 = x (or) x = 2

Thus, it can be clearly concluded that the equation, that is, 16 + 2×___= 20 can be made true by filling in the blank with 2.

Page 39 Exercise 1.17 Problem 14

Given data: 48÷(4 × 2)−2 = 2×______

To fill: In the blank to make the equation true.

Let the blank or the missing value be represented by x

Solve the equation as shown below

∴ 48 ÷ (4 × 2) − 2 = 2 × x

48 ÷ 8 − 2 = 2x

6 − 2 = 2x

2 = x (or) x = 2

Thus, it can be clearly concluded that the equation, that is, 48 ÷ (4 × 2)−2 = 2×___can be made true by filling the blank with 2.

Page 39 Exercise 1.17 Problem 15

Given data: 18 − (10 − 6) = 10 +______

To fill: In the blank to make the equation true.

Let the blank or the missing value be represented by x

Solve the equation as shown below

∴ 18−(10−6) = 10 + x

18 − 4 − 10 = x

4 = x (or) x = 4

Thus, it can be clearly concluded that the equation, that is, 18−(10−6) = 10 +____can be made true by filling in the blank with 4.

Page 39 Exercise 1.17 Problem 16

Given data: (7 + 5) × (15−12) =_____× 12

To fill: In the blank to make the equation true.

Let the blank or the missing value be represented by x

Solve the equation as shown below

∴ (7 + 5) × (15−12) = x × 12

12 × 3 = x

$\frac{12×3}{12}$ = x

3 = x (or) x = 3

Thus, it can be clearly concluded that the equation, that is, (7 + 5) × (15 − 12)=___× 12 can be made true by filling in the blank with 3.

Page 39 Exercise 1.17 Problem 17

Given data: 16 + 4 × 8 =______+ 32

To fill: In the blank to make the equation true.

Let the blank or the missing value be represented by x

Solve the equation as shown below

∴ 16 + 4 × 8 = x + 32

16 + 32−32 = x

16 = x (or) x = 16

Thus, it can be clearly concluded that the equation, that is, 16 + 4 × 8 =___+ 32 can be made true by filling in the blank with 16.

Page 39 Exercise 1.17 Problem 18

Given data: 9 + 12 ÷ 3 − 2 = 4+____

To fill: In the blank to make the equation true.

Let the blank or the missing value be represented by x

Solve the equation as shown below

∴ 9 + 12 ÷ 3 − 2 = 4 + x

9 + 4 − 2−4 = x

7=x (or) x = 7

Thus, it can be clearly concluded that the equation, that is, 9 + 12 ÷ 3 − 2 = 4 +___can be made true by filling in the blank with 7.

Page 39 Exercise 1.17 Problem 19

Given data: 12 − 3 × 2 + 9 = 15

To insert: Parentheses, where necessary, to make the given equation true.

Solve the equation by inserting the parentheses as shown below

∴ 12−3 × 2 + 9 = 15

Observe that no parentheses is required to be inserted in this equation as it is already true.

Thus, it can be clearly concluded that the equation, that is, 12 − 3 × 2 + 9 = 15 can be made true by inserting no parentheses as 12−3 × 2 + 9 = 15.

Page 39 Exercise 1.17 Problem 20

Given data: 12 − 3 × 2 + 9 = 99

To insert: Parentheses, where necessary, to make the given equation true.

Solve the equation by inserting the parentheses as shown below

∴ 12 − 3 × 2 + 9 = 99

⇒ (12−3) × (2 + 9) = 99

Thus, it can be clearly concluded that the equation, that is, 12 − 3 × 2 + 9 = 99 can be made true by inserting no parentheses as (12 − 3) × (2 + 9) = 99.

Primary Mathematics Workbook 4A Common Core Edition Solutions Chapter 1 Whole Numbers Exercises 1.7

March 20, 2023March 14, 2023 by Marksparks

Primary Mathematics Chapter 1 Whole Numbers

Page 21 Exercise 1.7 Problem 1

Given: Multiples of 6

To find – Several multiples of 6

Put different values of k to find different multiples

Given: Multiples of 6

Multiples of 6 can be written as 6k

For the first multiple of 6 (k=1)

⇒ 6 × 1

⇒ 6

For second multiple of 6( k = 2 )

⇒ 6 × 2

⇒ 12

For the third multiple of 6 (k = 3)

⇒ 6 × 3

⇒ 18

For the fourth multiple of 6 (k=4)

⇒ 6 × 4

⇒ 24

The first, second, third, and fourth multiple of 6 is 6,12,18, and 24.

Page 21 Exercise 1.7 Problem 2

Given: Multiples of 7

To find – Several multiples of 7

Put different values of K to find different multiples

Given: Multiples of 7

Multiples of 7 can be written as 7k

For the first multiple of 7 (k = 1)

⇒ 7 × 1

⇒ 7

For the second multiple of 7 (k = 2)

⇒ 7 × 2

⇒ 14

For the third multiple of 7 (k = 3)

⇒ 7 × 3

⇒ 21

For the fourth multiple of 7 (k = 4)

⇒ 7 × 4

⇒ 28

For the fifth multiple of 7 (k = 5)

⇒ 7 × 5

⇒ 35

First, five multiples of 7 are 7,14,21,28,35.

Page 21 Exercise 1.7 Problem 3

Given: Multiples of 2,3,4,6,8,10

To find – Next five multiples of 2,3,4,6,8,10

Put different values of k to find different multiples

Multiples of 2

Multiples of 2 can be written as 2k

For the third multiple of 2 (k = 3)

⇒ 2 × 3

⇒ 6

For the fourth multiple of 2 (k = 4)

⇒ 2 × 4

⇒ 8

For the fifth multiple of 2 (k = 5)

⇒ 2 × 5

⇒ 10

For the sixth multiple of 2 (k=6)

⇒ 2 × 6

⇒ 12

For seventh multiple of 2(k = 7)

⇒ 2 × 7

⇒ 14

Multiples of 3

Multiples of 3 can be written as 3k

For the third multiple of 3 (k = 3)

⇒ 3 × 3

⇒ 9

For the fourth multiple of 3 (k = 4)

⇒ 3 × 4

⇒ 12

For the fifth multiple of 3 (k = 5)

⇒ 3 × 5

⇒ 15

For the sixth multiple of 3 (k = 6)

⇒ 3 × 6

⇒ 18

For the seventh multiple of 3 (k = 7)

⇒ 3 × 7

⇒ 21

Multiples of 4

Multiples of 4 can be written as 4k

For the third multiple of 4 (k = 3)

⇒ 4 × 3

⇒ 12

For the fourth multiple of 4 (k = 4)

⇒ 4 × 4

⇒ 16

For the fifth multiple of 4 (k = 5)

⇒ 4 × 5

⇒ 20

For the sixth multiple of 4 (k = 6)

⇒ 4 × 6

⇒ 24

For the seventh multiple of 4 (k = 7)

⇒ 4 × 7

⇒ 28

Multiples of 6

Multiples of 6 can be written as 6k

For the third multiple of 6 (k = 3)

⇒ 6 × 3

⇒ 18

For the fourth multiple of 6 (k = 4)

⇒ 6 × 4

⇒ 24

For the fifth multiple of 6 (k = 5)

⇒ 6 × 5

⇒ 30

For the sixth multiple of 6(k = 6)

⇒ 6 × 6

⇒ 36

For the seventh multiple of 6 (k = 7)

⇒ 6 × 7

⇒ 42

Multiples of 8

Multiples of 8 can be written as 8k

For the third multiple of 8 (k = 3)

⇒ 8 × 3

⇒ 24

For the fourth multiple of 8 (k = 4)

⇒ 8 × 4

⇒ 32

For the fifth multiple of 8 (k = 5)

⇒ 8 × 5

⇒ 40

For the sixth multiple of 8 (k = 6)

⇒ 8 × 6

⇒ 48

For the seventh multiple of 8 (k = 7)

⇒ 8 × 7

⇒ 56

Multiples of 10

Multiples of 10 can be written as 10k

For the third multiple of 10 (k = 3)

⇒ 10 × 3

⇒ 30

For the fourth multiple of 10 (k = 4)

⇒ 10 × 4

⇒ 40

For the fifth multiple of 10 (k = 5)

⇒ 10 × 5

⇒ 50

For the sixth multiple of 10 (k = 6)

⇒ 10 × 6

⇒ 60

For the seventh multiple of 10 (k = 7)

⇒ 10 × 7

⇒ 70

Therefore

The next five multiples of 2 is 6,8,10,12,14

The next five multiples of 3 is 9,12,15,18,21

The next five multiples of 4 is 12,16,20,24,28

The next five multiples of 6 is 18,24,30,36,42

The next five multiples of 8 is 24,32,40,48,56

The next five multiples of 10 is 30,40,50,60,70.

Page 22 Exercise 1.7 Problem 4

Given: Random digits and numbers

To check that digits are multiples of number

Check the pattern of numbers multiple and mark it YES or NO

Statement: 57 is a multiple of 3

Multiple of 3 have digits add up to multiple of 3.

Sum of digits of 57 is 12 which is a multiple of 3.

YES, 57 is a multiple of 3.

Statement: 57 is a multiple of 6

Multiple of 6 are even and have digits add up to multiple of 3.

Sum of digits of 57 is 12 which is a multiple of 3 but 57 is not even.

No, 57 is not a multiple of 6.

Statement: 78 is a multiple of 2

Multiple of 2 are even and have last digit as 0,2,4,6,8, last digit of 78 is 8

YES, 78 is a multiple of 2.

Statement: 33,450 is a multiple of 10

Multiple of 10 have 0 at the ones place. 33,450 has 0 at the ones place.

YES, 33,450 is a multiple of 10.

Statement: 452 is a multiple of 3

Multiple of 3 have 3 has digits add up to multiple of 3.

The sum of digits of 452 is 11 which is not a multiple of 3.

No, 452 is not a multiple of 3.

Statement: 4985 is a multiple of 2

Multiple of 2 have the last digit as 0,2,4,6,8.

The last digit of 4985 is 5.

No, 4985 is not a multiple of 2.

Statement: 4985 is a multiple of 5

Multiple of 5 have the last digit as 0,5.

Last digit of 4985 is 5.

YES, 4985 is a multiple of 5.

So, we can conclude the table as

YES, 57 is a multiple of 3.

No, 57 is not a multiple of 6.

YES, 78 is a multiple of 2.

YES, 33,450 is a multiple of 10.

No, 452 is not a multiple of 3.

No, 4985 is not a multiple of 2.

YES, 4985 is a multiple of 5.

Page 22 Exercise 1.7 Problem 5

Given: Multiple of 100.

To find – Rule for multiples of 100.

Check the pattern of the multiple.

Multiples of 100 can be written as 100k

⇒ 100 × k = k00

The first multiple of 100 is 100

The second multiple of 100 is 200 and so on.

Each multiple 100 of has 0 at its ones and tenth place.

Therefore, the rule of multiples of 100 is found that each multiple of 100 has 0 at its ones and tenth place.

Page 23 Exercise 1. 8 Problem 1

Given: Integer 20

To find- The factors of 20

Find the factors of 20 from the shown above.

Given: Integer 20

We know that

1 × 20 = 20

2 × 10 = 20

4 × 5 = 20

From this, we have Divisors of 20 are 1,2,4,5,10,20

Factors of 20 are divisors of 20 are 1,2,4,5,10,20

Therefore, the Factors of 20 are 1,2,4,5,10,20.

Page 23 Exercise 1.8 Problem 2

Given: Integer 12

To find – The factors of 12

Find the factors of 12 from the shown above.

Given: Integer 12

We know that

2 × 6 = 12

From this, we have Divisors of 12 are 2,6

Factors of 12 are divisors of 12 are 2,6

Therefore, the factors of 12 are 2,6.

Page 23 Exercise 1.8 Problem 3

Given: Integer 8

To find – The factors of 8

Find the factors of 8 from the shown above.

Given: Integer 8

We know that

1 × 8 = 8

From this, we have Divisors of 8 are 1,8

Factors of 8 are divisors of 8 are 1,8

Therefore the factors of 8 are 1,8.

Page 23 Exercise 1.8 Problem 4

Given: Integer 21

To find – The factors of 21

Find the factors of 21 from the shown above.

Given: Integer 21

We know that

3 × 7 = 21

From this, we have Divisors of 21 are 3,7

Factors of 21 are divisors of 21 are 3,7

Therefore, the factors of 21 are 3,7.

Page 24 Exercise 1.8 Problem 5

Given: 2×__= 8

Question is to fill the blank

Find the value in blank using factors of 8

Given: 2×___= 8

Let the number be x, we have

2x = 8

Now, evaluating the value of x

2x = 8

x = 4

The value in the blanks is 4, 2 × − 4 = 8

Therefore, the value of the missing factor in the blanks is 4, 2× − 4 = 8

Page 24 Exercise 1.8 Problem 6

Given: __×6 = 18

Question is to fill the blank

Find the value in blank using factors of 18

Given: ___× 6 = 18

Let the number be x, we have

6x = 18

Now, evaluating the value of x

6x = 18

x = 3

The value in the blanks is 3, −3 × 6 = 18

Therefore, the value of the missing factor in the blank is 3, −3 × 6 = 18.

Page 24 Exercise 1.8 Problem 7

Given: 5 ×___= 45

Question is to fill the blank

Find the value in blank using factors of 45

Given: 5×___ = 45

Let the number be x, we have

5x = 45

Now, evaluating the value of x

5x = 45

x = 9

The value in the blanks is 9, 5×−9 = 45

Therefore, the value of the missing factor in the blanks is 9, 5×− 9 = 45.

Page 24 Exercise 1.8 Problem 8

Given: 6×___= 48

Question is to fill the blank

Find the value in blank using factors of 48

Given: 6 × ___= 48

Let the number be x, we have

6x = 48

Now, evaluating the value of x

6x = 48

x = 8

The value in the blanks is 8, 6 × 8 = 48

Therefore, the value of the missing factor in the blanks is 8, 6 × 8 = 48.

Page 24 Exercise 1.8 Problem 9

Given: 7×___ = 56

Question is to fill the blank

Find the value in blank using factors of 56

Given: 7 ×___= 56

Let the number be x, we have

7x = 56

Now, evaluating the value of x

7x = 56

x = 8

The value in the blanks is 8, 7 × 8 = 56

Therefore, the value of the missing factor in the blanks is 8, 7 × 8 = 56

Page 24 Exercise 1.8 Problem 10

Given: 9×__= 72

Question is to fill the blank

Find the value in blank using factors of 72

Given: 9 × __= 72

Let the number be x, we have

9x = 72

Now, evaluating the value of x

9x = 72

x = 9

The value in the blanks is 8, 9 × 8 = 72

Therefore, the value of the missing factor in the blanks is 8, 9×8 = 72

Page 24 Exercise 1.8 Problem 11

Given: ___×4 = 72

Question is to find the missing factors and then fill the blank

Find the value in blank using factors of 72.

Given: ___×4 = 72

Let the number be x, we have

4x = 72

Now, evaluating the value of x

4x = 72

x = 18

The value in the blanks is 18, 18 × 4 = 72.

Therefore, the value of the missing factor in the blanks is 18, 18 × 4 = 72.

Page 24 Exercise 1.8 Problem 12

Given: ___× 6 = 54

Question is to fill the blank

Find the value in blank using factors of 54

Given: ___ × 6 = 54

Let the number be x, we have

6x = 54

Now, evaluating the value of x

6x = 54

x = 9

The value in the blanks is 9, 9 × 6 = 54

Therefore, the value of the missing factor in the blanks is 9, 9 × 6 = 54

Page 24 Exercise 1.8 Problem 13

Given: ___× 7 = 70

Question is to fill the blank

Find the value in blank using factors of 70

Given: ___ × 7 = 70

Let the number be x, we have

7x = 70

Now, evaluating the value of x

7x = 70

x = 10

The value in the blanks is 10, 10 × 7 = 70

Therefore, the value of the missing factor in the blanks is 10, 10 × 7 = 70

Page 24 Exercise 1.8 Problem 14

Given: ___× 8 = 64

Question is to fill the blank

Find the value in blank using factors of 64

Given: ___× 8 = 64

Let the number be x, we have 8x=64

Now, evaluating the value of x

8x = 64

x = 8

The value in the blanks is 8, 8 × 8 = 64

Therefore, the value of the missing factor in the blanks is 8, 8 × 8 = 64

Page 24 Exercise 8 Problem 15

Given:

8=1 × ___

8=2 × ___

Question is to fill the blanks and find the factors of 8

Find the value in blank using factors of 8

Given: 8 = 1×___

Let the number be x, we have

x = 8

8 = 1 × 8

Given: 8 = 2 × ___

Let the number be x, we have

2x = 8

8 = 2 × 4

Factors of 8 are 1,2,4,8

Therefore, the blank values are 8,4, 8 = 1 × 8, and 8 = 2 × 4. Factors of 8 are 1,2,4 and 8.

Page 24 Exercise 8 Problem 16

Given:

15 = 1×___

15 = 3×___

Question is to fill the blanks and find the factors of 15

Find the value in blank using factors of 15

Given: 15 = 1×___

Let the number be x, we have

x = 15

15 = 1 × 15

Given: 15 = 3 × ___

Let the number be x, we have

3x = 15

15 = 3 × 5

Factors of 15 are 1,3,5,15

Therefore, the blank values are 15,5 15 = 1 × 15 and 15 = 3 × 5. Factors of 15 are 1,3,5 and 15

Page 24 Exercise 8 Problem 17

Given: 16,27,13,19,21

To find – The prime numbers out of these five numbers

Check the number according to the definition of prime number

Given: 16

As we know

1 × 16 = 16

2× 8 = 16

4 × 4 = 16

It means the factor of 16 is 1,2,4,8,16. 16 is not a prime number.

Given: 27

As we know

1 × 27 = 27

3 × 9 = 27

It means the factor of 27 is 1,3,9,27. 27 is not a prime number.

Given: 13

As we know

1 × 13 = 13

It means the factor of 13 is 1,13. 13 is a prime number.

Given: 19

As we know

1 × 19 = 19

It means the factor of 19 is 1,19. 19 is a prime number.

Given: 21

As we know

1 × 21 = 21

3 × 7 = 21

It means factor of 21 is 1,3,7,21. 21

Therefore, the prime numbers out of 16,27,13,19,21 are 13 and 19.

Page 25 Exercise 1.9 Problem 1

Given: Is 2 a factor of 35

Question is to check the statement

Use the Divisor method to find the factors of 35 and check that 2 is a factor of 35

Given: Is 2 a factor of 35

Using Factors of 35 by divisor method

Dividing 35 by 1, we have

$\frac{35}{1}$ = 35

1 is a factor of 35

Dividing 35 by 5, we have

$\frac{35}{5}$ = 7

5 is a factor of 35

Dividing 35 by, we have

$\frac{35}{7}$ = 5

7 is a factor of 35

Dividing 35 by 35, we have

$\frac{35}{35}$ = 1

35 is a factor of 35

Factors of Number 35 are 1,5,7,35. If we divide the number 35 by any integer other than these the remainder will not be 0.

It means if we divide 35 by 2 the remainder will not be. Or we can say 2 is not a factor of 35.

Factors of Number 35 are 1,5,7,35. If we divide the number 35 by any integer other than these the remainder will not be 0 Or we can say 2 is not a factor of 35.

Page 25 Exercise 1.9 Problem 2

Given: Is 3 a factor of 45

Question id to check the statement

Use the Divisor method to find the factors of 45 and check that 3 is a factor of 45

Given: Is 3 a factor of 45

Using Factors of 45 by divisor method

Dividing 45 by 1, we have

$\frac{45}{1}$ = 45

1 is a factor of 45

Dividing 45 by 3, we have

$\frac{45}{3}$ = 15

3 is a factor of 45

Dividing 45 by 5, we have

$\frac{45}{5}$=9

9 is a factor of 5

Dividing 45 by 15, we have

$\frac{45}{15}$= 3

15 is a factor of 45

Dividing 45 by 45, we have

$\frac{45}{45}$=1

45 is a factor of 45

Factors of Number 45 are 1,3,5,9,15,45.

If we divide the number 45 by any integer other than these the remainder will not be 0.

It means if we divide 45 by 3 the remainder will be 0. Or we can say 3 is a factor of 45.

Factors of Number 45 are 1,3,5,9,15,45. If we divide the number 45 by any integer other than these the remainder will not be 0 Or we can say 3 is a factor of 45.

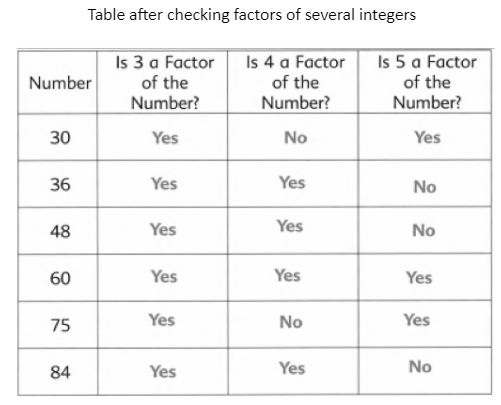
Page 25 Exercise 1.9 Problem 3

Given: 36,48,60,75,84 and digit 3,4,5

To check that integer from 36,48,60,75,84 has 3,4,5 as a factor of integer

Use condition for multiples of 3,4,5 and check it

Factors of 36

Sum of digits of 36 is 9 which is a multiple of 3 so 3 is a factor of 36

Integer 36 is an even number and its last two digits are divisible by 4 so 4 is a factor of 36.

Integer 36 has 6 at ones place so 5 is not a factor of 36.

Factors of 48

Sum of digits of integer 48 is 12 which is a multiple of 3 so 3 is a factor of 48

Integer 48 is an even number and its last two digits are divisible by 4 so 4 is a factor of 48.

Integer 48 has 8 at ones place so 5 is not a factor of 48.

Factors of 60

Sum of digits of integer 60 is 6 which is a multiple of 3 so 3 is a factor of 60

Integer 60 is an even number and its last two digits are divisible by 4 so 4 is a factor of 60.

Integer 60 has 0 at ones place so 5 is a factor of 60.

Factors of 75

Sum of digits of integer 75 is 12 which is a multiple of 3 so 3 is a factor of 75

Integer 75 is an odd number so 4 is not a factor of 75.

Integer 75 has 5 at ones place so 5 is a factor of 75.

Factors of 84

Sum of digits of integer 84 is 12 which is a multiple of 3 so 3 is a factor of 84

Integer 84 is an even number and its last two digits are divisible by 4 so 4 is a factor of 84.

Integer 84 has 4 at ones place so 5 is not a factor of 84.

Combining all the answers, we have

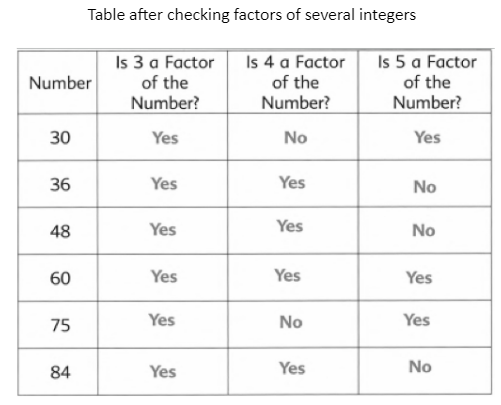
Primary Mathematics Workbook 4A Common Core Edition Chapter 1 Whole Numbers Exercises 1.7 - 1.12 Page 25 Exercise 1.9 Problem 3, table 1

Therefore

Integer 36 : 3 is a factor of 36, 4 is a factor of 36, and 5 is not a factor of 36.

Integer 48 : 3 is a factor of 48,4 is a factor of 48, and 5 is not a factor of 48.

Integer 60 : 3 is a factor of 60,4 is a factor of 60, and 5 is a factor of 60.

Integer 75 : 3 is a factor of 75,4 is not a factor of 75, 5 is a factor of 75.

Integer 84 : 3 is a factor of 84, 4 is a factor of 84, and 5 is not a factor of 84.

The combined answers of questions above are:

Primary Mathematics Workbook 4A Common Core Edition Chapter 1 Whole Numbers Exercises 1.7 - 1.12 Page 25 Exercise 1.9 Problem 3 , table 2

Page 25 Exercise 1.9 Problem 4

Given: Answer from above Question

To find – Common factor of all the integer

Observe the result of the above Question and find the common factor

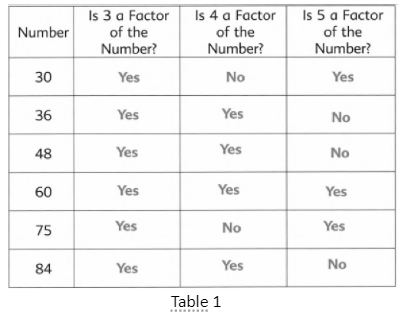
Result of above Question

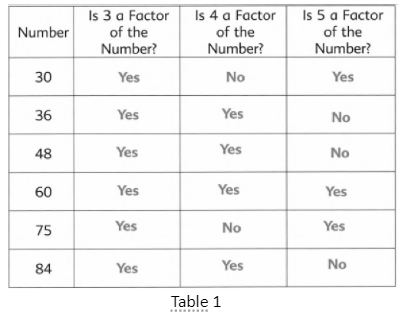
Primary Mathematics Workbook 4A Common Core Edition Chapter 1 Whole Numbers Exercises 1.7 - 1.12 Page 25 Exercise 1.9 , Problem 4, table 1

As we can see from the result of above Question, 3 is a common factor of all integers 30,36,48,60,75,84

As we can see from the result of above Question, 3 is a common factor of all integers 30,36,48,60,75,84.

Primary Mathematics Workbook 4A Common Core Edition Chapter 1 Whole Numbers Exercises 1.7 - 1.12 Page 25 Exercise 1.9 , Problem 4, table 2

Page 26 Exercise 1.9 Problem 5

Given: 64

To find all the factor of the given number.

We have to find all the numbers when multiplied is equal to 64

1 × 64 = 64

2 × 32 = 64

4 × 16 = 64

8 × 8 = 64

64 is a multiple of 1,2,4,8,16,32 and 64

Therefore, the factors of 64 are 1,2,4,8,16,32 and 64

The factors of 64 are 1,2,4,8,16,32 and 64.

Page 26 Exercise 1.9 Problem 6

Given: 72

To find all the factor of the given number.

We have to find all the numbers when multiplied is equal to 72

1 × 72 = 72

2 × 36 = 72

4 × 18 = 72

8 × 9 = 72

72 is a multiple of 1,2,4,8,9,18,36 and 72

Therefore, the factors of 72 are 1,2,4,8,9,18,36 and 72

The factors of 72 are 1,2,4,8,9,18,36 and 72

Page 26 Exercise 1.9 Problem 7

Given: 84

To find all the factor of the given number.

We have to find all the numbers when multiplied is equal to 84

1 × 84 = 84

2 × 42 = 84

4 × 21= 84

7 × 12 = 84

84 is a multiple of 1,2,4,7,12,21,42 and 84

Therefore, the factors of 84 are 1,2,4,7,12,21,42 and 84

The factors of 84 are 1,2,4,7,12,21,42 and 84

Page 26 Exercise 1.9 Problem 8

Given: 98

To find all the factor of the given number.

We have to find all the numbers when multiplied is equal to 98

1 × 98 = 98

2 × 49 = 98

14 × 7 = 98

98 is a multiple of 1,2,7,14,49, and 98

Therefore, the factors of 98 are 1,2,7,14,49, and 98

The factors of 98 are 1,2,7,14,49, and 98

Page 26 Exercise 1.9 Problem 9

Given: 60

To find all the factor of the given number.

We have to find all the numbers when multiplied is equal to 60

1 × 60 = 60

2 × 30 = 60

4 × 15 = 60

5 × 12 = 60

6 × 10 = 60

60 is a multiple of 1,2,4,5,6,10,12,15,30 and 60

Therefore, the factors of 60 are 1,2,4,5,6,10,12,15,30 and 60

The factors of 60 are 1,2,4,5,6,10,12,15,30 and 60

Page 27 Exercise 1.9 Problem 10

Given: 64 = 2 × 8 ×_____ × _____

To find – The missing factors.

We have to use prime numbers for the missing factors.

Solve for x.

Let the missing factor be x

64 = 2 × 8 × x

⇒ x = $\frac{64}{16}$

⇒ x = 4

Here, the missing factor should be of the form of prime numbers.

We know that 4 can be written as 4=2×2 and we also know that is a prime number.

Therefore, the missing factors are 2 and 2

64 = 2 × 8 × 2 × 2

Page 27 Exercise 1.9 Problem 11

Given: 84 = 6 ×_____ × _____

To find- The missing factors.

We have to use prime numbers for the missing factors.

Solve for x.

Let the missing factor be x

84 = 6 × x

⇒ x = $\frac{84}{6}$

⇒ x = 14

Here, the missing factor should be of the form of prime numbers.

We know that 14 can be written as 14 = 7 × 2 and we also know that 2 and 7 are prime number.

Therefore, the missing factors are 2 and 7

Hence, the missing factors are 84=6 × 2 × 7

Page 27 Exercise 1.9 Problem 12

Given: 45 =_____×_____×5

To find – The missing factors.

We have to use prime numbers for the missing factors.

Solve for x.

Let the missing factors be x

45 = x × 5

⇒ x = $\frac{45}{5}$

⇒ x = 9

Here, the missing factor should be of the form of prime numbers.

We know that 9 can be written as 9 = 3 × 3 and we also know that 3 and 3 are prime number.

Therefore, the missing factors are 3 and 3

Hence the factors are 45 = 3 × 3 × 5

Page 27 Exercise 1.9 Problem 13

Given: 72=_____×4×_____×_____

To find – The missing factors.

We have to use prime numbers for the missing factors.

Solve for x.

Let the missing factors be x

72 = x × 4

⇒ x = $\frac{72}{4}$

⇒ x = 18

Here, the missing factor should be of the form of prime numbers.

We know that 18 can be written as 18 = 2 × 3 × 3 and we also know that 2 and 3 are prime number.

Therefore, the missing factors are 2 ,3 and 3

Hence, the missing factors are 72 = 2 × 4 × 3 × 3

Page 27 Exercise 1.9 Problem 14

Given: 24 = 3 × 2 × n

To find – The missing factors n.

Solve the equation for n.

Simplifying the equation

24 = 3 × 2 × n

⇒ 24 = 6n

Dividing

⇒ n = $\frac{24}{6}$

⇒ n = 4

The missing factor is n = 4

Page 27 Exercise 1.9 Problem 15

Given: 18 = 3 × 2 × n

To find – The missing factors n.

Solve the equation for n.

Simplifying the equation

18 = 3 × 2 × n

⇒ 18 = 6n

Dividing

⇒ n = $\frac{18}{6}$

⇒ n = 3

Therefore the value of n in the expression 18=3×2×n will be n = 3

Page 27 Exercise 1.9 Problem 16

Given: 25 × 4 = 5 × n × 4

To find – The missing factors n.

Solve the equation for n.

Simplifying the equation

25 × 4 = 5 × n × 4

⇒ 25 × 4 = 20n

Dividing

⇒ n = $\frac{25×4}{20}$

⇒ n = 5

The value of n in the equation 25 × 4 = 5 × n × 4 will be n=5

Page 27 Exercise 1.9 Problem 17

Given: 21 × 20 = 21 × n × 2

To find- The missing factors n.

Solve the equation for n.

Simplifying the equation

21 × 20 = 21 × n × 2

⇒ 21 × 20 = 21 × 2 × n

Dividing

⇒ n = $\frac{21×20}{21×2}$

⇒ n = 10

The missing factors represented by n is 10.

Page 27 Exercise 1.9 Problem 18

Given: 3 × 32 = 6 × n

To find- The missing factors n.

Solve the equation for n.

Simplifying the equation

3 × 32 = 6 × n

⇒ n=$\frac{3×32}{6}$

Dividing

⇒ n= $\frac{1×32}{2}$

⇒ n = 16

The value of n in the equation 3 × 32 = 6 × n will be n = 16

Page 27 Exercise 1.9 Problem 19

Given: 16 × 2 = 4 × n

To find- The missing factors n.

Solve the equation for n.

Simplifying the equation

16 × 2 = 4 × n

⇒ n = $\frac{16×1}{2}$

n = 8

The value of n in the equation 16×2=4×n will be n = 8

Page 27 Exercise 1.9 Problem 20

Given: To find if 4 is a common factor of 36 and 60

To solve this question, 36 and 60 by divide 4

If it leaves a remainder, then 4 is not a factor.

If it doesn’t leave a remainder, then 4 is a factor

Divide 36 by divide 4

⇒ $\frac{36}{4}$

⇒ 9

When dividing 36 by 4, there is no reminder.

Therefore, 4 is a common factor of 36

Divide 60 by divide 4

⇒ $\frac{60}{4}$

⇒ 15

When divided 60 by 4, there is no reminder.

Therefore, 4 is a common factor of 60

4 is a common factor of 36 and 60.

Page 27 Exercise 1.9 Problem 21

Given: To find if 4 is a common factor of 48 and 90

To solve this question, 48 and 90 by divide 4

If it leaves a remainder, then 4 is not a factor.

If it doesn’t leave a remainder, then 4 is a factor

Divide 48 by divide 4

$\frac{90}{4}$

⇒ 22.5

Since when we divide 90 by 4, the answer is of the decimal form. Therefore it has a reminder.

Therefore, 4 is not the common factor of 90

⇒ 90

4 is a common factor of 48 but not the common factor of 90.

Page 27 Exercise 1.9 Problem 22

Given: To find if 6 is a common factor of 30 and 78

To solve this question, 30 and 78 by divide 6

If it leaves a remainder, then 6 is not a factor.

If it doesn’t leave a remainder, then 6 is a factor

Divide 30 by divide 6

⇒ $\frac{30}{6}$

⇒ 5

When dividing 30 by 6, there is no reminder.

Therefore, 6 is a common factor of 30

Divide 78 by divide 6

⇒ $\frac{78}{6}$

⇒ 13

When dividing 78 by 6, there is no reminder.

Therefore, 6 is a common factor of 78

6 is a common factor of 30 and 78.

Page 28 Exercise 9 Problem 23

Given:

Multiple of 3: 3,6,9,12,15,18,…

Multiple of 2: 2,4,6,8,10,12,…

To find –The first two common multiples of 3 and 2

The numbers which are both the multiple of 3 and 2 are the common multiples of 3 and 2.

By observing the multiples of 3 and 2, 6 and 12 are both the multiple of 3 and 2.

Therefore, 6 and 12 are the first two common multiples of 3 and 2.

The first two common multiples of 3 and 2 are 6 and 12.

Page 27 Exercise 1.9 Problem 24

Given:

Multiple of 8: 8,16,24,32…….

Multiple of 4: 4,8,12,16,…

To find the first two common multiples of 8 and 4

The numbers which are both the multiple of 8 and 4 are the common multiples of 8 and 4.

By observing the multiples of 8 and 4, 8and 16are both the multiples of 8 and 4.

Therefore, 8 and 16 are the first two common multiples of 8 and 4.

The first two common multiples of 8 and 4 are 8 and 16.

Page 27 Exercise 1.9 Problem 25

To find – The first two common multiples of 9 and 6

The numbers which are both the multiple of 9 and 6 are the common multiples of 9 and 6.

First, find the multiples of 9 and 6

Then observe the multiples of 9 and 6 and find their first two common multiples.

Multiple of 9: 9,18,27,36,45…

Multiple of 6:6,12,18,24,30,36…

By observing the multiples of 9 and 6, 18 and 36 are both the multiples of 9 and 6.

Therefore, 9 and 6 are the first two common multiples of 18 and 36.

The first two common multiples of 9 and 6 are 18 and 36.

Page 27 Exercise 1.9 Problem 26

To find – The first two common multiples of 8 and 6

The numbers which are both the multiple of 8 and 6 are the common multiples of 8 and 6.

First, find the multiples of 8 and 6

Then observe the multiples of 8 and 6 and find their first two common multiples.

Multiple of 8: 8,16,24,32,40,48,56,…

Multiple of 6: 6,12,18,24,30,36,42,48,54,…

By observing the multiples of 8 and 6, 24 and 48 are both the multiple of 8 and 6.

Therefore, 8 and 6 are the first two common multiples of 24 and 48.

The first two common multiples of 8 and 6 are 24 and 48.

Page 29 Exercise 1.10 Problem 1

Given: 8 + 12 + 20

To find – The value of each of the given expressions.

Add the first two-term

⇒ 8 + 12 + 20

⇒ 20 + 20

Now add the remaining terms

Therefore, the value of the expression, 8 + 12 + 20 = 40

Page 29 Exercise 1.10 Problem 2

Given: 40 − 14 −9

To find – The value of each of the given expressions.

Add the terms that are of the same signs.

⇒ 40 − 14 − 9

⇒ 40 − 23

Now subtract the remaining terms (since the sign of the greatest number is positive, the answer also has a positive sign.)

⇒ 17

Therefore, the value of the expression, 40 − 14 − 9 = 17

Page 29 Exercise 1.10 Problem 3

Given: 26 + 8 − 9

To find – The value of each of the given expressions.

Add the terms that are of the same signs.

⇒ 26 + 8 − 9

⇒ 34 − 9

Now subtract the remaining terms (since the sign of the greatest number is positive, the answer also has a positive sign.)

⇒ 25

Therefore, the value of the expression, 26 + 8 − 9 = 25

Page 29 Exercise 1.10 Problem 4

Given: 21−5 + 8

To find – The value of each of the given expressions.

Add the terms that are of the same signs.

⇒ 21 − 5 + 8

⇒ 29 − 5

Now subtract the remaining terms (since the sign of the greatest number is positive, the answer also has a positive sign.)

⇒ 24

Therefore, the value of the expression, 21−5 + 8 = 24

Page 29 Exercise 1.10 Problem 5

Given: 3 × 5 × 8

To find – The value of each of the given expressions.

First, multiply the first two terms.

⇒ 3 × 5 × 8

⇒ 15 × 8

Now multiply the remaining terms.

⇒ 120

Therefore, the value of the expression,3 × 5 × 8 = 120

Page 29 Exercise 1.10 Problem 6

Given: 36 ÷ 3 ÷ 4

To find – The value of each of the given expressions.

First, divide the first two terms.

⇒ 36 ÷ 3 ÷ 4

⇒ 12 ÷ 4

Now divide the remaining terms.

⇒ 3

Therefore, the value of the expression, 36 ÷ 3 ÷ 4 = 3

Page 29 Exercise 1.10 Problem 7

Given: 4 × 9 − 3

To find – The value of each of the given expressions.

Use BODMAS rule to find the value of the expression.

By BODMAS rule, first, multiply the first two terms.

⇒ 4 × 9 − 3

⇒ 36 − 3

Now subtract the remaining terms. (Since the sign of the greatest number is positive, the answer also has a positive sign.)

⇒ 36 − 3 = 33

Therefore, the value of the expression,4 × 9 − 3 = 33

Page 29 Exercise 1.10 Problem 8

Given: 64 ÷ 8 × 5

To find – The value of each of the given expressions.

By BODMAS rule, we have to first divide the given expression and then multiply.(since in the order of operations division is followed by multiplication)

First, divide the first two terms.

⇒ 64 ÷ 8 × 5

⇒ 8 × 5

Now multiply the remaining terms.

⇒ 40

Therefore, the value of the expression,64 ÷ 8 × 5 = 40

Page 29 Exercise 1.10 Problem 9

Given: 24 ÷ 6 × 8

To find – The value of each of the given expressions.

By BODMAS rule, we have to first divide the given expression and then multiply.(Since in the order of operations division is followed by multiplication)

First divide the first two terms.

⇒ 24 ÷ 6 × 8

⇒ 4 × 8

Now multiply the remaining terms.

⇒ 32

Therefore, the value of the expression, 24 ÷ 6 × 8 = 32

Page 29 Exercise 1.10 Problem 10

Given: 140 − 40 × 3

To find- The value of each of the given expressions.

By BODMAS rule, we have to first multiply the given expression and then subtract.(Since in the order of operations multiplication is followed by subtraction)

First, multiply the last two terms.

⇒ 140−40 × 3 = 140 − 120

Now subtract the remaining terms.

Here, since the greatest number is negative the answer holds a negative sign.

⇒ 140 − 120 = 20

Therefore, the value of the expression,140 − 40 × 3 = 20

Page 29 Exercise 1.10 Problem 11

Given: 46 + 32 ÷ 8

To find – The value of each of the given expression.

By BODMAS rule, we have to first divide the given expression and then add.(Since in the order of operations division is followed by addition)

First divide the last two terms.

⇒ 46 + 32 ÷ 8

⇒ 46 + 4

Now add the remaining terms.

⇒ 50

Therefore, the value of the expression,46 + 32 ÷ 8 = 50

Page 29 Exercise 1.10 Problem 12

Given: 100−60÷4

To find – The value of each of the given expressions.

By BODMAS rule, we have to first divide the given expression and then subtract.(Since in the order of operations division is followed by subtraction)

First divide the last two terms.

⇒ 100−60 ÷ 4

⇒ 100 −15

Now subtract the remaining terms. (since the sign of the greatest number is positive, the answer takes a positive sign.)

⇒ 85

Therefore, the value of the expression,100−60 ÷ 4 = 85

Page 29 Exercise 1.10 Problem 13

Given: 8 × 6 + 14

To find – The value of each of the given expressions.

By BODMAS rule, we have to first multiply the given expression and then add.(Since in the order of operations multiplication is followed by addition)

First multiply the first two terms.

⇒ 8 × 6 + 14

⇒ 48 + 14

Now add the remaining terms. (since the sign of the greatest number is positive, the answer takes a positive sign.)

⇒ 62

Therefore, the value of the expression,8 × 6 + 14 = 62

Page 29 Exercise 1.10 Problem 14

Given: 12 + 18 ÷ 6

To find – The value of each of the given expressions.

By BODMAS rule, we have to first divide the given expression and then add.(Since in the order of operations multiplication is followed by addition)

First divide the last two terms.

⇒ 12 + 18 ÷ 6

⇒12 + 3

Now add the remaining terms.

⇒15

Therefore, the value of the expression,12 + 18 ÷ 6 = 15

Page 29 Exercise 1.10 Problem 15

Given: 6 × 10 − 5

To find- The value of each of the given expressions.

By BODMAS rule, we have to first multiply the given expression and then subtract. (Since in the order of operations multiplication is followed by addition)

First multiply the first two terms.

⇒ 6 × 10 − 5

⇒ 60 − 5

Now subtract the remaining terms. (Since the sign of the greatest number is positive, the answer takes a positive sign.)

⇒ 55

Therefore, the value of the expression,6 × 10 − 5 = 55

Page 29 Exercise 1.10 Problem 16

Given: 72 + 6 × 6

To find – The value of each of the given expressions.

By BODMAS rule, we have to first multiply the given expression and then add.(Since in the order of operations multiplication is followed by addition)

First, multiply the last two terms.

⇒ 72 + 6 × 6

⇒ 72 + 36

Now add the remaining terms.

⇒ 108

Therefore, the value of the expression, 72 + 6 × 6 = 108

Page 30 Exercise 1.10 Problem 17

Given: 20 + 4 ÷ 4 − 4

To find the value of each of the given expressions.

By BODMAS rule, we have to first divide the given expression, then add and then subtract. (Since in the order of operations multiplication is followed by addition and then by subtraction)

First divide the middle two terms.

⇒ 20 + (4 ÷ 4) − 4

⇒ 20 + 1 − 4

Now add the first two terms and then subtract.(Since the sign of the greatest number is positive, the answer takes a positive sign.)

⇒ 21 − 4

⇒ 17

Therefore, value of the expression,20 + 4 ÷ 4 − 4 = 17

Page 30 Exercise 1.10 Problem 18

Given: 6 × 2−10 ÷ 5

To find – The value of each of the given expression.

By BODMAS rule, we have to first divide the given expression, then multiply and then subtract.(Since in the order of operations division is followed by multiplication and then by subtraction)

First divide the last two terms.

⇒ 6 × 2 − 10 ÷ 5

⇒ 6 × 2−2

Now multiply the first two terms and then subtract. (Since the sign of the greatest number is positive, the answer takes a positive sign.)

⇒ 12 − 2

⇒ 10

Therefore, the value of the expression,6 × 2−10 ÷ 5 = 10

Page 30 Exercise 1.10 Problem 19

Given: 80−36 ÷ 4 × 3

To find – The value of each of the given expressions.

By BODMAS rule, we have to first divide the given expression, then multiply and then subtract. (Since in the order of operations division is followed by multiplication and then by subtraction)

First divide the middle two terms.

⇒ 80 − (36÷4) × 3

⇒ 80−9 × 3

Now multiply the last two terms and then subtract.(Since the sign of the greatest number is positive, the answer takes a positive sign.)

⇒ 80 − 27

⇒ 53

Therefore, the value of the expression, 80−36 ÷ 4 × 3 = 53

Page 30 Exercise 1.10 Problem 20

Given: 32 + 8 + 10 × 2.

To find – The value of each of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be multiplication and then addition.

Referring to the question

32 + 8 + 10 × 2

Using BODMAS

32 + 8 + 20

= 60

Hence, the value of 32 + 8 + 10 × 2 is 60.

Page 30 Exercise 1.10 Problem 21

Given: 52−35 ÷ 7 − 7 × 2.

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be division, then multiplication, and then addition and subtraction.

Referring to the question

52 − 35 ÷ 7 − 7 × 2

Using BODMAS

52−5−14

= 33

Hence, the value of 52−35÷7−7 × 2 is 33.

Page 30 Exercise 1.10 Problem 22

Given: 9 × 8 − 6 × 10.

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be multiplication and subtraction.

Referring to the question

9 × 8−6 × 10

Using BODMAS

72 − 60

Hence, the value of 9 × 8−6 × 10 is 12.

Page 30 Exercise 1.10 Problem 23

Given: 7 × 8 + 24 ÷ 8.

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be division, then multiplication and then addition.

Referring to the question

7 × 8 + 24 ÷ 8

Using BODMAS

56 + 3

= 59

Hence, the value of 7 × 8 + 24 ÷ 8 is 59.

Page 30 Exercise 1.10 Problem 24

Given: 63÷9 + 20 ÷ 10.

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be division and then addition.

Referring to the question

63 ÷ 9 + 20 ÷ 10

Using BODMAS

7 + 2

= 9

Hence, the value of 63 ÷ 9 + 20 ÷ 10 is 9.

Page 30 Exercise 1.10 Problem 25

Given:

To find – The value of 12 + 49 ÷ 7 × 3.

Use the strategy of BODMAS.

Thus, the first operation will be division, then multiplication, and then addition.

Referring to the question

12 + 49 ÷ 7 × 3

Using BODMAS

12 + 21

= 33

Hence, the value of 12 + 49 ÷ 7 × 3 is 33.

Page 30 Exercise 1.10 Problem 26

Given: 5×8−32÷4+18.

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be division, then multiplication, and then addition and subtraction.

Referring to the question

5 × 8 − 32 ÷ 4 + 18

Using BODMAS

40 − 8 + 18

= 50

Hence, the value of 5 × 8 − 32 ÷ 4 + 18 is 50.

Page 31 Exercise 1. 11 Problem 1

Given: 9 + (26−15).

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be opening the brackets followed by addition and subtraction.

Referring to the question

9 + (26 − 15)

Using BODMAS

9 + 26 − 15

= 20

Hence, the value of 9 + (26−15) is 20.

Page 31 Exercise 1. 11 Problem 2

Given: 90−(4 + 6).

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be opening the brackets followed by addition and subtraction.

Referring to the question

90− (4 + 6)

Using BODMAS

90 − 4 − 6

= 80

Hence, the value of 90 − (4 + 6) is 80.

Page 31 Exercise 1. 11 Problem 3

Given: 12 − (10 − 8).

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be opening the brackets followed by addition and subtraction.

Referring to the question

12 − (10 − 8)

Using BODMAS

12 − 10 + 8

= 10

Hence, the value of 12−(10−8) is 10.

Page 31 Exercise 1. 11 Problem 4

Given: (31 − 20) − 8

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be opening the brackets followed by addition and subtraction.

Referring to the question

(31−20)−8

Using BODMAS

31−20−8

= 3

Hence, the value of (31−20)−8 is 3.

Page 31 Exercise 1. 11 Problem 5

Given: 8 × (3 × 2).

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be opening the brackets followed by multiplication.

Referring to the question

8 × (3 × 2)

Using BODMAS

8 × 3 × 2

= 48

Hence, the value of 8 × (3 × 2) is 48.

Page 31 Exercise 1. 11 Problem 6

Given: 20 ÷ (4 ÷ 2).

To find – The value of the given expression.

Use the strategy of BODMAS. Thus, the first operation will be solving the operation in the bracket followed by division.

Referring to the question

20 ÷ (4 ÷ 2)

Using BODMAS

20 ÷ 2

= 10

Hence, the value of 20 ÷ (4 ÷ 2) is 10.

Page 31 Exercise 1. 11 Problem 7

Given: 9 × (15 ÷ 3)

To find – The value of the given expression.

Use the strategy of BODMAS. Thus, the first operation will be solving the operation in the bracket followed by multiplication.

Referring to the question

9 × (15 ÷ 3)

Using BODMAS

9 × 5

= 45

Hence, the value of 9 × (15 ÷ 3) is 45.

Page 31 Exercise 1. 11 Problem 8

Given: 15 ÷ (5 × 3).

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division.

Referring to the question

15 ÷ (5 × 3)

Using BODMAS

15 ÷ 15

= 1

Hence, the value of 15 ÷ (5 ×3 ) is 1.

Page 31 Exercise 1. 11 Problem 9

Given: (9+6)÷5

To find the value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division.

Referring to the question

(9 + 6) ÷ 5

Using BODMAS

15 ÷ 5

= 3

Hence, the value of (9 + 6) ÷ 5 is 3.

Page 31 Exercise 1. 11 Problem 10

Given: 2 × (9−4)

To find – The value of

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication.

Referring to the question

2 × (9−4)

Using BODMAS

2 × 5

= 10

Hence, the value of 2 × (9−4) is 10.

Page 31 Exercise 1. 11 Problem 11

Given: 12 ÷ (8−6)

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division.

Referring to the question

12 ÷ (8−6)

Using BODMAS

12 ÷ 2

= 6

Hence, the value of 12 ÷ (8−6) is 6.

Page 31 Exercise 1. 11 Problem 12

Given: (4 + 6) × 5.

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication.

Referring to the question

(4 + 6) × 5

Using BODMAS

10 × 5

= 50

Hence, the value of (4 + 6) × 5 is 50.

Page 31 Exercise 1. 11 Problem 13

Given: 10 × (15 ÷ 5)

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication.

Referring to the question

10 × (15 ÷ 5)

Using BODMAS

10 × 3

= 30

Hence, the value of 10 × (15 ÷ 5) is 30.

Page 31 Exercise 1. 11 Problem 14

Given: (51 − 44) ÷ 7

To find – The value of the given expression

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division.

Referring to the question

(51 − 44) ÷ 7

Using BODMAS

7 ÷ 7

= 1

Hence, the value of (51− 44) ÷ 7 is 1.

Page 31 Exercise 1. 11 Problem 15

Given: 72 ÷ (9 − 3)

To find – The value of the given expression 72÷(9−3).

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division.

Referring to the question

72 ÷ (9−3)

Using BODMAS

72 ÷ 6

= 12

Hence, the value of 72 ÷ (9−3) is 12.

Page 31 Exercise 1. 11 Problem 16

Given: (28 − 18) × 10

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication.

Referring to the question

(28−18) ×10

Using BODMAS

10 × 10

= 100

Hence, the value of (28−18)×10 is 100.

Page 32 Exercise 1. 11 Problem 17

Given: 20 + (8 + 4) ÷ 3 .

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division and addition.

Referring to the question

20 + (8 + 4) ÷ 3

Using BODMAS

20 + 12 ÷ 3

⇒ 20 + 4

= 24

Hence, the value of 20+(8+4)÷3 is 24.

Page 32 Exercise 1. 11 Problem 18

Given: 16 + (9 − 3)× 5

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication and addition.

Referring to the question

16 + (9 − 3) × 5

Using BODMAS

16 + 6 × 5

⇒ 16 + 30

= 46

Hence, the value of 16 + (9−3) × 5 is 46.

Page 32 Exercise 1. 11 Problem 19

Given: 3 × (4 + 2) ÷ 2

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division and then multiplication.

Referring to the question

3 × (4 + 2) ÷ 2

Using BODMAS

3 × 6 ÷ 2

⇒ 3 × 3

Hence, the value of 3 × (4 + 2) ÷ 2 is 9.

Page 32 Exercise 1. 11 Problem 20

Given: 7 × (13 − 6) −19

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication and subtraction.

Referring to the question

7 × (13 − 6) − 19

Using BODMAS

7 × 7−19

⇒ 49 − 19

= 30

Hence, the value of 7 × (13−6)−19 is 30.

Page 32 Exercise 1. 11 Problem 21

Given: 60 + (18 + 7) ÷ 5

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division and addition.

Referring to the question

60 + (18 + 7) ÷ 5

Using BODMAS

60 + 25 ÷ 5

⇒ 60 + 5

= 65

Hence, the value of 60 + (18 + 7) ÷ 5 is 65.

Page 32 Exercise 1. 11 Problem 22

Given: 8 × (11 − 8) ÷ 6

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication and division.

Referring to the question

8 × (11− 8) ÷ 6

Using BODMAS

8 × 3 ÷ 6

⇒ 24 ÷ 6

Hence, the value of 8 × (11− 8) ÷ 6 is 4.

Page 32 Exercise 1. 11 Problem 23

Given: 24 ÷ 6 + 3 × (6−4)

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division and multiplication, and then addition.

Referring to the question

24 ÷ 6 + 3 × (6−4)

Using BODMAS

24 ÷ 6 + 3 × 2

⇒ 4 + 6

= 10

Hence, the value of 24 ÷ 6 + 3 × (6−4) is 10.

Page 32 Exercise 1. 11 Problem 24

Given: 10 + (28 − 8) ÷ 5 × 2

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division and multiplication, and then addition.

Referring to the question

10 + (28−8) ÷ 5 × 2

Using BODMAS

10 + 20 ÷ 5 × 2

⇒ 10 + 4 × 2

⇒ 10 + 8

= 18

Hence, the value of 10 + (28−8) ÷ 5 × 2 is 18.

Page 32 Exercise 1. 11 Problem 25

Given: 15×(8+2)−25÷5

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by division and multiplication, and then subtraction.

Referring to the question

15 × (8 + 2)−25 ÷ 5

Using BODMAS

15 × 10 − 25 ÷ 5

⇒ 150 − 5

= 145

Hence, the value of 15 × (8 + 2) − 25 ÷ 5 is 145.

Page 32 Exercise 1. 11 Problem 26

Given: 100−8 × (5 + 2) ÷ 4

To find – The value of the given expression.

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication and division, and then subtraction.

Referring to the question

100 − 8 × (5 + 2) ÷ 4

Using BODMAS

100 − 8 × 7 ÷ 4

⇒ 100 − 14

=86

Hence, the value of 100−8 × (5 + 2) ÷ 4 is 86.

Page 33 Exercise 1. 12 Problem 1

Given: Total number of beads = 50

Number of beads used to make necklace = 32

Number of beads bought = 18

To Find: Number of beads left

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by addition.

Referring to the question, the following is the required expression

(50−32) + 18

Using BODMAS

18 + 18

= 36

Hence, the number of beads left is 36.

Page 33 Exercise 1. 12 Problem 2

Given: Total number of muffins = 20

Number of muffins eaten = 5

Number of muffins given away = 10

To Find: Number of muffins left

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by subtraction.

Referring to the question, the following is the required expression

20−(5 + 10)

Using BODMAS

20 − 15

= 5

Hence, the number of muffins left with Patrick is 5.

Page 33 Exercise 1. 12 Problem 3

Given: Total number of baseball cards with Sean = 15

Number of baseball cards with Adam = twice the number of cards with Sean

Number of baseball cards Adam bought = 10

To Find: Number of baseball cards with Adam

Use the strategy of BODMAS.

Thus, the first operation will be solving the operation in the bracket followed by multiplication.

Referring to the question, the following is the required expression

(15 × 2) + 10

Using BODMAS

30 + 10

= 40

Hence, the number of baseball cards that Adam has is 40

Page 34 Exercise 1. 12 Problem 4

To Check: 24 = 3 × 8

Use the strategy of BODMAS.

Compare the LHS and RHS.

Referring to the question

LHS = 24

Using BODMAS

RHS = 3 × 8

= 24

Therefore, LHS = RHS so the given equation is true.

Page 34 Exercise 1. 12 Problem 5

To Check: 9 × 11 = 88

Use the strategy of BODMAS.

Compare the LHS and RHS.

Referring to the question

RHS =88

Using BODMAS

LHS = 9 × 11

= 99

Therefore, LHS ≠ RHS so the given equation is false.

Page 34 Exercise 1. 12 Problem 6

To Check: 8 + 35 = (3 × 5) + 8

Use the strategy of BODMAS.

Compare the LHS and RHS.

Referring to the question and using BODMAS

LHS = 8 + 35

⇒ 43

Referring to the question and using BODMAS

RHS = (3 × 5) + 8

⇒ 15 + 8

⇒ 23

Therefore, LHS ≠ RHS so the given equation is false.

Page 34 Exercise 1. 12 Problem 7

To Check: 16−9 = (7 × 8) − 49

Use the strategy of BODMAS.

Compare the LHS and RHS.

Referring to the question and using BODMAS

LHS = 16 − 9

⇒ 7

Referring to the question and using BODMAS

RHS = (7 × 8) − 49

⇒ 56 − 49

⇒ 7

Therefore, LHS = RHS so the given equation is true.

Page 34 Exercise 1. 12 Problem 8

To find – The value of (1×5)×10=5×10

Use the strategy of BODMAS.

Thus, the first operation will be multiplication in the brackets.

Check LHS = RHS

Referring to the question and using BODMAS

LHS = (1 × 5) × 10

= 5 × 10

= 50

Further Simplifying the equation

RHS = 5 × 10

= 50

So, LHS = RHS

Therefore, the equation (1 × 5) × 10 = 5 × 10 is True as

LHS=RHS

Page 34 Exercise 1. 12 Problem 9

To find – The value of 8+6=(2×3)+(5×2)

Use the strategy of BODMAS.

Thus, the first operation will be multiplication in the brackets.

Check LHS = RHS

Referring to the question and using BODMAS

LHS = 8 + 6

=14

Further Simplifying the equation

RHS = (2 × 3) + (5 × 2)

= 6 + 10

= 16

So, LHS ≠ RHS

Therefore, the equation 8+6=(2×3)+(5×2) is False as LHS ≠ RHS

Page 34 Exercise 1. 12 Problem 10

To find – The value of (20÷5)+2=(2×4)−(1×2)

Use the strategy of BODMAS.

Thus, the first operation will be multiplication in the brackets.

Check LHS = RHS

Referring to the question and using BODMAS

LHS = (20 ÷ 5) + 2

= 4 + 2

= 6

Further Simplifying the equation

RHS = (2 × 4) − (1 × 2)

= 8 − 2

= 6

So, LHS = RHS

Therefore, the equation (20 ÷ 5) + 2 = (2 × 4) − (1 × 2) is True as LHS = RHS

Page 34 Exercise 1.12 Problem 11

To find – The value of the blank in 36−(20−7)=……..−13

Use the strategy of BODMAS.

Thus, the first operation will be subtraction in the brackets.

Check LHS=RHS

Solve LHS to find the blank.

Referring to the question and using BODMAS

LHS = 36 − (20 − 7)

= 36 −13

Further Simplifying and comparing the equation

RHS= ………−13

So, Comparing LHS and RHS the value of the blank is 36

Therefore, the value of the blank is 36 making the equation True.

Page 34 Exercise 1.12 Problem 12

To find – The value of the blank in (5+8)×(4+6)=……..×10

Use the strategy of BODMAS.

Thus, the first operation will be an addition in the brackets.

Check LHS = RHS

Solve LHS to find the blank.

Referring to the question and using BODMAS

LHS = (5 + 8) × (4 + 6)

= 13 × 10

Further Simplifying and comparing the equation

RHS=………×10

So, Comparing LHS and RHS the value of the blank is 13

Therefore, the value of the blank is 13 making the equation True.

Page 34 Exercise 1.12 Problem 13

To find – The value of the blank in 100×(9÷3)=……..×3

Use the strategy of BODMAS.

Thus, the first operation will be division in the brackets.

Check LHS=RHS

Solve LHS to find the blank.

Referring to the question and using BODMAS

LHS = 100 × (9 ÷ 3)

= 100 × 3

Further Simplifying and comparing the equation

RHS =………×3

So, Comparing LHS and RHS the value of the blank is 100

Therefore, the value of the blank is 100 making the equation True.

Page 34 Exercise 1.12 Problem 14

To find – The value of the blank in (26−11)÷5 × 4 =……..×4

Use the strategy of BODMAS.

Thus, the first operation will be division in the brackets.

Check LHS = RHS

Solve LHS to find the blank.

Referring to the question and using BODMAS

LHS = (26−11) ÷ 5 × 4

= 15 ÷ 5 × 4

= 3 × 4

Further Simplifying and comparing the equation

RHS =………×4

So, Comparing LHS and RHS the value of the blank is 3

Therefore, the value of the blank is 3 making the equation True.

Page 34 Exercise 1.12 Problem 15

To insert the parentheses in the given equation 2+4÷2=3

Use the strategy of BODMAS to insert the brackets.

Check LHS = RHS

Solve LHS using parentheses to make it true for the equation.

Adding the parentheses at the addition rule to make the equation true

LHS = 2 + 4 ÷ 2

= (2 + 4) ÷ 2

Further Simplifying and comparing the equation

RHS = 3

LHS = (2 + 4) ÷ 2

= 6 ÷ 2

= 3

So, the parentheses will be inserted at addition making LHS as (2 + 4) ÷ 2

Therefore, the parentheses will be inserted at addition making LHS as (2+4)÷2 making the equation True.

Page 34 Exercise 1.12 Problem 16

To insert the parentheses in the given equation 6−2×3=0

Use the strategy of BODMAS to insert the brackets.

Check LHS = RHS

Solve LHS using parentheses to make it true for the equation.

Adding the parentheses at the multiplication rule to make the equation true

LHS = 6 − 2 × 3

= 6 − (2 × 3)

Further Simplifying and comparing the equation

RHS = 0

LHS = 6 − (2× 3)

= 6−6

= 0

So, the parentheses will be inserted at multiplication making LHS as 6−(2 × 3)

Therefore, the parentheses will be inserted at multiplication making LHS as 6−(2×3) making the equation True.

Page 34 Exercise 1.12 Problem 17

To insert the parentheses in the given equation 2 × 4− 3 + 2 = 7

Use the strategy of BODMAS to insert the brackets.

Check LHS = RHS

Solve LHS using parentheses to make it true for the equation.

Adding the parentheses at the multiplication rule to make the equation true

LHS = 2 × 4 − 3 + 2

= (2 × 4) − 3 + 2

Further Simplifying and comparing the equation

RHS = 7

LHS = (2 × 4) − 3 + 2

=8 − 3 + 2

= 5 + 2

= 7

So, the parentheses will be inserted at multiplication making LHS as (2 × 4)−3 + 2

Therefore, the parentheses will be inserted at multiplication making LHS as (2 × 4)−3 + 2 making the equation True.

Page 34 Exercise 1.12 Problem 18

To insert the parentheses in the given equation 2 × 4− 3 + 2 = 4

Use the strategy of BODMAS to insert the brackets.

Check LHS = RHS

Solve LHS using parentheses to make it true for the equation.

Adding the parentheses at the addition rule to make the equation true

LHS = 2 × 4 − 3 + 2

= 2 × (4−3) + 2

Further Simplifying and comparing the equation

RHS = 4

LHS = 2 × (4 − 3) + 2

= 2 × 1 + 2

= 2 + 2

= 4

So, the parentheses will be inserted at subtraction making LHS as 2 × (4 − 3) + 2

Therefore, the parentheses will be inserted at subtraction making LHS as 2 × (4 − 3) + 2 making the equation True.

Page 34 Exercise 1.12 Problem 19

To insert the parentheses in the given equation 2 × 4 − 3 + 2 = 3

Use the strategy of BODMAS to insert the brackets.

Check LHS = RHS

Solve LHS using parentheses to make it true for the equation.

Adding the parentheses at the addition rule to make the equation true

LHS = 2 × 4 − 3 + 2

= 2 × 4 − (3 + 2)

Further Simplifying and comparing the equation

RHS = 3

LHS = 2 × 4−(3 + 2)

= 8 − 5

= 3

So, the parentheses will be inserted at addition making LHS as 2 × 4 − (3 + 2)

Therefore, the parentheses will be inserted at addition making LHS as 2 × 4 − (3 + 2) making the equation True.

Glencoe Math Course 2 Volume 1 Common Core Student Edition Chapter 4 Rational Numbers Exercise 4.2

June 27, 2024March 11, 2023 by Marksparks

Glencoe Math Course 2 Volume 1 Common Core Chapter 4 Rational Numbers

Page 271 Exercise 1 Problem 1

To add or subtract the fractions we see the denominator.

If the denominators are the same we only function with the numerator.

If the denominators are not the same we take the L.C.M of it.

Multiplication: Multiply the numerator with the numerator.

Multiply the denominator with the denominator.

Divide: Dividing a fraction by another fraction is the same as multiplying the fraction by the reciprocal (inverse) of the other.

Here we have shown the addition, subtraction, multiplication, and division of two fractions

Page 271 Exercise 1 Problem 2

The Greek letter π (pi) represents the nonterminating and nonrepeating number whose first few digits are 3.14.….

This number is an irrational number.

Using the internet we have to find the value of π

The fractional value of π is $\frac{22}{7}$

We divide them
$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 271 Exercise 1 Answer$

The value of π is 3.1428… and so on. Because the value of π is an irrational number, it is an irrational number.

The value of π is presented as 3.14159, whose ration is unknown when it is originally introduced in the earlier lessons.

The most common estimate is in the form of a rational number, such as 227=3.1428571428571 which is the closest but not exact approximation to the actual value of π

Therefore, the value of π: is 3.1428. The non-terminating and non-repeating decimal expansion ofπ makes it an irrational number.

Page 272 Exercise 1 Problem 3

Given: $\frac{5}{6}$ ◯ $\frac{7}{9}$

To find – Fill in the ◯ with <, > or=

We know that

$\frac{5}{6}$ ◯ $\frac{7}{9}$

The LCD of the denominators 6 and 9 is 18

$\frac{5}{6}$ = $\frac{5×3}{6×3}$

⇒ $\frac{15}{18}$

$\frac{7}{9}$ = $\frac{7×2}{9×2}$

⇒ $\frac{14}{18}$

Since, $\frac{15}{18}$ > $\frac{14}{18}$, $\frac{5}{6}$ > $\frac{7}{9}$

Finally, We compare the numerators of each fraction and order them from least to largest or greatest to least. $\frac{15}{18}$ > $\frac{14}{18}$, $\frac{5}{6}$ > $\frac{7}{9}$

Given: $\frac{1}{5}$ ◯ $\frac{7}{50}$

To find – Fill in the ◯ with <, > or =

$\frac{1}{5}$ ◯ $\frac{7}{50}$

The LCD of the denominators 5 and 50 is 50

$\frac{1}{5}$ = $\frac{1×10}{5×10}$

⇒ $\frac{10}{50}$

⇒ $\frac{1}{5}$

$\frac{7}{50}$ = $\frac{7×1}{50×1}$

⇒ $\frac{7}{50}$

$\frac{10}{50}$>$\frac{7}{50}$, $\frac{1}{5}$>$\frac{7}{50}$

Finally, We compare the numerators of each fraction and order them from least to largest or greatest to least. $\frac{10}{50}$>$\frac{7}{50}$, $\frac{1}{5}$>$\frac{7}{50}$

Given: − $\frac{9}{16}$ ◯ −$\frac{7}{10}$

To find – Fill in the◯ with <,>, or =

We know that

$\frac{9}{16}$ ◯−$\frac{7}{10}$

The LCD of the denominators 16 and 10 is 80

$\frac{9}{16}$ = $\frac{−9×5}{16×5}$

⇒ −$\frac{45}{50}$

−$\frac{7}{10}$= −$\frac{7×8}{10×8}$

⇒ −$\frac{56}{80}$

Since, −$\frac{45}{50}$>$\frac{56}{80}$,−$\frac{9}{16}$> −$\frac{7}{10}$

Finally, We compare the numerators of each fraction and order them from least to largest or greatest to least. −$\frac{45}{50}$>$\frac{56}{80}$,−$\frac{9}{16}$> −$\frac{7}{10}$

Given:

In a second-period class,37.5% of students like to bowl. In a fifth-period class, 12 out of 29 students like to bowl.

Convert the given percentage to decimal and then find which class does a greater fraction of the students like to bowl

In the second period class which means 5 th period37.5% of students like to bowl.

37.5% = 0.375

12 out of 29. Which means

$\frac{12}{29}$ = 0.1413.

While comparing both the value.

0.375 < 0.413.

Hence, in the fifth-period class the fraction of students who like to bowl is greater than the second-period class of students.

Given:

{23%,0.21,$\frac{1}{4}$$\frac{1}{5}$}

First, convert % to decimal Then convert the fractions to decimal and then arrange them from least to greatest.

Convert % to a decimal. 23% = 0.23

This number is already in decimal. 0.21

Convert fractions to decimals.

$\frac{1}{4}$ = 0.25

$\frac{1}{5}$ = 0.2

The order from least to greatest is 0.2,0.21,0.23,0.25

Page 274 Exercise 1 Problem 4

Given: The fraction −$\frac{4}{5}$, −$\frac{1}{5}$

To find – Make the true sentence

Since we can write

−4 < −1

And since the denominator are the same, we know that

−$\frac{4}{5}$<−$\frac{1}{5}$

The value of true sentence is −$\frac{4}{5}$<−$\frac{1}{5}$

Page 274 Exercise 2 Problem 5

Given:

Two fractions are : 1$\frac{3}{4}$, 1$\frac{5}{8}$

To find – Make the true sentence

Since 0.75>0.625

And since the denominator are the same, we know that

$\frac{3}{4}$ = 0.75 >$\frac{5}{8}$

= 0.625

Add 1 to both sides of the inequality

1$\frac{3}{4}$ > 1$\frac{5}{8}$

0.75 > 0.625

True sentence of this fraction is 1$\frac{3}{4}$ > 1$\frac{5}{8}$, 0.75 > 0.625

Page 274 Exercise 3 Problem 6

Given: Elliot saves the goals $\frac{3}{4}$

Shanna saves the goals$\frac{7}{11}$

To find – Better average

Since we can write

0.75 > 0.636

And since the denominator are the same, we know that

$\frac{3}{4}$ = 0.75 > $\frac{7}{11}$ = 0.636

0.75 > 0.636

Elliot saves the goal better than Shanna, Elliot > Shanna.

Page 274 Exercise 4 Problem 7

Given:

The insects inches are 0.02, $\frac{1}{8}$ , 0.1, $\frac{2}{3}$

To find – List the insects from least to greatest

Since 0.02, $\frac{1}{8}$ = 0.125, 0.1, $\frac{2}{3}$ = 0.667

The length of four insects from least to greatest is

0.02<0.1<0.125<0.667

The length of four insects from least to greatest is 0.02 < 0.1 < 0.125 < 0.667, 0.02 < 0.1 < $\frac{1}{8}$ < $\frac{2}{3}$.

Page 274 Exercise 5 Problem 8

Add the fractions to the number line, the fraction that is the most to the right is the greatest fraction.

Hence we can compare two fractions by using the above method.

Page 275 Exercise 3 Problem 9

Given: The fractions 6$\frac{2}{3}$ , 6$\frac{1}{2}$

To find – Make a true sentence

Since we can write

4>3

And since the denominator are the same, we know that

$\frac{2}{3}$ = $\frac{4}{6}$>$\frac{3}{6}$ = $\frac{1}{2}$

Add 6 to both sides of the inequality

The fraction value is 6$\frac{2}{3}$>6$\frac{1}{2}$

Page 275 Exercise 4 Problem 10

Given: −$\frac{17}{24}$ and − $\frac{11}{12}$

Make the denominators the same for both the fractions and then relate them with <,> or =

Make the denominators of the two fractions the same by multiplying and dividing with the appropriate number.

−$\frac{17}{24}$ and − $\frac{11}{12}$

−$\frac{17}{24}$× $\frac{12}{12}$ > − $\frac{11}{12}$× $\frac{24}{24}$

−$\frac{288}{24}$ > −$\frac{264}{288}$

The true sentence is −$\frac{17}{24}$ > − $\frac{11}{12}$.

The true sentence is −$\frac{17}{24}$ > − $\frac{11}{12}$.

Page 275 Exercise 5 Problem 11

Given: Meg answered in the first quiz is 92%, next quiz is

$\frac{27}{30}$ First, convert the decimal to percentage and find which quiz she answered the better score

The number of questions answered in the second quiz is 27 out of 30

Then, $\frac{27}{30}$ = 0.9

Convert to percentage

= 0.9 × 100

= 90 %

Therefore, Meg answered 90 % of the questions correctly in the second quiz.

But in the First quiz, Meg answered 92 % of the questions correctly. 92 %>90%

So, the First quiz is better than the second quiz.

Meg answered the First quiz better than the second quiz.

Page 275 Exercise 6 Problem 12

Given: The sets are 0.23,19

To find – Set least to greatest 0.23,19%, $\frac{1}{5}$

Since Percentage converted into number is divided by hundreds

0.23, $\frac{19}{100}$ = 1.9, $\frac{1}{5}$ = 0.2

And since set least to greatest is

0.2 < 0.23 < 1.9

Sets least to greatest is 0.2< 0.23<1.9, $\frac{1}{5}$ < 0.23 < 19

Page 275 Exercise 7 Problem 13

Given: Sets of numbers

To find – Least to the greatest

Since The value of −$\frac{5}{8}$

= −0.625

And since the value arranged from least to greatest is given below

{−0.625<−0.62<−0.615}

Sets arrange least to greatest is {−0.625<−0.62<−0.615} , {−$\frac{5}{8}$ <−0.62<−0.615}

Page 275 Exercise 8 Problem 14

Given:

The sixth-graders have raised 52 % of their goal amount. The seventh- and eighth-graders have raised 0.57 and $\frac{2}{5}$ of their goal amounts, respectively

Convert the percentage to decimal and find the order of least to greatest of their goal amounts.

The amount raised by the eighth graders = $\frac{2}{5}$ of their goal amount.

= 0.4 of their goal amount.

The amount raised by sixth graders =52 % of their goal amount

$\frac{52}{100}$

= 0.52

The amount raised by seventh graders = 0.57

Compare the amount raised by the sixth, seventh, and eighth graders

Arranging, the least to greatest is:

0.4 < 0.52 > 0.57

The classes in order from least to greatest of their goal amounts is Eighth grade < Sixth grade < seventh grade.

Page 275 Exercise 9 Problem 15

Given: Two mixed fraction

1$\frac{7}{12}$ gallons, 1$\frac{5}{8}$

Make the denominators of the two fractions the same by multiplying and dividing with the appropriate number.

1$\frac{7}{12}$< 1$\frac{5}{8}$

$\frac{7}{12}$ × $\frac{8}{8}$ < $\frac{5}{8}$ × $\frac{12}{12}$

$\frac{56}{96}$ < $\frac{60}{96}$

1$\frac{7}{12}$ < 1$\frac{5}{8}$

The True sentence is 1$\frac{7}{12}$ < 1$\frac{5}{8}$

Page 275 Exercise 10 Problem 16

Given: Two mixed fraction

To find – The greater number between two fractions

Since we have two different times but one in a fraction

We 2$\frac{5}{6}$ = $\frac{12+5}{6}$

$\frac{17}{6}$ = 2.83

⇒ 2.83 > 2.8

2$\frac{5}{6}$> 2.8

The fraction is greater than number

True sentence is 2$\frac{5}{6}$> 2.8.

Page 276 Exercise 11 Problem 17

According to the graphic novel frame, the total width of the closet organizer is

69 $\frac{1}{8}$ = $\frac{553}{8}$

= 69.125

The total width of the closet organizer is

69$\frac{3}{4}$ = $\frac{279}{4}$

= 69.75

69.125 < 69.75

That is total width of the closet<total width of the closet

That means the closet can fit into the organizer.

Finally, we concluded That the closet can fit into the organizer.

Page 276 Exercise 12 Problem 18

Here it is given that

12 out of 15 ⇒ $\frac{12}{15}$ = 0.8

0.08 ≠ 0.8

80% = $\frac{80}{100}$

= 0.8

$\frac{4}{5}$ = 0.8

The ratio that does not have the same value as the other three is $\frac{4}{5}$ = 0.8

The ratio that does not have the same value as the other three is 0.08.

Page 277 Exercise 15 Problem 19

Given: Two simple fractions

To find – True sentence or sign

Since denominators are same easily put the value-based in numerators −$\frac{5}{7}$<$\frac{2}{7}$

The symbol of this statement is <

The true sentence of this fraction is −$\frac{5}{7}$<$\frac{2}{7}$

Page 277 Exercise 16 Problem 20

Given:

Two simple fractions : −3$\frac{2}{3}$ and −3$\frac{2}{3}$

To find – True sentence or sign

Denominators are different so take simple fractions in cross multiplication method

$\frac{2}{3}$ × $\frac{6}{6}$= $\frac{12}{18}$

$\frac{4}{6}$ × $\frac{3}{3}$

= $\frac{12}{18}$

$\frac{12}{18}$ = $\frac{12}{18}$

$\frac{2}{3}$ = $\frac{4}{6}$

The values are same so we put an equal sign

The values are same so we put an equal sign $\frac{2}{3}$ = $\frac{4}{6}$

Page 277 Exercise 17 Problem 21

Given:

Two simple fractions : $\frac{4}{7}$and $\frac{5}{8}$

To find – True sentence or sign

$\frac{4}{7}$ = $\frac{4×8}{7×8}$

= $\frac{32}{56}$

$\frac{5}{8}$ = $\frac{5×7}{8×7}$

= $\frac{35}{56}$

$\frac{32}{56}$ < $\frac{35}{56}$

$\frac{4}{7}$ < $\frac{5}{8}$

The sign of this fraction is <

The true sentence of this fraction is $\frac{4}{7}$ < $\frac{5}{8}$

Page 277 Exercise 18 Problem 22

Given:

Two mixed fractions : 2$\frac{3}{4}$ and 2$\frac{2}{3}$

To find – True sentence or sign

Since denominators are different we take cross multiplication

2$\frac{3}{4}$ = $\frac{3×3}{4×3}$

= $\frac{9}{12}$

2$\frac{2}{3}$ = $\frac{2×4}{3×4}$

= $\frac{8}{12}$

$\frac{9}{12}$>$\frac{8}{12}$

= 2$\frac{3}{4}$> 2$\frac{2}{3}$

Hence the true value of the sign is

Hence the true value of sign is 2$\frac{3}{4}$ > 2$\frac{2}{3}$.

Page 277 Exercise 19 Problem 23

Given:

Garcia made − $\frac{4}{15}$

Jim missed− $\frac{6}{16}$

To find – a greater fraction of the time

Garcia and Jim throw different values we find who made free throw a greater fraction

$\frac{4}{15}$ = $\frac{4×16}{15×16}$

= $\frac{64}{240}$

$\frac{6}{16}$ = $\frac{6×15}{16×15}$

= $\frac{90}{240}$

⇒ $\frac{64}{240}$<$\frac{90}{240}$

⇒ $\frac{4}{15}$<$\frac{6}{16}$

Jim throws better than Garcia $\frac{4}{15}$<$\frac{6}{16}$.

Page 277 Exercise 20 Problem 24

Given:

Sets of numbers fraction : {7.49,7$\frac{49}{50}$,7.5}

To find – Least to the greatest

Since we convert fractions into numbers

7$\frac{49}{50}$ = $\frac{350+49}{50}$

= 7.98

{0.75 < 7.49 < 7.98}

The order of a set of fractions from least to greatest {0.75 < 7.49 < 7.98}

Page 277 Exercise 21 Problem 25

Given:

Sets of numbers fraction : {-1.4,−1$\frac{1}{25}$-1.25}

To find- Least to the greatest

Since we convert mixed fractions into numbers

−1$\frac{1}{25}$ = −$\frac{25+1}{25}$

− 1.04

{−1.25<−1.4<−1.04}

{−1.25<−1.4<−1$\frac{1}{25}$}

The sets of value least to greatest is {−1.25<−1.4<−1$\frac{1}{25}$}

Page 277 Exercise 22 Problem 26

Given:

The four mammals’ length in the table is :

Eastern Chipmunk − $\frac{1}{3}$

European Mole − $\frac{5}{12}$

Masked Shrew − $\frac{1}{6}$

Spiny Pocket Mouse − 0.25

To find – Which animal is the smallest mammal

Since we have different animals’ lengths in tables, now we can find which animal length is small in size,

($\frac{1}{3}$ = 0.33),($\frac{5}{12}$ = 0.416), ($\frac{1}{6}$ = 0.166), (0.25)

0.166 < 0.25 < 0.33 <0.416

Since 0.166 ft

The masked shrew is the smallest mammal in this table The length is 0.166ft

Given:

The four mammals’ length in the table is :

Eastern Chipmunk − $\frac{1}{3}$

European Mole − $\frac{5}{12}$

Masked Shrew − $\frac{1}{6}$

Spiny Pocket Mouse − 0.25

To find – Which animal is smaller than the European mole and larger the spiny pocket mouse

Since we have four different animals’ length

European mole length is

$\frac{5}{12}$ = 0.416ft

Spiny pocket mouse length is

0.25ft

And since all length

0.416>0.33>0.25>0.166

In between the length of mammal is

0.33ft

The eastern chipmunk is smaller than the European mole and larger than a spiny pocket mouse.

Given:

The four mammals’ length in the table is :

Eastern Chipmunk − $\frac{1}{3}$

European Mole − $\frac{5}{12}$

Masked Shrew − $\frac{1}{6}$

Spiny Pocket Mouse − 0.25

To find – Order the animals from greatest to least size

Since we have four different animals in different size, we order the animal’s length in greater to the smallest

European mole length is

$\frac{5}{12}$ = 0.416 ft

Spiny pocket mouse length is

0.25ft

And since we order in greatest to smallest is

0.416>0.33>0.25>0.166

Order the animals in greatest to smallest is 0.416>0.33>0.25>0.166.

Page 278 Exercise 23 Problem 27

Given: Four points in the line

To find – Which point located in $\frac{7}{2}$

Since we have four points in that line, The value of $\frac{7}{2}$ = 3.5

This value is located at point C in the line

$\frac{7}{2}$ = 3.5

This value is located at point C in the line.

Page 278 Exercise 24 Problem 28

Given: we have four list of numbers in order

To find – Order the list which is in least to greatest

Since we have four list of number in different values

4$\frac{1}{4}$ = $\frac{16+1}{4}$

$\frac{17}{4}$ = 4.25

$\frac{1}{4}$ = 0.25

Since percentage converted into number is given below

4%= $\frac{4}{100}$ = 0.04

0.04<0.25<0.4<4.25.

The list of numbers is order from least to greatest is 0.04<0.25<0.4<4.25.

Page 278 Exercise 25 Problem 29

Given: Price changes list in every day

To find – which day the price decrease from the greatest amount

Since every day price change

On Thursday is + 0.45 price increased then next day decreased, so on Friday−1.15 the price decreased from the greatest amount

Friday is the price decreased from the greatest amount.

Page 278 Exercise 26 Problem 30

Given:

Two different numbers are −2 and 2.

To find- Make a true sentence

Since the negative value is smallest than the positive value so we put a greater sign in the positive side

−2<2

The true sentence of this value is −2<2.

Page 278 Exercise 28 Problem 31

Given:

Two values in different signs: −20 and 20

To find- Make a true sentence

Since the value of number is same but the sign is different so we put greater sign in the positive side

−20<20

The true sentence is −20<20.

Page 278 Exercise 30 Problem 32

Given:

Two different values in same negative sign: −10 and −1

To find- The number that is greater

Both sides are negative sign so when dealing with negative numbers, the number closer to zero is the bigger number.

−10<−1

The true sentence of the value is −10<−1.

Page 278 Exercise 31 Problem 33

Given:

Two different values in different signs: 50 and −100

To find- The number that is greater

Since the sign are different negative is smaller than positive

50>−100

The true sentence of this value is 50>−100.

Page 278 Exercise 32 Problem 34

Given: Three students read books and spend money for this

To find – The student who has read least amount

Three student spend the value of money given in the fraction

Victoria − $\frac{2}{5}$ = 0.4

Cooper − $\frac{1}{5}$ = 0.2

Diego − $\frac{3}{5}$ = 0.6

Since Cooper has read the least amount $\frac{1}{5}$ = 0.2

Cooper has read the least amount $\frac{1}{5}$ = 0.2.

Page 281 Exercise 1 Problem 35

Given: $\frac{1}{5}$+$\frac{2}{5}$

First, divide the number line into fifths.

Since we don’t know if our answer is negative or positive include fractions to left and to right of zero.

Graph$\frac{1}{5}$ on number line.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 1 graph 1$

Move 2 units to the right to show adding of $\frac{2}{5}$

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 1 graph 2$

So, $\frac{1}{5}$+$\frac{2}{5}$= $\frac{3}{5}$

The final solution of$\frac{1}{5}$+$\frac{2}{5}$= $\frac{3}{5}$.

Page 281 Exercise 2 Problem 36

Given: −$\frac{3}{7}$+(−$\frac{1}{7}$)

To find – Addition of fractions

First, divide the number line into sevenths.

Since we don’t know if our answer is negative or positive include fractions to left and to right of zero.

Graph −$\frac{3}{7}$ on number line.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 2 graph 1$

Move 1 unit to the left to show subtraction of $\frac{1}{7}$

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 2 graph 2$

So, −$\frac{3}{7}$+(−$\frac{1}{7}$) is –$\frac{4}{7}$

The final solution of −$\frac{3}{7}$ + (−$\frac{1}{7}$) is –$\frac{4}{7}$

Page 281 Exercise 3 Problem 37

Given:

Here it is − $\frac{3}{8}$ + $\frac{5}{8}$

To find- Addition of fractions

First, divide the number line into eighths.

Since we don’t know if our answer is negative or positive include fractions to left and to right of zero.

Graph-$\frac{3}{8}$on number line.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 3 graph 1$

Move 5 units to the right to show adding of $\frac{5}{8}$

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 3 graph 2$

So, − $\frac{3}{8}$+$\frac{5}{8}$ is $\frac{2}{8}$

The final solution of –$\frac{3}{8}$+$\frac{5}{8}$ is $\frac{2}{8}$

Page 281 Exercise 4 Problem 38

Given: $\frac{8}{12}$ – $\frac{4}{12}$

To find – Subtraction of fractions

First, divide the number line into twelfths.

Since we don’t know if our answer is negative or positive include fractions to left and to right of zero.

Graph $\frac{8}{12}$ on number line.

Move 4 units to the left to show subtraction of $\frac{4}{12}$

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 4 graph$

So, $\frac{8}{12}$–$\frac{4}{12}$ = $\frac{4}{12}$

The final solution of $\frac{8}{12}$–$\frac{4}{12}$ is $\frac{4}{12}$.

Page 281 Exercise 5 Problem 39

Given: $\frac{4}{9}$+$\frac{5}{9}$

To find – Addition of fractions

First, divide the number line into nineths.

Since we don’t know if our answer is negative or positive include fractions to left and to right of zero.

Graph − $\frac{4}{9}$

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 5 graph 1$

Move 5 units to the right to show addition of $\frac{5}{9}$

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 4.2 Compare and Order Rational Numbers Page 281 Exercise 5 graph 2$

So, −$\frac{4}{9}$ + $\frac{5}{9}$

= $\frac{1}{9}$

The final solution of −$\frac{4}{9}$ + $\frac{5}{9}$ is $\frac{1}{9}$

Page 282 Exercise 10 Problem 40

Using only numerators:

For like fractions, take the numerators separately.

Do the operations such as addition or subtraction for numerators.

Using number line:

Plot the fraction intervals on number line.

For addition operation, move to right, and for subtraction operation, move to left.

Rules for addition and subtraction: Add the numerators and place the sum over the common denominator.

Fraction subtraction: Subtract the numerators and place the difference over the common denominator.

The rules for addition and subtraction of like fractions are add the numerators and place the sum over the common denominator and Subtract the numerators and place the difference over the common denominator.

Glencoe Math Course 2 Volume 1 Common Core Student Edition Chapter 1 Ratios and Proportional Reasoning Exercise 1.2

June 27, 2024March 8, 2023 by Marksparks

Glencoe Math Course 2 Volume 1 Common Core Chapter 1 Ratios and Proportional Reasoning

Page 17 Exercise 1 Problem 1

Let the two ratios be a:b and c:d

If both the ratios are equal, a:b = c:d

Then the given ratio is said to be in proportion.

And also, if the sets increase or decrease in the very same ratio, then the ratio is said to be in proportion.

In terms of objects, if the two objects are said to be in the very same shape but in different sizes.

For example: If the two objects are spheres, but one is smaller while the other one is larger.

Here, even though the sizes differ, the corresponding angles will be the same.

This makes their ratios be in proportion.

Hence, the two objects are proportional.

Two objects are said to be in proportion if they have the same shape, and same angles but in different sizes. This makes their ratios be in proportion.

Page 17 Exercise 2 Problem 2

Given: Dana is skating laps to train for a speed skating competition.

She can skate 1 lap in 40 seconds.

Suppose Dana skates for 20 seconds.

We need to determine how many laps she will skate.

Given:

The number of laps she can skate is 1 lap.

The number of time taken to do one lap is 40 seconds.

The ratio is = $\frac{40}{1}$

If she skates only for 20 Seconds, then the number of laps will be

$\frac{40}{x}$ = $\frac{40}{1}$

x = $\frac{20}{40}$

x = $\frac{2}{4}$

x = $\frac{1}{2}$

x = 0.5

The number of laps she will skate is 0.5 laps.

Page 17 Exercise 3 Problem 3

Given: Dana is skating laps to train for a speed skating competition.

She can skate 1 lap for 40 seconds.

In exercise 2, Dana skates 0.5 laps for 20 seconds.

We need to write the ratio of Dana’s time from Exercise 2 to her number of laps.

Given: From exercise 2

The number of laps she can skate is 0.5 lap.

The number of time taken to do one lap is 20 seconds.

The ratio from exercise 2 is

$\frac{\text { Dana’s time }}{\text { Number of laps }}=\frac{20}{0.5}$

We need to substitute this ratio in her number of laps. The ratio is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.2 Complex Fractions And Unit Rates Page 17 Exercise 3$

The ratio is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.2 Complex Fractions And Unit Rates Page 17 Exercise 3.$

Page 17 Exercise 4 Problem 4

Given: Dana is skating laps to train for a speed skating competition.

She can skate 1 lap in 40 seconds.

We need to detect how we simplify the ratio we wrote in Exercise 3.

The ratio in exercise is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.2 Complex Fractions And Unit Rates Page 17 Exercise 4$

Simplifying the ratio, we get

$\frac{\text { Dana’s time }}{\text { Number of Laps }}=\frac{40}{\frac{20}{0.5}}$

= 40 × $\frac{0.5}{20}$

= 40 × $\frac{0.5}{2}$

= 2 × 0.5

= 1

The simplification of the ratio we wrote in Exercise 3 results in a 1:1

Page 20 Exercise 1 Problem 5

Given: The complex fraction is$\frac{18}{\frac{3}{4}}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{18}{\frac{3}{4}}$ =$\frac{18}{1}$ ÷ $\frac{3}{4}$

Multiply it by the reciprocal of $\frac{3}{4}$ , we get

$\frac{18}{\frac{3}{4}}$ =$\frac{18}{1}$ ÷ $\frac{3}{4}$

$\frac{18}{\frac{3}{4}}$ =$\frac{18}{1}$ × $\frac{4}{3}$

Simplifying it further, we get

$\frac{18}{\frac{3}{4}}$ =$\frac{18}{1}$ × $\frac{4}{3}$

= $\frac{6}{1}$×$\frac{4}{1}$

= 24

The value of $\frac{18}{\frac{3}{4}}$ is equal to 24

Page 20 Exercise 2 Problem 6

Given: The complex fraction is $\frac{\frac{3}{6}}{4}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{\frac{3}{6}}{4}$ = $\frac{3}{6}$÷ $\frac{4}{1}$

Multiply it by the reciprocal of $\frac{4}{1}$, we get

$\frac{\frac{3}{6}}{4}$ = $\frac{3}{6}$÷$\frac{4}{1}$

$\frac{\frac{3}{6}}{4}$ = $\frac{3}{6}$×$\frac{1}{4}$

Simplifying it further, we get

$\frac{\frac{3}{6}}{4}$= $\frac{3}{6}$×$\frac{1}{4 }$

= $\frac{1}{2}$×$\frac{1}{4}$

= $\frac{1}{8}$

The value of $\frac{\frac{3}{6}}{4}$ is equal to $\frac{1}{8}$

Page 20 Exercise 3 Problem 7

Given: The complex fraction is $\frac{\frac{1}{3}}{\frac{1}{4}}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{\frac{1}{3}}{\frac{1}{4}}$ = $\frac{1}{3}$ ÷$\frac{1}{4}$

Multiply it by the reciprocal of, we get

$\frac{\frac{1}{3}}{\frac{1}{4}}$ = $\frac{1}{3}$ ÷ $\frac{1}{4}$

$\frac{\frac{1}{3}}{\frac{1}{4}}$ = $\frac{1}{3}$ × $\frac{4}{1}$

Simplifying it further, we get

$\frac{\frac{1}{3}}{\frac{1}{4}}$ = $\frac{1}{3}$ × $\frac{4}{1}$

= $\frac{4}{3}$

The value of $\frac{\frac{1}{3}}{\frac{1}{4}}$ is equal to $\frac{4}{3}$

Page 20 Exercise 4 Problem 8

Given: That, Pep Club members are making spirit buttons.

They make 490 spirit buttons in 3$\frac{1}{2}$hours.

We need to determine the number of buttons the Pep Club makes per hour.

Given:

Number of spirit buttons = 490

Time taken = 3$\frac{1}{2}$= $\frac{7}{2}$hours

The unit rate is

Unite rate = $\frac{490}{\frac{7}{2}}$

= 490×$\frac{2}{7}$

= 70 × 2

= 140 Sprit buttons per hour

The number of buttons the Pep Club makes per hour is 140 spirit buttons per hour.

Page 20 Exercise 5 Problem 9

Given: That, the country sales tax is 6$\frac{2}{3}$%

We need to write the given percent as a fraction and to simplify it.

Given: Percent is 6$\frac{2}{3}$%

Converting the given mixed fraction into an improper fraction, we get

6$\frac{2}{3}$ = $\frac{20}{3}$%

Thus, the percent becomes

$\frac{20}{3}$ percentage = $\frac{20}{3}$ ÷ 100

Multiply it by the reciprocal of $\frac{100}{1}$ we get

$\frac{20}{3}$ percentage = $\frac{20}{3}$÷ 100

$\frac{20}{3}$ percentage = $\frac{20}{3}$ × $\frac{1}{100}$

= $\frac{1}{3}$ × $\frac{1}{5}$

= $\frac{1}{15}$

The given percent 6$\frac{2}{3}$% $\frac{1}{15}$

Page 20 Exercise 6 Problem 10

A complex fraction is nothing but a fraction that has fractions in its denominator or in the numerator or in both.

For example

$\frac{\frac{5}{2}}{10}, \frac{11}{\frac{6}{8}}, \frac{\frac{12}{15}}{\frac{3}{4}}$

These are all complex fractions.

Solving a complex fraction:

Let us consider a complex fraction

$\frac{\frac{3}{4}}{\frac{10}{12}}$

Simplifying it, we get

$\frac{\frac{3}{4}}{\frac{10}{12}}$= $\frac{3}{4}$× $\frac{12}{10}$

= $\frac{3}{1}$×$\frac{3}{10}$

= $\frac{9}{10}$

A complex fraction is a fraction that has more than one fraction. That is, fractions will be in their denominator or in the numerator, or in both.

Page 21 Exercise 1 problem 11

Given: The complex fraction is $\frac{1}{\frac{2}{3}}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{1}{\frac{2}{3}}$= $\frac{1}{1}$ ÷ $\frac{2}{3}$

Multiply it by the reciprocal of $\frac{2}{3}$ , we get

$\frac{1}{\frac{2}{3}}$= $\frac{1}{1}$ ÷ $\frac{2}{3}$

$\frac{1}{\frac{2}{3}}$= $\frac{1}{1}$ × $\frac{3}{2}$

Simplifying it further, we get

$\frac{1}{\frac{2}{3}}$= $\frac{1}{1}$ × $\frac{3}{2}$

= $\frac{3}{2}$

The value of $\frac{1}{\frac{2}{3}}$ is equal to = $\frac{3}{2}$

Page 21 Exercise 2 Problem 12

Given: The complex fraction is $\frac{2}{\frac{3}{11}}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{2}{\frac{3}{11}}$= $\frac{2}{1}$ ÷$\frac{3}{11}$

Multiply it by the reciprocal of $\frac{3}{11}$ , we get

$\frac{2}{\frac{3}{11}}$= $\frac{2}{1}$ ÷ $\frac{3}{11}$

$\frac{2}{\frac{3}{11}}$= $\frac{2}{1}$ ×$\frac{11}{3}$

Simplifying it further, we get

$\frac{2}{\frac{3}{11}}$ = $\frac{2}{1}$ ×$\frac{11}{3}$

= $\frac{22}{3}$

The value of $\frac{2}{\frac{3}{11}}$ is equal to $\frac{22}{3}$

Page 21 Exercise 3 Problem 13

Given: The complex fraction is $\frac{\frac{8}{9}}{6}$

We need to simplify the given complex fraction

Rewrite the given fraction as a division.

The expression becomes

$\frac{\frac{8}{9}}{6}$ = $\frac{8}{9}$÷$\frac{6}{1}$

Multiply it by the reciprocal of $\frac{1}{6}$ we get,

$\frac{\frac{8}{9}}{6}$ = $\frac{8}{9}$÷$\frac{6}{1}$

$\frac{\frac{8}{9}}{6}$ = $\frac{8}{9}$×$\frac{1}{6}$

Simplifying it further, we get,

$\frac{\frac{8}{9}}{6}$= $\frac{8}{9}$×$\frac{1}{6}$

= $\frac{4}{9}$×$\frac{1}{3}$

= $\frac{4}{27}$

The value of $\frac{\frac{8}{9}}{6}$ is equal to $\frac{4}{27}$

Page 21 Exercise 4 Problem 14

Given: The complex fraction is $\frac{\frac{2}{5}}{9}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{\frac{2}{5}}{9}$= $\frac{2}{5}$÷$\frac{9}{1}$

Multiply it by the reciprocal of 9 , and we get

$\frac{\frac{2}{5}}{9}$= $\frac{2}{5}$÷$\frac{9}{1}$

$\frac{\frac{2}{5}}{9}$= $\frac{2}{5}$× $\frac{1}{9}$

Simplifying it further, we get

$\frac{\frac{2}{5}}{9}$= $\frac{2}{5}$× $\frac{1}{9}$

= $\frac{2}{45}$

The value of $\frac{\frac{2}{5}}{9}$ is equal to $\frac{2}{45}$

Page 21 Exercise 5 Problem 15

Given: The complex fraction is $\frac{\frac{4}{5}}{10}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{\frac{4}{5}}{10}$ = $\frac{4}{5}$÷$\frac{10}{1}$

Multiply it by the reciprocal of $\frac{10}{1}$ , we get,

$\frac{\frac{4}{5}}{10}$ = $\frac{4}{5}$÷$\frac{10}{1}$

$\frac{\frac{4}{5}}{10}$ = $\frac{4}{5}$×$\frac{1}{10}$

Simplifying it further, $\frac{1}{10}$ we get

$\frac{\frac{4}{5}}{10}$ = $\frac{4}{5}$×$\frac{1}{10}$

= $\frac{2}{5}$×$\frac{1}{5}$

= $\frac{2}{25}$

The value of $\frac{\frac{4}{5}}{10}$ is equal to$\frac{2}{25}$

Page 21 Exercise 6 Problem 16

Given: The complex fraction is $\frac{\frac{1}{4}}{\frac{7}{10}}$

We need to simplify the given complex fraction.

Rewrite the given fraction as a division.

The expression becomes

$\frac{\frac{1}{4}}{\frac{7}{10}}$ = $\frac{1}{4}$ ÷ $\frac{7}{10}$

Multiply it by the reciprocal of $\frac{7}{10}$ , we get

$\frac{\frac{1}{4}}{\frac{7}{10}}$ = $\frac{1}{4}$ ÷ $\frac{7}{10}$

$\frac{\frac{1}{4}}{\frac{7}{10}}$ = $\frac{1}{4}$ × $\frac{10}{7}$

Simplifying it further, we get

$\frac{\frac{1}{4}}{\frac{7}{10}}$ = $\frac{1}{4}$ × $\frac{10}{7}$

= $\frac{1}{2}$× $\frac{5}{7}$

= $\frac{5}{14}$

The value of $\frac{\frac{1}{4}}{\frac{7}{10}}$ is equal to $\frac{5}{14}$

Page 21 Exercise 8 Problem 17

Given: Doug entered a canoe race. He rowed $3\frac{1}{2}$ miles in $\frac{1}{2}$ hour.

We need to determine his average speed in milés per hour.

Given:

Number of miles rowed = $3\frac{1}{2}$miles

Time taken = $\frac{1}{2}$ hour

The unit rate is

Unite rate $ = \frac{\text { Number of miles }}{\text { Time taken }}$

= $\frac{3 \frac{1}{2}}{\frac{1}{2}}$

= $\frac{\frac{7}{2}}{\frac{1}{2}}$

= $\frac{7}{2} \times \frac{2}{1}$

= 7 miles per hour

Hence, The average speed of Doug in miles per hour is 7.

Page 21 Exercise 9 Problem 18

Given: Monica reads $7\frac{1}{2}$ pages of a mystery book in 9minutes.

We need to determine her average reading rate in pages per minute.

Given:

Number of pages = $7\frac{1}{2}$

Number of minutes taken = 9

The unit rate is

Unite rate = $\frac{\text { Number of pages }}{\text { Number of minutes }}$

= $\frac{7 \frac{1}{2}}{9}$

= $\frac{\frac{15}{2}}{9}$

= $\frac{15}{2} \times \frac{1}{9}$

= $\frac{5}{2} \times \frac{1}{3}$

= $\frac{5}{6}$

Her average reading rate in pages per minute is $\frac{5}{6}$.

Page 21 Exercise 10 Problem 19

Given: The percent is $56 \frac{1}{4} \%$

We need to write the given percent as a fraction and simplify it.

Given percent is $56 \frac{1}{4} \%$

Converting the given mixed fraction into an improper fraction, we get

$56 \frac{1}{4}$=$\frac{225}{4}$%

Thus, the percent becomes

$\frac{225}{4}$percent = $\frac{225}{4}$ ÷ 100

Multiply it by the reciprocal of $\frac{100}{1}$, we get

$\frac{225}{4}$ percent = $\frac{225}{4}$ × $\frac{1}{100}$

$=\frac{9}{4} \times \frac{1}{4}$

= $\frac{9}{16}$

The given percent $56 \frac{1}{4} \%$as a fraction in the simplest form is $\frac{9}{16}$

Page 21 Exercise 11 Problem 20

Given: The percent is $15 \frac{3}{5} \%$

We need to write the given percent as a fraction and simplify it.

Given percent is $15 \frac{3}{5} \%$

Converting the given mixed fraction into an improper fraction, we get

$15 \frac{3}{5}$ = $\frac{78}{5}%$

Thus, the percent becomes

$\frac{78}{5}$ percent = $\frac{78}{5}$ ÷ $\frac{100}{1}$

Multiply it by the reciprocal of $\frac{100}{1}$ we get

$\frac{78}{5}$ percent = $\frac{78}{5}$ ÷ $\frac{100}{1}$

$\frac{78}{5}$ percent = $\frac{78}{5}$× $\frac{1}{100}$

= $\frac{78}{500}$

= $\frac{39}{250}$

The given percent $15 \frac{3}{5} \%$as a fraction in the simplest form is $\frac{39}{250}$

Glencoe Math Course 2 Volume 1 Common Core Student Edition Chapter 1 Ratios and Proportional Reasoning Exercise 1.5

June 27, 2024March 8, 2023 by Marksparks

Glencoe Math Course 2 Volume 1 Common Core Chapter 1 Ratios and Proportional Reasoning

Page 45 Exercise 1 Problem 1

We need to label the given coordinate plane with the terms ordered pair, x-coordinate, and y-coordinate.

We need to graph the points(2,3) and (−3,−2) and to connect the three points on the coordinate plane.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 45 Exercise 1 , graph 1$

The point which is already there on the graph is (1,2)

Labeling the mentioned blanks, we get

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 45 Exercise 1 , graph 2$

Graphing the points (2,3),(−3,−2) on the graph and connecting the three points on the coordinate plane, we get,

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 45 Exercise 1 , graph 3$

The obtained graph is a straight line.

Labeling the coordinate plane below

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 45 Exercise 1 , graph 4$

Graphing the points and connecting them on the coordinate plane

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 45 Exercise 1 , graph 5$

Page 48 Exercise 1 problem 2

Given the cost of 3-D movie tickets is $12 for 1 ticket, $24 for 2 tickets, and $36 for 3 tickets.

We need to determine whether the cost is proportional to the number of tickets by graphing on the coordinate plane.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 48 Exercise 1 graph 1$

Make a table to find the cost of tickets for 1,2 and 3 members.

Now, graph the ordered pairs (tickets, cost) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 48 Exercise 1 , graph 2$

The line passes through the origin and it is a straight line.

Therefore, the cost is proportional to the number of tickets.

The cost is proportional to the number of tickets.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 48 Exercise 1 , graph 3$

Page 48 Exercise 2 Problem 3

Given that, the number of books the two stores sell after 1,2, and 3 days is shown.

We need to find which book sale represents a proportional relationship between time and books.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 48 Exercise 2 , graph 1$

The given graph is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 48 Exercise 2 , graph 2$

The graph for Discount books is not a straight line.

Hence, the relationship is not proportional.

The graph for The Book Palace is a straight line that passes through the origin.

Hence, it represents a proportional relationship between time and books. The Book Palace represents a proportional relationship between time and books.

Page 48 Exercise 3 Problem 4

At first, we need to graph the ordered pairs (x,y) on the coordinate plane.

The x – represents the x−coordinate point.

The y- represents the y−coordinate point.

After graphing the ordered pairs, determine whether the graph obtained is a straight line or not.

If it was not a straight line, then the relationship is not proportional to each other.

If it is a straight line, check whether the straight line passes through the origin or not.

If the straight line passes through the origin, then the relationship is proportional to each other.

In this way, we can find the proportional relationship between the quantities.

If the graph represents the straight line that passes through the origin, then the quantities are said to be proportional. In this way, the graphing relationships help you determine whether the relationship is proportional or not.

Page 49 Exercise 1 Problem 5

Given that, we need to determine whether the relationship between the two quantities shown in the table is proportional by graphing on the coordinate plane.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 1 , graph 1$

The given table is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 1 table$

Now, graph the ordered pairs (weeks, balance) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 1 , graph 2$

The line doesn’t pass through the origin and it is a straight line.

Thus, the number of weeks is not proportional to the account balance.

The number of weeks is not proportional to the account balance.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 1 , graph 3$

Page 49 Exercise 2 Problem 6

Given that, we need to determine whether the relationship between the two quantities shown in the table is proportional by graphing on the coordinate plane.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 2 , graph 1$

The given table is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 48 Exercise 2 , table$

Now, graph the ordered pairs (servings, calories) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 2 , graph 2$

The line passes through the origin and it is a straight line.

Thus, the number of servings is proportional to the calories.

The number of servings is proportional to the calories.

Page 49 Exercise 3 Problem 7

Given that the height of two plants is recorded after 1,2, and 3 weeks as shown in the graph at the right.

We need to determine which plants’ growth represents a proportional relationship between time and height.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 3, graph 1$

The given graph is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 49 Exercise 3, graph 2$

The graph for Plant A is not a straight line.

Hence, the relationship is not proportional.

The graph for Plant B is a straight line that passes through the origin.

Hence, it represents a proportional relationship between time and height.

Plant B’s growth represents a proportional relationship between time and height.

Page 50 Exercise 4 Problem 8

Given that, the perimeter of a square is 4 times as great as the length of any of its sides.

We need to find if the perimeter of a square is proportional to its side length.

Given that, Perimeter P = 4a

Let a = Length of the sides.

Make a table to find the perimeter for the side’s length1,2,3, and 4

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 4 , table$

Now, graph the ordered pairs (side length, perimeter) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 4, graph$

The line passes through the origin and it is a straight line.

Therefore, the perimeter of a square is proportional to its side length.

The perimeter of a square is proportional to its side length.

Page 50 Exercise 5 Problem 9

Given that, a health club charges $35 a month for membership fees.

We need to determine whether the cost of membership is proportional to the number of months.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 5 , table$

Make a table to find the membership fees for 1,2,3 and 4 months.

Now, graph the ordered pairs (months, fees) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 5 , graph$

The line passes through the origin and it is a straight line.

Therefore, the cost of membership is proportional to the number of months.

The cost of membership is proportional to the number of months.

Page 50 Exercise 6 Problem 10

We need to describe some data that when graphed would represent a proportional relationship.

Let us assume that the perimeter of a square is 4 times as great as the length of any of its sides.

We need to find if the perimeter of a square is proportional to its side length.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 6, table$

Now, graph the ordered pairs (side length, perimeter) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 6 , graph$

The line passes through the origin and it is a straight line.

Therefore, the perimeter of a square is proportional to its side length.

The perimeter of a square is proportional to its side length.

Page 50 Exercise 7 Problem 11

Given that the greenhouse temperatures at certain times are shown in the table.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 7 , table 1$

The greenhouse maintains temperatures between 65 degrees Fahrenheit and 85 degrees Fahrenheit and the temperature increases at a constant rate.

We need to create a graph of the time and temperatures at each hour from 1:00 P.M. to 8:00 P.M. and check whether the relationship is proportional.

The given table is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 7 , table 2$

Now, graph the ordered pairs (time, temperature) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 7 , graph$

The line doesn’t pass through the origin and it is a straight line.

Therefore, the relationship is not proportional.

The time is not proportional to the temperature in Fahrenheit.

Page 50 Exercise 8 Problem 12

The graph is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 8, graph 1$

Given graph represents the Calories burned for exercising for various numbers of minutes are shown in the graph.

We need to determine which of the below statement about the graph is not true.

The statements are

(A) The number of Calories burned is proportional to the number of minutes spent exercising.

(B) The number of Calories burned is not proportional to the number of minutes spent exercising.

(D) The line is straight.

The given graph is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 50 Exercise 8, graph 2$

The given graph is a straight line.

It also passes through the origin.

Thus, the time in minutes is proportional to the number of calories represented in the graph.

Thus, the relationship between the two quantities is proportional.

The incorrect statement among them is “The number of Calories burned is not proportional to the number of minutes spent exercising.”

The Calories burned for exercising for various numbers of minutes are shown in the graph. Statement (B) about the graph is not true. (B) The number of Calories burned is not proportional to the number of minutes spent exercising.

Page 51 Exercise 12 Problem 13

Given that, Frank and Allie purchased cell phone plans through different providers.

Their costs for several minutes are shown.

We need to graph each plan to determine whose plan is proportional to the number of minutes the phone is used

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 51 Exercise 12, table 1$

The given table is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 51 Exercise 12, table 2$

Now, graph the ordered pairs (time, cost) on the coordinate plane.

Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 51 Exercise 12, graph$

For frank, the line passes through the origin and it is a straight line.

Therefore, the relationship is proportional.

For Allie, the line doesn’t pass through the origin and it is a straight line.

Therefore, the relationship is not proportional.

Frank’s plan is proportional to the number of minutes the phone is used.

Page 52 Exercise 13 Problem 14

We need to determine whether the relationship between the number of heartbeats and the time shown in the graph is proportional.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 52 Exercise 13, graph 1$

The given graph is

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 52 Exercise 13, graph 2$

The graph given is a straight line that passes through the origin.

Hence, it represents a proportional relationship between the number of heartbeats and the time.

The relationship between the number of heartbeats and the time shown in the graph is proportional.

Page 52 Exercise 14 Problem 15

We need to the graph below and to determine which of the following ordered pairs represent the unit rate.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 52 Exercise 14, graph$

The given ordered pairs are

(A) (0,0)

The unit rate is generally defined for only one quantity.

Here, the quantity is zero.

Thus, it cannot be the unit rate.

(B) (1,2)

Here, it represents for each second, the number of heartbeats is two.

Thus, this ordered pair represents the unit rate.

(C) (2,4)

Here, it represents for two seconds, and the number of heartbeats is four.

Thus, this ordered pair doesn’t represent the unit rate.

(D) (3,6)

Here, it represents for three seconds, and the number of heartbeats is six.

Thus, this ordered pair doesn’t represent the unit rate.

The ordered pair (B) (1,2) represents the unit rate.

Page 52 Exercise 17 Problem 16

Given that, a car dealership has 55 cars and 11 Vans.

We need to determine the ratio of cars to vans.

Number of cars = 55

Number of vans = 11

The ratio of cars to vans is

$\frac{\text{Cars}}{\text{Vans}}$ = $\frac{55}{11}$

= $\frac{5}{1}$

The ratio of cars to vans is = 5:1

The ratio of cars to vans is 5:1

Page 52 Exercise 18 Problem 17

Given that, a drawer has 4 red shirts and 8 green shirts.

We need to determine the ratio of red shirts to the total number of shirts.

Number of red shirts = 4

Number of green shirts = 8

The ratio of red shirts to the total number of shirts is

$\frac{\text{Red shirts}}{\text{Total shirts}}$ = $\frac{4}{4+8}$

= $\frac{4}{12}$

= $\frac{1}{3}$

= 1:3

The ratio of red shirts to the total number of shirts is 1:3

Page 52 Exercise 19 Problem 18

Given that, a store sells 13 coffees and 65 hot chocolates.

We need to determine the ratio of coffee to hot chocolates.

Number of coffees = 13

Number of hot chocolates = 65

The ratio of coffee to hot chocolates is

$\frac{\text{Cofees}}{\text{Hot chocolates}}$ = $\frac{13}{65}$

= $\frac{1}{5}$

= 1:5

The ratio of coffee to hot chocolates is 1:5

Page 54 Exercise 1 Problem 19

We need to determine whether Albert’s graph represents a proportional relationship or Bianca’s.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 1, table 1$

Make a table with the given information, we get

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 1, table 2$

Now, graph the ordered pairs on the coordinate plane. Then, connect the points together

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 1, graph$

The graph for Bianca’s comments is a straight line but doesn’t pass through the origin.

Hence, the relationship is not proportional.

The graph for Albert’s comments is a straight line that passes through the origin.

Hence, it represents a proportional relationship between comment number and replies.

Albert’s graph represents a proportional relationship while Bianca’s doesn’t.

Page 54 Exercise 2 Problem 20

The proportionality of two different quantities can easily be determined by using constant ratios.

The constant ratios indicate that the value of the ratios is all the same.

If the ratios are constant, then the ratios are said to be proportional to each other.

When two ratios are equal, it is said to be in proportion.

Let the two ratios be a:b and c:d

If both the ratios are equal, a:b = c:d

Then the given ratio is said to be in proportion.

Two quantities are said to be in proportion if they have the same ratios. This makes their ratios be in proportion.

Page 54 Exercise 3 Problem 21

We need to describe the type of relationship shown by each set of ordered pairs.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 3 , table 1$

Mark the order part on the given graph

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 3 , graph$

Here, we can see that the ordered pair 3 is a straight line and it passes through the origin.

Thus, it is linear and proportional.

The ordered pair 4 is not a straight line.

Thus, it is neither linear nor proportional.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 3 , table 2$

Page 54 Exercise 4 Problem 22

We need to describe the type of relationship shown by each set of ordered pairs.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 4, table 1$

Mark the ordered pairs given on the graph

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 4, graph$

Here, we can see that the ordered pair 3 is a straight line and it passes through the origin.

Thus, it is linear and proportional.

The ordered pair 4 is not a straight line.

Thus, it is neither linear nor proportional.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 4, table 2$

Page 54 Exercise 5 Problem 23

We need to describe a real-world situation that represents a proportional relationship.

Then explain how we could change the situation so that it represents a nonproportional relationship.

Let the real-world situation be, James earns $5 an hour babysitting.

We need to determine whether the amount of money James earns babysitting is proportional to the number of hours he babysits by graphing on the coordinate plane.

Make a table to find the amount of money he earned for 1,2,3,4 and 5 hours

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 5, table$

Now, graph the ordered pairs (time, earnings) on the coordinate plane. Then, connect the points together.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.5 Graph Proportional relationships Page 54 Exercise 5, graph$

The line passes through the origin and it is a straight line.

Therefore, the amount of money James earns while babysitting is proportional to the number of hours he babysits.

We can change the situation so that it represents a nonproportional relationship by changing the amount of money he earns per hour.

For example, if he gets $5 for the first hour, and $7 for the next consecutive hours.

Then the graph will not be linear. Thus, it exhibits a nonproportional relationship.

The amount of money James earns babysitting is proportional to the number of hours he babysits. We can change the situation so that it represents a nonproportional relationship by changing the amount of money he earns every hour.

Glencoe Math Course 2 Volume 1 Common Core Student Edition Chapter 1 Ratios and Proportional Reasoning Practice Exercise 1.2

June 27, 2024March 8, 2023 by Marksparks

Glencoe Math Course 2 Volume 1 Common Core Chapter 1 Ratios and Proportional Reasoning

Page 21 Exercise 12 Problem 1

Given that, the percent is 13$\frac{1}{3}$ %

We need to write the given percent as a fraction and simplify it.

Given percent is 13$\frac{1}{3}$ %

Converting the given mixed fraction into an improper fraction, we get

13$\frac{1}{3}$ = $\frac{40}{3}$ %

Thus, the percent becomes

$\frac{40}{3}$ percent = $\frac{40}{3}$÷ $\frac{100}{1}$

Multiply it by the reciprocal of $\frac{100}{1}$ , we get

$\frac{40}{3}$ percent = $\frac{40}{3}$÷ $\frac{100}{1}$

= $\frac{40}{3}$× $\frac{1}{100}$

= $\frac{4}{3}$× $\frac{1}{10}$

= $\frac{2}{3}$× $\frac{1}{5}$

= $\frac{2}{15}$

The given percent 13$\frac{1}{3}$ % as a fraction in the simplest form is $\frac{2}{15}$

Page 22 Exercise 15 Problem 2

A complex fraction is nothing but a fraction that has fractions in its denominator or in the numerator or in both.

For example:

$\frac{\frac{5}{10}}{2}, \frac{11}{\frac{6}{5}}, \frac{\frac{13}{12}}{\frac{9}{8}}$. These are all complex fractions.

Solving a complex fraction:

Let us consider a complex fraction that involves ratios

$\frac{\frac{5}{2}}{10}$

Simplifying it, we get

$\frac{\frac{5}{2}}{10}$

= $\frac{5}{2}$ × $\frac{1}{10}$

= $\frac{1}{2}$ × $\frac{1}{2}$

= $\frac{1}{4}$

A complex fraction is a fraction that has more than one fraction.

That is, fractions will be in their denominator or in the numerator, or in both.

Page 22 Exercise 16 Problem 3

We need to write three different complex fractions that simplify to $\frac{1}{4}$

So, we will use the definition

The three different complex fractions and their simplification is given below

1) $\frac{\frac{1}{2}}{2}$

Simplifying it, we get

$\frac{\frac{1}{2}}{2}$= $\frac{1}{2}$×$\frac{1}{2}$

= $\frac{1}{4}$

2)$\frac{\frac{5}{4}}{5}$

Simplifying it, we get

$\frac{5}{4}$× $\frac{1}{5}$

= $\frac{1}{4}$

3)$\frac{\frac{1}{3}}{\frac{4}{3}}$

Simplifying it, we get

$\frac{\frac{1}{3}}{\frac{4}{3}}$ = $\frac{1}{3}$×$\frac{3}{4}$

= $\frac{1}{4}$

The three different complex fractions that simplify to $\frac{1}{4}$ are $\frac{\frac{1}{2}}{2}$, $\frac{\frac{5}{4}}{5}$, $\frac{\frac{1}{3}}{\frac{4}{3}}$

Page 22 Exercise 17 Problem 4

We need to determine the value of ,$\frac{15}{124} \cdot \frac{230}{30} \div \frac{230}{124} $

Simplifying it, we get

$\frac{15}{124} \cdot \frac{230}{30} \div \frac{230}{124} $ = $\frac{15}{124} \times \frac{230}{30} \times \frac{124}{230}$

= $\frac{15}{1}$ × $\frac{1}{30}$ × $\frac{1}{1}$

= $\frac{15}{30}$

= $\frac{1}{2}$

The value of $\frac{15}{124} \cdot \frac{230}{30} \div \frac{230}{124} $ = $\frac{1}{2}$

Page 22 Exercise 18 Problem 5

We need to determine which statement explains how to use the model to simplify the complex fraction.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.2 Complex Fractions And Unit Rates Page 22 Exercise 18$

Count the twelfths that fit within ${2}{3}$ of the above equation

There are 12 number of $\frac{1}{12}$‘s are there.

Thus , $\frac{2}{3}$ rd of the are

$\frac{2}{3}$ ×12 = 2 × 4 = 8

The result of the given complex fraction is

$\frac{\frac{2}{3}}{\frac{1}{12}}$= $\frac{2}{3}$×$\frac{12}{1}$

= $\frac{2}{1}$×$\frac{4}{1}$

= 8

Thus, both are the same. Hence, this statement is correct.

Count the twelfths that fit within $\frac{2}{3}$. This statement explains how to use the model to simplify the complex fraction.

Page 23 Exercise 20 Problem 6

The objective is to Simplify the value of $\frac{12}{\frac{3}{5}}$

We will use the definition.

Given is $\frac{12}{\frac{3}{5}}$

Simplifying it, we get

$\frac{12}{\frac{3}{5}}$ = 12 ÷ $\frac{3}{5}$

= 12 × $\frac{5}{3}$

= 4 × 5

= 20

The value of $\frac{12}{\frac{3}{5}}$ = 20

Page 23 Exercise 21 Problem 7

We are given $ \frac{\frac{9}{10}}{9}$

To Find: The objective is to simplify the given fraction $ \frac{\frac{9}{10}}{9}$

Given is $ \frac{\frac{9}{10}}{9}$

Simplifying it, we get

$ \frac{\frac{9}{10}}{9}$ = $\frac{9}{10}$÷ 9

= $\frac{9}{10}$ × $\frac{1}{9}$

= $\frac{1}{10}$

The value of $ \frac{\frac{9}{10}}{9}$= $\frac{1}{10}$

Page 23 Exercise 22 Problem 8

Simplify the value of $\frac{\frac{1}{2}}{\frac{1}{4}}$

Given is $\frac{\frac{1}{2}}{\frac{1}{4}}$

Simplifying it, we get

$\frac{\frac{1}{2}}{\frac{1}{4}}$= $\frac{1}{2}$ × $\frac{4}{1}$

= $\frac{4}{2}$

= 2

The value of $\frac{\frac{1}{2}}{\frac{1}{4}}$= 2

Page 23 Exercise 23 Problem 9

The objective is to Simplify the value of $\frac{\frac{1}{12}}{\frac{5}{6}}$

We will use the Definition.

Given $\frac{\frac{1}{12}}{\frac{5}{6}}$

Simplifying it, we get

$\frac{\frac{1}{12}}{\frac{5}{6}}$= $\frac{1}{12}$ × $\frac{6}{5}$

= $\frac{1}{2}$ × $\frac{1}{5}$

= $\frac{1}{10}$

Hence, The value of $\frac{\frac{1}{12}}{\frac{5}{6}}$ = $\frac{1}{10}$

Page 23 Exercise 24 Problem 10

Simplify the value of $\frac{\frac{5}{6}}{\frac{5}{9}}$

Given is $\frac{\frac{5}{6}}{\frac{5}{9}}$

Simplifying it, we get

$\frac{\frac{5}{6}}{\frac{5}{9}}$ = $\frac{5}{6}$× $\frac{9}{5}$

= $\frac{9}{6}$

= $\frac{3}{2}$

The value of $\frac{\frac{5}{6}}{\frac{5}{9}}$ = $\frac{3}{2}$

Page 23 Exercise 25 Problem 11

Given that, Mrs. Frasier is making costumes for the school play. Each costume requires 0.75 yards of fabric. She bought 6 yards of fabric.

We need to determine how many costumes Mrs. Frasier can make.

Number of yards needed to make one costume = 0.75

Total yards of fabric bought = 6

The ratio is, $\frac{6}{0.75}$

= 8

She can make eight costumes out of it.

Hence, Mrs. Frasier can make 8 costumes.

Page 23 Exercise 26 Problem 12

Given that, A lawn company advertises that they can spread 7,500 square feet of grass seed in 2$\frac{1}{2}$ hours.

We need to determine the number of square feet of grass seed that can be spread per hour.

Given that
Square feet of grass that they can spread = 7500

Time taken = 2$\frac{1}{2}$

The unit rate is

Unite rate $ = \frac{7500}{2 \frac{1}{2}} $

= $\frac{7500}{\frac{5}{2}}$

= 7500 × $\frac{2}{5}$

= 1500 × 2

= 3000

Hence, The number of square feet of grass seed that can be spread per hour is 3000 square feet.

Page 23 Exercise 28 Problem 13

Given that, the percent is 7 $\frac{3}{4}$%

We need to write the given percent as a fraction and simplify it.

Given percent is 7 $\frac{3}{4}$%

Converting the given mixed fraction into an improper fraction, we get

7 $\frac{3}{4}$ = $\frac{31}{4}$%

Thus, the percent becomes

$\frac{31}{4}$ percent = $\frac{31}{4}$ ÷ $\frac{100}{1}$

Multiply it by the reciprocal of $\frac{100}{1}$ , we get

$\frac{31}{4}$ percent = $\frac{31}{4}$ ÷ $\frac{100}{1}$

$\frac{31}{4}$ percent = $\frac{31}{4}$×$\frac{100}{1}$ we, get

= $\frac{31}{400}$

The given percent 7$\frac{3}{4}$% as a fraction in the simplest form is $\frac{31}{400}$

Page 23 Exercise 30 Problem 14

The value of a certain stock increased by 1 $\frac{1}{4}$%

We need to explain how to write it as a fraction in the simplest form

Given percent is 1$\frac{1}{4}$%

Converting it into fractions, we get

1$\frac{1}{4}$ percent = $\frac{5}{4}$percent

= $\frac{\frac{5}{4}}{100}$

= $\frac{5}{4}$×$\frac{1}{100}$

= $\frac{1}{4}$ × $\frac{1}{20}$

= $\frac{1}{80}$

Hence, The value of $\frac{1}{4}$ percent = $\frac{1}{80}$

Page 24 Exercise 31 Problem 15

Given that, Debra can run 20$\frac{1}{2}$ miles in 2 $\frac{1}{4}$

We need to determine how many miles per hour she can run.

Given that = 20$\frac{1}{2}$

= $\frac{41}{2}$ miles

Time taken = 2 $\frac{1}{4}$

= $\frac{9}{4}$

The unit rate is

Unite rate = $\frac{\text { Number of miles }}{\text { Time taken }}$

= $\frac{\frac{41}{2}}{\frac{9}{4}}$

= $\frac{41}{2}$ × $\frac{4}{9}$

= 41 × $\frac{2}{9}$

= $\frac{82}{9}$

= 9 $\frac{1}{9}$ miles per hour

She can run 9 $\frac{1}{9}$ miles per hour

Page 24 Exercise 32 Problem 16

Among the given options.

We need to determine which of the given complex fraction is equivalent to the value

1) $\frac{\frac{1}{4}}{\frac{1}{2}}$= $\frac{1}{4}$ × $\frac{2}{1}$

= $\frac{2}{4}$

= $\frac{1}{2}$

2) $\frac{\frac{1}{2}}{\frac{1}{2}}$= $\frac{1}{2}$ × $\frac{2}{1}$

= $\frac{2}{2}$

= 1

3) $\frac{\frac{1}{4}}{\frac{4}{1}}$= $\frac{1}{4}$ × $\frac{4}{1}$

= $\frac{4}{4}$

= 1

4) $\frac{\frac{1}{8}}{\frac{1}{2}}$= $\frac{1}{8}$ × $\frac{2}{1}$

= $\frac{2}{8}$

= $\frac{1}{4}$

Here, Option (1) is equal to $\frac{1}{2}$

Hence, Option (1) $\frac{\frac{1}{4}}{\frac{1}{2}}$ is equivalent to $\frac{1}{2}$

Page 24 Exercise 35 Problem 17

We need to determine how many inches does two feet measure using the equivalent customary measurement.

We will use the definitions

We know that the equivalent customary measurement of foot and inches will be

1 foot = 12 inches

We need to calculate how many inches are there for two feet.

Thus

2×1 foot = 2 × 12 inches

2 feet = 24 inches

Hence, 2 feet = 24 inches

Page 24 Exercise 37 Problem 18

We need to determine how many quarts does 8 gallons measure using the equivalent customary measurement.

We know that the equivalent customary measurement of gallons and quarts will be

1 gallon = 4.8038 quarts

We need to calculate how many quarts are there for 8 gallons.

Thus

8 × 1 gallon = 8 × 4.8038 quarts

8 gallons = 38.4304 quarts

8 gallons = 38.4304 quarts

Page 24 Exercise 40 Problem 19

We need to determine how many grams does one-kilogram measures using the equivalent metric measurement.

We need to calculate how many grams are there in one kilogram.

We know that kilo refers to the number 1000

According to the equivalent metric measurement of kilograms and grams

1 kilogram = 1000 grams

1 kilogram = 1000 grams

Page 24 Exercise 40 Problem 20

We need to determine how many grams does one-kilogram measures using the equivalent metric measurement.

We need to calculate how many grams are there in one kilogram.

We know that kilo refers to the number 1000

According to the equivalent metric measurement of kilograms and grams

1 kilogram = 1000 grams

1 kilogram = 1000 grams

Glencoe Math Course 2 Volume 1 Common Core Student Edition Chapter 1 Ratios and Proportional Reasoning Exercise 1.3

June 27, 2024March 8, 2023 by Marksparks

Glencoe Math Course 2 Volume 1 Common Core Chapter 1 Ratios and Proportional Reasoning

Page 25 Exercise 2 Problem 1

We need to determine the given calculations using the equivalent metric measurement.

1 minute = _____ seconds

1 hour = ______ seconds

We need to calculate how many seconds are there in one minute.

According to the equivalent metric measurement of minutes and seconds, we get

1 minute = 60 seconds

Thus, for one hour

We know that

1 hour = 60 minutes

= 60 × 1 minute

= 60 × 60 seconds

= 3600 seconds

1 minute = 60 seconds

1 hour = 3600 seconds

Page 25 Exercise 3 Problem 2

Given:

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.3 Convert Unit Rates Page 25 Exercise 3$

We need to determine the number of feet per second a squirrel can run.

From the given table, the squirrel can run 10 mph Thus

Speed = $\frac{10 \text { miles }}{1 \text { hour }}$

We know that

1 mile = 5280 feet

10 miles = 52800 feet

Similarly

1 hour = 60 minutes

= 60 × 60 seconds

= 3600 seconds

The speed of the squirrel be

Speed $=\frac{52800 \text { feet }}{3600 \text { seconds }}$

= 14. 6667 feet per second

≈ 14.67 feet per second

A squirrel can run 14.67 feet per second.

Page 25 Exercise 4 Problem 3

We need to determine the number of feet per second does it measure for 10 miles per hour

Given that, 10 miles per hour.

We know that

1 mile = 5280 feet

10 miles = 52800 feet

Also, by converting hour to second, we get

1 hour = 60 minutes

= 60×60 seconds

= 3600 seconds

Therefore

$\frac{10 \text { miles }}{1 \text { hour }}=\frac{52800 \text { miles }}{3600 \text { seconds }}$

= 14.667 feet per second

≈ 14.67 feet per second.

10 , miles per hour = 14.67 feet per second.

The number of feet per second does it measure for 10 miles per hour is 14.67 feet per second.

Page 28 Exercise 2 Problem

Given that, A skydiver is falling at about 176 feet per second.

We need to determine how many feet per minute is he falling.

Given falling speed is 176 feet per second.

We know that 1 minute = 60 seconds

Converting the given, we get

$\frac{176 \text { feet }}{1 \text { second }}=\frac{176 \text { feet }}{1 \text { second }} \times \frac{60 \text { second }}{1 \text { minutes }}$

= $\frac{176 \times 60 \text { feet }}{1 \text { minutes }}$

= 10560 feet per minute

He is falling at 10560 feet per minute.

Page 28 Exercise 4 problem 4

Given that, the ratio of$\frac{3 \text { feet }}{1 \text { yard }}$ has a value of one.

We need to determine how it is equal to one.

Given that the ratio is having two different units.

Converting the two units into one.

We know that 1 yard = 3 feet

Thus, the given ratio becomes

$\frac{3 \text { feet }}{1 \text { yard }}=\frac{1 \text { yard }}{1 \text { yard }}$ = 1

Thus, the value of the ratio is one.

Hence, The ratio $\frac{3 \text { feet }}{1 \text { yard }}$ = 1 since the value of 3 feet = 1 yard

Page 29 Exercise 1 Problem 5

Given that, A go-kart’s top speed is 607,200 feet per hour.

We need to determine its speed in miles per hour.

The speed of the go-kart = $\frac{607,200 \text { feet }}{1 \text { hour }}$

We must convert the speed in feet to miles.

We know that 1 feet = 0.000189394 miles

Thus, by converting, we get

$\frac{607200 \text { feet }}{1 \text { hour }} \times \frac{0.000189394 \text { miles }}{1 \text { feet }}$

=$\frac{607200 \times 0.000189394 \text { miles }}{1 \text { hour }}$

= 115 miles per hour

Hence, The speed is 115 miles per hour.

Page 29 Exercise 2 problem 6

Given that, the fastest a human has ever run is 27 miles per hour.

We need to determine how many miles per minute the human ran.

Given:

The fastest speed of the human = $\frac{27 \text { miles }}{1 \text { hour }}$

Converting the hour into minutes, we get

1 hour = 60 minutes

Thus, the speed becomes

$\frac{27 \text { miles }}{1 \text { hour }}=\frac{27 \text { miles }}{1 \text { hour }} \times \frac{1 \text { hour }}{60 \text { minutes }}$

= $\frac{27 \text { miles }}{60 \text { minutes }}$

= $\frac{0.45 \text { miles }}{1 \text { minute }}$

The human ran 0.45 miles per minute.

Page 29 Exercise 3 Problem 7

Given that, A peregrine falcon can fly 322 kilometers per hour.

We need to determine how many meters per hour the falcon can fly.

Given:

Speed of the falcon = $\frac{322 \text { Kilometers }}{1 \text { hour }}$

Converting kilometers to meters, we get

= $\frac{322 \text { kilometers }}{1 \text { hour }}=\frac{322 \text { kilometers }}{1 \text { hour }} \times \frac{1000 \text { meters }}{1 \text { kilometer }}$

= $\frac{322 \times 1000 \text { meters }}{1 \text { hour }}$

= $\frac{322 \text { meters }}{1 \text { hour }}$

The falcon can fly 322000 meters per hour.

Page 29 Exercise 4 Problem 8

Given that, A pipe is leaking at 1.5 cups per day.

We need to determine how many gallons per week the pipe is leaking.

Also, given 1 gallon is equivalent to 16 cups.

Determine one cup is equivalent to how many gallons.

1 gallon = 16 cups

$\frac{1}{16}$ gallon = 1 cup

Determine one day is equivalent to how many weeks

1 week = 7 days

$\frac{1}{7}$ week = 1 day

Converting the given cups to gallons, we get

$\frac{1.5 \text { cups }}{1 \text { day }}=\frac{1.5 \text { cups }}{1 \text { day }} \times \frac{\frac{1}{16} \text { gallon }}{1 \text { cup }} \times \frac{1 \text { day }}{\frac{1}{7} \text { week }}$

= $\frac{1.5 \times \frac{1}{16} \text { gallons }}{\frac{1}{7} \text { week }}$

= $\frac{0.09375 \text { gallons }}{\frac{1}{7} \text { week }}$

= 0.65625 gallons per week

The pipe is leaking at 0.65625 gallons per week.

Page 30 Exercise 7 Problem 9

Given that, the speed at which a certain computer can access the Internet is 2 megabytes per second.

We need to determine how fast is this in megabytes per hour.

Given:

Speed of the computer = $\frac{2 \text { megabytes }}{1 \text { second }}$

We know that

1 hour = 3600 seconds

$\frac{1}{3600}$ hour = 1 second

Converting we get

$\frac{2 \text { megabytes }}{1 \text { second }}$ = $\frac{2 \text { megabytes }}{1 \text { second }} \times \frac{1 \text { second }}{\frac{1}{3600} \text { hour }}$

= $\frac{2 \text { megabytes }}{\frac{1}{3600} \text { hour }}$

= 7200 megabytes per hour

The computer can access the internet in 7200 megabytes per hour.

Page 30 Exercise 8 Problem 10

Given that, the approximate metric measurement of length is given for the U.S.

a customary unit of length. We need to use our estimation skills to complete the graphic organizer below.

Fill in each blank with the foot, yard, inch, or mile.

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.3 Convert Unit Rates Page 30 Exercise 8$

We know that the metric to customary measurements are
1 inch = 2.54 centimeters
1 feet = 0.30 meter
1 yard = 0.91 meter
1 miles = 1.61 kilometer

Thus

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.3 Convert Unit Rates Page 30 Exercise 8 .$

Hence, The Complete Figure is given below:

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.3 Convert Unit Rates Page 30 Exercise 8 ..$

Page 30 Exercise 9 Problem 11

The unit rate is nothing but the rate per one unit of the material or a thing.

For example, if we come across a grocery store in the market and if we want to buy five kilograms of tomato, the shopkeeper tells us that the price of the tomatoes per kilogram will be $5

In this way, we can easily calculate how much the price will be if we want to buy more kilograms of tomatoes.

In this case, we need to buy 5 kilograms.

Thus, the price will be

Unit price × Number of Kilograms of tomatoes we need to buy

= 5 × 5

= 25 dollars

Like this, we can easily calculate the price of the thing for any quantity if we know the unit price.

The rate or price of anything for any varying quantity can be easily deduced if we know the unit rate or unit price.

Page 30 Exercise 10 Problem 12

Given that, we need to determine and explain if we convert 100.

feet per second to inches per second, will there be more or less than 100 inches.

Given that

1 feet = 12 inches

Converting the given, we get

$\frac{100 \text { feet }}{1 \text { second }}=\frac{100 \text { feet }}{1 \text { second }} \times \frac{12 \text { inches }}{1 \text { feet }}$

= $\frac{100 \times 12 \text { inches }}{1 \text { second }}$

= $\frac{1200 \text { inches }}{1 \text { second }}$

When you convert 100 feet per second to inches per second, there be more than 100 inches.

Since 100 feet per second = 1200 inches per second

Page 30 Exercise 11 problem 13

We need to convert 7 meters per minute to yards per hour.

We know that

1 meters = 1.09361 yards

1 hour = 60 minutes

$\frac{1}{60}$hour = 1 minute

Converting the given, we get

$\frac{7 \text { meters }}{1 \text { minute }}=\frac{7 \text { meters }}{1 \text { minute }} \times \frac{1.09361 \text { yards }}{1 \text { meter }} \times \frac{1 \text { minute }}{\frac{1}{60} \text { hour }}$

$ = \frac{7.65529 \text { yards }}{\frac{1}{60} \text { hour }}$

= 7.65529 × 60

= 459.3174 yards per hour

Thus, 7 meters per minute will be 459.3174 yards per hour

Page 30 Exercise 12 Problem 14

Given that, A salt truck drops 39 kilograms of salt per minute.

We need to determine how many grams of salt the truck drops per second From the given options.
(1) 600
(2) 625
(3) 650
(4) 6,000

Converting the given, we get

$\frac{39 \text { kilograms }}{1 \text { minute }}=\frac{39 \text { kilograms }}{1 \text { minute }} \times \frac{1000 \text { grams }}{1 \text { kilogram }} \times \frac{1 \text { minute }}{60 \text { seconds }}$

$ = \frac{39000 \text { grams }}{60 \text { seconds }}$

= 650 grams per second

Hence, Option (3) 650 grams of salt the truck drops per second.

Page 31 Exercise 13 Problem 15

We need to convert 20 mi/h to feet per minute.

Converting the given we get

$\frac{20 \text { miles }}{1 \text { hour }}=\frac{20 \text { miles }}{1 \text { hour }} \times \frac{5280 \text { feet }}{1 \text { mile }} \times \frac{1 \text { hour }}{60 \text { minutes }}$

= $\frac{20 \times 5280 \mathrm{feet}}{60 \text { minutes }}$

= 1760 feet per minute

20 mi/h = 1760 feet/ minute

Page 31 Exercise 14 Problem 16

Converting the given we get

$\frac{16 \mathrm{~cm}}{1 \mathrm{~min}}=\frac{16 \mathrm{~cm}}{1 \mathrm{~min}} \times \frac{\frac{1}{100} \mathrm{~m}}{1 \mathrm{~cm}} \times \frac{1 \mathrm{~min}}{\frac{1}{60} \text { hour }}$

= $\frac{16 \times \frac{1}{100} \mathrm{~m}}{\frac{1}{60} \text { hour }}$

= $\frac{16}{100} \times 60 \text { meter / hour }$

= $\frac{16 \times 6}{10} \text { meter / hour }$

= 9.6 meter/hr

16 cm/min = 9.6 meter/hr

Page 31 Exercise 16 Problem 17

We need to convert 26 cm/s to m/min.

Converting the given we get

$\frac{26 \mathrm{~cm}}{1 \mathrm{~second}}=\frac{26 \mathrm{~cm}}{1 \mathrm{~second}} \times \frac{1 \text { meter }}{100 \mathrm{~cm}} \times \frac{60 \text { seconds }}{1 \text { minute }}$

$ = \frac{26 \times 60 \text { meter }}{100 \text { minute }}$

= 15.6 meter/ minute

26cm/s = 15.6 m/minute

Page 31 Exercise 17 Problem 18

We need to convert 24 mi/h to feet per second.

Converting the rates, we get

$\frac{24 \text { miles }}{1 \text { hour }}=\frac{24 \text { miles }}{1 \text { hour }} \times \frac{5280 \text { feet }}{1 \text { mile }} \times \frac{1 \text { hour }}{3600 \text { seconds }}$

= $\frac{24 \times 5280 \text { feet }}{3600 \text { seconds }}$

= 35.2 ft/sec

24 mi/h = 35.2 ft/s

Page 31 Exercise 18 Problem 19

We need to convert 105.6 L/h to L/min.

Converting the given we get

$\frac{105.6 \mathrm{~L}}{1 \text { hour }}$=$\frac{105.6 \mathrm{~L}}{1 \text { hour }} \times \frac{1 \text { hour }}{60 \text { minutes }}$

= $\frac{105.6 \mathrm{~L}}{60 \text { minutes }}$

= 1.76 L/min

1056 L/h = 1.76L/min

Page 32 Exercise 20 Problem 20

We need to convert Thirty-five miles per hour to feet per minute.

Converting the rates, we get

$\frac{35 \text { miles }}{1 \text { hour }}=\frac{35 \text { miles }}{1 \text { hour }} \times \frac{5280 \text { feet }}{1 \text { mile }} \times \frac{1 \text { hour }}{60 \text { minutes }}$

$ = \frac{35 \times 5280 \text { feet }}{60 \text { minutes }}$

= 3080 feet per minute

Thirty-five miles per hour is the same rate as 3,080 feet per minute.

Page 32 Exercise 21 Problem 21

Given that, a boat is traveling at an average speed of 15 meters per second.

We need to determine how many kilometers per second the boat is traveling.

Converting the rates, we get

$\frac{15 \text { meters }}{1 \text { second }}=\frac{15 \text { meters }}{1 \text { second }} \times \frac{1 \text { kilometer }}{1000 \text { meter }}$

$=\frac{15 \text { Kilometer }}{1000 \text {second}}$

= 0.015 Kilometer/ second

A boat is traveling at an average speed of 15 meters per second.

The boat is traveling at 0.015 kilometers per second.

Page 32 Exercise 22 Problem 22

Given that, an oil tanker empties at 3.5 gallons per minute.

We need to convert this rate to cups per second.

Converting the rates, we get

$\frac{3.5 \text { gallons }}{1 \text { minute }}=\frac{3.5 \text { gallons }}{1 \text { minute }} \times \frac{16 \text { cups }}{1 \text { gallon }} \times \frac{1 \text { minute }}{60 \text { seconds }}$

= $\frac{3.5 \times 16 \mathrm{cups}}{60 \text { seconds }}$

= 0.93 cups/second

An oil tanker empties at 3.5 gallons per minute.

This rate in cups per second is 0.93 cups /second

Page 32 Exercise 23 Problem 23

Given that

$36 for 4 basketball hats; $56 for 7 basketball hats.

We need to show that they are equivalent are not.

Find the values of each ratio:

For the first case

$\frac{36 \text { dollars }}{4 \text { basketball hats }}=9 \text { dollars/hat }$

For the second case

$\frac{56 \text { dollars }}{7 \text { basketball hats }}=8 \text { dollars/hat }$

Both the values of the ratios are different.

Thus, they are not equivalent.

The values of the ratios are different. Thus, they are not equivalent.

Page 32 Exercise 24 Problem 24

Given that 12 posters for 36 students; 21 posters for 63 students

We need to show that they are equivalent are not.

Find the values of each ratio:

For the first case:

$\frac{12}{36}$ = $\frac{1}{3}$

For the second case:

$\frac{21}{63}$ = $\frac{1}{3}$

Both the values of the ratios are the same. Thus, they are equivalent.

The values of the ratios are the same. Thus, they are equivalent.

Page 32 Exercise 25 Problem 25

Given that, an employer pays $22 for 2 hours.

We need to use the ratio table to determine how much she charges for 5 hours.

The unit rate per hour is

$\frac {22}{2}$=$\frac{11 \text { dollars }}{1 \text { hour }}$

Thus, the employer pays 11 dollars per hour. Thus, for 5 hours

5 hours $\times \frac{11 \text { dollars }}{1 \text { hour }}=5 \times 11 \text { dollars }$

= 55 dollars

For 5 hours, she charges $55 26

$Glencoe Math Course 2, Volume 1, Common Core Student Edition, Chapter 1.3 Convert Unit Rates Page 25 Exercise 32$