Big Idea Math

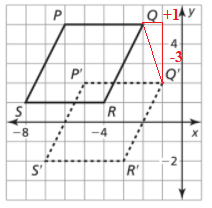
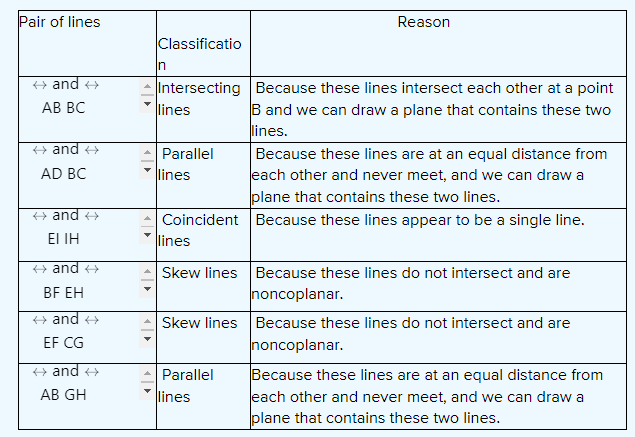
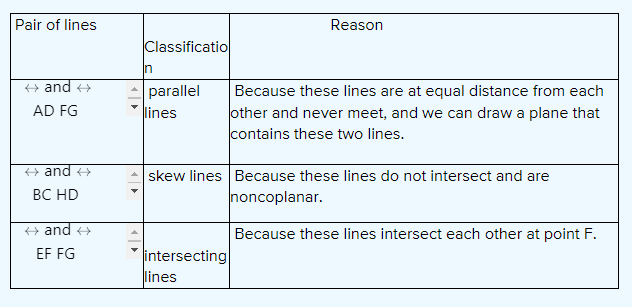
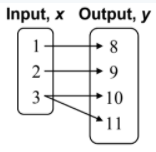
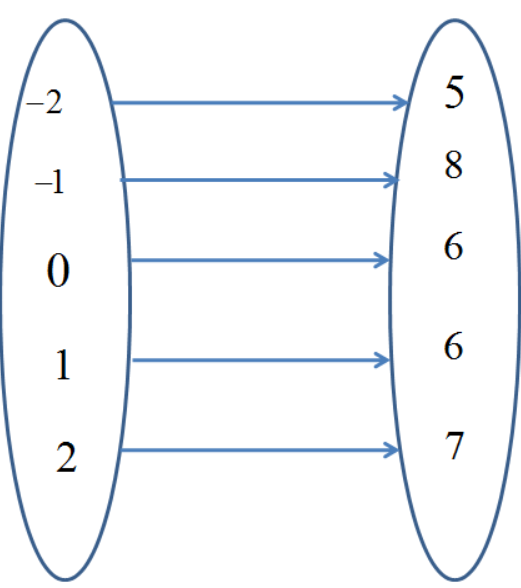
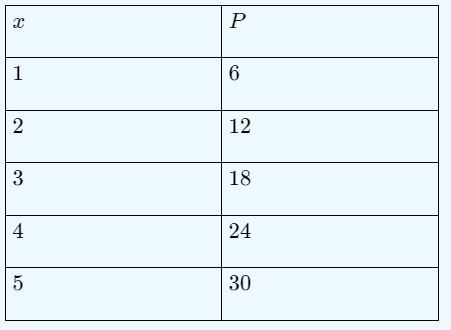
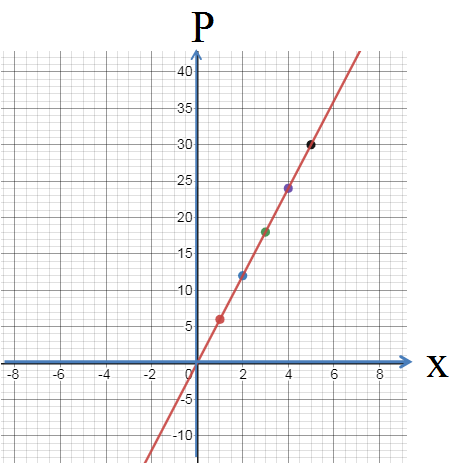
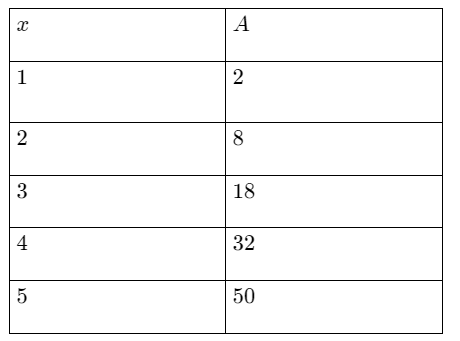
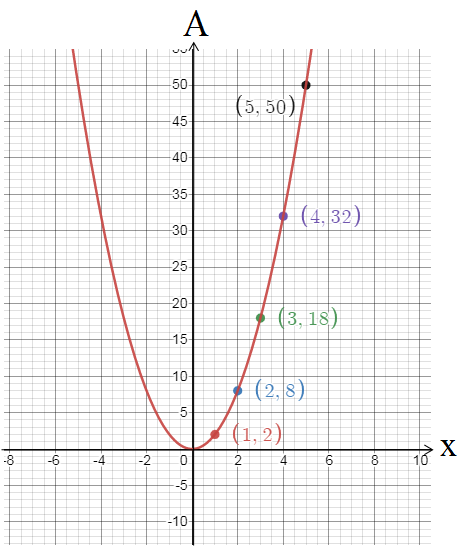
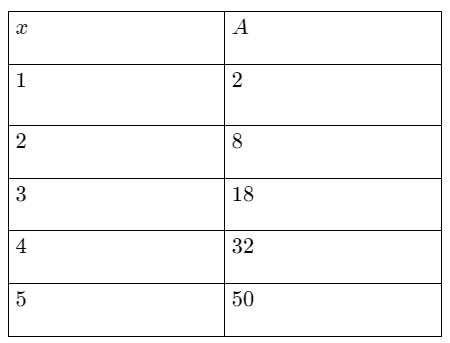
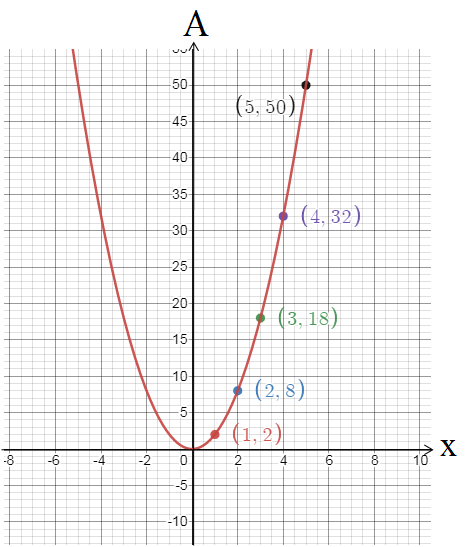
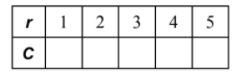
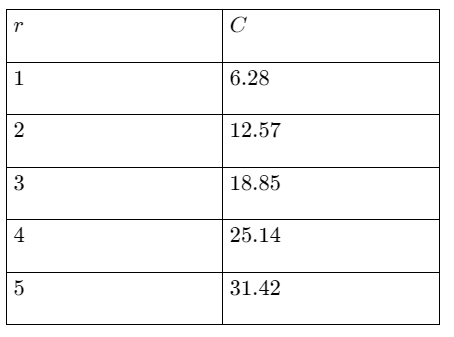
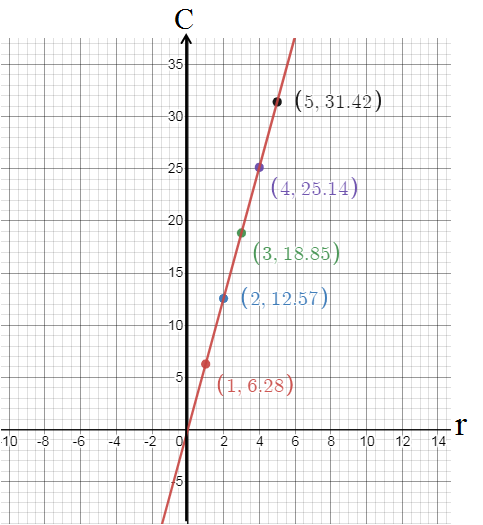
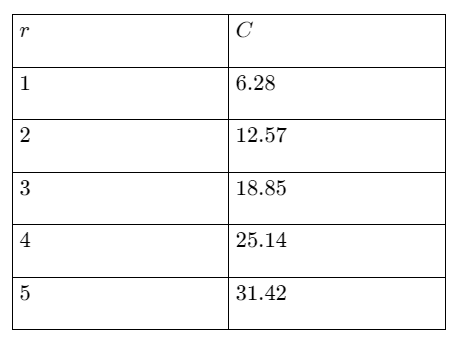
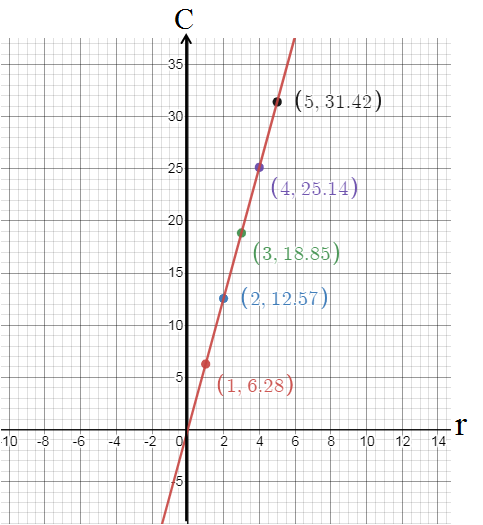
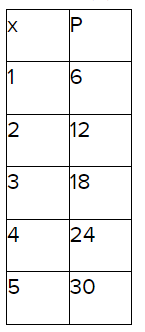
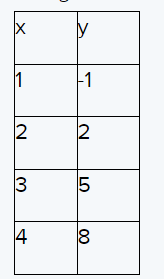
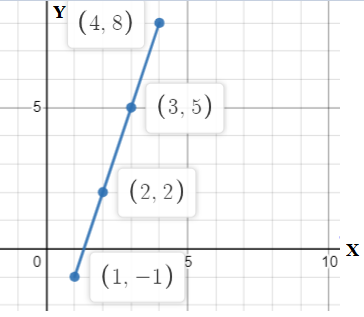
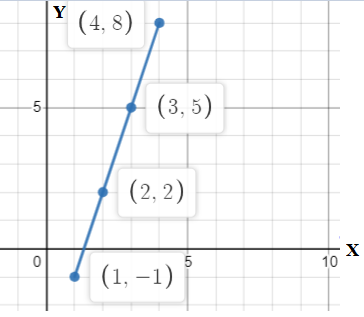
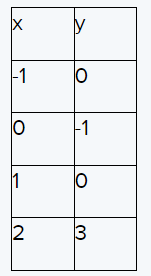
Big Ideas Math Integrated Math Chapter 12 Maintaining Mathematical Proficiency Exercise

 

Page 322  Exercise 1  Problem 1

Question 1.

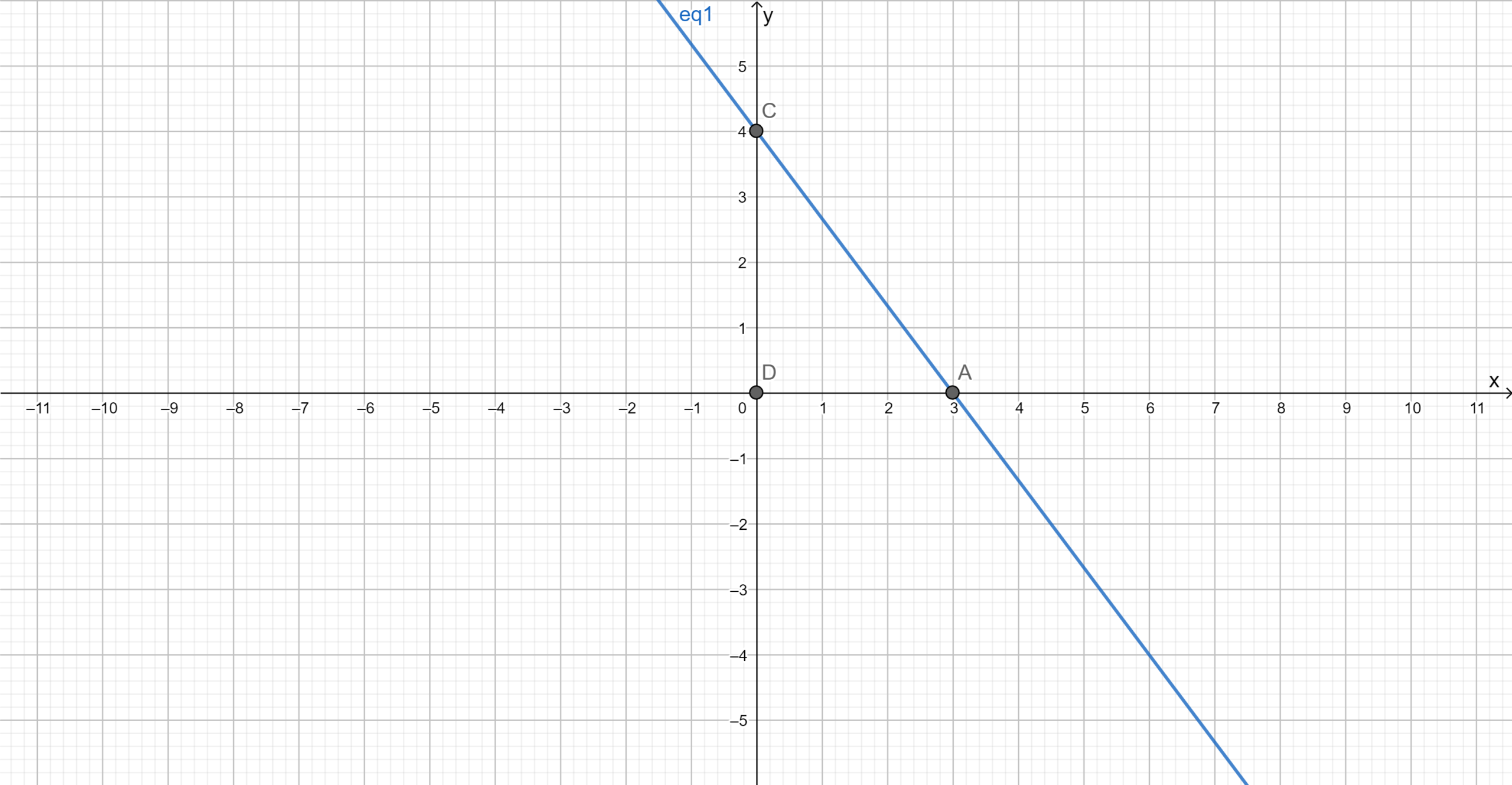
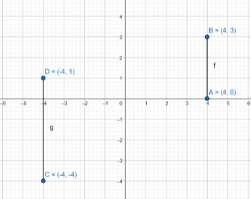
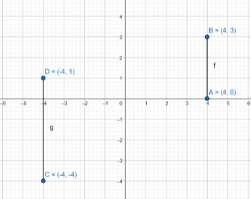
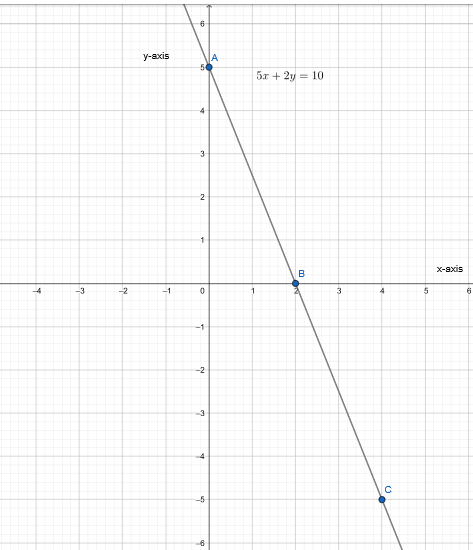
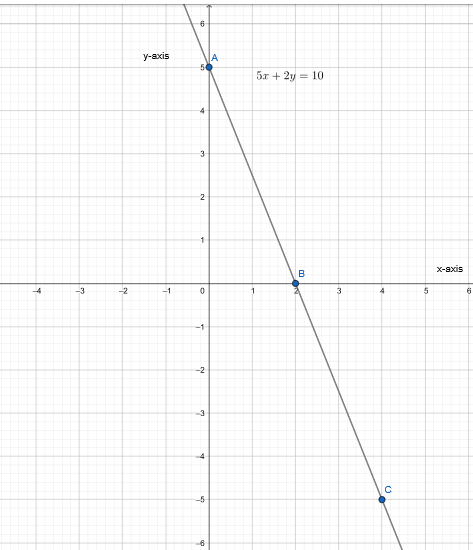
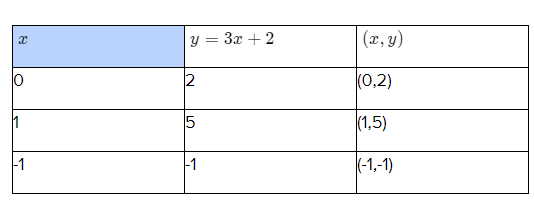
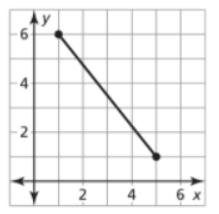
Given a line segment AB with endpoints A(3,1) and B(5,5):

1. Calculate the coordinates of the midpoint M of the line segment AB.
2. Calculate the distance between the endpoints A and B.

Answer:

Given

In this question, we are given a line segment AB, the coordinates of the end point of which are given as-A(3,1) and B(5,5).

We have to calculate the following-

(1) The coordinates of the mid point, M of the line segment AB.

(2) The distance between the end points i.e. the length of the line

Let the coordinates of the midpoint M be given as(x,y).

We rewrite the midpoint formula as-

⇒  (x,y) = (\(\frac{a+c}{2}\),\(\frac{b+d}{2}\))———— (1)

Here, we havea,b,c &d as 3,1,5 &5 respectively.

Substituting these values in eq(1),we get

⇒  (x,y) =  (\(\frac{3+5}{2}\),\(\frac{1+5}{2}\))

Adding the values, we get

⇒  (x,y) = (\(\frac{8}{2}\),\(\frac{6}{2}\))

Simplifying it further, we get

⇒  (x,y) = (4,3)

The coordinates of the midpoint of the line segment AB is given as M (4,3).

Now, we have to compute the distance between the endpoints A and B of the line segment AB.

So, denoting the distance between the endpoints as AB.

We rewrite the formula as-

⇒  AB = \(\sqrt{(c-a)^2+(d-b)^2}\) ——– (2)

Substituting the values as per the question in equation (2),we get

⇒  AB = \(\sqrt{(5-3)^2+(5-1)^2}\)

Solving it we get

⇒  AB = \(\sqrt{2^2+4^2}\)

⇒  AB = \(\sqrt{4+16}\)

⇒  AB = \(\sqrt{20}\)

⇒  AB = 2\(\sqrt{5}\)

Thus, the distance between the endpoints is given as 2\(\sqrt{5}\) units.

The midpoint M of the line segment AB is found to be(4,3) the distance between the endpoints is calculated as 2\(\sqrt{5}\) units.

 

Page 322  Exercise 2  Problem 2

Question 2.

Given a line segment PB with endpoints P(-2,-7) and B(-4,5):

1. Calculate the coordinates of the midpoint M of the line segment PB.
2. Calculate the distance between the endpoints P and B of the line segment PB.

Answer:

Given

In this question, we are given a line segment PB, the coordinates of the end point of which are given as P(−2,−7) and B(−4,5).

We have to calculate the following-

(1) The coordinates of the mid point, M of the line segment PB.

(2) The distance between the end points i.e. the length of the line segment PB.

We will use the midpoint formula and the distance formula for calculating these.

Let the coordinates of the midpoint M be given as (x,y).

We rewrite the midpoint formula as-

⇒ (x,y) = (\(\frac{a+c}{2}\),\(\frac{b+d}{2}\))——- (1)

Here, we have a,b,c & d as−2,−7,−4&5 respectively.

Substituting these in equation (1), we get

⇒  (x,y)  = (\(\frac{-2+(-4)}{2}\),\(\frac{-7+5}{2}\))

Solving it, we get

⇒  (x,y) = (\(\frac{−6}{2}\),\(\frac{-2}{2}\))

The coordinates of the midpoint of the line segment PB is given as M (-3,-1).

Now, we have to compute the distance between the endpoints P and B of the line segment PB.

So, denoting the distance between the endpoints as PB.

We rewrite the distance formula as-

⇒ PB = \(\sqrt{(c-a)^2+(d-b)^2}\) ——– (2)

Substituting the values as per the question in equation (2),we get

⇒  PB = \(\sqrt{(-4+2)^2+(5+7)^2}\)

⇒  PB = \(\sqrt{(-2)^2+12^2}\)

⇒  PB = \(\sqrt{4+144}\)

⇒  PB = \(\sqrt{148}\)

⇒  PB = 2\(\sqrt{37}\) Units.

Thus, the distance between the endpoints of the line segment is given as

⇒ PB = 2\(\sqrt{37}\) units.

The coordinates of the midpoint of the line segment PB is found to be M(−3,−1). the distance between the endpoints of the line segment is calculated as 2\(\sqrt{37}\) units.

 

Page 322  Exercise 3  Problem 3

Question 3.

Solve the following equation for the variable x:

9x – 6 = 7x

Answer:

Given

9x – 6 = 7x

Here, we are given an equation and we have to solve for the value of the variable x.

We will solve it by taking all the like terms on one side of the equality and then solve for the value of x.

We rewrite the given equation as follows-

⇒  9x − 6 = 7x

Taking the like terms on one side of the equality, we get

⇒  9x − 7x = 6

⇒  2x = 6

⇒  x = 3

The value of the variable x is found to 3.

 

Page 322  Exercise 4  Problem 4

Question 4.

Solve the following equation for the variable r:

2r + 6 = 5r − 9

Answer:

Given

2r + 6 = 5r − 9

Here, we are given an equation and we have to solve for the value of the variabler.

We will solve it by taking all the like terms on one side of the equality and then solve for the value of r.

We rewrite the given equation as follows-

⇒  2r + 6 = 5r − 9

Taking the variable term on right side of the equality an the constant term on the left side of the equality, we get

⇒  6 + 9 = 5r   2r

Rearranging it, we get

5r − 2r = 6 + 9

Solving it, we get

⇒  3r = 15

⇒  r = 5

The equation is solved and the value of the variable r is determined as r= 5.

 

Page 322  Exercise 5  Problem 5

Question 5.

Solve the following equation for the variable n:

20 – 3n = 2n + 30

Answer:

Given

20 – 3n = 2n + 30

Here, we are given an equation and we have to solve for the value of the variable n.

We will solve it by taking all the like terms on one side of the equality and then solve for the value of n.

We rewrite the given equation as follows-

⇒ 20−3n = 2n + 30

Taking the variable terms on the left side of the equality and the constants on the right, we get

⇒ −3n− 2n = 30 − 20

Solving it, we get

⇒ −5n = 10

⇒ n = \({10}{−5}\)

⇒ n = −2

The equation is solved and the value of the variablen is determined as n = −2.

 

Page 323  Exercise 6  Problem 6

Question 6.

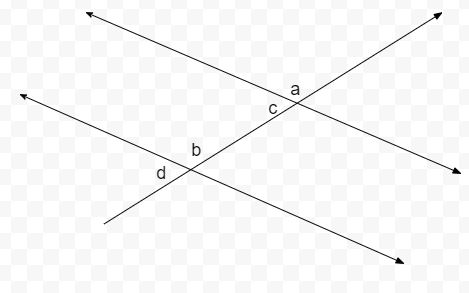
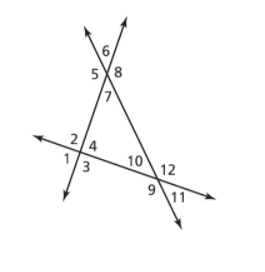
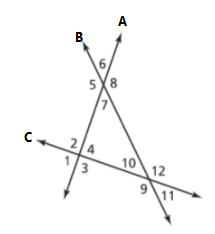
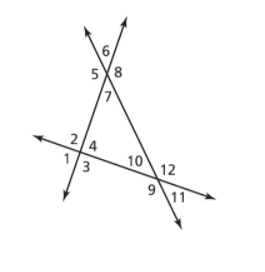
What is the relation between the measures of the interior angles of a triangle?

Answer:

The relation between the measures of the interior angles of a triangle

Here, we are asked the relation between the angle measures of a triangle.

The angles or more specifically the interior angles of a triangle are not related to each other directly, but combinedly, they form a relation.

We will be finding that relation here as follows

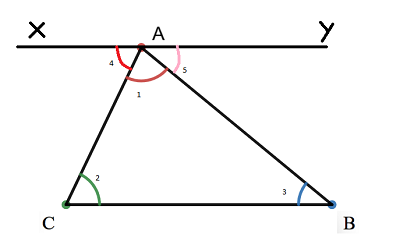
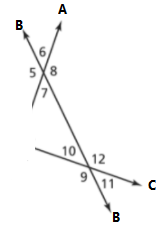
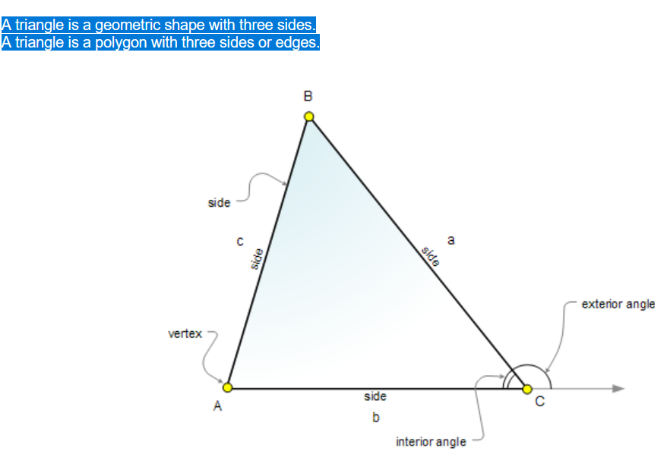
First, we construct a triangle as follows – 

The triangle is named ABC and its interior angles are named as 1,2 and 3.

Next, we have constructed a line XY passing through the vertex A of the triangle and parallel to the base BC of the triangle.

We can observe that at the vertex A, ∠1,∠4  and ∠5 form a linear pair.

Thus, we can say that

∠1 + ∠4 + ∠5 = 180°

Now, as XY//BC, so AB forms a transversal.

So, we can say that ∠3 = ∠5 (Alternate∠s)

Similarly, AC also forms a transversal, hence we can say that
∠2 = ∠4  (Alternate∠s)

Thus, we can say that ∠1 + ∠2 + ∠3 = 180°

Thus, we get the relation between the angles of a triangle.

The relation between the angles of a triangle is that their sum is equal to 180°. This relation is true for any triangle.

 

Page 323  Exercise 7  Problem 7

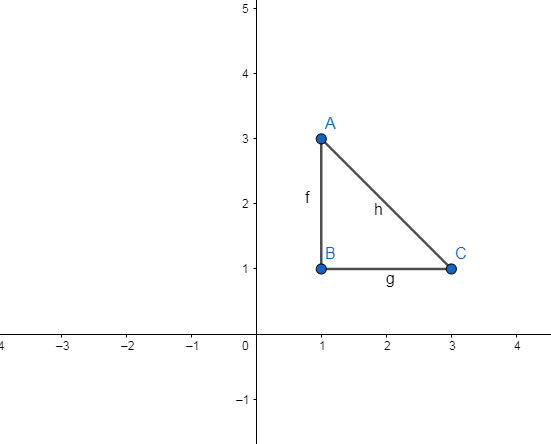
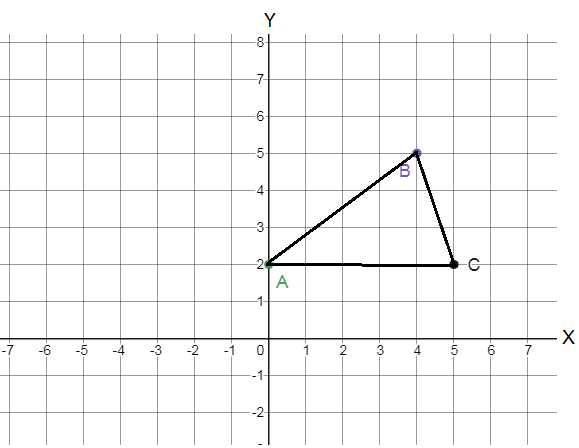
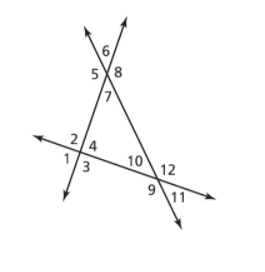
Question 7.

Construct a triangle using dynamic geometry software, such as Desmos.com. Label the vertices of the triangle and determine the type of triangle based on the lengths of its sides and its interior angles.

Answer:

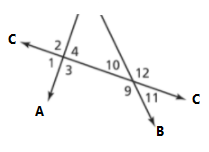
Here, we are asked to construct a triangle using any dynamic geometry software.

So, here we have used Desmos.com, an online geometry tool for constructing the triangle  ΔABC.

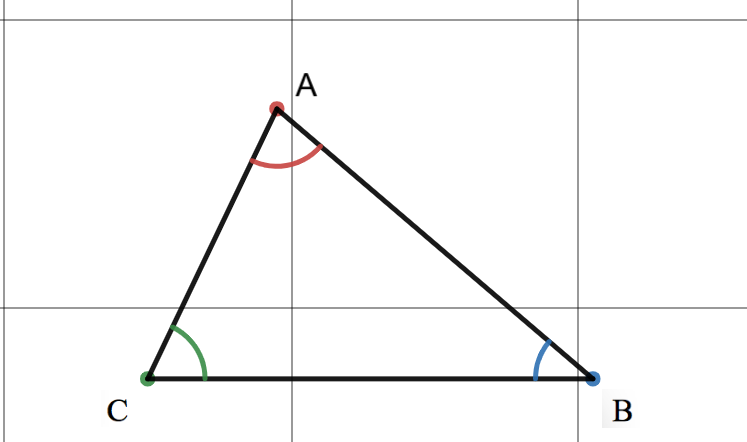
We have labelled the top vertex as A and the bottom two as B and C.

The triangle here is a scalene triangle as all the sides are of different lengths.

Also the interior angles of the triangle constructed are not equal to each other.

The triangle constructed is as follows

 

Page 323  Exercise 7  Problem 8

Question 8.

Using a protractor, measure the interior angles of ΔABC. The measures are as follows.

• ∠A = 70°
• ∠B = 60°
• ∠C = 50°

Answer:

Now we use a protractor to measure the interior angles of the ΔABC.

​∠A = 70°

∠B = 60°

∠C = 50°

​

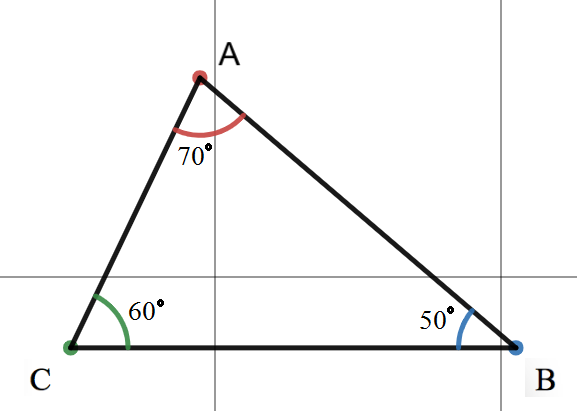
The measures of the interior angles of theΔABC are as follow ∠A = 70° , ∠B = 60° , ∠C = 50°

 

Page 323  Exercise 7 Problem 9

Question 9.

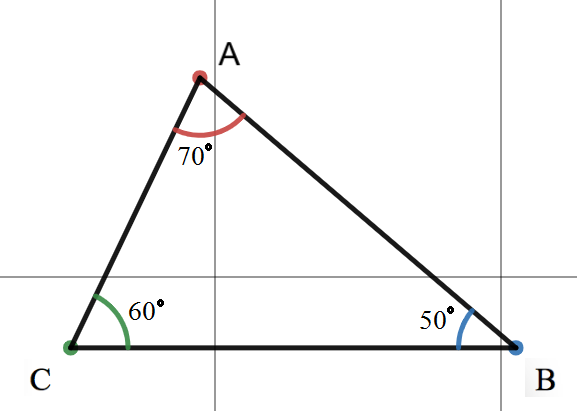
Given the measures of the interior angles of ΔABC

• ∠A = 70°
• ∠B = 60°
• ∠C = 50°

Verify that the sum of the interior angles of ΔABC is 180°.

Answer:

From Page 323 Exercise 7 Problem 8 we have

​∠A = 70°

∠B = 60°

∠C = 50°

⇒ ∠A + ∠B + ∠C = 70° + 60° + 50°

⇒ ∠A + ∠B + ∠C = 180°

The sum of the interior angle measures is 180° i.e.∠A + ∠B + ∠C = 180°.

 

Page 323   Exercise 7   Problem 10

Question 10.

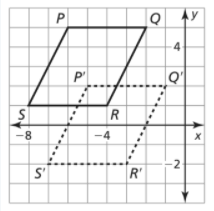
Construct one or two more triangles, measure the interior angles of each triangle, and find the sum of the interior angles of each triangle. Verify that the sum of the interior angles of a triangle is always 180°.

Answer:

Construct one or two more triangles and then measure the interior angles of the triangles.

Find the sum of interior angles of each triangle.

We found that sum of interior angles of a triangle is always 180∘

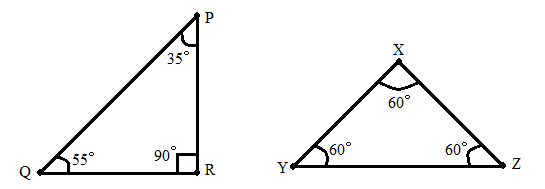
From diagram it is clear that

∠P + ∠Q + ∠R

= 35° + 55° + 90°

= 180°

Again

∠X + ∠Y + ∠Z

= 60° + 60° + 60°

= 180°

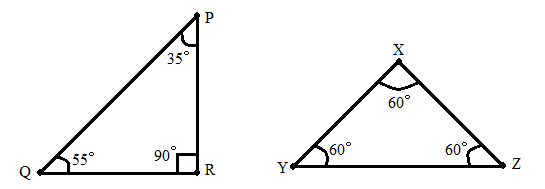
The sum of interior angles of a triangle is always 180°.

 

Page 324  Exercise 8  Problem 11

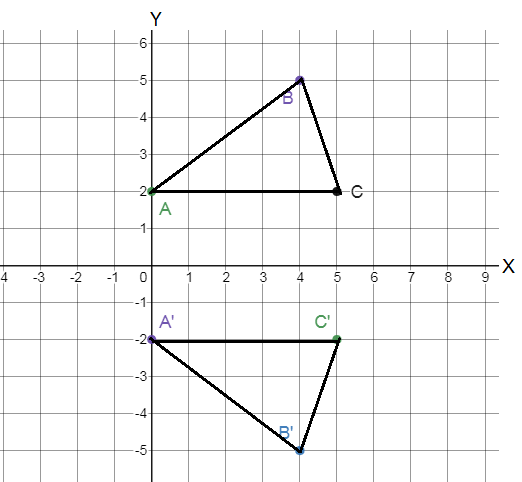
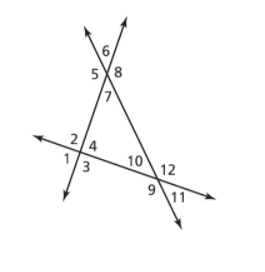
Question 11.

Using Desmos.com, an online geometry tool, construct a triangle ABC. After constructing the triangle, measure the interior angles and verify that the sum of the interior angles is 180°.

Answer:

Here, we are asked to construct a triangle using any dynamic geometry software.

So, here we have used Desmos.com, an online geometry tool for constructing the triangle ΔABC.

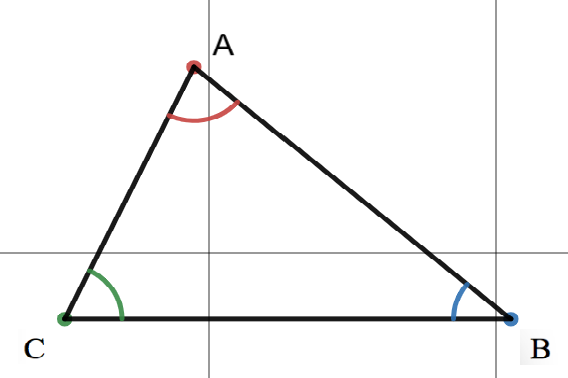
The triangle constructed is as follows-

 

Page 324  Exercise 8  Problem 12

Question 12.

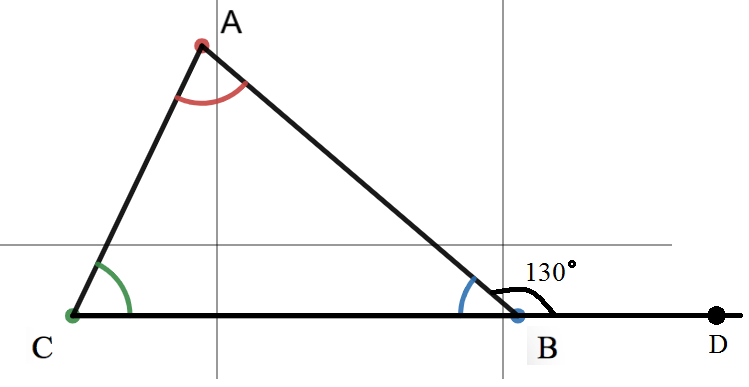
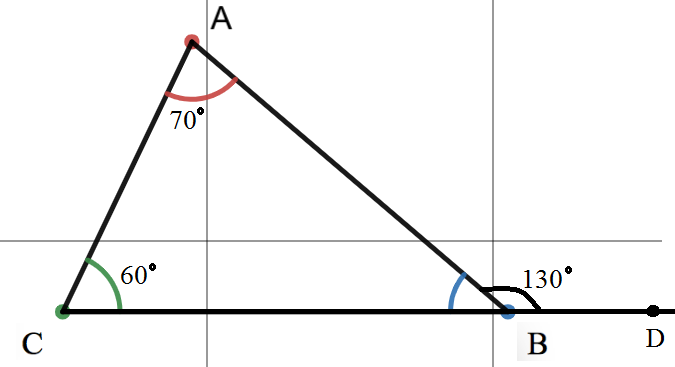
In ΔABC drawn on Page 324 Exercise 8 Problem 11, extend CB to point D to construct an exterior angle at vertex B.

1. Measure the exterior angle ∠ABD using a protractor.
2. Verify the relationship between the exterior angle ∠ABD and the remote interior angles ∠A and ∠C.

Answer:

In ΔABC drawn in Page 324 Exercise 8 Problem 11 , extend CB to a point D to construct exterior angle at vertex B.

Now use protractor to find measure of the exterior angle.

By using protractor we can measure Exterior angle i.e. ∠ABD = 130°

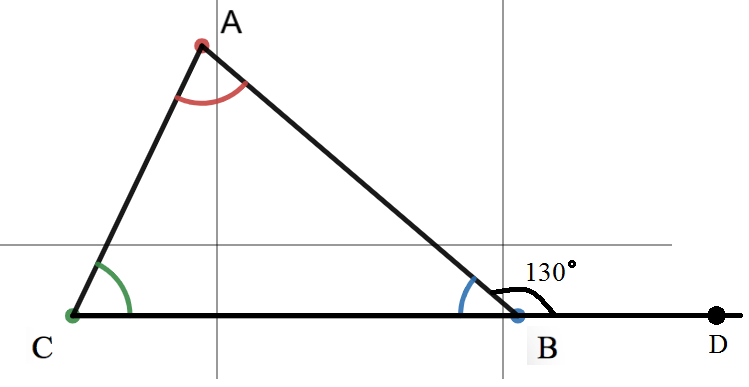
Measure of exterior angle i.e.∠ABD is 130°.

 

Page 324  Exercise 8  Problem 13

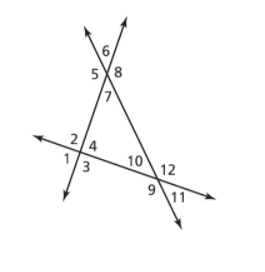
Question 13.

Using a protractor, measure the two nonadjacent interior angles of ΔABC and verify the relationship between these angles and the exterior angle at vertex B.

Given Measurements:

• ∠CAB = 70°
• ∠ACB = 60°

Answer:

By using protractor we can measure the two nonadjacent interior angles of the triangle.

So we have

∠CAB  = 70°

∠ACB = 60°

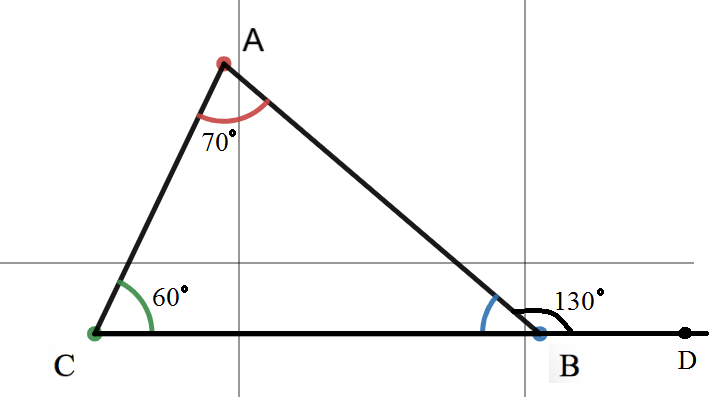
The measures of the two nonadjacent interior angles of ΔABC are given by ∠CAB = 70°,  ∠ACB = 60°

 

Page 324  Exercise 8  Problem 14

Question 14.

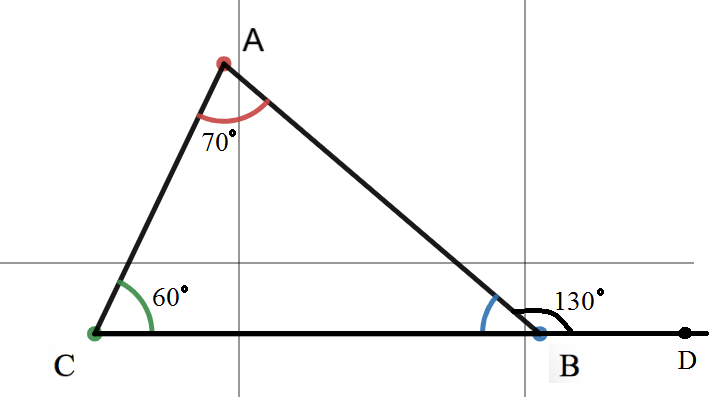
Given the measurements of two nonadjacent interior angles of ΔABC and the exterior angle at vertex B:

• ∠CAB = 70
• ∠ACB = 60
• ∠ABD = 130

Verify the relationship between the sum of the measures of the two nonadjacent interior angles and the exterior angle at vertex B.

Answer:

From Page 324 Exercise 8 Problem 13 we have

∠CAB = 70°

∠ACB = 60°

⇒ ∠CAB + ∠ACB = 70° + 60°

⇒ ∠CAB + ∠ACB = 130°

Now ∠ABD = 130°

⇒ ∠ABD = ∠CAB + ∠ACB

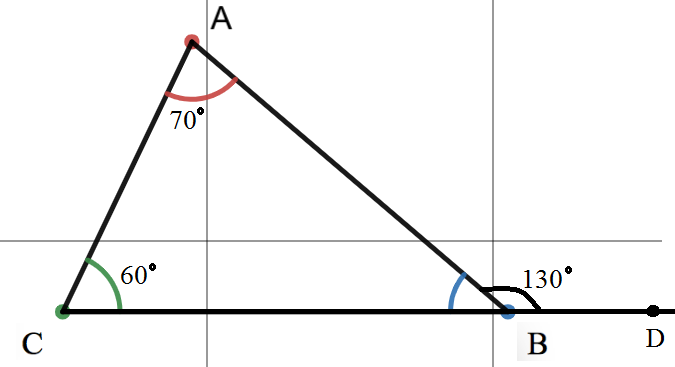
The sum of the measures of the two nonadjacent interior angles is 130° i.e. ∠CAB + ∠ACB = 130°. Also ∠ABD = ∠CAB + ∠ACB

 

Page 324  Exercise 9  Problem 15

Question 15.

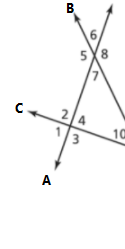
Find the relationship between the angle measures of a triangle. Use a construction to demonstrate this relationship.

Answer:

Here, we are asked the relation between the angle measures of a triangle.

The angles or more specifically the interior angles of a triangle are not related to each other directly, but in combination, they form a relation.

We will be finding that relation here as follows

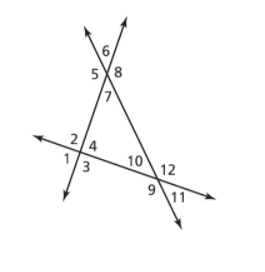
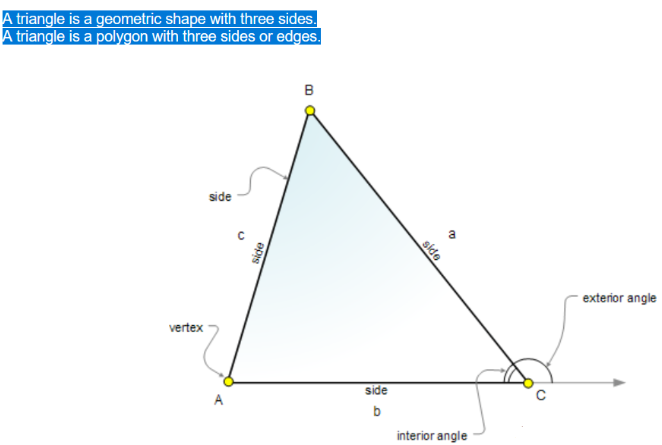
First, we construct a triangle as follows-

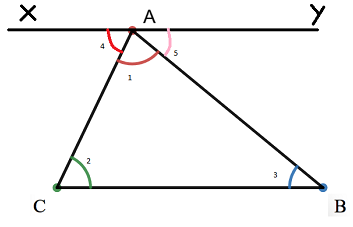
The triangle is named ABC and its interior angles are named as 1,2 and 3.

Next, we have constructed a line XY passing through the vertex A of the triangle and parallel to the base BC of the triangle.

We can observe that at the vertex A∠1,∠4 ,and ∠5 form a linear pair.

Thus, we can say that

∠1 + ∠4 + ∠5 = 180°

Now, as XY//BC, so AB forms a transversal. So, we can say that

∠3 = ∠5 (Alternate∠s)

Similarly, AC also forms a transversal, hence we can say that

∠2 = ∠4 (Alternate∠s)

Thus, we can say that

∠1 + ∠2 + ∠3 = 180°

Thus, we get the relation between the angles of a triangle.

The relation between the angles of a triangle is that their sum is equal to180°

This relation is true for any triangle.

 

Page 324  Exercise 10  Problem 16

Question 16.

Given that the exterior angle of a triangle measures 32°:

1. Use the property of exterior angles to determine the sum of the measures of the two nonadjacent interior angles.
2. Explain the relationship between the exterior angle and the two nonadjacent interior angles.

Answer:

Given an exterior angle of a triangle measures 32°.

We can say that the sum of the measures of the two nonadjacent interior angles is also 32°.

Since we know that the measure of an exterior angle of a triangle is equal to the sum of the measures of the two nonadjacent interior angles.

If an exterior angle of a triangle measures 32°. We can say that the sum of the measures of the two nonadjacent interior angles is also 32°.

 

Page 327  Exercise 11  Problem 17

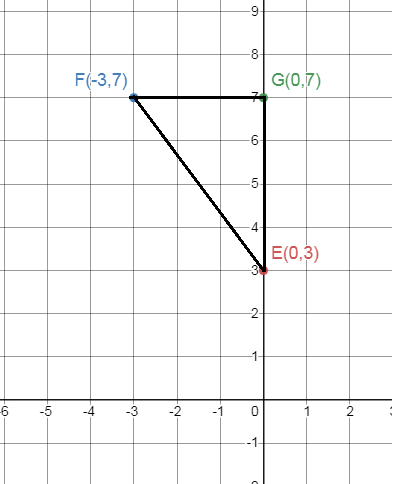
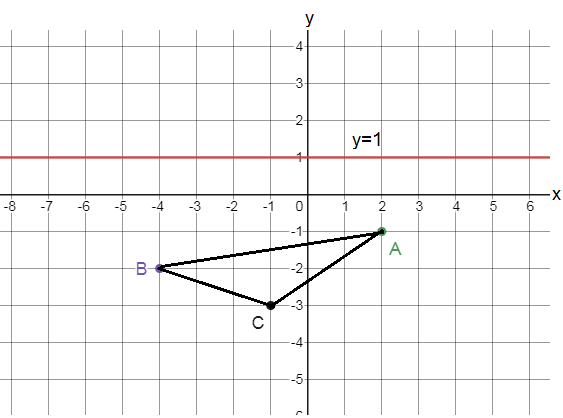
Question 17.

Given tat GE = GF in ΔGEF:

1. Determine the type of triangle ΔGEF based on the given side lengths.
2. Explain why ΔGEF is classified as this type of triangle.

Answer:

From diagram, we have GE = GF in ΔGEF.

So it is an Isosceles triangle. 

Since GE = GF so ΔGEF is an Isosceles triangle. 

 

Page 327  Exercise 12  Problem 18

Question 18.

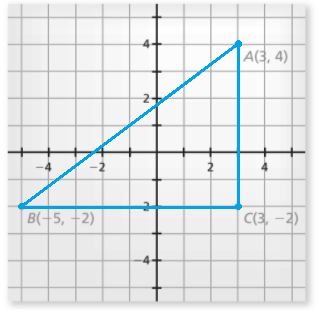
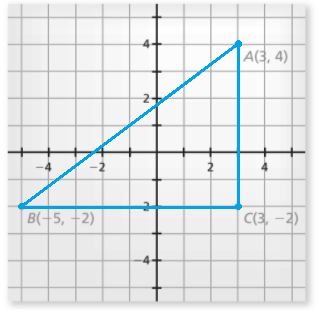
Given a ΔABC with vertices A(6,6), B(9,3) and C(2,2)

1. Find the lengths of AB, BC, and CA using the distance formula.
2. Examine these lengths to determine if they form a Pythagorean triplet.

Answer:

Given a ΔABC where A(6,6), B(9,3), C(2,2).

Find length of AB, BC, CA using distance formula.

Now examine these length for Pythagorean triplet.

We have A(6,6), B(9,3), C(2,2).

AB = \(\sqrt{(9−6)^2+(3−6)^2}\)

AB = \(\sqrt{3^2+(-3)^2}\)

AB = \(\sqrt{18}\)

BC = \(\sqrt{(2-9)^2+(2-3)^2}\)

BC = \(\sqrt{(−7)^2+(−1)^2}\)

BC = \(\sqrt{50}\)

CA = \(\sqrt{(6-2)^2+(6-2)^2}\)

CA = \(\sqrt{(4)^2+(4)^2}\)

CA = \(\sqrt{32}\)

Now examining AB, BC, CA for Pythagorean triplet.

BC² = 50

AB² = 18

CA² = 32

So

50 = 18 + 32

BC² = AB² + CA²

Hence ΔABC is a right triangle.

ΔABC is a scalene right triangle as it satisfy the relation BC² = AB² + CA².

 

Page 327  Exercise 13  Problem 19

Question 19.

Given the exterior angle of a triangle is (8x – 5)° and the two nonadjacent interior angles are 40° and (5x + 15)°:

1. Use the property of exterior angles to find the value of x.
2. Determine the measure of the exterior angle.

Answer:

To find the exterior angle

The Exterior angle is (8x−5)°

The two interior angles are 40°,(5x+15)°

According to the property, sum of opposite interior angles = Exterior angle

So, 40 + (5x + 15) = (8x − 5)

​⇒ 5x + 55 = 8x − 5

⇒ 5x − 8x = −5 − 55
​
⇒− 3x = −60

⇒ x = 20

So the exterior angle is (8x−5)° = (8(20)−5)°

(8x−5)°  = (160 − 5)°

= 155°

The exterior angle is 155°.

 

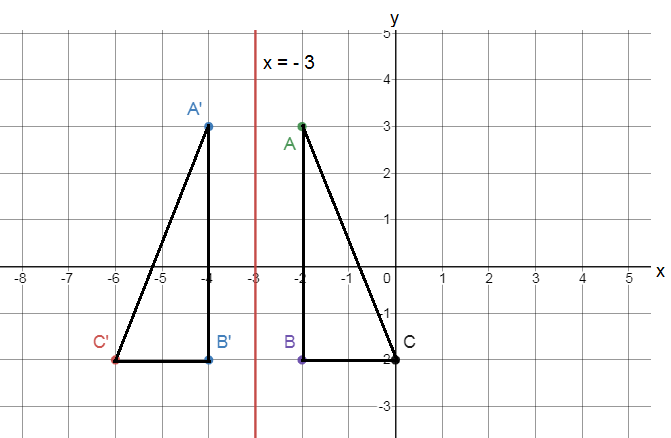
Page 328  Exercise 14  Problem 20

Question 20.

1. Explain what a rigid motion is.
2. Explain why the image of a triangle is always congruent to the original triangle under rigid motion.
3. Describe the three types of rigid motions and how each affects a figure.

Answer:

At first we explain which one is rigid motion.

Then we explain why the image of a triangle always congruent to the original triangle under rigid motion.

A translation moves every point of a diagram the same distance in the same direction.

More specifically, a translation maps, or moves, the points P and Q of a plane diagram along a vector ⟨a,b⟩ to the points P′ and Q′ so that P′ P = Q′ Q and P′ P∥Q′ Q or P′ P and Q′ mQ are co-linear.

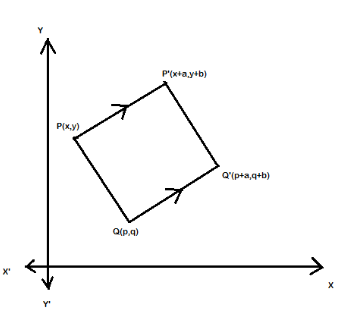
In translation the object is moved but the size and shape remains same.

A reflection is a transformation that uses a line like a mirror to reflect a diagram.

The mirror line is called the line of reflection.

A reflection in a line m maps every point P in the plane to a point P′

So that for each point on of the following properties is true.

If P is not on m , then m is the perpendicular bisector of P′ P or.

If P is on m then P = P′

 

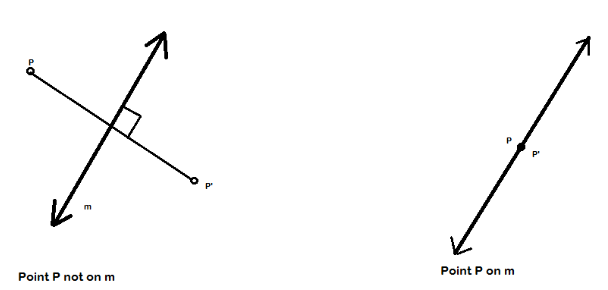
In reflection the object is moved but the size and shape remains same.

A rotation is a transformation in which a figure is turned about a fixed point called the center of rotation .

Rays drawn from the center of rotation to a point and its image form the angle of rotation.

A rotation about a point P through an angle x° maps every point Q in the plane to a point Q′, so that one of the following properties is true.

If Q is not the center of rotation p then QP = Q′P and ∠QPQ′ = x°

If Qis the center of rotation P then

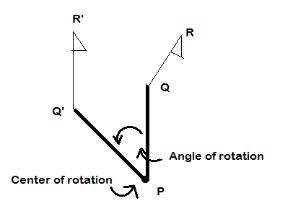
In rotation the object is moved but the size and shape remains same.

All of three transformation is rigid motion.

Under a rigid motion , the shape and size of the triangle remains same ,only the object is moved.

So the length of sides and the measurements angles of the triangle remain same.

So the image of the triangle is congruent to the original.

All the three transformation is rigid motion.

The diagram  of the triangle under rigid motion is congruent to the original.

 

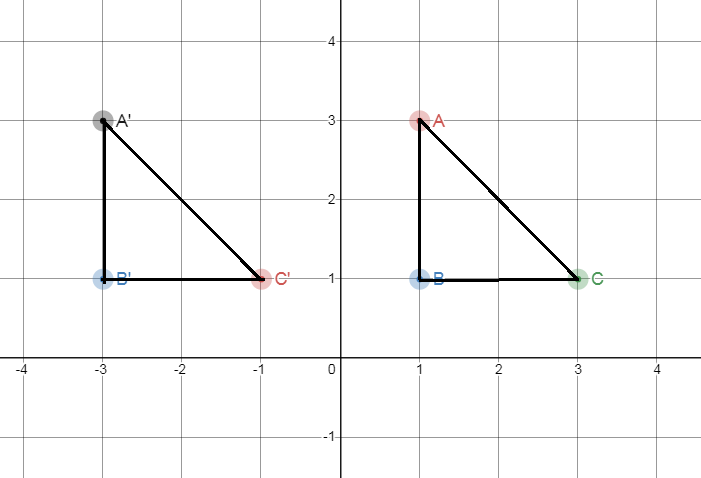
Page 329  Exercise 15  Problem 21

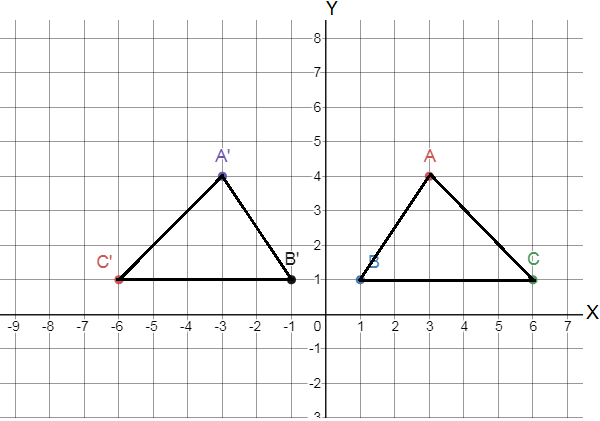
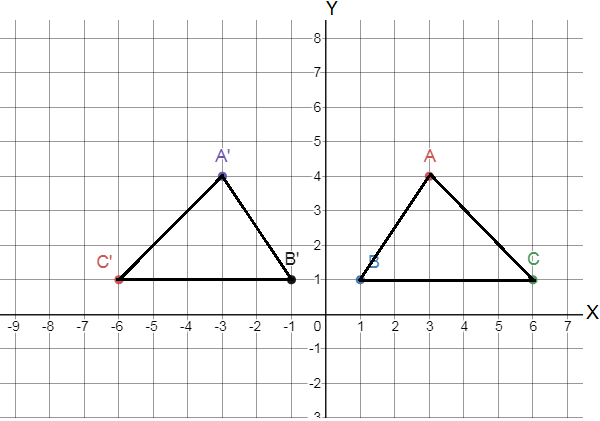
Question 21.

Given two congruent triangles ΔABC and ΔPQR, use rigid motions to map one triangle onto the other. Verify the congruence of the triangles by comparing the lengths of the sides and the measures of the included angles.

Answer:

Given:

Consider ,two congruent triangles ABC and PQR.

we need to use rigid motions to map one triangle to the other triangle.

First we need to find the sides of triangles and their included angles to draw rigid motions between them.

Now

We need to find the length of the sides of triangle ABC and PQR using the given vertices as follows:

AB = \(\sqrt{\left(x_{1-} x_2\right)^2+\left(y_1-y_2\right)^2}\)

BC = \(\sqrt{\left(x_{2-} x_3\right)^2+\left(y_2-y_3\right)^2}\)

CA = \(\sqrt{\left(x_{3-} x_1\right)^2+\left(y_3-y_1\right)^2}\)

Similarly for PQR:

PQ = \(\sqrt{\left(x_{1-} x_2\right)^2+\left(y_1-y_2\right)^2}\)

QR = \(\sqrt{\left(x_{2-} x_3\right)^2+\left(y_2-y_3\right)^2}\)

PR = \(\sqrt{\left(x_{3-} x_1\right)^2+\left(y_3-y_1\right)^2}\)

As they are congruent they have same size for sides of both the triangles.

As the sides are same then we if find the included angles ,they produce the same for both.

Hence,these both triangles are said to be congruent and they are equivalent to each other.

△ABC ≡ △PQR

So,the given two congruent triangles by using rigid motions to map one triangle to the other triangle are equivalent is given by: △ABC ≡ △PQR.

 

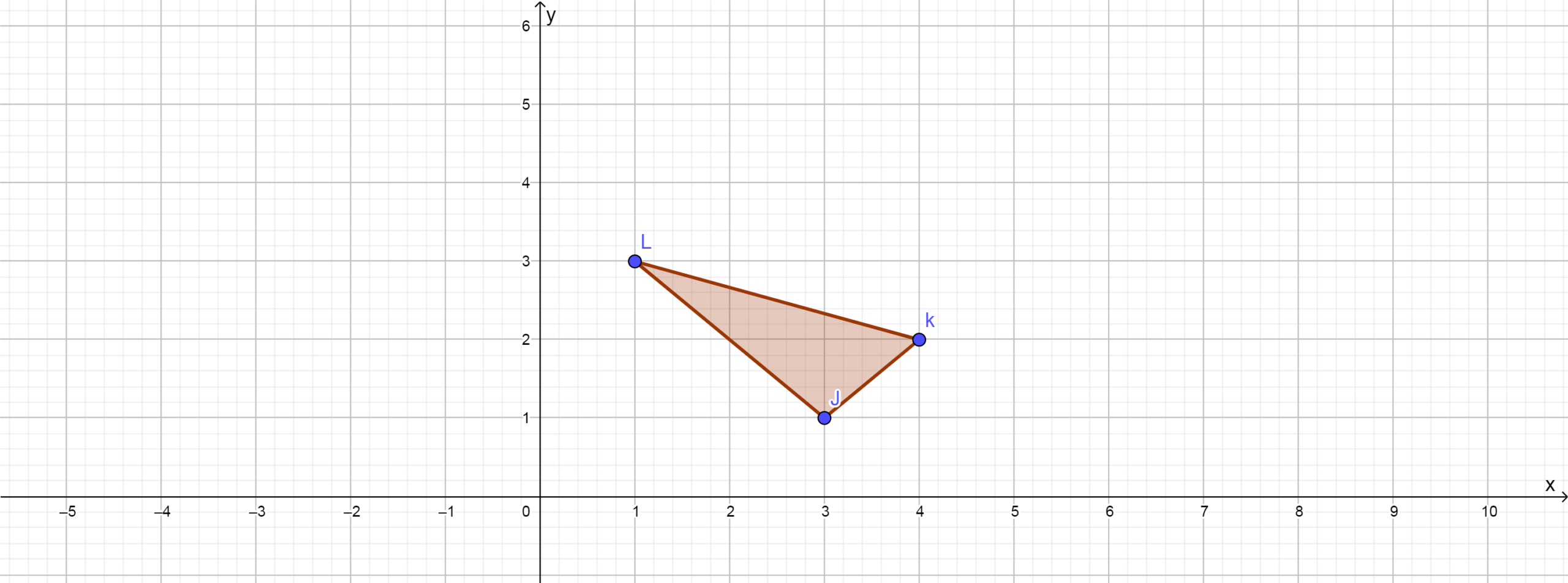
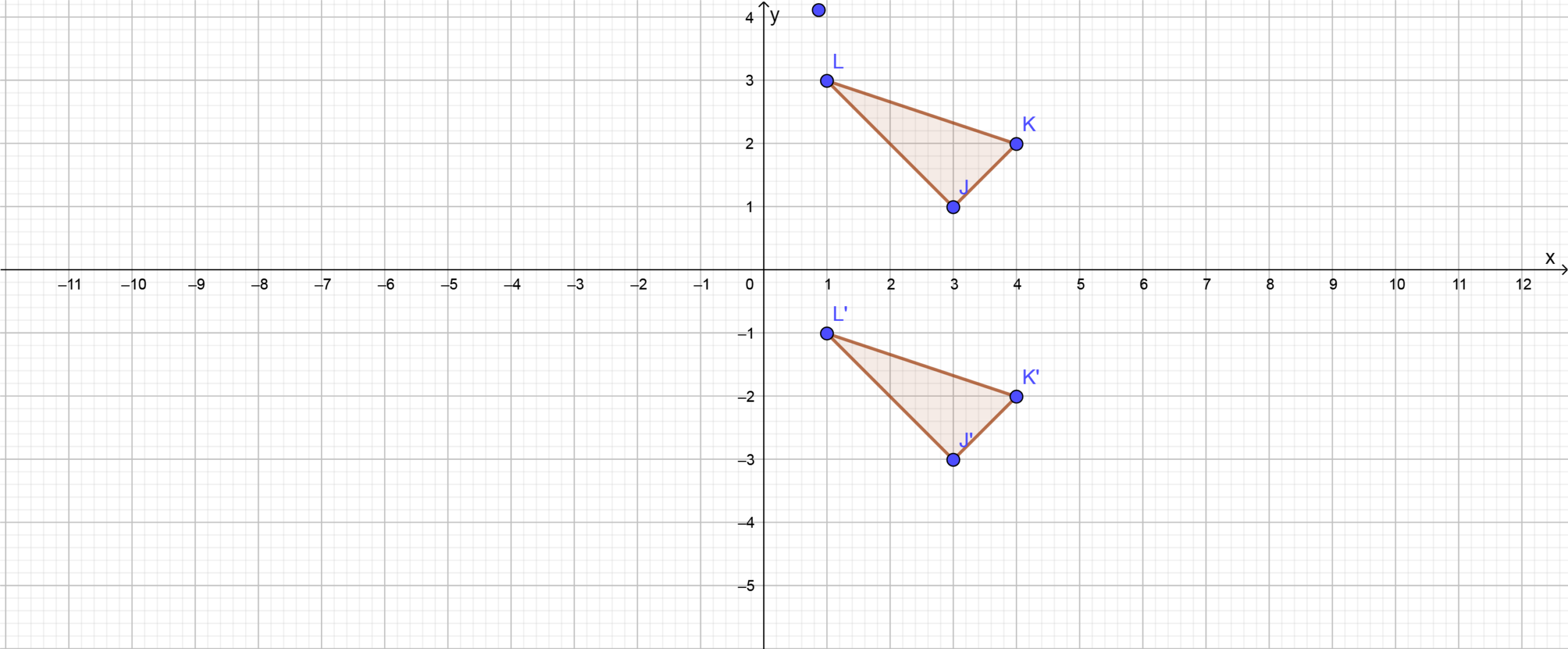
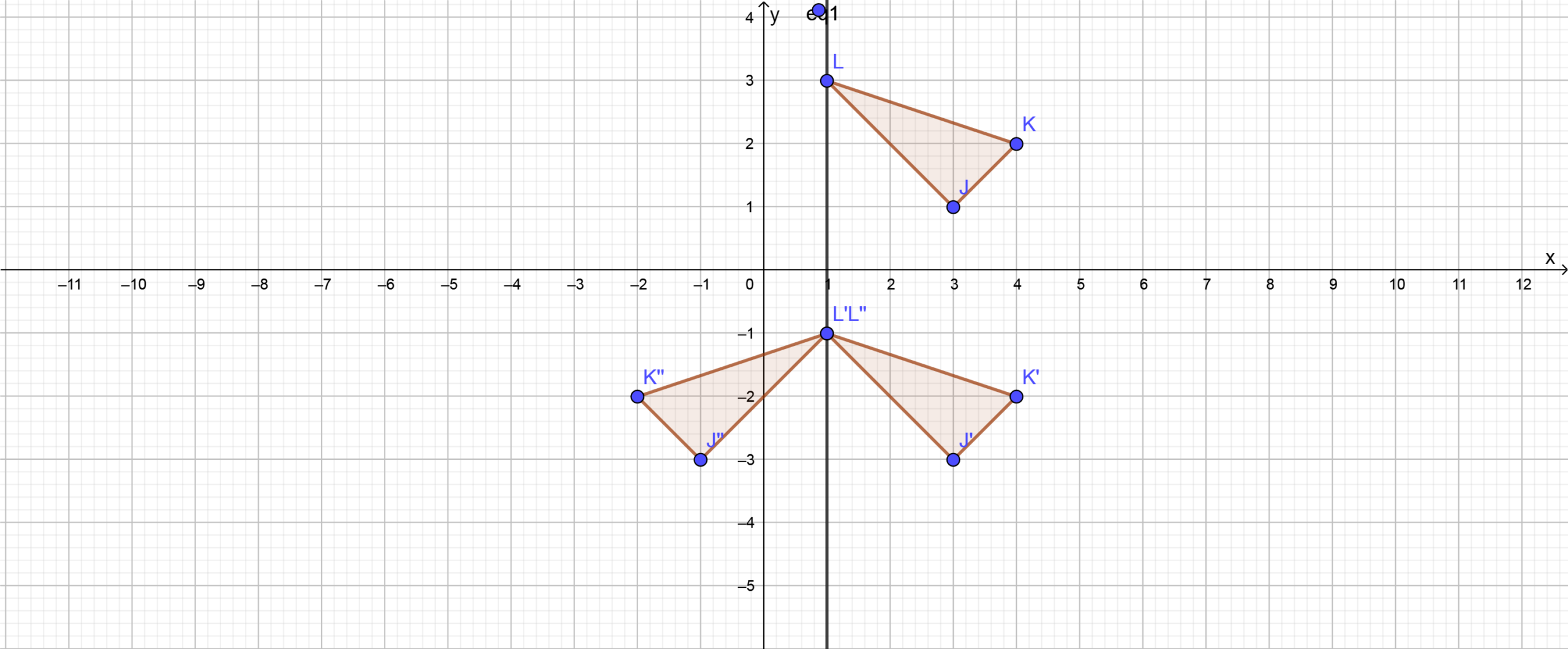
Page 329  Exercise 16  Problem 22

Question 22.

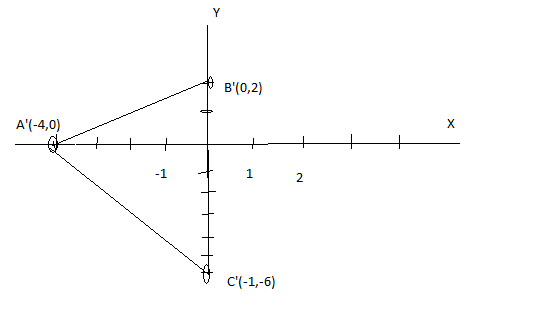
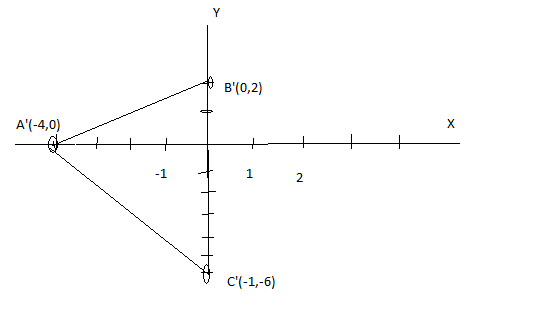
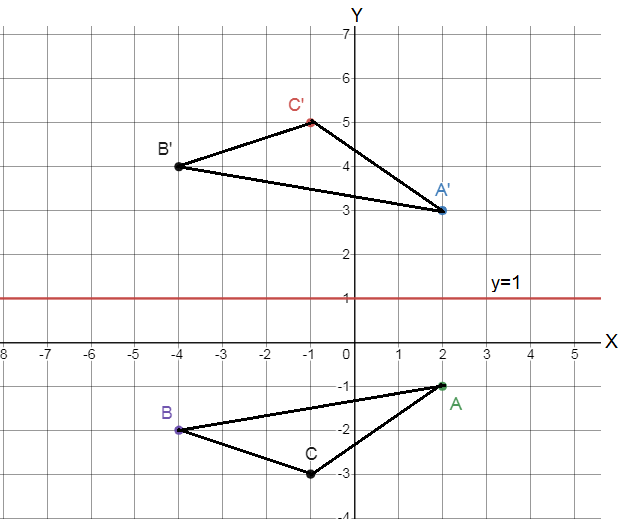
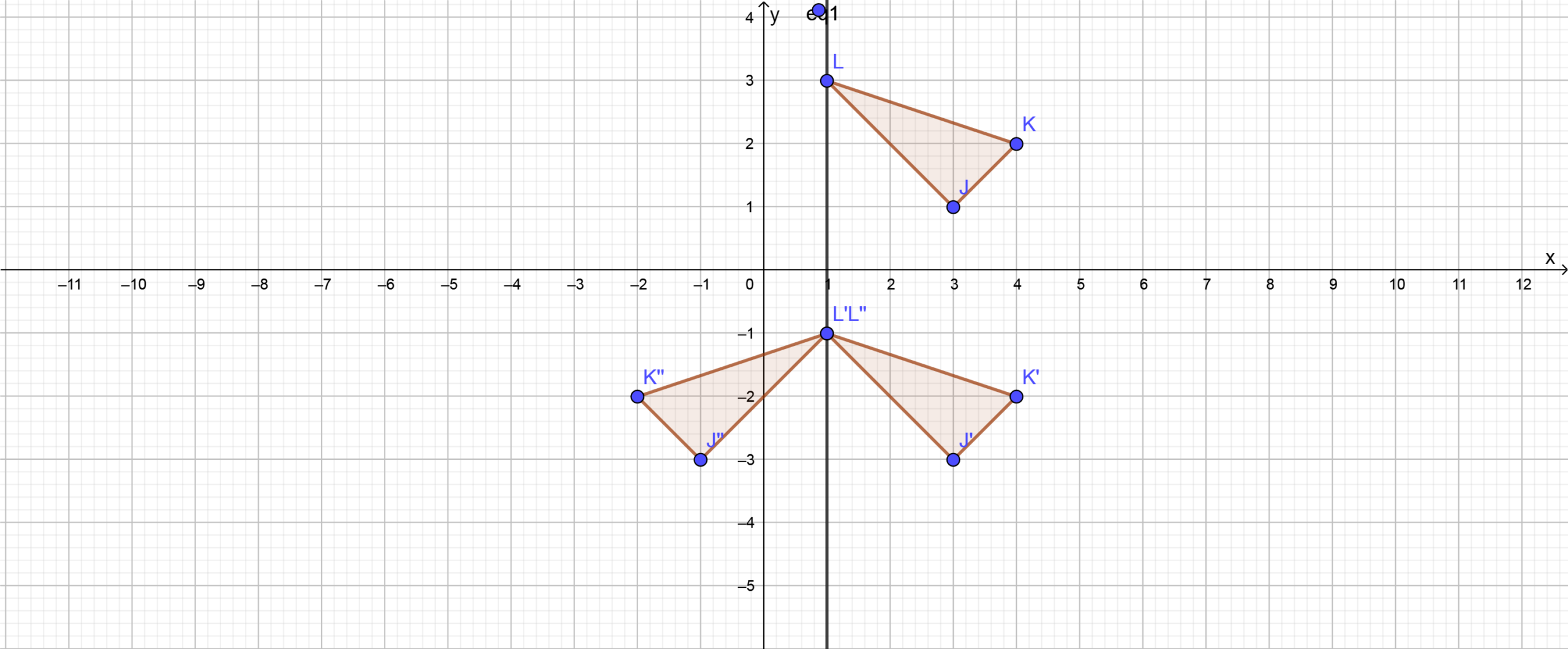
Given the vertices of ΔABC are A(1,1), B(3,2), C(4,4) and the vertices of ΔDEF are D(2,-1), E(0,0) and F(-1,2):

1. Calculate the lengths of the sides of ΔABC and ΔDEF using the distance formula.
2. Determine if the triangles ΔABC and ΔDEF are congruent.
3. Describe a composition of rigid motion that maps ΔABC onto ΔDEF.

Answer:

Given:

The vertices of ΔABC are A(1,1),B(3,2) and C(4,4).

The vertices of ΔDEF areD(2,−1),E(0,0), and  F(−1,2).

We need to describe a composition of rigid motions that maps ΔABC and ΔDEF.

Now Using the vertices of the triangleABC , we can find the sides of triangle.

AB = \(\sqrt{(1-3)^2+(1-2)^2}\)‘

= \(\sqrt{5}\)

BC = \(\sqrt{(4-3)^2+(4-2)^2}\)

= \(\sqrt{5}\)

CA = \(=\sqrt{(4-1)^2+(4-1)^2}\)

=  2 \(\sqrt{3}\)

Similarly,for triangle DEF

DE = \(\sqrt{(0-2)^2+(0+1)^2}\)

= \(\sqrt{5}\)

EF = \(\sqrt{(-1-0)^2+(2-0)^2}\)

= \(\sqrt{5}\)

DF = \(\sqrt{(2+1)^2+(-1-2)^2}\)

= 2 \(\sqrt{3}\)

So,from the above it is clear that the sides of triangle ABC and DEF are same and equal.

Hence,△ABC ≡ △DEF.

So,from the given vertices of triangle ABC and triangle DEF are same and equal. Hence,the triangles are said to be equivalent. △ABC ≡ △DEF

Big Ideas Math Integrated Math Chapter 11 Maintaining Mathematical Proficiency

 

Page 301  Exercise 1  Problem 1

Question 1.

A diagram is given. Determine whether the given shaded diagram is a translation, reflection, or rotation of the non-shaded diagram.

Steps:

1. Observe the given diagram with shaded and non-shaded parts.
2. Draw a line between the shaded and non-shaded diagrams to help with the visualization.
3. Analyze the relationship between the shaded and non-shaded diagrams.

Given Diagram:

• A diagram with a shaded and a non-shaded part.

Answer:

A diagram is given 

We have to find whether the given shaded diagram is a translation, reflection, or rotation of the non-shaded diagram.

First, draw a line between shaded and non-shaded diagrams.

The given diagram is

From the above diagram, we can see that the shaded diagram is flipped along the non-shaded diagram.

Hence, the given shaded diagram is a reflection of the non-shaded diagram.

Hence, the given shaded diagram is a reflection of the non-shaded diagram.

 

Page 301  Exercise 2  Problem 2

Question 2.

A diagram is given. Determine whether the given shaded diagram is a translation, reflection, or rotation of the non-shaded diagram.

Steps:

1. Observe the given diagram with shaded and non-shaded parts.
2. Analyze the relationship between the shaded and non-shaded diagrams to determine the type of transformation.

Given Diagram:

• A diagram with a shaded and a non-shaded part.

Answer:

A diagram is given 

We have to find whether the given shaded diagram is a translation, reflection, or rotation of the non-shaded diagram.

The given diagram is

From the above diagram, we can see that the shaded diagram is a clockwise rotation of the non-shaded diagram.

Hence, the given shaded diagram is a rotation of the non-shaded diagram.

Hence, the given shaded figure is a rotation of the non-shaded diagram.

 

Page 301  Exercise 3  Problem 3

Question 3.

Given the vertices of a polygon at E(0,3), F(-3, 7), and G(0,7), find the perimeter and area of the polygon.

Steps:

1. Mark the given vertices on the coordinate plane.
2. Join the vertices E, F, and G to form the polygon ΔEFG.
3. Calculate the lengths of the sides EF, FG, and GE using the distance formula.
4. Determine the area of the polygon.
5. Calculate the perimeter of the polygon.

Given Coordinates:

• E(0,3)
• F(-3,7)
• G(0,7)

Answer:

Given:

The vertices are  E(0,3), F(−3,7), G(0,7)

We have to find the perimeter and area of the polygon with given vertices.

Mark the given vertices on the coordinate plane.

Join the EF,FG and GE.

Find the area and perimeter.

Mark the given vertices on the coordinate plane.

And join the vertices EF,FG and GE.

Since the given polygon makes a right-angle triangle.

Base = FG

Height = GE

Find the sides of the triangle EF ,FG and GE by using the distance formula

Since E(0,3) and F(−3,7)

EF = \(\sqrt{(-3-0)^2+(7-3)^2}\)

EF = \(\sqrt{(-3)^2+(4)^2}\)

EF = \(\sqrt{9+16}\)

EF = \(\sqrt{25}\)

EF = 5 units

Similarly, Since F(−3,7) and G(0,7)

FG = \(\sqrt{(0-(-3))^2+(7-7)^2}\)

FG = \(\sqrt{(3)^2}\)

FG = \(\sqrt{9}\)

FG = 3 units

Similarly, Since G(0,7) and E(0,3)

GE = \(\sqrt{(0-0)^2+(3-7)^2}\)

GE = \(\sqrt{-4}^2\)

GE = \(\sqrt{16}\)

GE = 4 units

Find the area of the right △EFG

Area of right △EFG = \(\frac{1}{2}\) × Base × Height

Area of right △EFG = \(\frac{1}{2}\) × FG × GE

Area of right △EFG = \(\frac{1}{2}\) × 3 × 4

Area of right △EFG = 1 × 3 × 2

Area of right △EFG = 6 square units

Find the perimeter of △EFG

Perimeter of △EFG = EF + FG + GE

Perimeter of △EFG = 5 + 3 + 4

Perimeter of △EFG = 12 units

Hence, the area and perimeter of the polygon whose vertices are E(0,3),F(−3,7),G(0,7) is 6 square units and 12 units.

 

Page 302  Exercise 4  Problem 4

Question 4.

Using dynamic geometry software, draw and label a triangle ΔABC with the following vertices:

1. Select the geometry tool in the software.
2. Input the vertices of ΔABC into the software.
3. Label the triangle as shown in the diagram below.

Vertices:

• A(x₁, y₁)
• B(x₂, y₂)
• C(x₃, y₃)

Answer:

Here, we draw a △ABC using dynamic geometry software

Select geometry in the software.

Input the vertices of the △ABC in the software.Label the triangle

Hence, by using dynamic geometry software we draw a △ABC as shown below.

 

Page 302  Exercise 4  Problem 5

Question 5.

Given triangle ΔABC, translate the triangle to form ΔA’B’C’ with the following vertex transformation:

• A(1, 3) → A'(-3, 3)
• B(1, 1) → B'(-3, 1)
• C(3, 1) → C'(-1, 1)

Answer:

Given

Triangle ΔABC, translate the triangle to form ΔA’B’C.

Since we draw a △ABC in Page 302 Exercise 4 Problem 4.

Now, translate the △ABC as △A′ B′ C′ by A(1,3)→ A′ (−3,3),B(1,1)→ B′ (−3,1) and C(3,1)→C′ (−1,1)

Hence, the translated triangle of △ABC is △A′ B′ C′ shown below

 

Page 302  Exercise 4  Problem 6

Question 6.

Given the coordinates of the vertices of ΔABC and the translated triangle ΔA’B’C’ determine the relationship between the coordinates of the vertices of ΔABC and those of ΔA’B’C’.

Given Coordinates:

• A (−1, 2)
• B (3, 2)
• C (2, −1)

Translated Coordinates:

• A’ (3, 3)
• B ‘(7, 3)
• C’ (6, 0)

Answer:

Given

The coordinates of the vertices of ΔABC and the translated triangle ΔA’B’C’ are

A (−1, 2)

B (3, 2)

C (2, −1)

A’ (3, 3)

B ‘(7, 3)

C’ (6, 0)

We write coordinates of A,B,C and A′ ,B′,C′

We write about the coordinates of vertices of both triangles.

We write the relationship between the coordinates of the vertices of ΔABC and that of ΔA′ B′C′.

We write coordinates of vertices of given triangles

A = (−1,2)

B = (3,2)

C = (2,−1)

A′= (3,3)

B′= (7,3)

C′= (6,0)

Adding 4 units to x−coordinate of A,B,C and adding 1 unit to y-coordinate of A,B,C

We get coordinates of A′ ,B′,C′

When we add 4 units to x-coordinates of A,B,C add 1 unit to y coordinates of A,B,C , nwe get coordinates of A′ B′ C′.

 

Page 302  Exercise 5  Problem 7

Question 7.

Given that a point (x,y) is translated a and b units vertically, write a rule to describe the coordinates of the image of (x,y) after the translation.

Given:

• A point (x,y) is translated a units horizontally.
• The same point (x,y) is also translated b units vertically.

Answer:

Given that (x,y) is translated a units horizontally and b units vertically.

We write coordinates of diagram  of (x,y)

we write rule to describe coordinates of image of (x,y)

Given that (x,y)is translated a units horizontally and b units vertically.

Coordinates of digram   of (x,y) is (x − a,y − b)

Thus we write a rule to describe coordinates of  diagram  of (x,y)

(x,y) → (x − a,y − b)

We write rule to describe the coordinates of image of (x,y)  , (x,y)→(x − a,y − b)

 

Page 302  Exercise 5  Problem 8

Question 8.

Given the coordinates of ΔABC and the translation rule, find the coordinates of the translated triangle ΔA’B’C’.

Given Coordinates:

• A = (0,3)
• B = (4,5)
• C = (3,-3)

Translation:

• The triangle ΔABC is translated as 4 units left(horizontally) and 3 units down(vertically).

Answer:

We write coordinates of A,B,C

Given that ΔABC is translated 4 units left (horizontally) and 3 units down(vertically) as ΔA′ B′C′ we write rule to translate (x,y)

Thus we write coordinates of A′ ,B′ ,C′

Coordinates of A = (0,3) B = (4,5) C = (3,−3) from diagram ΔABC is translated 4b units left and 3 units down.

We use rule in last example as (x,y) → (x − 4, y − 3)

Here a = 4 b = 3

A′ = (0 − 4, 3 − 3)

A′ = (−4,0)

B′ = (4 − 4,5 − 3)

B′ = (0,2)

C′ = (3 − 4,−3 − 3)

C′ = (−1,−6)

Given A = (0,3)

B = (4,5)

C = (3,−3)

Rule used in last example is (x,y)→(x − 4, y − 3)

Here a = 4

b = 3

ΔABC is translated into Δ A′B′C′

A′ =(−4,0), B′ = (0,2) ,C′ = (−1,−6)

 

Page 302  Exercise 5  Problem 9

Question 9.

Given the coordinates of ΔABC and its transformed ΔA’B’C’, use the distance formula to verify that the side lengths of ΔABC and ΔA’B’C’ are equal.

Given coordinates:

• A = (0,3)
• A = (4,5)
• A = (3,-3)

Transformed Coordinates:

• A’ = (-4,0)
• B’ = (0,2)
• C’ = (-1,-6)

Answer:

ΔA′B′C′ is as shown in diagram

We write coordinates of A,B,C

We calculate AB,BC,AC using distance formula.

We write coordinates of A′ ,B′ ,C′

Now we calculate A′ B′ ,B′ C′ ,A′ C′ using distance formula

We write coordinates of

A =(0,3)

B = (4,5)

C = (3,-3)

AB = \(\sqrt{(0-4)^2+(3-5)^2}\)

= \(\sqrt{20}\)

BC = \(\sqrt{(4-3)^2+(5+3)^2}\)

= \(\sqrt{65}\)

AC =\(\sqrt{(0-3)^2+(3+3)^2}\)

= \(\sqrt{45}\)

We write coordinates of A′ ,B′,C′now

A’ = (-4,0)

B’ = (0,2)

C’ = (−1,−6)

We calculate A′B′= \(\sqrt{(-4-0)^2+(5-3)^2}\)

= \(\sqrt{20}\)

B’C’ = \(\sqrt{(0+1)^2+(2+6)^2}\)

= \(\sqrt{65}\)

A’C’ = \(\sqrt{(-4+1)^2+(0+6)^2}\)

= \(\sqrt{45}\)

Thus AB = A’B’

BC = B’C’

AC = A’c’

Thus side lengths of Δ A′B′C′ is same as that of ΔABC

Δ A′B′C′ is as shown in above digram. Side lengths AB = A′B′,BC = B′, AC = A′C′

 

Page 305  Exercise 6  Problem 10

Question 10.

Given a vector in standard position on a coordinate plane, find the components of the vector from the graph.

Given Vector:

• The vector starts at the origin (0,0) and moves in the downward direction.

Answer:

Given

A vector in standard position on a coordinate plane,

The given vector is

Now, find the components of the vector from the graph.

Since, the vectors standard position has its starting point in origin and vector is moving in downward direction. Therefore

So, the changes in x – axis and  y – axis is < −5 − 0, −1 − 0>​.

Therefore, the name of the vector \(\overline{B A}\)  with its component form as <−5,−1>.

Hence, the vector is \(\overline{B A}\) with components as <−5,−1>.

 

Page 306  Exercise 7  Problem 11

Question 11.

Given the vertices of triangle ΔABC with vertices A(1,2), and B(5,1), translate the triangle using the vector (0,-5).

Answer:

Given

The vertices of triangle ΔABC with vertices A(1,2), and B(5,1)

It is given that the vertices of triangle ABC having vertices A(1,2), B(5,1) and C(5,4)

Now, translate the triangle ABC using the vector <0,−5>.

Use <0 ,−5 > to move each vertex 5 units down

Label the image vertices as A’B’C’ and also vectors drawn from pre-image vertices to image vertices are parallel.

Hence, the graph of triangle ABC and its image A’B’C’ using the given vector <0,− 5> is

 

Page 306  Exercise 8  Problem 12

Question 12.

Given the quadrilateral PQRS (pre-image) and its image P’Q’R’S’, use the rule of translation for the given to find the translation vector and the corresponding rule.

Answer:

The given Quadrilateral PQRS (pre-image) and image P’Q’R’S’ is

Use the rule of translation for the given diagram.

In the diagram draw vector components form and apply the translation rule such that 

It can be seen that the diagram of quadrilateral moves 1 unit towards right and 3 units down.

So, (x,y) = (x + 1,y − 3)

Hence, the rule of translation is along the vector <1, -3> with notation is (x,y) = (x + 1,y − 3)

 

Page 306  Exercise 9  Problem 13

Question 13.

Given a translation (x,y) → (x + 6, y-3) and the vector (4,5), find the image of the point J(4,5) after applying the translation.

Answer:

The given translation is (x,y) = (x + 6, y − 3)and the given vector is (4,5).

Use the rule mentioned above to solve this.

So, the digram of <4,5> from (x,y) = (x + 6,y − 3)is

(x,y) = (4 + 6,5 − 3)

(x,y) = (10,2)

Hence, the image of J(4,5) is J′(10,2).

 

Page 306  Exercise 10  Problem 14

Question 14.

Given the translation rule (x,y) → (x + 6, y-3) and the vector R'(0,-5), find the image point of R'(0,-5) after applying the translation.

Answer:

The given translation is (x,y) = (x + 6, y − 3) and the given vector is R′(0,−5).

Use the rule mentioned above to solve this

So, the  digram  of <4,5> from (x,y) = (x + 6,y − 3)

(x,y) = (0 + 6,− 5 − 3)

(x,y) = (6,−8)

Hence, the image of  R′ (0,−5)  is  R(6,−8).

 

Page 306  Exercise 11  Problem 15

Question 15.

Given the coordinate plane diagram, perform the following moves for a spaceship and rewrite the given composition as a single transformation.

Moves:

1. Move the spaceship 1 unit left and 4 units up.
2. Move the spaceship to 2 units left.

Answer:

Given: The Coordinate plane diagram

First move: Move the spaceship 1 unit left and 4 units up.

Second move: Move the spaceship to 2 units left.

We have to rewrite the given composition as a single transformation.

Since the first move of the spaceship is 1 unit left and 4 units up.

Which can be written as (−1,4)

Since the first move of the spaceship is 2 units left.

Which can be written as (−2,0)

Combine both the composition

(−1+−2, 4 + 0) = (−3,4)

Hence, the final composition for the given spaceship is

(x,y) → (x − 3, y + 4)

Hence, in a video game, we move given spaceship 1 unit left and 4units up. Then, you move the spaceship to 2 units left. Then the composition can be written as a single transformation such that (x,y) → (x − 3, y + 4).

 

Page 307  Exercise 12  Problem 16

Question 16.

A diagram can be reflected in a coordinate plane in two ways: over the x-axis or over the y-axis. Given a point on the diagram, apply the appropriate reflection rules to find its image.

Answer:

A diagram can be reflected in a coordinate plane in two ways:

1. Reflect over the x−axis

2. Reflect over the y− axis.

1. Reflect over the x-axis:

When the diagram is reflecting over the x−axis.

In this case, the axis of reflection is the x−axis.

Rule: In reflection of the digram across x−axis, change the sign of the coordinate value of y, and leave the coordinate value of x
same.

Such that (x,y) → (x,−y)

2. Reflect over the y-axis:

When the diagram is reflecting over the y− axis, the axis of reflection is the y−axis.

Rule: In reflection of the figure across the y−axis, change the sign of the coordinate value of x and leave the coordinate value of ysame, such that(x,y)→(−x,y)

A diagramcan be reflected over the x−axis and over the y−axis.

Rules to reflect over x−axis: Change the sign of y coordinate value such that  (x,y) → (x,−y)  Rules to reflect over  y−axis: Change the sign of x coordinate value such that (x,y) → (−x,y).

 

Page 308  Exercise 13  Problem 17

Question 17.

Using dynamic geometry software, draw the triangle ΔABC with the given coordinates and then reflect it to cover the y-axis to obtain ΔA’B’C’.

Given Coordinates:

• A(3,4)
• B(1,1)
• C(6,1)

Answer:

Draw a △ABC using software.

Let say the coordinates are A(3,4), B(1,1) and C(6,1).

Now, to draw the reflection, change the sign of the xcoordinate value such that (3,4) → (−3,4) ,(1,1) → (−1,1)and (6,1) → (−6,1).

Name the new triangle as △A′B′C′. 

Hence, the reflection of ΔABCin the y-axis to △A′B′C′ by using a dynamic geometry software is shown below

 

Page 308  Exercise 13  Problem 18

Question 18.

Given ΔABC and its reflection ΔA’B’C’ over the y-axis, verify that the corresponding sides and angles of ΔABC and ΔA’B’C’ are congruent.

Given Coordinates:

• A(3,4)
• B(1,1)
• C(6,1)

Reflected Coordinates:

• A'(-3,4)
• B'(-1,1)
• C'(-6,1)

Answer:

Given

ΔABC and its reflection ΔA’B’C’ over the y-axis,

Since, △A′B′C′is the mirror image or reflection of △ABC

So, the side of the triangles are congruent such that

AB = A′B′

BC = B′C′

CA = C′A′

That means the corresponding sides of the △A′B′C′and △ABC are equal.

Also, the corresponding angles of the triangle

△A′B′C′and △ABC are equal such that

∠ABC = ∠A′B′C′

∠BCA = ∠B′C′A′

∠BAC = ∠B′A′C′

The corresponding also side lengths and angle measures of the  △ABC  and  △A′B′C′ are equal .

 

Page 308  Exercise 14  Problem 19

Question 19.

Given a point on a coordinate plane, reflect it over the x-axis and the y-axis using the appropriate reflection rules.

Answer:

A diagram can be reflected in a coordinate plane in two ways:

1. Reflect over the x−axis

2. Reflect over the y−axis

1. Reflect over the x-axis:

When the diagram is reflecting over the x−axis.

In this case, the axis of reflection is the x−axis.

Rule: In reflection of the digram  across the x−axis, change the sign of the coordinate value of yand leave the coordinate value of x, such that(x,y)→(x,−y).

2. Reflect over the y-axis:

When the diagram  is reflecting over the y−axis, the axis of reflection is the y−axis.

Rule: In reflection of the figure across the y−axis, change the sign of the coordinate value of xand leave the coordinate value of y,such that (x,y) → (−x,y).

A diagram can be reflected over thex−axis and over the y−axis. Rules to reflect over x−axis: Change the sign of y coordinate value such that (x,y)→(x,−y).Rules to reflect over y−axis: Change the sign of x coordinate value such the (x,y)→(−x,y)

 

Page 310  Exercise 15  Problem 20

Question 20.

Given the coordinates of the vertices of ΔABC:

• A(-1,5)
• B(-4,4)
• C(-3,1)

Graph ΔABC and its reflection ΔA’B’C’ in the y-axis.

Answer:

Given:

The coordinates of the vertices of △ABC.

A(−1,5),B(−4,4),C(−3,1)

We have to graph △ABC and its reflection digram in the y−axis.

To draw the reflection image in the y−axis use (x,y)→(−x,y)

Plot the given vertices of △ABCwhich are A(−1,5),B(−4,4),C(−3,1) on the Coordinate plane.

Join the AB,BC and CA.

Now, find the reflection of △ABC named it △A′B′C′

Since the reflection in the y−axis.

So, change the sign of x value of the given coordinates such that A(−1,5) → A′(1,5), B(−4,4) → B′(4,4) and C(−3,1) → C′(3,1).

Now, plot the coordinates A′(1,5),B′(4,4),C′(3,1)on the Same coordinate plane.

Join A′B′,B′C′and C′A′.

Hence, the graph of ΔABCwhose vertices coordinates are A(−1,5),B(−4,4),C(−3,1)and its diagram after reflection in they−axis is

 

Page 310  Exercise 16  Problem 21

Question 21.

Given the coordinates of the vertices of ΔABC:

• A(0,2)
• B(4,5)
• C(5,2)

Graph ΔABC and its reflection ΔA’B’C’ in the x-axis.

Answer:

Given:

The coordinates of the vertices of △ABC A(0,2),B(4,5),C(5,2)

We have to graph △ABCand its reflection image in the x− axis.

To draw the reflection image in the x−axis use (x,y) → (x,−y)

Plot the given vertices of △ABCwhich are A(0,2),B(4,5),C(5,2)on the coordinate plane.

Join AB, BC and CA.

Now, find the reflection of △ABC and named it △A′B′C′

Since the reflection in the x−axis.

So, change the sign of y value of the given coordinates such that A(0,2)→A′(0,−2),B(4,5)→B′(4,−5),C(5,2)→C′(5,−2)

Now, plot the coordinates A′(0,−2),B′(4,−5),C′(5,−2)on the Same coordinate plane.

Join A′B′,B′C′ and C′A′.

Hence, the graph of △ABC whose vertices coordinates are A(0,2),B(4,5),C(5,2) and its diagram after reflection in the x−axis is

 

Page 310  Exercise 17  Problem 22

Question 22.

Given the coordinates of the vertices of ΔABC:

• A(2,-1)
• B(-4,-2)
• C(-1,-3)

Graph ΔABC and its reflection image ΔA’B’C’ across the line y = 1.

Answer:

Given:

The coordinates of the vertices of △ABC A(2,−1),B(−4,−2),C(−1,−3)

We have to graph △ABC and its  diagram after a reflection in the given line y = 1.

First, draw the line y = 1 in the coordinate plane to find the reflection diagram.

Plot the given vertices A(2,−1),B(−4,−2),C(−1,−3) of △ABC on the coordinate plane.

Join AB,BC and CA.

And, draw the line y = 1 on the graph.

Now draw the reflection of △ABC

Since, y = 1 is the reflection line

Hence, the graph of △ABC whose vertices coordinates are A(2,−1),B(−4,−2),C(−1,−3) and its diagram after reflection in the y = 1 is 

 

Page 310  Exercise 18  Problem 23

Question 23.

Given the coordinates of the vertices of ΔABC:

• A(-2,3)
• B(-2,-2)
• C(0,-2)

Graph ΔABC and its reflection image ΔA’B’C’ across the line x = -3.

Answer:

Given: The coordinates of the vertices of △ABC

A(−2,3),B(−2,−2),C(0,−2)

We have to graph △ABC and its diagram  after a reflection in the given line x = −3 .

First, plot the given coordinates on the coordinate plane and draw the line x = −3.

Plot the given vertices A(−2,3),B(−2,−2),C(0,−2) of △ABC on the coordinate plane.

Join AB,BC and CA.

And, draw the line x = −3 on the graph.

Now draw the reflection of △ABC  and named it △A′B′C′

Since, x = −3 is the reflection line

Hence, the graph of △ABCwhose vertices coordinates are A(−2,3),B(−2,−2),C(0,−2)and its image after reflection in the x = −3 is

 

Page 311  Exercise 19  Problem 24

Question 24.

Graph ΔJKL with vertices J(3,1), K(4,2), and L(1,3) and its image after a glide reflection. The glide reflection consists of a translation followed by a reflection.

Steps:

Translation: (x,y) → (x,y-4)

Reflection: Across the line x = 1

Answer:

Given

In this question, we have to graph ΔJKL with vertices J(3, 1), K(4, 2), and L(1, 3) and its diagram after the glide reflection.

Translation: (x,y)→(x,y−4)

Reflection: In the line x = 1

First we draw the graph of ΔJKL

The graph of ΔJKL with vertices J(3, 1), K(4, 2), and L(1, 3) is

Now we have to draw digram of ΔJKL after the glide reflection

Translation:(x,y) → (x,y − 4)

We have J = (3,1)now we put the coordinates of J in the given translation to find J′

(x,y) → (x,y − 4)

(x,y)→(3,1 − 4)

(x,y)→(3,−3)

K = (4,2) now we put the coordinates of K in the given translation to find K′

(x,y) → (x,y − 4)

(x,y) → (4,2 − 4)

(x,y)→ (4,−2)

L = (1,3) now we put the coordinates of L in the given translation to find L′

(x,y) → (x, y − 4)

(x,y)→ (1, 3 − 4)

(x,y) → (1,−1)

Now we graph the △J′K′L′ with vertices J′(3,−3),K′(4,−2),L′(1,−1)

Now we apply reflection in the line x = 1

We know that if(a,b)is reflected in the line x = 1 then its diagram  is the point (−a,b) so the poin t J′′ which is the reflection of J′ is  J′′(−3,−3)  the point K′′ which is the reflection of K′ is K′′(−4,−2)t he point  L′′ which is the reflection of L′is L′′(−1,−1)

The graph of ΔJKL with vertices J(3, 1), K(4, 2), and L(1, 3) and its digram after the glide reflection.

Translation: (x,y) → (x, y − 4)

Reflection: In the line x = 1

The diagram of triangle JKL after translation is triangle J′K′L′

After reflection the diagram  of triangle J′K′L′is triangle J′′K′′L′′

The graph contains the triangle JKL with vertices J(3,1), K (4,2), and L(1,3) and the diagram of triangle J′K′L′ with vertices J′(3,−3), K′(4,−2), L′(1,−1) and triangle J′′K′′L′′ with vertices J′′(−3,−3),K′′(−4,−2),L′′(−1,−1).

 

Page 311  Exercise 20  Problem 25

Question 25.

Identify the line of symmetry of the word “WOW”.

Answer:

In this question have to identify the line of symmetry of the word WOW.

As from the definition of the line of symmetry:

The line of symmetry can be defined as the axis or imaginary line that passes through the center of the shape or object and divides it into identical halves.

As from the diagram given below we conclude that line of symmetry of the word WOW is possible because we get two identical halves.

The line of symmetry of the word WOW is

 

Page 311  Exercise 21  Problem 26

Question 26.

Identify the line of symmetry of the word “KID”.

Answer:

In this question have to identify the line of symmetry of the word KID

As from the definition of the line of symmetry:

The line of symmetry can be defined as the axis or imaginary line that passes through the center of the shape or object and divides it into identical halves.

As from the diagram given below we conclude that line of symmetry of the word KID is possible because we get two identical halves.

The line of symmetry of the word KID is

Page 311  Exercise 22  Problem 27

Question 27.

Given that power strips are placed along wall w, which connects two computers: Computer A and Computer B. Determine where to place the power strip to minimize the length of the connecting cables.

Answer:

Given that power strip are placed along wall w which connects to two computers that is Computer A −and Computer B.

We need to determine that where to place the power strip to minimize the length of the connecting cables.

Here, wis the power strip line.

Now, draw the reflection of Computer A at point A.

Then, join point A to Computer B and the line intercept wat point B and it is the nearest distance to minimize the length of the connecting cables.

Place at equal distance from the both the computers that at point B to minimize the length of the connecting cables.

Big Ideas Math Integrated Math Chapter 9 Maintaining Mathematical Proficiency

 

246  Exercise 1  Problem 1

Question 1.

Given the arithmetic sequence 22, 34, 46, 58, …

1. Derive the formula for the nth term of the sequence.
2. Use the formula to find the 20th term of the sequence.

Answer:

Given

The arithmetic sequence 22, 34, 46, 58, …

To find the nth term and 20th term of the sequence 22,34,46,58 …………..

Here, the sequence is  22,34,46,58 ………..

​⇒ a = 22

⇒  d = 34 − 22

= 12
​
The nth term of the sequence is a_n

=  a + (n−1)d

​⇒  a_n =  22 +(n−1)12

⇒ a_n = 22 + 12n−12

⇒ a_n = 12n + 10

​The 20^th  term is a₂₀

= 12(20) + 10

​a₂₀ = 240 + 10

a₂₀ = 250

​The n^th term of the sequence is  a_n= 12n + 10  and  a₂₀ = 250.

 

Page 246  Exercise 2  Problem 2

Question 2.

Given the arithmetic sequence −13, 0, 13, 26, …

1. Derive the formula for the nth term of the sequence.
2. Use the formula to find the 20th term of the sequence.

Answer:

Given

The arithmetic sequence −13, 0, 13, 26, …

To find the n^th term and a₂₀

Here, the sequence is −13,0,13,26….

​⇒  a = −13

⇒  d = 0−(−13)

= 13

d = 13

​The nth term of the sequence is :

a_n  = a + (n−1)d

​⇒  a_n  = −13 + (n−1)13

⇒  a_n = −13 + 13n − 13

⇒  a_n  = 13n− 26
​
The ​20^th term is a₂₀

=  13(20) − 26

​⇒  260 − 26

⇒  234
​
The nth term  of the sequence  is  a_n=  13n−26  and  a₂₀ = 234

 

Page 246   Exercise 3  Problem 3

Question 3.

Given the arithmetic sequence −4.5, −4.0, −3.5, −3.0, …

1. Derive the formula for the nth term of the sequence.
2. Use the formula to find the 20th term of the sequence.

Answer:

Given

The arithmetic sequence −4.5, −4.0, −3.5, −3.0, …

To find n^th term and a₂₀

Here, the sequence is −4.5,−4.0,−3.5,−3.0, ………..

a = − 4.5

​d = −4.0 − (−4.5)

d = 0.5
​
The n^th  term of the sequence an

=  a + (n−1)d

​⇒  a_n =−4.5 + (n−1)(0.5)

⇒  a_n =−4.5 + 0.5n − 0.5

⇒  a_n = 0.5n − 5.0
​
The 20^th term of the sequence is a₂₀

=  0.5(20) − 5.0

​⇒  a₂₀ = 10.0 − 5.0

⇒  a₂₀ = 5.0
​
The nth ^th of the sequence is a_n = 0.5n − 5.0 and  a₂₀ = 5.0

 

Page 246  Exercise 4 Problem 4

Question 4.

Given the arithmetic sequence −12, 12, 32, 52, …

1. Derive the formula for the nth term of the sequence.
2. Use the formula to find the 20th term of the sequence.

Answer:

Given

The arithmetic sequence −12, 12, 32, 52, …

To find the n^th term of the sequence and a₂₀ term of the sequence.

Here, the sequence is \(\frac{-1}{2}, \frac{1}{2}, \frac{3}{2}, \frac{5}{2}\)

a = \(\frac{−1}{2}\)

d = \(\frac{1}{2}\) − (\(\frac{−1}{2}\))

d = 1

The n^th term of the sequence is an = a + (n−1)d

​⇒  a_n = \(\frac{1}{2}\)+ (n−1)1

⇒  a_n = \(\frac{1}{2}\) + n − 1

⇒  a_n  = n−\(\frac{3}{2}\)

The 20^th term of the sequence is a₂₀

= 20− \(\frac{3}{2}\)

⇒  \(\frac{37}{2}\)

The n^th  term of the sequence is a_n = n− \(\frac{3}{2}\) and  a₂₀ = \(\frac{37}{2}\)

 

Page 246  Exercise 5  Problem 5

Question 5.

Solve for x in the equation: 3x − 9 = 12.

Answer:

Given

Solve for x: 3x − 9 = 12

The equation is  3x − 9 = 12

​⇒  3x = 12 + 9

⇒  3x = 21

⇒  x = 7
​
The value for x = 7

 

Page 246  Exercise 6  Problem 6

Question 6.

Solve for y in the equation: 16 − 4y = 40.

Answer:

Given:  16−4y = 40

To find –  The value of y.

Calculate the value y

The equation is 16 − 4y = 40

⇒ −4y = 40 − 16

⇒ −4y = 4

⇒  y = −6

The solution of the equation 16−4y = 40 is y = −6.

 

Page 246  Exercise 7  Problem 7

Question 7.

Solve for y in the equation: 6z + 4 = 23.

Answer:

Given: 6z + 5 = 23

Put all the terms without z

On the right side to find the value of z.

Given the equation:

6z + 5 = 23

⇒ 6z = 23

⇒  z =  \(\frac{18}{6}\)

⇒  z = 3

The value of z = 3.

 

Page 246  Exercise 8  Problem 8

Question 8.

Solve for q in the equation: 15 = 11q – 23.

Answer:

Given: 15 = 11q − 18.

Put all the terms without q.

On the right side to find the value of q

Given the equation:

​15 = 11q − 18

−11q = −18 − 15

−11q = −33

11q = 33

q = \(\frac{33}{11}\)

q = 3
​
The value of q = 3.

 

Page 246   Exercise 9  Problem 9

Question 9.

Solve for r in the equation: 6r + 3 = 33.

Answer:

Given:  6r + 3 = 33.

Put all the terms without r.

On the right side to find the value of r.

Given the equation:

​6r + 3 = 33

6r = 33 − 3

6r = 30

r = \(\frac{30}{6}\)

r = 5
​
The value of r = 5.

 

Page 246  Exercise 10  Problem 10

Question 10.

Solve for s in the equation: 27 = 4s – 9.

Answer:

Given: 27 = 4s−9.

Put all the terms without s.

On the right side to find the value of s.

Given the equation:

27 = 4s − 9

−4s = −9 − 27

−4s = −36

4s = 36

s = \(\frac{36}{4}\)

s = 9

The value of s = 9.

 

Page 247  Exercise 11  Problem 11

Question 11.

Consider the conditional statement: “If a student studies hard, then the student will pass the exam.”

1. Identify the hypothesis and the conclusion in the given conditional statement.
2. Using symbols, represent the conditional statement.
3. Construct a truth table for the conditional statement p → q, where p represents “the student studies hard” and q represents “the student will pass the exam”.
4. Explain under what condition the conditional statement p → q is false.

Answer:

When a conditional statement is written in if-then form, the “if” part contains the hypothesis and the “then” part contains the conclusion.

Words If p, then q. Symbols p → q (read as “p implies q”)

A conditional statement is symbolized by

p → q it is an if-then statement in which p is a hypothesis and q is conclusion. The logical connector in a conditional statement is denoted by the symbol →.

The conditional is defined to be true unless a true hypothesis leads to a false conclusion.

A truth table for p → q is shown below.

A conditional statement is only false when the hypothesis (p) is true and the conclusion (q) is false, otherwise it is true.

 

Page 248  Exercise 12  Problem 12

Question 12.

Given the points A = (3,0), B = (4,0), and D = (0,0):

1. Show that ADC is a right triangle by identifying the right angle.
2. Calculate the lengths of DA and DC using the distance formula.
3. Using the Pythagorean Theorem, verify that AC is the hypotenuse of the right triangle ΔADC.
4. Prove that the Pythagorean Theorem holds for ΔADC by showing that AC² = AD² + DC².

Answer:

Every right angle satisfy the Pythagoras theorem ΔADC is an right angle triangle.

So, it holds Pythagoras theorem.

ΔADC is an right angle triangle

A = (3,0) , B = (4,0) , D = (0,0)

DA = 3

DC = 4

Therefore by distance formula

AC = \(\sqrt{(4-0)^2+(0-3)^2}\)

AC = \(\sqrt{16+9}\)

AC = 5

Now 5² = 3² + 4²

Therefore, AC² =  AD² + DC²

It is the statement of “Pythagoras” theorem

It implies ΔADC holds Pythagoras theorem.

 

The given statement “If ΔADC  is a right triangle, then the Pythagorean Theorem is valid for ΔADC ” is true.

 

Page 248  Exercise 12  Problem 13

Question 13.

Consider the statement: ” If ∠A and ∠B are complementary, then the sum of their measures is 180°.”

1. Define what it means for two angles to be complementary.
2. Using the definition, explain why the given statement is false.
3. Provide a correct statement about the sum of the measures of complementary angles.
4. Given that ∠A = 35°, find the measure of ∠B if A and ∠B are complementary.

Answer:

We will use the definition of complementary angle to show that given statement is false.

∠A and ∠B are complementary.

Therefore, ∠A+∠B = 90°

It implies sum of their angle measures is not equal to 180°

The given statement ” If ∠A and ∠B are complementary, then the sum of their measures is 180° “is false.

 

Page 248  Exercise 12  Problem 14

Question 14.

Consider the statement: “If figure ABCD is a quadrilateral, then the sum of its angle measures is 180°.”

1. Explain why the given statement is false.
2. State the correct sum of the interior angles of a quadrilateral.
3. Using the angle sum property of a quadrilateral, calculate the sum of the interior angles.
4. Verify your result by using the formula 180° ×(n−2), where n is the number of sides in the quadrilateral.

Answer:

Given statement is

If figure ABCD is a quadrilateral, then the sum of its angle measures is 180°

We will use the angle sum property of a quadrilateral

A quadrilateral has four sides. sum of their angle measure is 180∘ (n−2) where n is number of sides.

A Quadrilateral has four sides.

Therefore, sum of their angles = 180°(n−2) where n is number of sides

= 180° (4−2) 

= 360°

So, sum of their angle measure is 360°

Given statement ” if   ABCD is a quadrilateral, then the sum of its angle measures is 180°” is false.

 

Page 248  Exercise 12  Problem 15

Question 15.

Consider the statement: “If points A, B, and C are collinear, then they lie on the same line.”

1. Explain why the given statement is true.
2. Using the slope property of a line, demonstrate how to check if points A, B, and C are collinear.
3. Given the coordinates of points A(1,2), B(3,6), and C(5,10), use the slope property to verify if these points are collinear.

Answer:

Given statement is

If points A, B, and C are “Collinear”, then they lie on the same line.

Using the slope property of a line we will check whether the points are colinear.

As point A , B , C are Collinear

Slope of AB =

Slope of BC

= Slope of AC

= m

It implies they all lie on the same line.

The given statement If points A, B, and C are collinear, then they lie on the same line” is true.

 

Page 248  Exercise 13  Problem 16

Question 16.

Consider the definition of a conditional statement: “A conditional statement holds true when the result of the first statement implies the other statement. In other words, they either have the same meaning or are properties.”

1. Explain when a conditional statement is true.
2. Explain when a conditional statement is false.
3. Provide an example of a true conditional statement and justify why it is true.
4. Provide an example of a false conditional statement and justify why it is false.

Answer:

A conditional statement holds true when the result of the first statement implies the other statement.

In other words, they either have the same meaning or are properties.

To show conditional statement to be true.

We need to satisfy both statements with each other.

Conditional statement will be false when first statement does not imply second.

A conditional statement can be true or false.

 

Page 248  Exercise 14  Problem 17

Question 17.

Consider the following conditional statements:

1. “If a rectangle does not have 4 sides, then a square is not a quadrilateral.”
2. “If 9 is composite, then 8 is an odd number.”
3. Identify the hypothesis and conclusion in each conditional statement.
4. Explain why the first conditional statement is true.
5. Explain why the second conditional statement is false.
6. Provide an additional example of a true conditional statement and justify why it is true.
7. Provide an additional example of a false conditional statement and justify why it is false.

Answer:

True conditional statement:

If a rectangle does not have 4 sides, then a square is not a quadrilateral.

Hypothesis- A rectangle does not have 4 sides.

Conclusion- A square is not a quadrilateral.

∵   Both hypothesis and conclusion statement are false so the conditional statement is true.

False conditional statement:

If 9 is composite, then 8 is an odd number.

Hypothesis- 9 is composite.

Conclusion- 8 is an odd number.

∵  Hypothesis is true and conclusion is false so the conditional statement is false.

The final answer is that the: True conditional statement is “If a rectangle does not have 4 sides, then a square is not a quadrilateral. “False conditional statement is “If 9 is composite, then 8 is an odd number.”

 

Page 252  Exercise 15  Problem 18

Question 18.

Given the conditional statement: “13x – 5 = -18, because x = -1.”

1. Rewrite the given conditional statement in if-then form.
2. Solve the equation 13x − 5 = −18 to verify the value of x.

Answer:

Given: A conditional statement 13x−5 = −18 , because x = −1.

To find – Rewrite the given conditional statement in if-then form.

Required if – Then form of given conditional statement is

“If 13x−5 = 18 , then x = −1.”

The final answer is that the required if-then statement of given conditional statement is“If 13x−5 = −18 , then x = −1 .”

 

Page 252  Exercise 16  Problem 19

Question 19.

Given the conditional statement: “The sum of the measures of interior angles of a triangle is 180°.”

1. Rewrite the given conditional statement in if-then form.
2. Explain why the rewritten statement is logically equivalent to the original statement.

Answer:

Given: A conditional statement “The sum of the measures of interior angles of a triangle is 180°.”

To find – Rewrite the given conditional statement in if-then form.

Required if- Then the form of given conditional statement is-

“If a shape is a triangle, then the sum of the measures of its interior angles is 180°.”

The final answer is that the required if-then statement of given conditional statement is “If a shape is a triangle, then the sum of the measures of its interior angles is 180°.”

 

Page 252 Exercise 17  Problem 20

Question 20.

A diagram and the statement “LM bisects JK” were given.

1. Let p be the statement “Given diagram” and q be the statement “LM bisects JK.”
2. Explain how you can determine from the diagram that LM bisects JK.
3. Verify whether the given statement q is true based on the diagram.
4. If the diagram shows that JR bisects RK, explain how this information supports the truth of the statement that LM bisects JK.

Answer:

Given: A diagram and a statement \(\overline{L M}\) bisects \(\overline{J K}\).

To find – Whether the given statement is true about the given diagram

Let p  Given diagram and q  \(\overline{L M}\) bisects \(\overline{J K}\)are two statements.

From the diagram we can say that \(\overline{L M}\)  bisects \(\overline{J K}\) because \(\overline{J R}\) bisects \(\overline{R K}\)

⇒  If the diagram is true then \(\overline{J K}\) because \(\overline{J R}\) bisects \(\overline{R K}\).

⇒ The given statement is true about the given diagram.

The final answer is that the given statement  \(\overline{J R}\)  bisects \(\overline{R K}\)  is true about the given diagram.

 

Page 252  Exercise 18  Problem 21

Question 21.

Given a diagram and the statement “∠JRP and ∠PRL are complementary.”

1. Let p be the statement “Given diagram” and q be the statement “∠JRP and ∠PRL are complementary.”
2. Use the diagram to verify whether ∠JRP + ∠PRL = 90°.
3. Explain why the angles ∠JRP and ∠PRL are complementary based on the diagram.
4. Conclude whether the given statement q is true about the diagram.

Answer:

Given: A diagram and a statement ∠JRP and ∠PRL are complementary.

To find – whether the given statement is true about the given diagram.

Let p  Given diagram  and q ∠JRP and ∠PRL are complementary” are two statements

∵ ∠JRP + ∠PRL = 90° (From the diagram)

⇒   ∠JRP and ∠PRL are complementary angles.

⇒   If the given diagram is true then∠JRP  and ∠PRL are complementary angles.

⇒  The given statement is true about the given diagram.

The final answer is that the given statement ∠JRP and ∠PRL are complementary angles is true about the given diagram.

 

Page 252  Exercise 19  Problem 22

Question 22.

A diagram and the statement “∠MRQ ≅ ∠PRL” were given.

1. Let p be the statement “Given diagram” and q be the statement “∠MRQ ≅ ∠PRL.”
2. Explain the relationship between ∠MRQ and ∠PRL using the properties of the diagram.
3. Verify whether ∠MRQ and ∠PRL are congruent based on the diagram.
4. Conclude whether the given statement q is true about the diagram.

Answer:

Given: A diagram and a statement ∠MRQ ≅ ∠PRL.

To find – whether the given statement is true about the given diagram.

Let p :  Given diagram and q  ∠MRQ ≅ ∠PRL  are two statements.

∵ ∠MRQ and ∠PRL are vertically opposite angles.

⇒ ∠MRQ ≅ ∠PRL.

⇒  If the given diagram is true then∠MRQ ≅ ∠PRL.

⇒  The given statement is true about the given diagram.

The final answer is that the given statement  ∠MRQ ≅ ∠PRL is true about the given diagram.

 

Page 253  Exercise 20  Problem 23

Question 23.

Consider the various uses of reasoning to solve problems as described below:

1. Reasoning is used to find a property that a series of steps shows.
2. Reasoning is used to find a general rule for a number of specific observations.
3. Reasoning is used so that factual statements can come to a logical conclusion.
4. Reasoning is also used to find a pattern in a series of observations.
5. Explain how reasoning can be used to find a property that a series of steps shows. Provide an example.
6. Describe how reasoning can help find a general rule from specific observations. Provide an example.
7. Explain how reasoning can lead factual statements to a logical conclusion. Provide an example.
8. Describe how reasoning is used to find patterns in a series of observations. Provide an example.

Answer:

Uses of reasoning to solve problems:

Reasoning is used to find a property that a series of steps shows.

Reasoning is used to find a general rule for a number of specific observations.

Reasoning is used to factual statements can come to a logical conclusion.

Reasoning is also used to find a pattern in a series of observations.

The final answer is that the uses of reasoning to solve a problem is:

Reasoning is used to find a property that a series of steps shows.

Reasoning is used to find a general rule for a number of specific observations.

Reasoning is used to factual statements can come to a logical conclusion.

Reasoning is also used to find a pattern in a series of observations.

 

Page 253  Exercise 21  Problem 24

Question 24.

Given a pattern shown in a diagram series:

1. Identify and describe the pattern in the sequence of diagrams.
2. Make a conjecture about the pattern based on your observations.
3. Determine which diagram will be the 10th object in the sequence.
4. Draw the 10th object based on your conjecture.

Answer:

Given: A pattern shown in a Diagram.

To find –  A conjecture about the pattern and draw the 10th object.

We can see that the Diagrams included here are repeating after every three Diagrams.

First Diagram is

Then second Diagram is

And the third Diagram is

And it keeps on repeating.

⇒  The conjecture about the pattern is that the circle is rotating from one vertex of triangle to the next vertex in clockwise direction.

⇒  10th object will be the 1st object only as difference between 10 and 1 is 9 which is a multiple of 3.

⇒  10th object is

The final answer is that the conjecture about the pattern is that the circle is rotating from one vertex of triangle to the next vertex in clockwise direction. and its 10th object is

 

Page 253  Exercise 21  Problem 25

Question 25.

Draw a pattern shown in a series of coordinate plane diagrams:

1. Identify and describe the pattern in the sequence of diagrams.
2. Make a conjecture about the pattern based on your observations.
3. Determine which diagram will be the 10th object in the sequence.
4. Draw the 10th object based on your conjecture.

Answer:

Given:  A pattern shown in a Diagram.

To find –  A conjecture about the pattern and draw the 10th object.

We can see that the Diagrams included here shows a pattern.

All the Diagrams include a 2D coordinate plane.

In first Diagram, there is a curve which is concave down in 1st quadrant.

In second Diagram, there is a straight line in 4th quadrant.

In third Diagram, there is a curve which is concave down in 3rd quadrant.

In fourth Diagram, there is a straight line in 2nd quadrant.

⇒  At even places there are straight lines in even quadrant (first 4th and then 2nd ).

At odd places there are curves which are concave up and concave down in odd quadrant (first two concave down and then two concave up).

⇒ The conjecture about the pattern is that there is a curve in odd quadrant that is repeating after every four Diagram and there is a line in even quadrant that is repeating after every two Diagram .

Place will be a line and 10 is 5th odd number so it will be same as 2nd object.

⇒  Object at the 10th place is

The final answer is that the conjecture about the pattern is that there is a curve in odd quadrant that is repeating after every four Diagrams and there is a line in even quadrant that is repeating after every two  digram  and the 10th object is

 

 Page 254  Exercise 21  Problem 26

Question 26.

Draw a pattern shown in a series of diagrams:

1. Identify and describe the pattern in the sequence of diagrams.
2. Make a conjecture about the pattern based on your observations.
3. Determine which diagram will be the 10th object in the sequence.
4. Draw the 10th object based on your conjecture.

Answer:

Given:  A pattern shown in a diagram.

 

To find – A conjecture about the pattern and draw the 10th object.

We can see from the series of diagram that there is an arrangement in the first three diagram and next three are the mirror images of the first three and then it is repeating as the first six images.

⇒  The conjecture about the pattern is that there are first three arrangements and next three are mirror images of first three and then it is repeating as first six arrangements.

⇒  10th object will be same as the 4th object which is mirror image of 1st object.

⇒  10th object is

The final answer is that the conjecture about the pattern is that there are first three arrangements and next three are mirror images of first three and then it is repeating as first six arrangements.

 

Page 254   Exercise 22  Problem 27 

Question 27.

Provide an example of how you used reasoning to solve a real-life problem. Use the following scenario as a basis:

You want to go for a drive outside, but it is freezing out.

1. Describe the initial situation and any relevant facts or observations.
2. Analyze the situation using reasoning to identify potential risks or concerns.
3. Formulate a conclusion based on your analysis.
4. Support your conclusion with additional evidence or reasoning.

Answer:

Here it is asked to give an example of how you used reasoning to solve a real-life problem.

Suppose that the current situation is that you want to go for a drive outside and it is freezing out.

So, first, we need to analyze the situation, that is for your safety on road the fact that freezing is to be taken care of.

So we can form a conclusion that since it’s freezing it will be dangerous on the road.

Finally, we can support our conclusion by looking into the number of accidents on the road due to freezing.

Therefore, an example of how to use reasoning to solve a real-life problem is shown.

Big Ideas Math Integrated Math Chapter 10 Maintaining Mathematical Proficiency

 

Page 274  Exercise 1  Problem 1

Question 1.

Given the following angle measurements in a diagram:

$\mathrm{\angle }𝐴𝐵𝐷=(5𝑥+2{)}^{\circ }$ $\mathrm{\angle }𝐷𝐵𝐶=(2𝑥+3{)}^{°}$

1. Find the values of ∠ABD and ∠DBC.
   $\mathrm{<span\; style="font-size:\; inherit;\; font-family:\; -apple-system,\; system-ui,\; BlinkMacSystemFont,\; \text{'}Segoe\; UI\text{'},\; Helvetica,\; Arial,\; sans-serif,\; \text{'}Apple\; Color\; Emoji\text{'},\; \text{'}Segoe\; UI\; Emoji\text{'},\; \text{'}Segoe\; UI\; Symbol\text{'};\; text-transform:\; none;"> \; <span\; style="font-size:\; inherit;"> </span></span>}$ 
2. Show all steps clearly, including the calculation of the unknown variable 𝑥.

Answer:

Given diagram

Given that

$\mathrm{\angle }𝐴𝐵𝐷=(5𝑥+2{)}^{\circ }$ $\mathrm{\angle }𝐷𝐵𝐶=(2𝑥+3{)}^{°}$

Here

∠ABD = (5x + 2)°

∠DBC = (2x + 3)°

We have to find the angle ∠ABD and ∠DBC

The total sum of angle on one side of a line is 1800.

Thus we add both the angles formed by this BD line on the AC line and equate them with 1800 to find both the angles and by extension the unknown variable.

In this question, we have a line BD meeting a point B on the line AC.

As it meets the line, the sum of both the angles must be the angle that is naturally formed on one side of the line which is 1800.

Thus

​∡ABD + ∡DBC = 180

(5x + 2) + (2x + 3) = 180

5x + 2x + 2 + 3 = 180

7x + 5 = 180

7x = 175

x = 25

​Thus we can calculate both angles.

​∡ABD = 5x + 2

∡ABD = (5 × 25) + 2

∡ABD = 125 + 2

∡ABD = 127
​
Now rather than calculating the angle DBC, we can just subtract this found angle from 1800

So that we can find the supplementary angle DBC.

​∡DBC = 180 − ∡ABD

∡DBC = 180 − 127

∡DBC = 53
​
Both the angles for the given diagram can be written as ∡ABD = 1270 and ∡DBC = 530.

 

Page 274  Exercise 2  Problem 2

Question 2. 

Given the following angle measurements in a right-angle shape EFG with a line FH meeting point F:

$\mathrm{\angle }𝐸𝐹𝐻=(11𝑥+1{)}^{\circ }$ $\mathrm{\angle }𝐻𝐹𝐺=(2𝑥-2{)}^{\circ }$

1. Find the values of ∠𝐸𝐹𝐻 and ∠HFG.
   $\mathrm{<span\; style="font-family:\; -apple-system,\; system-ui,\; BlinkMacSystemFont,\; \text{'}Segoe\; UI\text{'},\; Helvetica,\; Arial,\; sans-serif,\; \text{'}Apple\; Color\; Emoji\text{'},\; \text{'}Segoe\; UI\; Emoji\text{'},\; \text{'}Segoe\; UI\; Symbol\text{'};\; font-size:\; inherit;\; text-transform:\; none;"> </span>}$ 
2. Show all steps clearly, including the calculation of the unknown variable x.

Answer:

Given

$\mathrm{\angle }𝐸𝐹𝐻=(11𝑥+1{)}^{\circ }$ $\mathrm{\angle }𝐻𝐹𝐺=(2𝑥-2{)}^{\circ }$

The total angle in a right angle or a perfect L shape is 900.

Thus we add both the angles formed by this FH line on the EFG shape and equate it with 900 to find both the angles and by extension the unknown variable.

In this question, we have a line FH meeting the point F on the shape EFG.

As it meets the shape, the sum of both the angles must be the angle that is naturally formed on this shape which is a right angle or 900.

Thus

​∡EFH + ∡HFG = 90

(11x + 1)+(2x − 2) = 90

11x + 2x + 1 − 2 = 90

13x − 1 = 90

13x = 90 + 1

x = \(\frac{91}{13}\)

x = 7
​
Thus we can calculate both angles.

​∡EFH = 11x + 1

∡EFH = (11 × 7) + 1

∡EFH = 77 + 1

∡EFH = 78
​
Now rather than calculating the angle HFG, we can just subtract this found angle from 900 so that we can find the complimentary angle HFG.

​∡HFG = 90 − ∡EFH

∡HFG = 90 − 78

∡HFG = 12
​
Both the angles for the given diagram can be written as ∡EFH = 780 and ∡HFG = 120.

 

Page 274  Exercise 3  Problem 3

Question 3. 

Given the following angle measurements in a diagram:

$\mathrm{\angle }𝐽𝐾𝑀=(2𝑥+9{)}^{\circ }$ $\mathrm{\angle }𝐿𝐾𝑀=(3𝑥+1{)}^{\circ }$

1. Find the values of ∠JKM and ∠𝐿𝐾𝑀.
2. Show all steps clearly, including the calculation of the unknown variable x.

Answer:

Given diagram

Given that

$\mathrm{\angle }𝐽𝐾𝑀=(2𝑥+9{)}^{\circ }$ $\mathrm{\angle }𝐿𝐾𝑀=(3𝑥+1{)}^{\circ }$

Here

∠JKM = (2x + 9)°

∠LKM = (3x + 1)°

We have to find out the angles ∠JKM and ∠LKM

The total sum of angle on one side of a line is 1800.

Thus we add both the angles formed by this KM line on the JL line and equate them with 1800 to find both the angles and by extension the unknown variable.

In this question, we have a line KM meeting a point K on the line JL.

As it meets the line, the sum of both the angles must be the angle that is naturally formed on one side of the line which is 1800.

Thus

​∡ JKM + ∡LKM = 1800

(2x+9)+(3x+1) = 180

2x + 3x + 9 + 1 = 180

5x + 10 = 180

5x = 180 − 10

5x = 170

x = 34
​
Thus we can calculate both angles.

​∡JKM = 2x + 9

∡JKM =(2 × 34) + 9

∡JKM = 68 + 9

∡JKM = 77
​
Now rather than calculating the angle LKM, we can just subtract this found angle from 1800 so that we can find the supplementary angle LKM.

​∡LKM = 180 − ∡JKM

∡LKM = 180 − 77

∡LKM = 1030

​Both the angles for the given diagram can be written as ∡JKM = 770 and ∡LKM = 1030

 

Page 274  Exercise 4  Problem 4

Question 4. 

Write the equation of a line that passes through the point (2, 7) and has a slope of 5.

Answer:

Given

The point (2, 7) and has a slope of 5.

When writing the equation of a line, all we need is a slope and a point through which the line passes obeying this slope.

Thus, we use the given point and the given slope to write the equation of the line.

We have the slope of the line represented by m which is 5.

We also have a point through which the line passes represented by (x1,y1) which is (2,7).

Thus we write the equation of a line using

​y − y₁ = m(x − x₁)

y − 7 = 5(x −2)

5x − 10 − y + 7 = 0

5x − y − 3 = 0

5x − y = 3
​
The line passing through point (2,7) and having slope  5 is 5x − y = 3.

 

Page 274 Exercise  5 Problem 5

Question 5. 

Write the equation of a line that passes through the point $(5,-9)$ and has a slope of \(\frac{1}{6}\)

Answer:

Given

The point $(5,-9)$ and has a slope of \(\frac{1}{6}\)

When writing the equation of a line, all we need is a slope and a point through which the line passes obeying this slope.

Thus, we use the given point and the given slope to write the equation of the line.

We have the slope of the line represented by m which is \(\frac{1}{6}\).

We also have a point through which the line passes represented by (x1,y1)which is (5,−9).

Thus we write the equation of a line using the formula y−y₁ = m(x−x₁)

As such y−y₁ = m(x−x₁)

y − (−9) = \(\frac{1}{6}\) (x−5)

6(y + 9) = x − 5

6y + 54 = x − 5

54 + 5 = x − 6y

x − 6y = 59
​
The line passing through point (5,−9)and having slope \(\frac{1}{6}\) is x − 6y = 59.

Page 274  Exercise 6  Problem 6

Question 6.

Write the equation of a line that passes through the point $(0,-8)$ and has a slope of \(\frac{3}{5}\)

Answer:

Given:

The slope of the line is m = \(\frac{3}{5}\)

The coordinate of the point is (0,−8)

We have to find an equation of the line that passes through the given point with the given slope.

We have the slope of the line represented by m which is \(\frac{3}{5}\).

We also have a point through which the line passes represented by (x1,y1) which is (0,−8).

Thus we write the equation of line using the formula y−y1= m(x−x1)

As such

​y−y₁ =  m(x−x₁)

y − (−8) = \(\frac{3}{5}\) (x − 0)

5(y + 8) = 3x

5y + 40 = 3x

3x − 5y = 40

​The line passing through point (0,−8) and having slope \(\frac{3}{5}\) is 3x − 5y = 40

 

Page 274  Exercise 7  Problem 7

Question 7. 

Write the equation of a line that passes through the point $(2,-1)$ and has a slope of 5.

Answer:

Given

The point $(2,-1)$ and has a slope of 5.

We are given a point (2,-1) and m = 5.

And we need to find the equation of a line that passes through (2,-1) and has the slope m =5.

As we know, there are different forms of equations of a line.

We will use the point-slope form of the equation of a line to find the required equation.

We are given a point (2,-1) and m = 5.

And we need to find the equation of a line that passes through (2,-1) and has the slope m =5.

As we know, there are different forms of equations of a line.

We will use the point-slope form of the equation of a line to find the required equation.

The equation of the line that passes through the point (2,-1) and has the slope m = 5 is 5x − y = 11.

 

Page 275 Exercise 8 Problem 8

Question 8. 

Explain the following types of lines and provide their definitions:

1. Parallel Lines
2. Intersecting Lines
3. Coincident Lines
4. Skew Lines

Answer:

We have to find out the meaning when two lines are parallel, intersecting, coincident, or skew.

Two lines are said to be parallel if they never intersect each other, regardless of how far they are extended on either side.

Parallel lines are at equal distances from each other and never meet.

Three sets of parallel lines are shown below:

When two lines cross each other in a plane, they are called intersecting lines. The intersecting lines share a common point.

The point where the lines intersect is called the point of intersection.

 A set of intersecting lines is shown below:

Coincide means that it occurs at the same time.

The coincident lines are lines that lie upon each other in such a way that when we look at them, they appear to be a single line.

A set of Coincident lines is shown below:

Any two lines are said to be skew lines if they do not intersect and also not parallel.

Since two lines in a plane must intersect or be parallel, skew lines can exist only in three or more dimensions.

A set of skew lines is shown below:

Parallel lines are at equal distance from each other and never meet.

When two lines cross each other in a plane, they are called intersecting lines.

The coincident lines are lines that lie upon each other in such a way that when we look at them, they appear to be a single line.

Any two lines are said to be skew lines if they do not intersect and also not parallel.

 

Page 275  Exercise 9  Problem 9

Question 9. 

We are given a right rectangular prism. Use the properties of different types of lines to classify each pair of lines as parallel, intersecting, coincident, or skewed. Refer to the provided rectangular prism diagram.

Given Pairs of Lines:

1. \(\overline{AB}\) and \(\overline{BC}\)
2. \(\overline{AD}\) and \(\overline{BC}\)
3. \(\overline{EI}\) and \(\overline{IH}\)
4. \(\overline{BF}\) and \(\overline{EH}\)
5. \(\overline{EF}\) and \(\overline{CG}\)
6. \(\overline{AB}\) and \(\overline{GH}\)

Answer:

We are given a right rectangular prism

We need to classify given pairs of lines as parallel, intersecting, coincident, or skew.

Pair of lines

1.\(\overline{A B}\) and \(\overline{B C}\)

2. \(\overline{A D}\) and \(\overline{B C}\)

3.\(\overline{E I}\) and \(\overline{I H}\)

4.\(\overline{B F}\) and \(\overline{E H}\)

5.\(\overline{E F}\) and \(\overline{C G}\)

6.\(\overline{A B}\) and \(\overline{G H}\)

We will do this by using properties of different types of lines and a table.

 

Page 276   Exercise 10  Problem 10

Question 10.

We are given a right rectangular prism. Use the properties of different types of lines to classify each pair of lines as parallel, intersecting, coincident, or skew. Refer to the provided rectangular prism diagram. In addition to the pairs above, find and classify three more pairs of lines from the right rectangular prism.

Additional Pairs of Lines:

1. \(\overline{AB}\) and \(\overline{BC}\)
2. \(\overline{AD}\) and \(\overline{BC}\)
3. \(\overline{EI}\) and \(\overline{IH}\)
4. \(\overline{BF}\) and \(\overline{EH}\)
5. \(\overline{EF}\) and \(\overline{CG}\)
6. \(\overline{AB}\) and \(\overline{GH}\)
7. \(\overline{CD}\) and \(\overline{DH}\)
8. \(\overline{AE}\) and \(\overline{FG}\)
9. \(\overline{BG}\) and \(\overline{HF}\)

Answer:

We are given a right rectangular prism.

Similar to Page 275 Exercise 9 Problem 9 we have to find three more pairs of lines and we need to classify each of the pairs of lines as parallel, intersecting, coincident, or skew.

 

Page 280  Exercise 11  Problem 11

Question 11.

Refer to the provided right rectangular prism diagram. Using the properties of parallel lines, determine which line contains point B and is parallel to line \(\overline{FG}\).

Answer:

Given: A diagram

To find – Lines that contain point B and parallel to line FG.

Line that contain point B and parallel to \(\overline{F G}\) is \(\overline{B A}\).

The final answer is that the line which contain point B and parallel to\(\overline{F G}\) is \(\overline{B A}\).

 

Page 280  Exercise 12  Problem 12

Question 12.

Refer to the provided right rectangular prism diagram. Using the properties of parallel planes, determine which plane contains point B and is parallel to plane FGH.

Answer:

Given: A diagram

To find – Planes that contain point B and parallel to plane FGH.

The plane that contains point B and parallel to plane FGH is plane ABC.

The final answer is that the plane that contains point B and parallel to plane FGH is ABC.

 

Page 280  Exercise 13  Problem 13

Question 13.

First, observe the provided diagrams. Then, compare the diagrams and identify the corresponding angles.

Diagrams to Consider:

• Corresponding Angles Diagram 1
• Corresponding Angles Diagram 2
• Corresponding Angles Diagram 3

Answer:

First we observe the Diagram after we compare the diagram

With

Here

Corresponding angles in this diagrams are
(∠1,∠5), (∠2,∠6), (∠3,∠8),(∠4,∠7),(∠5,∠10),(∠8,∠9),(∠6,∠12),(∠7,∠11),(∠1,∠9),(∠3,∠11),(∠2,∠10),(∠4,∠12)

​Corresponding angles of this is are,   (∠1,∠5), (∠2,∠6), (∠3,∠8),(∠4,∠7),(∠5,∠10),(∠8,∠9),(∠6,∠12),(∠7,∠11),(∠1,∠9),(∠3,∠11),(∠2,∠10),(∠4,∠12)

 

Page 280  Exercise 14  Problem 14

Question 14.

First, observe the provided diagrams and compare them to identify the alternate interior angles.

Diagrams to Consider:

• Alternate Interior Angle Diagram 1
• Alternate Interior Angle Lines
• Alternate Interior Angle Diagram 2
• Alternate Interior Angle Diagram 3

Answer:

First we will observe the diagram and compare diagram

With

After that write angles.

Alternate interior angles pairs in this diagram are ​(∠5,∠4 ), (∠2,∠7)

Alternate interior angles pair are (​∠2,∠7 ),(∠5,∠4), (∠2,∠7)

 

Page 280  Exercise 15  Problem 15

Question 15.

First, observe the given diagrams and compare them to identify the alternate exterior angles.

Diagrams to Consider:

• Alternate Exterior Angle Diagram 1
• Alternate Exterior Angle Lines
• Alternate Exterior Angle Diagram 2
• Alternate Exterior Angle Diagram 3

Case 1:

• Transversal Line A: Alternate exterior angles in this diagram are (∠3, ∠6), (∠1, ∠8)

Case 2:

• Transversal Line A: Alternate exterior angles in this diagram are (∠3, ∠6), (∠1, ∠8)

Case 3:

• Transversal Line C: Alternate exterior angles in this diagram are (∠1, ∠12), (∠2, ∠11)

Given Diagram:

• Identify all alternate exterior angles.

Answer:

First we will observe the diagram then compare the diagram with

Compare with

And write the angles.

Given diagram is

Case 1:

Here line A is transversal.

Alternate exterior angles in this diagram are (∠3,∠6), (∠1,∠8)

Case 2:

Here line A is transversal.

Alternate exterior angles in this diagram(∠3,∠6),(∠1,∠8)

Case 3:

Here C is transversal

Alternate exterior angles in this diagram are (∠1,∠12),(∠2,∠11)

Alternate exterior angles in this diagram are (​∠6,∠3),(​∠1,∠8),(​∠9,∠6),(​∠11,∠5),(​∠1,∠12),(∠11,∠2)

Alternate exterior angles of this diagram is  (​∠6,∠3),(​∠1,∠8),(​∠9,∠6),(​∠11,∠5),(​∠1,∠12),(∠11,∠2)

 

Page 280  Exercise 16  Problem 16

Question 16.

First, observe the given diagrams and identify the consecutive interior angles.

Diagrams to Consider:

• Consecutive Interior Angle Diagram 1
• Consecutive Interior Angle Diagram 2

Answer:

First we observe the diagram and according to definitions angles presented in one side of transversal line also inside of two lines are consecutive interior angles.

Consecutive interior angles pair of the diagram are ​(∠4,∠7), (∠7,∠10), (∠4,∠10)

Consecutive interior angles of this diagram are ​(∠4,∠7), (∠7,∠10), (∠4,∠10)

Page 281  Exercise 17  Problem 17

Question 17.

When two parallel lines are cut by a transversal, several pairs of angles are formed. Based on the angle relationships, identify and list the pairs of angles that are congruent.

Definitions to Consider:

1. Corresponding Angle Postulate: If two parallel lines are cut by a transversal, pairs of corresponding angles are congruent.
2. Alternate Interior Angle Theorem: If two parallel lines are cut by a transversal, pairs of alternate interior angles are congruent.
3. Alternate Exterior Angle Theorem: If two parallel lines are cut by a transversal, pairs of alternate exterior angles are congruent.

Answer:

When two parallel lines are cut by a transversal, then the resulting pairs of angles are congruent are

The corresponding angle postulate states that if two parallel lines are cut by a transversal, pairs of corresponding angles are congruent.

The alternate interior angle theorem states that if two parallel lines are cut by a transversal, pairs of alternate interior angles are congruent.

The alternate exterior angle theorem states that if two parallel lines are cut by a transversal, pairs of alternate exterior angles are congruent.

Pairs of corresponding angles, pairs of alternate interior angles, and pairs of alternate exterior angles are congruent.

Page 284  Exercise 19  Problem 18

Question 18.

Given that one angle in a transversal setup is 63°, find the values of the angles m∠1 and m∠2. Identify which theorems are applied to determine these values.

Given:

• One angle is 63º. Assume this angle is represented by m∠3 such that m∠3 = 63º.

Answer:

Given: One angle is given and the value is 63°.

Find the value of the angles m∠1 and m∠2.

Determine which theorem is applied to find the values of m∠1 and m∠2.

Since one of the angle is 63°, assume the given angle is represented by m∠3 such that m∠3 = 63°.

To find, apply the theorem of corresponding angles.

We get, m∠3 = m∠1

m∠1 = 63°

To find m∠2, apply the theorem of consecutive interior angles.

m∠3 + m∠2 = 180°

Substitute the value of m∠3 = 63°.

Thus, we have  63° + m∠2 = 180°

m∠2 = 180° − 63°

m∠2 = 117°

The values are m∠1 = 63° and m∠2 = 117°.The theorems applied are corresponding angles and consecutive interior angles.

Page 284  Exercise 20  Problem 20

Question 19.

Given that one angle in a transversal setup is 95°, find the values of the angles m∠1 and m∠2. Identify which theorems are applied to determine these values.

Given:

• One angle is 95°. Assume this angle is represented by m∠3 such that m∠3 = 95º.

Answer:

Given: One angle is given and the value is 95°.

Find the value of the angles  m∠1 and m∠2.

Determine which theorem is applied to find the values of m∠1 and  m∠2.

Since one of the angle is 95° , assume the given angle is represented by m∠3
such that  m∠3 = 95°.

To find, apply the theorem of consecutive interior angles.

We get, m∠3 = m∠2

m∠2 = 95°

The values are m∠1 = 95°  and  m∠2 = 95° .The theorems applied are consecutive interior angles and alternate exterior angles.

Page 285  Exercise 21  Problem 21

Question 20.

Given two angles, 110° and (x + 12)°, find the value of x by applying the appropriate theorem.

Given:

• One angle is 110°. Assume this angle is represented by m∠1.
• The other angle is (x + 12)°. Assume this angle is represented by m∠2.

Answer:

Given: The angles are 110° and (x + 12)

Find the value of x by applying the theorem.

Since one angle is 110° and the other is (x+12)°, assume the given angle are represented by m∠1 and m∠2.

Therefore, we have m∠1 = 110°

m∠2 = (x + 12)°

By the theorem of alternate exterior angles, we have

m∠2  =  m∠1

x + 12 = 110

x = 110 − 12

x = 98

Hence, the value is x = 98.

Substitute the value of  x = 98 into m∠2.

We get, m∠2 = (98 + 12)°

m∠2 = 110°

The value of x is 98.

Page 285  Exercise 22  Problem 22

Question 21.

Given three angles, 4x°, m∠6, and 52º, find the value of x by applying the appropriate theorems.

Given:

• One angle is 4xº. Assume this angle is represented by m∠1.
• Another angle is 52º. Assume this angle is represented by m∠2.

Answer:

Given: The angles are 4x°, m∠6, and 52°.

Find the value of x by applying the theorem.

Since one angle is 4x∘ and the other is 52° , assume the given angle are represented by m∠1 and m∠2.

Therefore, we have

m∠1 = 4x°

m∠2 = 52°

Find the value of m∠6.

Apply the theorem of corresponding angles.

We get, m∠2 = m∠6

m∠6 = 52°

Consider one interior angle to be m∠3 such thatm∠6 and m∠3 are consecutive interior angles.

Apply the theorem of consecutive interior angles.

Thus, we have  m∠6 + m∠3 = 180°

52° + m∠3 = 180°

m∠3 = 180° −52°

m∠3 = 128°

To find the value of x, apply the corresponding angles.

m∠1 = m∠3

4x°= 128x° 

= 32

The value of x is 32.

 

Big Ideas Math Integrated Math Chapter 8 Maintaining Mathematical Proficiency

 

Page 215  Exercise 1  Problem 1

Question 1.

Evaluate the expression |−3 + (−1)|.

Answer:

Given  Expression

|−3 + (−1)|.

We have to find the value of

∣−3 + (−1)∣

we will first solve the number inside the modulus then get its positive value.

Then use the conditions of modulus function to evaluate it further.

⇒ ∣−3+(−1)∣

⇒ ∣−3−1∣

⇒ ∣−4∣

Since for the modulus function when x<0

f(x) = −x

We can write

∣−4∣ = −(−4)

∣−4∣ = 4

The value of the expression: ∣−3+(−1)∣ is 4

 

Page 215  Exercise 2  Problem 2

Question 2.

Evaluate the expression |10 – 11|.

Answer:

Given  Expression

|10 – 11|.

We have to find the value of the expression

∣10 − 11∣

We will first solve the expression inside the bracket

⇒ ∣10 − 11∣ = ∣−1∣

Now since x<0 the value of

f(x) = −x

⇒ ∣−1∣ = −(−1)

⇒ 1

Hence the value of the given expression is 1

The solution of the expression: ∣10 − 11∣ is 1

 

Page 215  Exercise 3  Problem 3

Question 3.

Evaluate the expression |9 – (-1)|.

Answer:

Given expression

|9 – (-1)|.

We have to find the value of the expression

∣9−(−1)∣​

We will first solve the expression inside the modulus function:

⇒ ∣9−(−1)∣ = ∣9 + 1∣

⇒ ∣9−(−1) = ∣10∣

Since for values greater than 0, the mod function is given by

∣x∣ = x , and 10>0 , therefore,∣10∣ = 10

The value of ∣9−(−1)∣ is 10

The value of the expression : ∣9−(−1)∣ upon solving is 10

 

Page 215  Exercise 4  Problem 4

Question 4.

Evaluate the expression |-15 – 7|.

Answer:

Given Expression

|-15 – 7|.

We have to find the value of the expression

∣−15−7∣

We will first solve the expression inside the modulus sign and then evaluate it further using the definition of modulus function

⇒ ∣−15−7∣ = ∣−22∣

We know from the definition of modulus functions that when x<0 like in this case the f(x) is given by

f(x) = −(−x)

Since −22<0

⇒ ∣−22∣ = −(−22)

⇒ ∣−22∣ = 22

Thus the required value is 22

The value of the expression: ∣−15−7∣ upon solving is given by 22

 

Page 215  Exercise 5  Problem 5

Question 5.

Evaluate the expression |-12 + 3|.

Answer:

Given Expression

|-12 + 3|.

We have to find the value of the expression

∣−12 + 3∣

We will first solve the expression inside the modulus sign and then evaluate it further using the definition of modulus function

⇒∣ −12 + 3∣ = ∣−9∣

Since we know from the definition of modulus function that when x<0 the value of the f(x) is given by

f(x) = −x

As we know that −9<0 , the expression will be written as

⇒ ∣−9∣ = −(−9)

⇒ ∣−9∣ = 9

Hence the value of the expression is 9

The value of the expression: ∣−12 + 3∣ upon solving is given by 9

 

Page 215  Exercise 6  Problem 6

Question 6.

Evaluate the expression |5 + (-15)|.

Answer:

Given Expression

|5 + (-15)|.

We have to find the value of the expression

 ∣5+(−15)∣

We will first solve the expression inside the modulus sign and then evaluate it further using the definition of modulus function

⇒ ∣5+(−15)∣ = ∣5 − 15∣

⇒ ∣5+(−15)∣ = ∣−10∣

Since we know from the definition of modulus function, when x<0 , the function f(x) is given by, f(x) = −(−x) since −10<0 , we will write

⇒ ∣−10∣ = −(−10)

⇒ ∣−10∣ = 10

Hence 10 is the value of the expression.

The value of the expression: ∣5+(−15)∣ upon solving is given by 10

 

Page 215  Exercise 7  Problem 7

Question 7.

Evaluate the expression |1 – 12|.

Answer:

Given Expression

|1 – 12|.

We have to find the value of the expression

∣1−12∣

We will first solve the expression inside the modulus sign and then evaluate it further using the definition of modulus function

⇒ ∣1 − 12∣ = ∣−11∣

Since we know from the definition of modulus function that when x<0 the value of the f(x) is given by

f(x) = −x

As −11<0 , we can write the expression ∣−11∣ as

⇒ ∣−11∣ = −(−11)

⇒ ∣−11∣ = 11

Hence 11 is the value of the expression.

The value of the expression:  ∣1 − 12∣   upon solving is given by 11

 

Page 216  Exercise 8  Problem 8

Question 8.

How can Dynamic Geometry Software (DGS) be used to visualize geometric concepts effectively?

Answer:

We need to explain that how can we use DGS to visualize geometric concepts.

We will do this by discussing how to create and manipulate the geometrical models in DGS.

We will also discuss the different uses of DGS to visualize geometrical concepts and also how DGS makes geometrical concepts more interesting and understandable.

We should use dynamic geometric software to show the real-time effects of changes on the geometric models and, it provides the visual of.

 What’s happening and what should be the result of any change, which is really helpful to visualize geometric concepts.

 

Page 216  Exercise 9  Problem 9

Question 9.

Given the lines formed by the walls, floor, and ceiling in a classroom, identify which lines intersect, which are parallel, and which are perpendicular. Provide examples of each type of line relationship using the classroom setting.

Answer:

Given two lines can intersect or not intersect.

We want to see examples using the lines formed by the walls, floor, and ceiling in classroom.

Identfy the lines in your classroom and check they intersect or not.

Intersecting lines are two or more lines that are coplanar to each other and meet at a common point.

An example of this would be the lines in the floor of classroom the lines of floors intersect each other as well and share points of intersection.

Two lines that intersect and form right angles are called perpendicular lines.

An example of this would be the lines in brick walls of classrooms.

Parallel lines are the lines that do not intersect or meet each other at any point in a plane. They are always parallel and are at equidistant from each other.

An example of this would be the lines in wooden ceiling of classrooms.

The lines of floors intersect each other as well and share points of intersection.

The lines in brick walls of classrooms intersect each other perpendicularly.

The lines in wooden ceiling of classrooms are parallel to each other

 

Page 216 Exercise 9 Problem 10

Question 10.

Given a line and a plane, determine whether they intersect or not. Use examples from the lines formed by the walls, floor, and ceiling in a classroom to illustrate each scenario.

Answer:

Given one line and one plane can intersect or not intersect.

We want to see examples using the lines formed by the walls, floor, and ceiling in classroom.

Identfy the lines in your classroom and check they intersect or not.

A line can lie entirely in a plane. An example of this would be the intersection of two walls in your classrom.

The two walls both represent planes and the intersection is the line that lies in both walls.

A line can pass through a plane at only one point as shown below.

An example of this would be the intersection of two walls and the floor since the intersection of the two walls represent a line, the floor represents a plane and the intersection of the walls only intersect the floor at one point.

A line could also nver intersect a plane.

An example of this would be the intersection of two walls and the wall on the opposite side of the room since the intersection of the two walls is a line that will never intersect a wall on the opposite side of the room.

The two walls both represent planes and the intersection is the line that lies in both walls.

The intersection of the two walls represent a line, the floor represents a plane and the intersection of the walls only intersect the floor at one point

The intersection of the two walls is a line that will never intersect a wall on the opposite side of the room.

 

Page 216  Exercise 9  Problem 11

Question 11.

Given two planes can intersect or not intersect.

We want to see examples using the planes formed by the walls, floor, and ceiling in a classroom.

Identify the planes in your classroom and check if they intersect or not.

Answer:

Given one line and one plane can intersect or not intersect.

We want to see examples using the lines formed by the walls, floor, and ceiling in classroom.

Identfy the lines in your classroom and check they intersect or not.

Two planes can intersect each other.

An example of this would be the intersection of two walls would be like the walls going through each other in the center to make a kind of cross like shape.

If two planes intersect each other, the intersection will always be a line.An example of this would be the intersection of two walls of a classroom.

Two non-intersecting planes are parallel to each other an example of this would be the stairs of a classroom.

The intersection of two walls would be like the walls going through each other.

The two walls of a classroom intersect at a line.

The stairs of a classroom are examples of planes that never intersecting

 

Page 217  Exercise 10  Problem 12

Question 12.

Using dynamic geometry software, explore the properties of different types of triangles. Describe how the software helps you understand the following concepts:

1. Definition of a triangle.
2. The sum of internal angles in a triangle.
3. Different types of triangles (equilateral, isosceles, scalene).
4. Verification of the Pythagorean theorem using a right triangle.

Explain how the interactive nature of the software enhances your understanding of these geometric concepts.

Answer:

We want to explore geometry using dynamic geometry software.

Search for a familiar concept as for example triangle.

Now search for the keyword “Triangle”.

The screen will show the definition of triangle along with a “Triangle” as follows.

 

The software explained the term “Triangle” as follows

 

Page 220  Exercise 11 Problem 13

Question 13.

In the given diagram, identify two other sets of non-collinear points that can be used to name plane M. Explain why these sets of points are considered non-collinear and provide the alternative names for plane M.

Answer:

We are given a diagram: 

Here, A, B, C, D, and E are different points and M is a

We need to give another name for plane M.

Here, A, C, and E are non-collinear points, so another name for plane M is plane ACE.

A, B, and E are also non-collinear points, so there is one more name possible for plane M that is plane ABE.

In the given diagram: The other name for plane M is plane ACE.

 

Page 220  Exercise 12  Problem 14

Question 14.

Identify all possible names for the line \(\overleftrightarrow{R S}\) using the points R, S, and T. Explain why each name is valid and provide an additional way to denote the line.

Answer:

We are given a diagram: Here, P, Q, R, S, and T are different points.

We need to find another name for ​\(\begin{aligned}
& \leftrightarrow \\
& \text { RS }
\end{aligned}\)

​
As R, S, and T are different points lying on the line ​\(\begin{aligned}
& \leftrightarrow \\
& \text { RS }
\end{aligned}\) ​ , so it can also be named as ​\(\begin{aligned}
& \leftrightarrow \\
& \text { RS }
\end{aligned}\)

\(\begin{aligned}
& \leftrightarrow \text { or } \leftrightarrow \text { or } \leftrightarrow \text { or } \leftrightarrow \text { or } \leftrightarrow \\
& \begin{array}{lllll}
\text { SR } & \text { ST } & \text { TS } & \text { RT } & \text { TR }
\end{array} \\
&
\end{aligned}\)
​
It can also be denoted as line k.

In diagram:

Another name for ​\(\begin{aligned}
& \leftrightarrow \\
& \text { RS }
\end{aligned}\) is ​\(\begin{aligned}
& \leftrightarrow \\
& \text { SR }
\end{aligned}\).

 

Page 220 Exercise 13 Problem 15

Question 15.

Given points P, Q, R, S, and T in the diagram, identify all rays that have T as the endpoint. Also, identify pairs of opposite rays from these rays. Explain why each identified pair is considered opposite rays.

Answer:

We are given a diagram: Here, P, Q, R, S, and T are different points.

We need to find all the rays with endpoint T and also opposite rays among these rays.

We know that a ray can be named by any letter or by any two points lying on it.

All the rays with endpoint T are and  ​→   TS,  →  TR,→   TP,  →  TQ.

In the given diagram, there are two pairs of opposite rays

1.  ​→   TS  and  →  TR

​2.  →   TP  and  →  TQ

​In the diagram:

All the rays with endpoint T are  →   TS,  →  TR,→   TP,  and →  TQ

​In the given diagram, there are two pairs of opposite rays

1.  ​→   TS  and  →  TR

​2.  →   TP  and  →  TQ

​

Page 220  Exercise 14  Problem 16

Question 16.

Using dynamic geometry software, we need to draw two planes, M and N, that intersect at line k.

Answer:

We are given a diagram:

We are going to draw two planes M and N that will intersect at line k using dynamic geometry software.

Draw plane M passing through line j.

Draw plane N passing through line k.

The diagram that shows planes M and N intersecting at line k:

 

Page 220  Exercise 15  Problem 17

Question 17.

Given a plane M, we need to sketch the graph of line k on plane M.

Steps to sketch the graph:

1. Identify and Plot Two Points:
   • Choose and plot two points on plane M that will lie on line k.
2. Draw Line K:
   • Draw a straight line passing through these two points.
   • Extend the line on both sides to represent the infinite length of the line.

Answer:

Given a plane M.

And we have to sketch the graph of line k on plane M.

To sketch the graph of line k plot two-point of the line and draw line between them and extend their both side.

So the graph of line k is

The graph of line k is

 

Page 221  Exercise 16  Problem 18

Question 18.

Explain how to measure and construct a line segment using a ruler. Provide a step-by-step guide for both processes, including diagrams.

Answer:

In this question, we have to tell how can we measure and construct a line segment.

So for construct, a line segment mark the starting point and put the ruler at zero mark at starting point mark endpoint.

Corresponding distance and then put a pencil at starting point and move along the edge of ruler till you reach the endpoint.

And for the measure, a line segment keep zero ruler at starting point and read the number on the scale where the line segment end.

Like this

For construct, a line segment mark the starting point and put the ruler at zero mark at starting point mark endpoint.

Corresponding distance and then put a pencil at starting point and move along the edge of ruler till you reach the endpoint and for the measure.

A line segment keep zero ruler at starting point and read the number on the scale where the line segment end.

Like this

 

Page 222  Exercise 17  Problem 19

Question 19.

Given a 3-inch index card, fold it along of its diagonals. Describe the steps and illustrate the process using diagrams.

Answer:

Given an index card 3-inch by 5-inch

And we have to fold an index card on one of its diagonals.

Let index card CDBP and is CB its diagonals

To fold the index card on one of its diagonals try to match one corner of the index card with the opposite side of an index card.

So P moved to A.

For fold a 3-inch by 5-inch index card on one of its diagonals do like this

 

Page 222  Exercise 18  Problem 20

Question 20.

Given a 3-inch by 5-inch index card, fold it along one of its diagonals. Use the Pythagorean Theorem to algebraically determine the length of the diagonal in inches. Then, use a ruler to check your answer.

Answer:

Given a fold 3-inch by 5-inch index card fold on one of its diagonals.

And we have to use the Pythagorean Theorem to algebraically determine the length of the diagonal in inches.

Use a ruler to check our answer.

To find the diagonal of index card use the pythagorean Theorem  a² + b² = c²

Where use a = 3 inch , b = 5 inch and c = length of the diagonal  therefore

3² + 5² = c²

⇒  c² = 9+25

⇒ c² = 34

⇒ c = \(\sqrt{34}\) ≈ 5.83 inch

Also by measuring the with ruler its same so our answer is correct.

Measuring the diagonal in inches. the with ruler its same therfore our answer is correct.

 

Page 225  Exercise 19  Problem 21

Question 21.

Given the points A(4,0), B(4,3), C(−4,4), and D(−4,1), plot these points on a coordinate plane and determine whether the line segments AB and CD are congruent. Use the distance formula to calculate the lengths of AB and CD.

Answer:

Given A(4,0), B((4,3) and C(−4,4), D(−4,1).

And we have to plot the points in the coordinate plane. Then determine whether AB and CD are congruent

Plot the points in the coordinate plane.

The distance between A and B is AB

Then AB =\(\sqrt{(4-4)^2+(3-0)^2}\)

= \(\sqrt{0+3^2}\)

= 3

The distance between C and D is CD

Then CD = \(\sqrt{(-4+4)^2+(1+4)^2}\)

= \(\sqrt{0+5^2}\)

= 5

Plot the points A(4,0), B((4,3) and C(−4,4), D(−4,1) in the coordinate plane is AB and CD lines are not congruent because there not lengths are equal

 

 Page 225  Exercise 20  Problem 22

Question 22.

Given the line segment UW with a length of 18 units, and a point V between U and W such that UV = 6 units, find the length of the segment VW.

Answer:

Given line segment is UW and V is any point between U and W.

UW = 18 and

UV = 6 then find VW.

UW = UV + VW

18 = 6 + VW

VW = 18−6

VW = 12

The value of VW is 12 .

 

Page 225   Exercise 21  Problem 23

Question 23.

Given the line segment WV with U as a point between W and V. The lengths of WU and UV are 45 units and 30 units respectively. Find the length of the segment VW.

Answer:

Given line segment is WV and U is any point between W and V.

WU = 45 and UV = 30 then find VW .

VW = VU + UW

VW = 30 + 45

VW = 75

The value of VW is 75 .

 

Page 225  Exercise 21  Problem 24

Question 24.

Given a line segment with endpoints A(3,4) and B(−5,−2). Add point C(3,−2) in such a way that it makes a right-angle triangle with AB as the hypotenuse. Use the Pythagorean theorem to find the length of AB.

Answer:

Given a line segment with endpoints A(3,4) and B(−5,−2).

Add point C (3,−2) in such a way that it makes a right angle triangle with AB as hypotenuse.

Now use Pythagoras theorem to find the length of AB.

From graph it is clear that BC = 8 and AC = 6 .

AB² = BC²+AC²

⇒  AB² = 64 + 36

⇒ AB² = 100

⇒ AB = 10

Length of the line segment AB is 10 units.

 

 

Page 227  Exercise 22   Problem 25

Question 25.

Solve the endpoints of the line segment are D(−10,−4) and E(14,6).

To find: The coordinates of the midpoint and the length of the line segment. Find the length of the given line segment.

Answer:

Given: The endpoints of the line segment are D(−10,−4),E(14,6)

To find: The coordinates of the midpoint and the length of the line segment.

Here, we will use the mid-point formula of a line segment.

And, distance formula to find the length of the given line segment.

The mid-point formula of the line segment.

(x,y) = \(\left(\frac{x_1+x_2}{2}, \frac{y_1+y_2}{2}\right)\)

Since the coordinates of the endpoints are D(−10,−4),E(14,6)

x₁ = −10, x₂ = 14, y₁ = −4, y₂ = 6

So, (x,y) = \(\left(\frac{-10+14}{2}, \frac{-4+6}{2}\right)\)

(x,y) = (\(\frac{4}{2}\), \(\frac{2}{2}\)

(x,y) = (2,1)

Hence, the coordinates of the midpoint of the given line segment is M(2,1)

Distance of the given line segment

Distance formula, D = \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

Since the coordinates are D(−10,−4), E (14,6)  x₁=  −10, x₂  = 14, y₁ = −4, y₂ = 6

DE = \(\sqrt{(14-(-10))^2+(6-(-4))^2}\)

DE = \(\sqrt{(14+10)^2+(6+4)^2}\)

DE = \(\sqrt{(24)^2+(10)^2}\)

DE = \(\sqrt{576+100}\)

DE = \(\sqrt{676}\)

Hence, the coordinates of the midpoint of the line segments whose coordinates of the endpoint are given as D(−10,−4) ,E(14,6)is M(2,1)and the length of the line segment is 24 unit.

Big Ideas Math Integrated Math Chapter 7 Maintaining Mathematical Proficiency Exercise

 

Page 188   Exercise 1  Problem 1

Question 1.

Given the following frequency table

\(\begin{array}{|l|l|}
\hline \text { Number Range } & \text { Frequency } \\
\hline 0-10 & 5 \\
\hline 11-20 & 8 \\
\hline 21-30 & 12 \\
\hline 31-40 & 7 \\
\hline 41-50 & 3 \\
\hline
\end{array}\)

1. Plot a histogram for the given data with the number range on the horizontal axis and frequency on the vertical axis.
2. Describe the shape of the histogram and explain what it indicates about the data distribution.
3. Determine the number range with the highest frequency and the number range with the lowest frequency.
4. Calculate the total number of data points represented in the frequency table.

Answer:

We plot on the horizontal axis the number range.

We plot on the vertical axis frequency which represents the amount of data that is present in each range.

Plot

Histogram of the above data is

 

Page 188  Exercise 2  Problem 2

Question 2.

Given the following frequency table

\(\begin{array}{|l|l|}
\hline \text { Number Range } & \text { Frequency } \\
\hline 0-5 & 4 \\
\hline 6-10 & 7 \\
\hline 11-15 & 10 \\
\hline 16-20 & 6 \\
\hline 21-25 & 3 \\
\hline
\end{array}\)

1. Plot a histogram for the given data with the number range on the horizontal axis and frequency on the vertical axis.
2. Describe the shape of the histogram and explain what it indicates about the data distribution.
3. Determine the number range with the highest frequency and the number range with the lowest frequency.
4. Calculate the total number of data points represented in the frequency table.

Answer:

We plot on the horizontal axis the number range.

We plot on the vertical axis frequency which represents the amount of data that is present in each range.

Plot

 

The data plotted in a histogram.

 

Page 188  Exercise 3  Problem 3

Question 3.

A survey of students’ favorite sports yielded the following results:

• Baseball: 10 students
• Tennis: 4 students
• Basketball: 8 students
• Soccer: 7 students
• Golf: 2 students

1. Calculate the percentage of students who prefer each sport.
2. Assign the calculated percentages to the area of a circle to create a circle graph (pie chart).
3. Draw the circle graph based on the percentages of students for each sport.

Answer:

We will find the percent of each sport which will be used to assign the area of a circle

The percentage is the ratio of a student with the sum of total students

The number of total students is 10 + 4 + 8 + 7 + 2  =  31

Percentage of students for

Baseball = \(\frac{10}{31}\) ×100

Baseball = 32.3

Tennis =  \(\frac{4}{31}\) ×100

Tennis = 12.9

Basketball = \(\frac{8}{31}\) ×100

Basketball = 25.8

Soccer = \(\frac{7}{31}\) ×100

Soccer = 22.6

Golf = \(\frac{2}{31}\) ×100

Golf = 6.5

Plot

The data is plotted in a circle graph

 

 Page 189  Exercise 4 Problem 4

Question 4.

Given the following data set:

4,8,6,5,3,7,9

1. Calculate the mean of the given data set.
2. Find the squared differences from the mean for each data point.
3. Compute the variance of the data set by summing the squared differences and dividing by the number of data points.

Answer:

Given data set

4,8,6,5,3,7,9

We first get the mean of the given data set.

We use mean to get the difference with all the measures but when sum negative & positive difference got cancel out, so to avoid it we square the difference.

We sum all the difference square error & divide them with a given number of data points which will represent the spread of data.

Variance is the sum of squares that measures how data varies around a mean

 

Page 189  Exercise 5  Problem 5

Question 5.

The given graphs show the weights of the players on a professional football team and a professional baseball team. The total number of football players is 53, and the total number of baseball players is 40. The total weight of the football team is 13,075 pounds, and the total weight of the baseball team is 8,290 pounds.

1. Calculate the mean weight of the football players and describe how much the weights vary from the mean.
2. Calculate the mean weight of the baseball players and describe how much the weights vary from the mean.
3. Explain the differences in the variation of weights between the football and baseball players.

Answer:

Given

The total number of football players is 53, and the total number of baseball players is 40. The total weight of the football team is 13,075 pounds, and the total weight of the baseball team is 8,290 pounds.

The given graphs show the weights of the players on a professional football team and a professional baseball team.

So, we need to describe the data in each graph in terms of how much the weights vary from the mean, and also we have to explain it.

Consider the following graph.

The above graph shows that total 53 football players, 40 baseball players and their total weight is 13075 and 8290 pound respectively.

Thus, the mean of football players weight is given by

\(\frac{13075}{53}\) = 246.25 ≈ 210

Here, the mean may vary up to 85 pounds.

The mean of baseball players weight is given by

\(\frac{8290}{40}\) =  207.25 ≈ 210

Here, the mean may vary up to 210−155 = 55 pounds.

Therefore, the mean of football players weight is approximately 250 pounds. It may vary up to 85 pounds. Also, the mean of baseball players weight is approximately 210 pounds. It may vary up to 55 pounds.

 

Page 189  Exercise 5  Problem 6

Question 6.

Given the graphs that show the weights of the players on a professional football team and a professional baseball team, compare how much the weights of the players on each team vary from their respective means. Use the following information:

• The mean weight of football players is approximately 250 pounds, with a variation of up to 85 pounds.
• The mean weight of baseball players is approximately 210 pounds, with a variation of up to 55 pounds.

1. Based on the given means and variations, explain which team’s players have weights that vary more from the mean.
2. Discuss possible reasons for the difference in variation between the weights of football players and baseball players.

Answer:

The given graphs show the weights of the players on a professional football team and a professional baseball team.

So, we need to compare how much the weights of the players on the football team vary from the mean to how much the weights of the players on the baseball team vary from the mean.

With respect to the previous question, we can say that the mean values of football players weight and baseball players weight are approximately 250 and 210 respectively.

Thus, the weight of football players varies more from the mean as compared to baseball players.

Therefore, we can say that the weight of football players varies more from the mean as compared to baseball players.

 

Page 189  Exercise 5  Problem 7

Question 7.

Given the graphs showing the weights of the players on a professional football team and a professional baseball team, determine if there is a correlation between the body weights and the positions of players in professional football. Explain your findings based on the following observations:

1. Similar positions in football have similar weights.
2. The graph for football players’ weights is more consistent compared to the more spread out graph for baseball players’ weights.

Answer:

The given graphs show the weights of the players on a professional football team and a professional baseball team.

So, we need to check whether there is a correlation between the body weights and the positions of players in professional football or not. Also, we have to explain it.

Consider the following graph.

From the above graph , we can say that similar positions in football have similar weights, whereas the graph in baseball is stretched apart.

Thus, there is a correlation between the body weights and the positions of players in football.

Therefore, there is a correlation between the body weights and the positions of players in football.

 

Page 19 0 Exercise 6  Problem 8

Question 8.

The weights (in pounds) of the players on a professional basketball team by position are as follows:

• Power Forwards (PF): 235, 255, 295, 245
• Small Forwards (SF): 235, 235
• Centers (C): 255, 245, 325
• Point Guards (PG): 205, 185, 205
• Shooting Guards (SG): 205, 215, 185

1. Create a graph that represents the weights and positions of the players.
2. Analyze the graph and determine if there appears to be a correlation between the body weights and the positions of players in professional basketball.
3. Explain your findings and describe any patterns observed in the weights of players by position.

Answer:

The weights (in pounds) of the players on a professional basketball team by position are as follows.

Power forwards: 235,255,295,245

Small forwards: 235,235

Centers: 255,245,325

Point guards: 205,185,205

Shooting guards: 205,215,185.

We to have find there appear to be a correlation between the body weights and the positions of players in professional basketball.

Power forwards: 235, 255, 295, 245

Small forwards: 235, 235; centers: 255, 245, 325

Point guards: 205, 185, 205

Shooting guards: 205, 215, 185.

Yes ,there appear to be a correlation between the body weights and the positions of players in professional basketball.

Guards tend to be lighter and forwards tend to be heavier.

Graph that represents the weights and positions of the players

PF – Power Forwards

SF – Small Forwards

C – Centers

PG – Points Guards

SG  – Shooting Guards

The weights (in pounds) of the players on a professional basketball team by position are as follows:

Power forwards: 235, 255, 295, 245; Small forwards: 235, 235; Centers: 255, 245, 325; Point guards: 205, 185, 205;  Shooting guards: 205, 215, 185. we make a graph that represents the weights and positions of the players.

Yes ,there appear to be a correlation between the body weights and the positions of players in professional basketball.

Guards tend to be lighter and forwards tend to be heavier.

 

 Page 190  Exercise 7  Problem 9

Question 9.

Given the following data set of weights (in pounds) of players on a professional basketball team:

235,255,295,245,235,235,255,245,325,205,185,205,205,215,185

1. Calculate the mean of the given data set.
2. Find the squared differences from the mean for each data point.
3. Compute the variance of the data set by summing the squared differences and dividing by the number of data points.

Answer:

Given data set

235,255,295,245,235,235,255,245,325,205,185,205,205,215,185

We first get the mean of the given data set.

We use mean to get the difference with all the measures but when sum negative & positive difference got cancel out, so to avoid it we square the difference.

We sum all the difference square error & divide them with a given number of data points which will represent the spread of data.

Variance is the sum of squares that measures how data varies around a mean

 

Page 193  Exercise 8  Problem 10

Question 10.

Given the data set:

2,5,16,2,2,7,3,4,4

1. Arrange the data points in ascending order.
2. Calculate the mean of the data set.
3. Determine the median of the data set.
4. Identify the mode of the data set.

Answer:

Given data set: 2,5,16,2,2,7,3,4,4

We will arrange all the given data points in ascending order & then count the number of given datapoints.

We get mean by dividing the sum of given with their counts.

We will get median by the middle number.

We will get mode by looking at data set that which number has highest frequency.

Ascending order: 2,2,2,3,4,4,5,7,15

Number of data points is 9 the sum of all data points 2 + 2 + 2 + 3 + 4 + 4 + 5 + 7 + 15  =  44

Mean \(\bar{x}\) = \(\frac{44}{9}\)

\(\bar{x}\) =  4.889

Median is 5th term 4 mode is 2

Mean, median, and mode of the data set are 4.889,4,2 respectively.

 

Page 193  Exercise 8  Problem 11

Question 11.

Given the data set:

2,5,16,2,2,7,3,4,4

1. Arrange the data points in ascending order.
2. Identify the median of the data set.
3. Explain why the median is considered the value in the center of the data set.

Answer:

Given data set: 2,5,16,2,2,7,3,4,4

We will see which number is in the centre of given data set by analysing mean & median

Half of the values are less than the median and half of the values are more than the median.

So The median is the value in the center of the data.

The median is the value in the center of the data.

 

Page 194 Exercise 9 Problem 12

Question 12.

Given the heights (in inches) of players on Team A and Team B:

• Team A Heights: 58, 75, 60, 48, 56, 78, 60, 57, 54, 59
• Team B Heights: 49, 50, 70, 56, 58, 66, 64, 57, 62, 63

1. Arrange the heights of Team A and Team B in ascending order.
2. Calculate the range of heights for Team A.
3. Calculate the range of heights for Team B.
4. Explain why the range of height for Team B is less than the range of height for Team A.

Answer:

Given:

Team A Heights (inches):: 58,75,60,48,56,78,60,57,54,59

Team B Heights (inches):: 49,50,70,56,58,66,64,57,62,63

We will set the data from least to greatest.

We will subtract the smallest value from the largest value

Team A Heights (inches) in order

48,54,56,57,58,59,60,60,75,78

Range of Team A Heights (inches) is 78 − 48 = 30

Team B Heights (inches) in order

49,50,56,57,58,62,63,64,66,70

Range of Team B Heights (inches) is  70 − 49 = 21

For team B is less because its highest player is 70 inches less then 78 inches which is team A highest player

Range of Team A Heights (inches) is 30 & range of Team B Heights (inches) is 21 for team B is less because its highest player is 70 inches less than 78 inches which is team A highest player

 

Page 194  Exercise 10  Problem 13

Question 13.

Given the heights (in inches) of players on Team A:

58,75,60,48,56,78,60,57,54,59:

1. Calculate the mean height \((\bar{x})\) of Team A.
2. Find the deviation of each data value from the mean.
3. Square each deviation.
4. Calculate the variance by finding the mean of the squared deviations.
5. Determine the standard deviation by taking the square root of the variance.

Answer:

Given:

Team A Heights (inches): 58,75,60,48,56,78,60,57,54,59

Step 1 –  Find the mean \(\bar{x}\)

Step 2 – Find the deviation of each data value \(x-\bar{x}\)

Step 3 –  Square each deviation (\(x-\bar{x}\))²

Step 4 –  Find the mean of the squared deviations. This is called variance.

Step 5 –  Take the square root of the variance.

Mean

\(\bar{x}\) = \(\frac{58+75+60+48+56+78+60+57+54+59}{10}\)

\(\bar{x}\) = 60.5

\(\sigma \) = \( \sqrt{\frac{756.5}{10}}\)

\(\sigma \) = \( \sqrt{75.65}\)

\(\sigma\) = 8.69

The standard deviation of the heights of Team A is 8.69

 

Page 194  Exercise 10  Problem 14

Question 14.

Given the heights (in inches) of players on Team B:

49,50,70,56,58,66,64,57,62,63:

1. Calculate the mean height \((\bar{x})\)of Team B.
2. Find the deviation of each data value from the mean.
3. Square each deviation.
4. Calculate the variance by finding the mean of the squared deviations.
5. Determine the standard deviation by taking the square root of the variance.

Answer:

Given:

Team B Heights (inches): 49,50,70,56,58,66,64,57,62,63

Step 1  – Find the mean,\(\bar{x}\)

Step 2 –  Find the deviation of each data value, \(x-\bar{x}\)

Step 3 – Square each deviation   (\(x-\bar{x}\))²

Step 4 –  Find the mean of the squared deviations This is called variance.

Step 5  – Take the square root of the variance.

Mean

\(\bar{x}\) = \(\frac{49+50+70+56+58+66+64+57+62+63}{10}\)

\(\bar{x}\) = 59.5

\(\sigma\) = \(\sqrt{\frac{408.5}{10}}\)

\(\sigma\) = \(\sqrt{40.85}\)

\(\sigma\) = 6.39

The standard deviation of the heights of Team B is 6.39

 

Page 194  Exercise 10  Problem 15

Question 15.

The standard deviations for the heights of players on Team A and Team B are 8.69 and 6.39, respectively:

1. Explain what the standard deviation tells us about a data set.
2. Compare the standard deviations of Team A and Team B and interpret what this comparison indicates about the spread of heights in each team.
3. Based on the standard deviations, which team has heights that are more consistently close to the mean? Provide a brief explanation.

Answer:

Given

The standard deviations for the heights of players on Team A and Team B are 8.69 and 6.39, respectively

We know standard deviations for Team A and Team B are 8.69 and 6.39.

The standard deviation of a numerical data set is a measure of how much a typical value in the data set differs from the mean

Standard deviations for Team A and Team B are 8.69 and 6.39.

Standard deviations for Team A is more than Team B which means values of Team A is more spread than Team B

Values of Team A is more spread than Team B

 

Page 194  Exercise 11  Problem 16

Question 16.

Given the following measures for a data set:

• Mean: 42
• Median: 40
• Mode: 38
• Range: 15
• Standard deviation: 4.9

If each value in the data set increases by 8, calculate the new values for the mean, median, mode, range, and standard deviation. Explain how each measure is affected.

Answer:

Given:

Mean: 42

Median: 40

Mode: 38

Range: 15

Standard deviation: 4.9

when values of the measures shown when each value in the data set increases by 8

Mean, Median, Mode will increase by 8

Range, Standard deviation will remain same

After the values of the measures shown when each value in the data set increases by 8

Mean = 42 + 8

Mean is 50

Median = 40 + 8

Median is  48

Mode is 38 + 8

Mode is  46

Range is 15

Standard deviation is 4.9

Mean is 50

Median is 48

mode is 46

Range is 15

The standard deviation is 4.9

 

Page 195  Exercise 12  Problem 17

Question 17.

Explain why box and whisker plots are ideal for comparing distributions. Describe what a box and whisker plot represents and how it can be used in explanatory data analysis. Use the following prompts to guide your explanation:

1. What information is conveyed by the center, spread, and overall range in a box and whisker plot?
2. How does a box and whisker plot summarize a set of data measured on an interval scale?
3. What features of a distribution are highlighted by a box and whisker plot?
4. Why are box and whisker plots often used in explanatory data analysis?

Answer:

Box and whisker plots are ideal for comparing distributions because the center, spread, and overall range are immediately apparent.

A box and whisker plot is a way of summarizing a set of data measured on an interval scale.

It is often used in explanatory data analysis.

This type of graph is used to show the shape of the distribution, its central value, and its variability.

Box and whisker plots are often used in explanatory data analysis.

 

Page 195  Exercise 13  Problem 18

Question 18.

Given a data set representing the number of first cousins of the students in a ninth-grade class, the data is ordered on a strip of grid paper with 24 equally spaced boxes.

1. Write the data in ascending order in the 24 boxes.
2. Fold the paper into halves to find the median.
3. Calculate the median as the mean of the 12th and 13th observations.

Answer:

Given: A data representing numbers of first cousins of the students in a ninth-grade class.

To find – Order the data on a strip of grid paper with 24 equally spaced boxes.

We will write the given data in ascending order in the boxes.

After folding the paper into halves, we get the median as mean of 12th and 13th Observation

⇒  Median = \(\frac{10+10}{2}\)

⇒  Median = 10.

The final answer is that the ordering of the given data into 24 equally spaced strip is and after folding the paper into halves, we get the median as 10 .

 

Page 195  Exercise 13  Problem 19

Question 19.

Given a data set representing the number of first cousins of the students in a ninth-grade class, arranged in ascending order in 24 equally spaced boxes, find the following:

1. The least value
2. The greatest value
3. The first quartile (Q1)
4. The third quartile (Q3)

Answer:

Given: A data representing numbers of first cousins of the students in a ninth-grade class.

To find – The least value, the greatest value, the first quartile, and the third quartile.

First of all we will divide the 24 equally spaces boxes into four groups such that each group will have 6 numbers.

Then using these groups we will find out the least value, the greatest value, the first quartile and the third quartile.

∵ The order of the data in a 24 equally spaced boxes is

After folding the paper in half again, we get the four groups as

⇒  Least value = 0

Greatest value = 45

∵ First quartile will be mean of 6th and 7th observation.

⇒  First quartile = \(\frac{3+5}{2}\)

⇒  First quartile = 4 .

∵  Third quartile will be mean of 18th and 19th observation.

⇒ Third quartile =  \(\frac{16+18}{2}\)

⇒ Third quartile = 17 .

The final answer is that after folding the boxes in another half, we get four groups of 6 numbers such that the least value is 0 ,the greatest value is 45 , the first quartile is 4 and the third quartile is 17.

 

Page 195  Exercise 13  Problem 20

Question 20.

Given a data set represented by a box-and-whisker plot, which shows the variability of the data along a number line using the least value, the greatest value, and the quartiles:

• The lowest value is 0.
• The greatest value is 45.
• The median (second quartile, Q2) is 10.
• The first quartile (Q1) is 4.
• The third quartile (Q3) is 17.

1. Describe how a box-and-whisker plot represents the given data set.
2. Identify the least value, greatest value, median, first quartile, and third quartile from the data set.
3. Explain the significance of the median, first quartile, and third quartile in dividing the data set.
4. Draw a box-and-whisker plot using the given values.

Answer:

A box-and-whisker plot shows the variability of a data set along a number line

using the least value, the greatest value, and the quartiles of the data.

Quartiles divide the data set into four equal parts.

The median (second quartile, Q2) divides the data set into two halves.

The median of the lower half is the first quartile, Q1.

The median of the upper half is the third quartile, Q3.

In this median is 10 the least value is 0 the greatest value is 45.

The median of the lower half is the first quartile, Q1 & The median of the upper half is the third quartile, Q3

The median of the lower half is 4

The median of the upper half 17

The data set has:

Median is 10

The least value is 0

The greatest value is 45

The median of the lower half is 4

The median of the upper half 17

 

Page 196  Exercise 14  Problem 21

Question 21.

Explain how a box-and-whisker plot represents the variability of a data set. Use the following points to guide your explanation:

1. Define the components of a box-and-whisker plot (least value, greatest value, first quartile, median, and third quartile).
2. Describe how quartiles divide the data set into four equal parts.
3. Explain the significance of the median, first quartile, and third quartile in the context of a box-and-whisker plot.
4. Discuss why box-and-whisker plots are often used in explanatory data analysis.

Answer:

A box-and-whisker plot shows the variability of a data set along a number line using the least value, the greatest value, and the quartiles of the data.

Quartiles divide the data set into four equal parts.

The median (second quartile, Q2) divides the data set into two halves.

The median of the lower half is the first quartile, Q1.

The median of the upper half is the third quartile, Q3.

Box and whisker plots are often used in explanatory data analysis.

 

Page 196  Exercise 15  Problem 22

Question 22.

Given the body mass indices (BMI) of students in a ninth-grade class as represented in a box-and-whisker plot:

1. Identify the median (second quartile, Q2).
2. Identify the first quartile (Q1).
3. Identify the third quartile (Q3).
4. Identify the least value.
5. Identify the greatest value.

Answer:

A box-and-whisker plot shows the variability of a data set along a number line using the least value, the greatest value, and the quartiles of the data.

Quartiles divide the data set into four equal parts.

The median (second quartile, Q2) divides the data set into two halves.

The median of the lower half is the first quartile, Q1.

The median of the upper half is the third quartile, Q3.

In given plot

The median (second quartile, Q2) is 21

The median of the lower half ( first quartile, Q1 ) is 19

The median of the upper half ( third quartile, Q3.) is 22

the least value is 17

the greatest value is 28

Body mass indices (BMI) of students in a ninth-grade class has:

The median (second quartile, Q2) is 21

The median of the lower half ( first quartile, Q1 ) is 19

The median of the upper half ( third quartile, Q3.) is 22

The least value is 17

the greatest value is 28

 

Page 196  Exercise 15  Problem 23

Question 23.

Given the heights of roller coasters at an amusement park as represented in a box-and-whisker plot:

1. Identify the median (second quartile, Q2).
2. Identify the first quartile (Q1).
3. Identify the third quartile (Q3).
4. Identify the least value.
5. Identify the greatest value.

Answer:

A box-and-whisker plot shows the variability of a data set along a number line using the least value, the greatest value, and the quartiles of the data.

Quartiles divide the data set into four equal parts.

The median (second quartile, Q2) divides the data set into two halves.

The median of the lower half is the first quartile, Q1.

The median of the upper half is the third quartile, Q3.

In given plot

The median (second quartile, Q2) is 180

The median of the lower half ( first quartile, Q1 ) is 140

The median of the upper half ( third quartile, Q3.) is 220

The least value is 120

The greatest value is 240

Heights of roller coasters at an amusement park has:

The median (second quartile, Q2) is 180

The median of the lower half ( first quartile, Q1 ) is 140

The median of the upper half ( third quartile, Q3.) is 220

the least value is 120

the greatest value is 240

Big Ideas Math Integrated Math Chapter 5 Maintaining Mathematical Proficiency

 

Page 121  Exercise 1  Problem 1

Question 1.

Given the equation 2x − y = 3, we need to find the points satisfying the equation and plot the graph of the equation.

1. Find the points satisfying the equation 2x − y = 3 by choosing values for x from the set of real numbers (R) and solving for y.
2. Plot the graph of the equation using the points obtained.

Answer:

Given

We have, 2x − y  =  3

We will find the points satisfying the given equation to plot the graph, by taking the value of y from R and obtaining the value ofx corresponding to the value of y

Find the points satisfying the given equation.

2x − y = 3

⇒  y =  2x − 3

Plot the graph.

The graph of an equation 2x − y = 3 is

 

Page 121  Exercise 2  Problem 2

Question 2.

Given the equation 5x+2y=10, we need to find points that satisfy this equation, plot them, and draw the graph of the equation.

1. Find points satisfying the equation 5x + 2y = 10.
2. Plot the graph of the equation using these points.

Answer:

We have, 5x + 2y  = 10

We will find the points satisfying the given equation to plot the graph, by taking the value of y from Rand obtaining the value of x corresponding to the value of y
or vice versa.

Find the points satisfying the given equation.

5x + 2y = 10

⇒ y = \(\frac{10−5x}{2}\)

 

Plot the graph.

The graph of an equation 5x + 2y = 10 is

 

Page 121  Exercise 3  Problem 3

Question 3.

Given the equation y−3 = x, we need to find points that satisfy this equation, plot them, and draw the graph of the equation.

1. Find points satisfying the equation y−3 = x.
2. Plot the graph of the equation using these points.

Answer:

We have, y − 3 = x

We will find the points satisfying the given equation to plot the graph, by taking the value of y from R and obtaining the value of x corresponding to the value of y.

Find the points satisfying the given equation.

y − 3 = x

⇒  y = x + 3

Plot the graph.

The graph of an equation y−3  =  x is

 

Page 121  Exercise 4  Problem 4

Question 4.

Given the equation 3x−y=−2, we need to find points that satisfy this equation, plot them, and draw the graph of the equation.

1. Find points satisfying the equation 3x−y = −2.
2. Plot the graph of the equation using these points.

Answer:

We have, 3x − y = − 2

We will find the points satisfying the given equation to plot the graph, by taking the value of y from R and obtaining the value of x corresponding to the value of y.

Find the points satisfying the given equation.

3x − y = −2

⇒ y = 3x + 2

Plot the graph.

The graph of an equation 3x − y = −2 is

 

Page 121   Exercise 5  Problem 5

Question 5.

1. Find points that satisfy the equation 3x + 4y = 12.
2. Plot the graph of the equation using these points.

Answer:

Find the points satisfying the given equation.

3x + 4y = 12

⇒  y = \(\frac{12−3x}{4}\)

Plot the graph.

The graph of an equation 3x + 4y = 12 is

 

Page 121  Exercise 6  Problem 6

Question 6.

1. Solve the inequality a−3 > −2.
2. Plot the graph of the solution.

Answer:

We have, a − 3 > − 2

⇒  a>1

The graph for a > 1 ⇒

The solution of an inequality a−3>−2 is a>1 and the graph of the solution is

 

Page 121  Exercise 7  Problem 7

Question 7.

1. Solve the inequality −4 ≥ −2c.
2. Plot the graph of the solution.

Answer:

We have,−4 ≥ − 2c

⇒ −2 ≥ − c

⇒ 2 ≤ c

⇒ c ≥ 2

The graph for c ≥ 2 ⇒

The solution of an inequalit y − 4 ≥ −2c is c≥2 and the graph of the solution is

 

Page 121  Exercise 8  Problem 8

Question 8.

1. Solve the inequality 2d − 5 < −3.
2. Plot the graph of the solution.

Answer:

We have ,2d − 5<− 3

⇒ 2d < 2

⇒ d < 1

The graph for d < 1 ⇒

The solution of an inequality 2d−5<−3 is d<1 and the graph of the solution is

 

Page 121  Exercise 9  Problem 9

Question 9.

1. Solve the inequality 8 − 3r ≤ 5 − 2r.
2. Plot the graph of the solution.

Answer:

We have, 8 − 3r ≤ 5 − 2r

⇒ −r ≤ −3

⇒ r ≥ 3

The graph for r ≥ 3 ⇒

The solution of an inequality 8−3r ≤ 5−2r is r ≥ 3

 

Page 122  Exercise 10  Problem 10

Question 10.

Solve the following system of linear equations using both the substitution and elimination methods:

1. x + y = 2
2. x − y = 0

Answer:

We can use any of the two methods to solve a system of linear equations with two variables: Substitution and Elimination.

Substitution: Apply the following steps to solve a system of linear equations.

Isolate any one variable from one of the equations.

Put the isolated value in the other equation and solve the resulting equation.

Put the value of the variable obtained from the resulting equation in any of the two equations and find the value of the other variable.i.e.

x + y = 2  ——— (1)

x−y = 0  ———–(2)

From equation (1)

⇒  x + y = 2

⇒  x = 2−y

Putting the value of x in equation (2), we get

(2−y)−y = 0

⇒ y = 1

x−y = 0  from equation (2)

x = 2−y

⇒  x = 2−1

⇒  x = 1

Hence,(1,1) is the solution of a given system of linear equations.

Elimination: Observe the value of a variable with the same coefficients  (i.e  2x & 2x  or − 2 x & 2x).

If not, then rewrite one or both equations to get the expected values.

Perform addition or subtraction between two equations to get the solution.

Find the value of the other variable by putting the value obtained from the solution in one of the equations.

x + y = 2  −−−——-(1)

x − y = 0  −−——-−(2)

​x + y = 2

(x − y) = 0

​+
_____________
x = 1 ⇒ 1 + y = 2 ⇒ y = 1

Hence,(1,1) is the solution of a given system of linear equations.

We can use any of the two methods to solve a system of linear equations with two variables: Substitution and Elimination.

 

Page 122   Exercise 11  Problem 11

Question 11.

A family spends $600 preparing a bedroom to rent. The cost of food and utilities is $15 per night. Write an equation that represents the total cost C in dollars for renting the bedroom for x nights. Then, find the total cost if the bedroom is rented for 10 nights.

Answer:

Given:

The family spends $600 preparing a bedroom to rent.

The cost of food and utilities is $15 per night.

Suppose the number of nights is x and the total cost is C.

Hence, Cost(in dollars)= Rent of the bedroom for x nights +  Amount spent on preparing a bedroom−−−(1)

Rent for x nights  ⇒  15x

Equation (1) ⇒ C = 15x + 600

C = 15x + 600 is an equation that represents the costs.

 

Page 122 Exercise 11 Problem 12

Question 12.

A family charges $75 per night to rent a bedroom. Write an equation that represents the total revenue R in dollars for renting the bedroom for x nights. Then, find the total revenue if the bedroom is rented for 10 nights.

Answer:

Given:

The family charges $75 per night to rent the bedroom.

Revenue for a night ⇒ 75 (dollars)

Total Revenue for x  nights  ⇒ 75x.

Revenue: R = 75x

R = 75x is an equation that represents the revenue.

 

Page 122  Exercise 11  Problem 13

Question 13.

A family spends $600 preparing a bedroom to rent and incurs a cost of $15 per night for food and utilities. They charge $75 per night to rent the bedroom. Suppose the number of nights is x, the total cost is C, and the total revenue is R.

1. Write a system of linear equations that represents the total cost and total revenue.
2. How many nights must the bedroom be rented to break even?

Answer:

Given:

The family spends $600 preparing a bedroom to rent.

The cost of food and utilities is $15 per night.

Suppose the number of nights is x and the total cost is C.

Hence, Cost(in dollars) =  Rent of the bedroom for x nights+ Amount spent on preparing a bedroom−−−(1)

Rent for x nights  ⇒ 15x

equation (1) ⇒ C = 15x + 600

The family charges $75 per night to rent the bedroom.

Revenue for a night ⇒ 75 (dollars)

Revenue: R = 75x

Hence, C = 15x + 600 and  R = 75x form the system of linear equations for this problem.

C = 15x + 600 and R = 75x forms the system of linear equations for this problem.

 

Page 123   Exercise 12  Problem 14

Question 14.

Given the system of linear equations:

x+y=2 (1)
x-y=0 (2)

Answer:

We can use any of the two methods to solve a system of linear equations with two variables: Substitution and Elimination.

Substitution: Apply the following steps to solve a system of linear equations.

Isolate any one variable from one of the equations.

Put the isolated value in the other equation and solve the resulting equation.

Put the value of the variable obtained from the resulting equation in any of the two equations and find the value of the other variable. i.e.

x + y = 2  −−———-(1)

x − y = 0  −——–−−(2)

From equation (1)

⇒  x + y = 2

x = 2−y

Putting the value of x in the equation (2), we get

(2−y)−y = 0

⇒  y = 1

x = 2 − y

⇒  x = 2 − 1

⇒  x = 1

Hence,(1,1) is the solution of a given system of linear equations.

Elimination:

Observe the value of a variable with the same coefficients(i.e or).

If not, then rewrite one or both equations to get the expected values.

Perform addition or subtraction between two equations to get the solution.

Find the value of the other variable by putting the value obtained from the solution in one of the equations.i.e.

x + y = 2  −−−——–(1)

x − y = 0  −−−——–(2)

​x + y = 2

(x−y) = 0

+

_________________________

x = 1 ⇒ 1 + y = 2 ⇒ y = 1

Hence,(1,1) is the solution of a given system of linear equations.

To verify the solution for given points you just put the given values of x
and y in the given equations and check whether it satisfies both of the equations or not.

It is a solution if it satisfies both of the equations.

It is not a solution if it does not satisfy any of the equations.

i.e for

x + y = 2  —−−—-−(1)

x − y = 0 −−——-−(2)

(1,0) is not a solution for a given system of linear equations as it gives 1 after putting the values of both variables in equation (1).

Whereas (1,1) is a solution for a given system of linear equations as it satisfies both of the equations for given values of x and y

We can use any of the two methods to solve a system of linear equations with two variables: Substitution and Elimination. By putting the given values of variables in given equations we can check whether it is a solution for a given system of linear equations or not.

 

Page 123  Exercise 13  Problem 15

Question 15.

Given the system of linear equations: 

y=-4.3 x-1.3 (1)
y=1.7 x+4.7 (2)

We will solve the system using the graphing method.

Answer:

Given

y=-4.3 x-1.3 (1)
y=1.7 x+4.7 (2)

We have,y = −4.3x − 1.3  −−−——(1)

And y = 1.7x + 4.7  −−−————(2)

We will use graphing method to solve the system as it is easier to apply.

Find the points satisfying the given equation.

For equation (1)

For equation (2)

Plot the graph.

From the graph we can observe that both the lines intersect each other at A(−1,3).

Hence,(−1,3)is the solution of the equations.

(−1,3) is the solution of y = −4.3x−1.3 and y = 1.7x + 4.7 .

 

Page 123  Exercise 13  Problem 16

Question 16.

Given: 
y=x (1)
y=-3 x+8 (2)

We will use the graphing method to solve the system.

Answer:

We have

y = x    −−−———(1)  and

y = −3x + 8   -−−− —–(2)

We will use graphing method to solve the system as it is easier to apply.

Find the points satisfying the given equation.

For equation  (1)

For equation  (2)

Plot the graph.

From the graph we can observe that both the lines intersect each other at E(2,2)

Hence,(2,2) is the solution of the equations.

(2,2) is the solution of y = x and y = −3x + 8

 

Page 123  Exercise 13  Problem 17

Question 17.

Given:

y=-x-1 (1)
y=3 x+5 (2)

We will use the graphing method to solve the system.

Answer:

We have

y = −x − 1    −−−—— (1) and

y = 3x + 5  −−−——- (2)

We will use graphing method to solve the system as it is easier to apply.

Find the points satisfying the given equation.

For equation (1)

For equation (1)

Plot the graph.

From the graph we can observe that both the lines intersect each other at E(\(\frac{-3}{2}\), \(\frac{1}{2}\)).

Hence,(\(\frac{-3}{2}\), \(\frac{1}{2}\))

(\(\frac{-3}{2}\), \(\frac{1}{2}\))is the solution of y = −x−1 and y = 3x + 5.

 

Page 125  Exercise 14  Problem 18

Question 18.

Given:

x+y=4 (1)
2 x-y=3 (2)

We have to solve the system of linear equations using both the substitution and elimination methods.

Answer:

We have

x + y = 4    −−−——(1)

2x − y = 3  −−−——(2)

For(x,y) = (3,1)

In equation x + y = 4   ———-(1)

x = 3

⇒ y = 4−3 = 1

In equation 2x − y = 3  ——-(2)

x = 3

⇒  y = 6−3

⇒  y = 3

⇒  y ≠ 1

Hence given pair is not a solution of linear equations as it does not satisfy the equation (2).

(1,3) is not a solution of the linear equation as it does not satisfy equation (2), where x + y = 4 and 2x − y = 3.

 

Page 125  Exercise 15  Problem 19

Question 19.

Given:

x-y=-2 (1)
2 x+y=5 (2)

We need to determine whether the point (1,3) is a solution to the system of linear equations.

Answer:

We have

x − y = −2 −−−——-(1)

2x + y = 5  −−−——(2)

For (x,y) =  (1,3)

In equation x − y = −2  ————-(1)

x = 1

⇒ y = 1 + 2

⇒  y = 3

In equation  2x + y = 5 (2)

x = 1

⇒ y = 5 − 2 ⇒ y = 3

Hence given pair is a solution of linear equations as it satisfies both of the equations.

(1,3) is a solution of linear equations as it satisfies both of the equations., Where x − y = −2 and  2x + y = 5 .

 

Page 125  Exercise 16  Problem 20

Question 20.

Given:

y=x-2 (1)
y=-3 x+6 (2)

We need to determine whether the point (2,0) is a solution to the system of linear equations.

Answer:

We have

y = x − 2  −−−——-(1)

y = −3x + 6  −−−—-(2)

For (x,y) = (2,0)

In equation y = x − 2  ———-(1)

x = 2

⇒  y = 0

In equation y = −3x + 6  —– —–(2)

x = 2

⇒ y = −6 + 6

⇒ y = 0

Hence given pair is a solution of linear equations as it satisfies both of the equations.

(2,0)is a solution of linear equations as it satisfies both of the equations., Where y = x−2 and y = −3x + 6 .

 

Page 125  Exercise 17  Problem 21

Question 21.

Given:

3 x-2 y=-12 (1)
2 x+4 y=9 (2)

Verify if the point (−2,3) is a solution to the system.

Answer:

We have

3x − 2y = −12  −−−——(1)

2x + 4y = 9 −−−——(2)

For(x,y) = (−2,3)

In equation 3x − 2y = −12 ———–(1)

x = −2

⇒ y = \(\frac{−6 + 12}{2}\)

⇒ y = 3

In equation  2x + 4y = 9 ———–(2)

x =− 2

⇒ y =\(\frac{9+4}{4}\)

⇒ y = \(\frac{13}{4}\) ≠ 3

Hence given pair is not a solution of linear equations as it does not satisfy equation (2).

(−2,3) is not a solution of the linear equation as it does not satisfy equation (2), where 3x−2y  = −12 and 2x + 4y = 9.

 

Page 125  Exercise 18  Problem 22

Question 22.

Given:

x-2 y=5 (1)
2 x+y=-5 (2)

Verify if (−1,-3) is a solution to the system.

Answer:

We have the graph of

x − 2y = 5  −−-−——– (1) and

2x + y = −5 −−−———(2)

From the graph, we can observe that(−1,−3) is the point of intersection for the given equations.

In equation(1)

x = −1

⇒  y = \(\frac{−1−5}{2}\)

⇒  y = −3

In equation (2)

x = −1

⇒  y = −5 + 2

⇒ y = −3

Hence,(−1,−3) is a solution of linear equations as it satisfies both of the equations.

The solution of the linear equations x − 2y = 5 and 2x + y = −5 is(−1,−3).

 

Page 126  Exercise 19   Problem 23

Question 23.

Given: 

y=\(\frac{1}{2}\) x+2(1)
y=\(-\frac{1}{2}\) x+4 (2

Verify if (2,3) is a solution to the system:

Answer:

We have, y = \(\frac{1}{2}\)  x + 2  −−−—- (1) and

y = −  \(\frac{1}{2}\) x + 4  −−−—— (2)

Find the points satisfying the given equation.

For equation(1)

For equation(2)

Plot the graph and find the solution.

From the graph, we can observe that both lines intersect at point A(2,3).

Hence,(2,3) is the solution of linear equations.

(2,3) is the solution of  y =\(\frac{1}{2}\)  x + 2 and y = −  \(\frac{1}{2}\) x + 4

 

Page 126  Exercise 20  Problem 24

Question 24.

Given the system of linear equations:

y=4 x (1)
y=-4 x+8 (2)

1. Verify if the point (2,8) is a solution to the system.
2. Solve the system using the substitution method.
3. Solve the system using the elimination method.
4. Plot both equations and find the intersection point.
5. Confirm the solution (1,4) by substituting it into both equations.

Answer:

We have

y = 4x   −−−——(1)  and

y = −4x + 8  −−−——(2)

We will find the points satisfying the given equation to plot the graph, by taking the value of x from R and obtaining the value of y corresponding to the value of x or vice versa.

Find the points satisfying the given equation.

For equation (1)

For equation (2)

Plot the graph and find the solution.

From the graph we can observe that both lines intersect at point A(1,4).

Hence,(1,4) is the solution of linear equations.

(1,4) is the solution of  y = 4x and  y = −4x + 8.

 

Page 126   Exercise 21  Problem 25

Question 25.

Given the system of linear equations:

y=\(\frac{1}{4}\) x+3 (1)
y=\(\frac{3}{4}\) x+5 (2)

Answer:

We have

y = \(\frac{1}{4}\) x + 3  −−−——- (1)  and

y = \(\frac{3}{4}\)  x + 5  −−−—— (2)

We will find the points satisfying the given equation to plot the graph, by taking the value of x from R and obtaining the value of y corresponding to the value of x
or vice versa.

Find the points satisfying the given equation.

From equation (1)

From equation (2)

Plot the graph and find the solution.

From the graph we can observe that both lines are parallel to each other.

Hence, the system of linear equations has no solution.

The system of linear equations, where y = \(\frac{1}{4}\) x + 3 and y = \(\frac{3}{4}\)  x + 5 has no solution.

 

Page 126  Exercise  22  Problem 26

Question 26.

The test consists of x true-false questions and y multiple-choice questions. The test consists of a total of 20 questions, and the total score for the test is 100 points. True-false questions are worth 4 points each, and multiple-choice questions are worth 8 points each.

Answer:

Given:

The test consists of x true-false questions and y multiple-choice questions.

The test consists of a total of 20 questions.

⇒  x + y = 20  −−−—–(1)

The true-false questions worth 4 points and multiple-choice questions worth 8 points each.

A test has a total of 100 points.

⇒ 4x + 8y = 100   −−−—–(2)

From equation (1)

⇒  x + y = 20

⇒ y = 20 − x

From equation (2)

⇒  4x + 8y = 100

⇒  y  = \(\frac{100-4x}{8}\)

The graph of the lines is:-

From the graph, we can observe that the point of intersection is (15,5).

Hence,(15,5) is the solution of the equations

Thus, there are total 15 true-false questions and 5 multiple choice questions in the test.

 

Page 127  Exercise 23  Problem 27

Question 27.

We can use the substitution method to solve a system of linear equations with two variables.

Substitution Method:

1. Isolate any one variable from one of the equations.
2. Substitute the isolated value into the other equation and solve for the second variable.
3. Substitute the value of the second variable back into the first equation to find the value of the first variable.

Given system of equations:

 x+y=2 (1)
 x-y=0 (2)

Answer:

We can use any of the two methods to solve a system of linear equations with two variables: Substitution and Elimination.

Substitution: Apply the following steps to solve a system of linear equations.
Isolate any one variable from one of the equations.

Put the isolated value in the other equation and solve the resulting equation.

Put the value of the variable obtained from the resulting equation in any of the two equations and find the value of the other variable.i.e.

x + y = 2   −−−——(1)

x − y = 0  −−−——(2)

From equation (1)

⇒  x + y = 2

x = 2−y

Putting the value of x in equation  (2), we get

(2−y) − y = 0

⇒ y = 1

x = 2− y

⇒ x = 2 − 1

⇒ x = 1

Hence,(1,1) is the solution of a given system of linear equations.

We can use Substitution by applying the following steps to solve a system of linear equations.

 

Page 128   Exercise 24  Problem 28

Question 28.

We can choose a randomly ordered pair with integers between (−5,5).

Let’s say the ordered pair is (2,−1).

Given a system of linear equations:

3 x+y=7 (1)
 x-2 y=1 (2)

Answer:

Given

3 x+y=7 (1)
x-2 y=1 (2)

We can choose a randomly ordered pair with integers between (−5,5).

We can use graphing calculator to find ordered pair.

One ordered pair between (-5, 5) is (-2, 3)

⇒ (−2,3) is solution of system of linear equations.

We can choose any random integers as (−1,3)

The ordered pair generated between (−5, 5)is (−1,3).

 

Page 128  Exercise 24  Problem 29

Question 29.

Choose a random ordered pair with integers between (−5,5).

One ordered pair between (−5,5) is (−1,3).

The system of linear equations which has the solution (−1,3) is given below:

x + y = 2(1)

-2x + 3y = 11(2)

We can solve the above system of equations using the substitution method.

Answer:

Given

x + y = 2(1)

-2x + 3y = 11(2)

We can choose random ordered pair with integers between (-5, 5).

We can use graphing calculator to find ordered pair.

One ordered pair between (-5, 5) is (-1, 3).

The system of linear equation which have solution as (-1, 3) are given below:

​x + y = 2  ——— (1)

−2x + 3y = 11  ——— (2)

We can solve the above system of solution using substitution method.

Solving by method 1: Solve for x first solve for x in one of the equation.

Substitute the expression for x into another equation to find y.

Then substitute the value of y into one of the original equation to find value of x.

From equation  (1)

⇒ ​x + y = 2

⇒  x = 2− y

Substituting the expression of x in equation (2) we get

−2x + 3y = 11

​−2(2−y) + 3y = 11

⇒ −4 + 2y + 3y = 11

⇒ y = 3
​
Substituting y = 3 in equation (1) we get

x + 3 = 2

⇒ x = −1
​
Hence solution of system of linear equation are

x = −1

y = 3
​
The solution of system of equation are:  x + y = 2 , −2x + 3y =11 , x = −1, y = 3

 

Page 128  Exercise 25  Problem 30

Question 30.

Using the substitution method to solve a system of linear equations involves the following steps:

1. Isolate one variable in one of the equations.
2. Substitute the isolated variable into the other equation and solve for the remaining variable.
3. Substitute the value of the solved variable back into one of the original equations to find the other variable.

Given the system of linear equations:

x + y = 2(1)

x – y = 0(2)

Answer:

Given

x + y = 2(1)

x – y = 0(2)

We can use any of the two methods to solve a system of linear equations with two variables: Substitution and Elimination.

Substitution: Apply the following steps to solve a system of linear equations.

Isolate any one variable from one of the equations.

Put the isolated value in the other equation and solve the resulting equation.

Put the value of the variable obtained from the resulting equation in any of the two equations and find the value of the other variable. i.e.

x + y =  2   −−−—–(1)

x − y = 0 −−−—–(2)

From equation (1)

x = 2 − y

Putting the value of x in equation (2), we get

(2−y)− y = 0

⇒ y= 1

x = 2 − y

⇒ x = 2 − 1

⇒ x = 1

Hence,(1,1) is the solution of a given system of linear equations.

We can use Substitution by applying the following steps to solve a system of linear equations.

 

Page 130  Exercise 26  Problem 31

Question 31.

Given the system of equations:

x – 3x = -1(1)

x = y (2)

1. Solve the system of equations using the substitution method:
   • Isolate x in equation (2).
   • Substitute the expression for x into equation (1) to find y.
   • Substitute the value of y back into equation (2) to find x.
2. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

We have

x − 3y = −1 −−−——(1)

x = y −−−——— (2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation (1), we get

x − 3y = −1

x = −1 + 3 y

Substituting the value of x in equation (2), we get

x = y

−1 + 3y = y

⇒  y = \(\frac{1}{2}\)

Hence,x = −1 + 3 (\(\frac{1}{2}\))

⇒  x = \(\frac{1}{2}\)

The solution of a given system of equations is( \(\frac{1}{2}\), \(\frac{1}{2}\))

Solve the equations for y.

From equation (1), we get

y =\(\frac{x+1}{3}\)

Substituting the value of y in equation(2), we get

x = \(\frac{x+1}{3}\)

⇒  x = \(\frac{1}{2}\)

Hence

y = \(\frac{\frac{1}{2}+1}{3}\)

⇒  y  =  \(\frac{1}{2}\)

The solution of a given system of equations is   (\(\frac{1}{2}\),\(\frac{1}{2}\))

(\(\frac{1}{2}\),\(\frac{1}{2}\)) is the solution of  x − 3y = −1 and x = y.

 

Page 130  Exercise 27  Problem 32

Question 32.

Solve the given system of equations using the substitution method:

x – 2y = -3(1)

y = x + 1(2)

1. Solve the system of equations using substitution:
   • Isolate x in equation (1).
   • Substitute the expression for x into equation (2) to find y.
   • Substitute the value of y back into equation (1) to find x.
2. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

We have

x − 2y = −3 −−−—–(1)

y = x + 1 −−−——-(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation (1), we get

x = −3 + 2y

Substituting the value of x in equation (2), we get

y = −3 + 2y + 1

⇒ y = 2

Hence,x =−3 + 4

⇒ x = 1

The solution of a given system of equations is (1,2).

Solve the equations for y.

From equation(1) , we get y = \(\frac{x+3}{2}\)

Substituting the value of y in equation (2), we get

\(\frac{x+3}{2}\) = x + 1

⇒  x + 3 = 2x + 2

⇒  x = 1

Hence,y = \(\frac{1+3}{2}\)

⇒ y = 2

The solution of a given system of equations is (1,2).

(1,2) is the solution of x − 2y = −3 and y = x + 1.

 

Page 130  Exercise 28  Problem 33

Question 33.

Solve the given system of equations using the substitution method:

2x + y = 3(1)

x = 3y + 5(2)

1. Solve the system of equations using substitution:
   • Isolate x in equation (1).
   • Substitute the expression for x into equation (2) to find y.
   • Substitute the value of y back into equation (1) to find x.
2. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

We have

2x + y = 3 −−−——(1)

x = 3y + 5 −−−——(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation(1) , we get

x = \(\frac{3−y}{2}\)

Substituting the value of x in equation (2), we get

\(\frac{3−y}{2}\)

= 3y + 5

⇒  3 −y = 6y + 10

⇒  y =−1

Hence,x = \(\frac{3+1}{2}\)

⇒  x = 2

The solution of a given system of equations is (2,−1).

Solve the equations for y.

From equation (1), we get

y = 3 − 2x

Substituting the value of y in equation (2), we get

x = 3(3−2x)+ 5

⇒  x = 14 − 6x

⇒ x = 2

Hence, y = 3−4

⇒  y = −1

The solution of a given system of equations is (2,−1)

(2,-1) is the solution of 2x + y = 3 and x = 3y + 5.

 

Page 130   Exercise 29  Problem 34

Question 34.

Solve the given system of equations using the substitution method:

3x + y = 5(1)

y = 2x + 5(2)

1. Solve the system of equations using substitution:
2. • Isolate x in equation (1).
   • Substitute the expression for x into equation (2) to find y.
   • Substitute the value of y back into equation (1) to find x.
3. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

We have

3x + y = −5  −−−—–(1)

y = 2x + 5  −−−——(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation (1), we get

x = \(\frac{−5−y}{3}\)

Substituting the value of x in equation (2), we get

y = \(\frac{2(−5−y)}{3}\) + 5

⇒  3y = 5 − 2y

⇒  y = 1

Hence,x = \(\frac{−5−1}{3}\)

⇒  x = −2

The solution of a given system of equations is(−2,1)

Solve the equations for y.

From equation (1), we get y = −5−3x

Substituting the value of y in equation (2), we get

−5−3x = 2x + 5

⇒  x = −2

Hence,y = −5−3(−2)

⇒  y = 1

The solution of a given system of equations is(−2,1)

(−2,1) is the solution of 3x + y = −5 and y = 2x + 5

 

Page 130  Exercise 30  Problem 35

Question 35.

Solve the given system of equations using the substitution method:

y = 2x + 8(1)

y = -2x(2)

1. Solve the system of equations using substitution:
   • Isolate x in equation (1).
   • Substitute the expression for x into equation (2) to find y.
   • Substitute the value of y back into equation (1) to find x.
2. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

We have

y = 2x + 8   -−−−—–(1)

y = −2x  −−−——-(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation (1), we get

x = \(\frac{y−8}{2}\)

Substituting the value of x in equation (2), we get

y = −(y−8)

⇒ y = 4

Hence,x = \(\frac{4−8}{2}\)

⇒ x = −2

The solution of a given system of equations is(−2,4)

Solve the equations for y.

From equation (1), we get

y =  2x + 8

Substituting the value of y in equation(2), we get

2x + 8 = −2x

⇒ x = −2

Hence,y = 2(−2)+  8

⇒ y = 4

The solution of a given system of equations is(−2,4)

(−2,4) is the solution of y = 2x + 8 and y = −2x .

 

Page 130  Exercise 31  Problem 36

Question 36.

Solve the given system of equations using the substitution method:

2x – 3y = 0(1)

y = 4(2)

1. Solve the system of equations using substitution:
   • Isolate x in one of the equations if necessary
   • Substitute the expression for y from one equation into the other equation to find x.
   • Substitute the value of x back into one of the original equations to find y.
2. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

We have

2x − 3y = 0  −−−—–(1)

y = 4 −−−——(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation(1) , we get

⇒ 2x − 3y = 0

x = \(\frac{3y}{2}\)

From equation (2), we get

y = 4

Hence,x = \(\frac{3(4)}{2}\)

⇒  x = 6

The solution of a given system of equations is(6,4).

Solve the equations for y.

From, equation (1), we get

⇒ 2x − 3y = 0

y = \(\frac{2x}{3}\)

Substituting the value of y in equation (2), we get

\(\frac{2x}{3}\) = 4

⇒ x = 6

Hence,y = \(\frac{2(6)}{3}\)

⇒ y = 4

The solution of a given system of equations is(6,4).

(6,4) is the solution of 2x−3y = 0 and y = 4 .

 

Page 131  Exercise 32  Problem 37

Question 37.

Solve the given system of equations using the substitution method:

y = 12 x + 1 (1)

y = -12 x -9 (2)

1. Solve the system of equations using substitution:
   • Isolate x in one of the equations if necessary
   • Substitute the expression for y from one equation into the other equation to find x.
   • Substitute the value of x back into one of the original equations to find y.
2. Verify the solution by substituting the values of x and y into both equations (1) and (2).

Answer:

y = \(\frac{1}{2}\) x + 1  ———(1)

y = \(\frac{-1}{2}\) x + 9 −−−——–(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x

From equation (1), we get

x = 2(y−1)

Substituting the value of x in equation (2), we get

y =−(y−1) + 9

⇒ y = 5

Hence, x = 2(5−1)

⇒ x = 8

The solution of a given system of equations is(8,5)

Solve the equations for y

From equation(1), we get

y = \(\frac{1}{2}\) x + 1

Substituting the value of y in equation (2), we get

\(\frac{1}{2}\) x + 1 = \(\frac{ −1}{2}\) x + 9

⇒  x = 8

Hence, y = 4 + 1

⇒  y = 5

The solution of a given system of equations is (8,5)

(8,5) is the solution of  y = \(\frac{1}{2}\) x + 1 and y = \(\frac{-1}{2}\) x + 9.

 

Page 131  Exercise 33  Problem 38

Question 38.

Given the system of equation:

7x – 4y = 8 (1)

5x – y = 2 (2)

Solve the given system of equations using the substitution method.

1. Isolate one variable from one of the equations.
2. Substitute the isolated value in the other equation and solve the resulting equation.
3. Substituting the value of the variable obtained from the resulting equation in any of the two equations and find the value of the other variable.
4. Verify the solution by substituting the values of (x) and (y) into both equations (1) and (2).

Answer:

We have

7x − 4y = 8 −−−——(1)

5x − y = 2 −−−——–(2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x

From equation (1), we get

⇒ 7x − 4y = 8

x = \(\frac{8+4y}{7}\)

Substituting the value of x in equation (2), we get

\(\frac{5(8+4y)}{7}\) −y = 2

⇒  20y + 40−7y = 14

⇒  13y + 40 = 14

⇒  13y = 14−40

⇒  13y = −26

⇒  y = \(\frac{−26}{13}\)

⇒  y = −2

Hence, x =\(\frac{8+4×{−2}}{7}\)

=\(\frac{8−8}{7}\)

⇒ x = 0

The solution of a given system of equations is x = 0,y = −2

Check my answer is correct or not.

When we check the answer is correct or not, this time we check the answer satisfies the given equation or not.

For equation number (1)

L.H.S. 7 × 0 − 4 × (−2)

=  8

=  RHS.

For equation number (2)

L.H.S. 5 × 0 − (−2)

= 2

= RHS.

Therefore x = 0,y =−2  satisfies both equations, then the answer is correct.

x = 0,y = −2 is the solution for 7x−4y = 8 and 5x−y = 2 .

 

Page 131  Exercise 34  Problem 39

Question 39.

We have the system of equations:

y 35x – 12 (1)

y = 18x – 8 (2)

We will solve the given system of equations for (x) and (y) separately by isolating them separately from the given equations.

Answer:

We have

y = \(\frac{3}{5}\) x−12   −−−—– (1)

y = \(\frac{1}{8}\) x−8  −−−——- (2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x

From equation (1), we get

x = \(\frac{5(y+12)}{3}\)

Substituting the value of x in equation (2), we get

y = \(\frac{5(y+12)}{9}\) − 8

⇒  9y = 5y − 12

⇒  y = −3

Hence, x = \(\frac{5(−3+12)}{3}\)

⇒  x = 15

The solution of a given system of equations is(15,−3)

Solve the equations for y

From equation (1), we get

y = \(\frac{3}{5}\) x−12

Substituting the value of y in equation (2), we get

\(\frac{3}{5}\) x−12 = \(\frac{1}{8}\) x−8

⇒  3(3x−60) = 5(x−24)

⇒  x = 15

Hence, y = 9 − 12

⇒  y = −3

The solution of a given system of equations is (15,−3)

(15,−3) is the solution of  y =  \(\frac{3}{5}\) x − 12 and  y =  \(\frac{1}{8}\)  x− 8.

 

Page 131  Exercise 35  Problem 40

Question 40.

Given the system of equations:

3x – 4y = -1(1)

5x + 2y = 7(2)

Solve the system of equations using both the substitution and elimination methods. Verify your solutions by substituting the values back into the original equations.

Answer:

We have

3x − 4y = −1  −−−— (1)

5x + 2y = 7  −−−—– (2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation(1) , we get

x = \(\frac{−1+4y}{3}\)

Substituting the value of x in equation (2), we get

\(\frac{5(−1+4y)}{3}\) + 2y = 7

⇒  26y−5 = 21

⇒  y = 1

Hence x = \(\frac{−1+4}{3}\)

⇒ x = 1

The solution of a given system of equations is  (1,1)

Solve the equations for y.

From equation (1), we get

y = \(\frac{3x+1}{4}\)

Substituting the value of y in equation (2), we get

5x + \(\frac{3x+1}{2}\) = 7

⇒  13x + 1 = 14

⇒  x = 1

Hence,y = \(\frac{3+1}{4}\)

⇒ y−1

The solution of a given system of equations is(1,1)

(1,1) is the solution of 3x−4y = −1 and 5x + 2y = 7.

 

Page 131  Exercise 36   Problem 41

Question 41.

Given:

4x – 8y = 3 (1)

8x + 4y = 1 (2)

We will solve the given system of equations for (x) and (y) separately by isolating them from the given equations.

Answer:

We have

4x − 8y = 3 −−−—— (1)

8x + 4y = 1 −−−—- (2)

We will solve the given system of equations for x and y separately by isolating them separately from the given equations.

Solve the equations for x.

From equation (1), we get

x = \(\frac{3+8y}{4}\)

Substituting the value of x in equation (2), we get

2(3 + 8y) + 4y = 1

⇒ 6 + 20y = 1

⇒ y = \(\frac{−1}{4}\)

Hence, x = \(\frac{3−2}{4}\)

⇒ x = \(\frac{1}{4}\)

The solution of a given system of equations is( \(\frac{1}{4}\),\(\frac{−1}{4}\))

Solve the equations for y.

From equation (1), we get

y = \(\frac{4x−3}{8}\)

Substituting the value of y in equation (2), we get

8x + \(\frac{4x−3}{2}\) = 1

⇒  16x + 4x − 3 = 2

⇒  x = \(\frac{1}{4}\)

Hence, y = \(\frac{1−3}{8}\)

⇒  y = \(\frac{−1}{4}\)

The solution of a given system of equations is(\(\frac{1}{4}\),\(\frac{−1}{4}\) ).

(\(\frac{1}{4}\),\(\frac{−1}{4}\) )is the solution of 4x−8y = 3and 8x + 4y = 1.

 

Page 131  Exercise 37  Problem 42

Question 42.

Given:

The cost of a children’s ticket is (x)

The cost of an adult’s ticket is (y)

We have the following conditions:

1. A museum ticket $3 more than a children’s ticket: y = x + 3
2. When 200 adult tickets and 100 children’s tickets are sold, the total revenue is $2100: 200y + 100x = 2100

Answer:

Suppose the cost of a children’s ticket is x and of an adult’s ticket is y at a museum.

A museum costs $3 more than a children’s ticket

⇒  y = x + 3 −−−—–(1)

When 200 adult tickets and 100 children’s tickets are sold, the total revenue is $2100.

⇒  200y + 100x = 2100 −−−—-(2)

Putting the value of equation (1) in equation (2)

⇒  200(x+3) + 100x = 2100

⇒  300x = 1500

⇒  x = 5

Hence, the cost of a children’s ticket is $5.

Big Ideas Math Integrated Math Chapter 6 Maintaining Mathematical Proficiency

 

Page 157  Exercise 1  Problem 1

Question 1.

Given the expression 8 ÷ 4.19 + 18 + 13, simplify it according to the order of operations (BODMAS/BIDMAS rule). show all steps and calculations clearly.

Answer:

The given expression  8 ÷4.19 + 18 + 13  is to be simplified as per the order of precedence of mathematical operators stated in “BODMAS” rule.

Here, we will first perform division, then multiplication, and finally addition.

Rewriting the given expression

⇒  8 ÷4.19 + 18 + 13

Upon division, we get

⇒  2.19 + 18 + 13

Multiplication follows

⇒  38 + 18 + 13

Finally adding them, we get

⇒ 69

Upon evaluation of the expression 8 ÷ 4.19 + 18 + 13, as per the precedence of operations stated by the “BODMAS” rule, we get 69 as the answer.

 

Page 157  Exercise 2  Problem 2

Question 2.

Given the expression 3.14.11 + 4² + 19, simplify it according to the order of operations (PEMDAS/BODMAS rule) show all the steps and calculations clearly.

Answer:

Given

The expression 3.14.11 + 4² + 19

Here, we have to evaluate the given expression as per the”PEMDAS” rule.

We will solve it as per the order of operations stated in this rule.

The expression given in the question is

⇒  3.14.11 + 4²+ 19

Solving the exponent first, we get

⇒  3.14.11 + 16 + 19

Performing multiplication, we get

⇒  462 + 16 + 19

Adding them all, we get

⇒  497

Simplifying the expression 3.14.11 + 4² + 19  as per the “PEMDAS” rule, the answer is evaluated as 497.

 

Page 157  Exercise 3  Problem 3

Question 3.

Given the expression (21 + 2)(14-6) + 3², simplify it according to the order of operations (PEMDAS/BODMAS rule). show all the steps and calculations clearly.

Answer:

Given

The expression (21 + 2)(14-6) + 3²

Here, we have to evaluate the given expression as per the“PEMDAS” rule.

We will solve it as per the order of operations stated in this rule.

We rewrite the given expression

⇒  (21 + 2)(14−6) + 3²

Solving both the parenthes is, we get

⇒ 23.8 + 3²

Now, solving the exponents, we get

⇒ 23.8 + 9

Now, performing multiplication, we get

⇒ 184 + 9

Finally, upon adding, we get

⇒ 193

Upon simplification of the expression given as (21 + 2)(14−6) + 3² as per the “PEMDAS” rule, the answer is evaluated as 193.

 

Page 157  Exercise 4  Problem 4

Question 4.

Explain the zero exponent rule and use it to evaluate the expression 64⁰. Provide a detailed proof and an example to illustrate the rule.

Answer:

Proof Of Zero Exponent Rule- Consider an exponential term a^m.

We divide it by the same exponential term, and find the quotient.

Mathematically, we can write

⇒  \(\frac{a^m}{a^m}\)

Both cancel out each other and we get 1 as our result.

But, by the rule of exponents, we have  a(m−m) = a⁰.

Thus, from here we observe that a⁰ = 1

Here, we need to evaluate the exponential expression given in the question.

The expression has two components viz; the base 64 and the exponent 0.

The complete expression is called as power which is often confused with exponent.

We will make use of the rule of zero exponent and evaluate the given expression.

Applying the rule of zero exponent in the expression, we have

⇒ 64⁰

= 1

 The expression is  64⁰ evaluated out to be 1.

 

Page 157  Exercise 5  Problem 5

Question 5.

Explain how to evaluate the exponential expression (4)^{− 2}. Provide a detailed explanation using the rules of exponents and show each step of the calculation. What is the value of 4^{− 2}?

Answer:

Given

(4)^{− 2}.

Here, we are asked to evaluate the exponential expression(4)^{− 2}

The expression has the base as 4

and has a negative exponent i.e(−2).

Since it is in the numerator, we need to flip it down to the denominator to make the complete expression evaluable.

We can rewrite the given expression in the following form

⇒  (4)^{− 2} = \(\frac{1}{(4)^2}\)

⇒ (4)^{− 2}  =  \(\frac{1}{(-4) \times(-4)}\)

⇒  (4)^{− 2}  =  \(\frac{1}{16}\)

The expression 4–2 is evaluated out to be \(\frac{1}{16}\).

 

Page 157  Exercise 6  Problem 6

Question 6.

Explain how to evaluate the exponential expression (-3)^-3. Provide a detailed explanation using the rules of exponents and show each step of the calculation. What is the value of (-3)^-3?

Answer:

We are given the exponential expression as(−3)^-3.

Here the base and its exponent both are the same i.e.−3.

In order to evaluate this expression, since it is in the numerator, we need to turn it down to the denominator.

We can rewrite the expression given in an evaluable form as

⇒ (−3)⁻³  =  \(\frac{1}{(-3)^3} \)

⇒ (−3)⁻³  =  \(\frac{1}{(-3) \times(-3) \times(-3)} \)

⇒ (−3)⁻³  =  \(\frac{1}{−27}\)

⇒ (−3)⁻³ =  \(\frac{1}{−27}\)

The expression(−3)⁻³ is evaluated out to be \(\frac{1}{−27}\).

 

Page 157  Exercise 7  Problem 7

Question 7.

Evaluate the exponential expression 7⁰+ 5^{− 2}. Use the rule for zero exponents, negative exponets, and positive exponents to simplify the expression step-by-step. What is the final value of the expression?

Answer:

The given exponential expression is 7⁰+ 5^{− 2}

Use zero exponential, negative exponential, positive exponential method Evaluate the expression

The given expression is 7⁰+ 5^{− 2}

=  1 +  \(\frac{1}{5^2}\)

=  1 +  \(\frac{1}{25}\)

=   \(\frac{26}{25}\)

The evaluation of the expression 7⁰ + 5^{− 2}  is \(\frac{26}{25}\)

 

Page 157  Exercise 8  Problem 8

Question 8.

Evaluate the exponential expression (−2)−6⋅8⁰. Use the rules for zero exponents, negative exponents, and positive exponents to simplify the expression step-by-step. What is the final value of the expression?

Answer:

The given exponential expression is (−2)−6⋅8⁰

Use zero exponential, negative exponential, positive exponential method
Evaluate the expression

The given exponential expression is (−2)−6 ⋅8⁰ (−2)−6⋅8⁰

=  \(\frac{1}{-2^6} \cdot 8^0\)

=  \(\frac{1}{64}\) . 1

=  \(\frac{1}{64}\)

The evaluation of the expression (−2)−6 ⋅8⁰ is \(\frac{1}{64}\)

 

Page 157  Exercise 9  Problem 9

Question 9.

Evaluate the exponential expression 7³ .7⁻³. Use the rules for zero exponents, negative exponents, and positive exponents to simplify the expression step-by-step. What is the final value of the expression?

Answer:

The given exponential expression is 7³ .7⁻³

Use zero exponential, negative exponential, positive exponential method
Evaluate the expression

The given exponential expression is 7³ . 7⁻³

= 7³. \(\frac{1}{7^3}\)

= \(\left(\frac{7}{7}\right)^3\)

= 1³

= 1

The evaluation of the expression 7³⋅7⁻³  is 1

 

Page 157  Exercise 10  Problem 10

Question 10.

Evaluate the exponential expression 6−2 ÷ 1⁹ . 9. Use the rules for zero exponents, negative exponents, and positive exponents to simplify the expression step-by-step. What is the final value of the expression?

Answer:

The given exponential expression is  6−2 ÷ 1⁹ . 9

Use zero exponential, negative exponential, positive exponential method
Evaluate the expression

The given exponential expression is

= \(\frac{1}{6^2} \times \frac{1}{1^9} \cdot 9\)

=\(\frac{1}{36}\). 1. 9

= \(\frac{1}{4}\)

The evaluation of the expression   6−2 ÷ 1⁹ . 9  is \(\frac{1}{4}\)

 

Page 157  Exercise 11  Problem 11

Question 11.

Given the arithmetic sequence 1,5,9,13, …, find the formula for the nth term of the sequence. Use the formula a_n = a + (n−1)d to determine the expression for the nth term. What is the nth term of the sequence?

Answer:

The given arithmetic sequence is

1,5,9,13………

Using the formula of the arithmetic sequence

a_n = a + (n−1)d

The common difference d = b − a

b = 5, a = 1

​d = 5−1 d = 4

​The n^th  term is

a_n = 1 + (n−1)4

a_n= 4n − 3

The n^th term of  1,5,9,13,….   is   a_n = 4n − 3

 

Page 157  Exercise 12  Problem 12

Question 12.

Given the arithmetic sequence 21, 15, 9, 3, …, find the formula for the nth term of the sequence. Use the formula a_n =  a + (n – 1) d to determine the expression for the nth term. What is the nth term of the sequence?

Answer:

The given arithmetic sequence is

21,15,9,3……

Using the formula of the arithmetic sequence

a_n =  a + (n – 1) d

The common difference d = b − a

d = 15 − 21

d = − 6

The nth term is

a_n = 21 + (n−1)(−6)

= 21 + 6−6n

a_n = 27−6n

The n^th term of the sequence 21,15,9,3,… is  27−6n

 

Page 157  Exercise 13  Problem 13

Question 13.

Given the arithmetic sequence -2, 1, 4, 7, …, find the formula for the nth term of the sequence. Use the formula a_n = a+(n-1)d to determine the expression for the nth term. What is the nth term of the sequence?

Answer:

The given arithmetic sequence is

−2,1,4,7……

Using the formula of the arthmetic sequence

a_n = a+(n-1)d

The common difference

d = b − a

d = 1−(−2)

d = 3

The n^th term of the sequence is

a_n =−2 + (n−1)3

= −2 + 3n − 3

= 3n − 5

The n^th term of the sequence −2,1,4,7,… is  3n−5

 

Page 157  Exercise 14  Problem 14

Question 14.

Given the arithmetic sequence -10, -4, 2, 8, …, find the formula for the nth term of the sequence. Use the formula a_n = a+(n-1)d to determine the expression for the nth term. What is the nth term of the sequence?

Answer:

The given arithmetic sequence is

−10,−4,2,8……

Using the formula of the arthmetic sequence

a_n = a+(n-1)d

The common difference

d = b−a

= −4 + 10

d = 6

The n^th term of the sequence is

a_n = −10 + (n−1)(6)

= −10−6 + 6n

= 6n−16

The n^th term of the sequence −10,−4,2,8,… is 6n−16

 

Page 158 Exercise 15 Problem 15

Question 15.

What are the key characteristics of an exponential function?

Describe the properties of its graph, including the points it passes through, the domain and range, the behavior as x approaches positive and negative infinity, and other relevant features.

Answer:

The characteristics of the exponential function are

The graph passes through the point (0,1).

The domain is all real numbers

The range is  y > 0

The graph is increasing

The graph is asymptotic to the x-axis as x approaches negative infinity

The graph increases without bound as x approaches positive infinity

The graph is continuous

The graph is smooth

These are the characteristics of the exponential function

 

Page 159 Exercise 16 Problem 16

Question 16.

Given the exponential functions y = 16(2)^x and  y = 16(\(\left(\frac{1}{2}\right)^x\), describe the process for sketching these graphs. Identify key points used for plotting each graph and explain the symmetry observed around the y-axis.

What are the steps to determine specific points for plotting, and what does this symmetry indicate about the relationship between these two functions?

Answer:

We sketch the graph of y=16(2)^x and

y=16\(\left(\frac{1}{2}\right)^x\) as shown above.

We sketch first graph using points x = −5 and y = 0.5

Also y = 5

For x = −1.67

We plot second graph using points x = 5

y = 0.5

Also,y = 5

x = 1.67

We find points to plot first graph y = 16(2)x

For x = −5

y = 16(2)−5

y = 0.5

Also, when y=5

x = \(\frac{\log \frac{5}{16}}{\log 2}\)

We now plot second graph y 16(\(\left(\frac{1}{2}\right)^x\) using points

x  =  5

y = \(16\left(\frac{1}{2}\right)^5\)

y = 0. 5

When y = 5

x = \(\frac{\log \frac{5}{16}}{\log \frac{1}{2}}\)

x = 1.67

Both the graphs are symmetric around Y axis

We plotted graph using few points like

First graph (−5,0.5) ,(−1.67,5)

Second graph (5,0.5),(1.67,5)

Graph is symmetric around Y axis

 

Page 159  Exercise 17  Problem 17

Question 17.

Given the exponential function y = 2^x, sketch the graph and describe its characteristics. Identify the key points used for plotting the graph and explain the behavior of the function as x approaches both negative and positive infinity.

What does the behavior of the function indicate about the relationship between the input x and the output y?

Answer:

The given expression is y = 2^x

The characteristics of the given expression are

The graph passes through the point (0,1).

The domain is all real numbers

The range is  y > 0

The graph is increasing

The graph is asymptotic to the x-axis as x approaches negative infinity

The graph increases without bound as x approaches positive infinity

The graph is continuous

The graph is smooth

The graph of the given expression y = 2x is

 

Page 159  Exercise 17  Problem 18

Question 18.

Given the exponential function y = 2.(3)^x, sketch the graph and describe its characteristics. Identify the key points used for plotting the graph and explain the behavior of the function as x approaches both negative and positive infinity.

What does the behavior of the function indicate about the relationship between the input x and the output y?

Answer:

The given expression is y = 2.(3)^x

The characteristics of the given expression are

The graph passes through the point (0,2).

The domain is all real numbers

The range is  y > 0

The graph is increasing

The graph is asymptotic to the x-axis as x approaches negative  infinity

The graph increases without bound as x approaches positive infinity

The graph is continuous

The graph is smooth

The graph of given expression is

 

 Page 159  Exercise  17  Problem 19

Question 19.

Given the exponential function y = 3⋅(1.5)^x, sketch the graph and describe its characteristics. Identify the key points used for plotting the graph and explain the behavior of the function as x approaches both negative and positive infinity.

What does the behavior of the function indicate about the relationship between the input x and the output y?

Answer:

 The given expression is y = 3⋅(1.5)^x

The characteristics of the given expression are

The graph passes through the point (0,3).

The domain is all real numbers

The range is  y > 0

The graph is increasing

The graph is asymptotic to the x-axis as x approaches negative infinity

The graph increases without bound as x approaches positive infinity

The graph is continuous

The graph is smooth

The graph of given expression is

 

Page 161  Exercise 18   Problem 20

Question 20.

Assume the given table represents an exponential function of the form y = ab^x.

Using the given values for x and y in the table, determine the values of a and b for the exponential function.

Verify if the determined exponential function satisfies all the given points in the table, particularly checking y for x = -1.

Answer:

We assume the table represents an exponential

Function y = ab^x

We substitute y = 6

For x = 1

Thus we get an expression in a and b

Now we substitute y = 3

For x = 2

Thus we solve the equation for a and b

We get an exponential equation

We check the equation for y using x = −1

Lets assume that table represents an exponential equation y = ab^x

From table y = 6

For x = 1

Thus ab = 6

From table y = 3

For x = 2

We substitute in exponential function

3 = abb

3 = 6.b

b =\(\frac{1}{2}\)

We substitute b

a .\(\frac{1}{2}\) = 6

a = 12

The exponential function is y = 12\(\left(\frac{1}{2}\right)^x\)

We check the above equation for x = −1

y = 12\(\left(\frac{1}{2}\right)^{-1}\)

y = 24

y ≠ 12

Thus table doesn’t represent any exponential function

y = 12\(\left(\frac{1}{2}\right)^x\) doesn’t satisfy  x = −1 for y = 12 Thus table doesn’t represent exponential function

 

Page 161  Exercise 19   Problem 21

Question 21.

Assume the given table represents an exponential function of the form y=ab^x.

\(\begin{array}{|l|l|}
\hline x & y \\
\hline-1 & 0.5 \\
\hline 0 & 1 \\
\hline 1 & 2 \\
\hline 2 & 8 \\
\hline
\end{array}\)

1. Using the given values for x and y in the table, determine the values of a and b for the exponential function.
2. Verify if the determined exponential function satisfies all the given points in the table, including additional points such as x=−1 and x=0.

Answer:

We assume table represents an exponential

Function y = ab^x

We substitute y = 2

For x = 1

Thus we get an expression in a and b

Now we substitute y = 8

For x = 2

Thus we solve the equation for a and b

We get an exponential equation

Lets assume that table represents an exponential equation y = ab^x

From table y = 2

For x = 1

Thus ab = 2

From table y = 8

For x = 2

We substitute in exponential function

8 = abb

8 = 2.b

b = 4

We substitute b

a.4 = 2

a = \(\frac{1}{2}\)

y = \(\frac{1}{2}\)(4)^x

It also satisfy x = −1 and x = 0

Table represents an exponential functiony = \(\frac{1}{2}\)(4)^x

 

Page 161  Exercise 20  Problem 22

Question 22.

Given the exponential function y = 3^x:

1. Substitute x=5 into the function to find the value of y.
2. Write the resulting value of y as the function evaluated at x=5.

Answer:

We write given equation as y = 3^x

We substitute x = 5

We get y by solving above equation

We thus write solved function

We write given equation y = 3^x

We substitute x = 5

y = 3⁵

Thus function is y = 243

Function for given value of x is y  =  243

 

Page 161 Exercise 21 Problem 23

Question 23.

Given the equation y=(14)^-x:

1. Substitute x=3 into the equation and solve for y.
2. Verify the value of y obtained by showing all the steps.
3. State the function with the substituted value of x and the corresponding value of y.

Answer:

We write given equation as y = \(\left(\frac{1}{4}\right)^x\)

We substitute x = 3

We get y by solving above equation

We thus write solved function

We write given equation y = \(\left(\frac{1}{4}\right)^x\)

We substitute x = 3

y = \(\left(\frac{1}{4}\right)^3\)

Thus function is y = 0.0156

Function for given value of  x is  y = 0.0156

 

Page 161  Exercise 22  Problem 24

Question 24.

Given the equation y=3(4)^x:

1. Substitute x=4 into the equation and solve for y.
2. Verify the value of y obtained by showing all the steps.
3. State the function with the substituted value of x and the corresponding value of y.

Answer:

We write given equation as y = 3(4)^x

We substitute x = 4 in above equation

We get y by solving above equation

We thus write solved function

We write given equation y = 3(4)^x

We substitute x = 4

y = 3(4)⁴

Thus function is  y = 768

Function for given value of x is y = 768

 

Page 162   Exercise 23  Problem 25

Question 25.

Consider an exponential function of the form y=abx represented by a graph. Based on the graph, the following points are known:

• When x = 0, y = -0.5
• When x = -0.5, y = -1
• When x = -1, y = -2

1. Using the point where x=0, determine the value of a.
2. Using the point where x = −0.5, determine the value of b.
3. Verify the function with the point where x=−1.
4. Write the exponential equation representing the graph.

Answer:

We assume table represents an exponential function is y = ab^x

We substitute y = −0.5

From graph

x = 0

Thus we get an expression in a

Now we substitute y = −1

From graph

For x = −0.5

Thus we get expression in a and b

We check the above equation for y =−2

From graph

For x = −1

Lets assume the graph represents exponential equation

y = ab^x

From graph y =−0.5

For x = 0

a = −0.5

We substitute y = −1 from graph

For x = −0.5

−1 = (−0.5)b−0.5

b = \(\frac{1}{4}\)

The exponential equation is  y = (−0.5)(\(\frac{1}{4}\))^x

We check the above equation for  x = −1 from graph

y = (−0.5)(\(\frac{1}{4}\))−1 

y = −2

Hence graph represents exponential function y = (−0.5)(\(\frac{1}{4}\))^x

Graph represents exponential equation y = (−0.5)(\(\frac{1}{4}\))^x

 

Page 163   Exercise 24  Problem 26

Question 26.

Explain the characteristics of exponential growth and decay functions by answering the following:

1. Define an exponential growth function and provide a real-world example.
2. Define an exponential decay function and provide a real-world example.
3. Write the general form of both exponential growth and decay functions.
4. Explain the role of the constant r in the function f(x)=a(1+r)x.
5. Describe the behavior of exponential growth and decay functions in terms of increasing or decreasing nature.

Answer:

We have to explain the characteristics of an exponential growth and decay functions.

We will first define both the functions and then give their salient characteristics.

An exponential growth function is a function in which the value of the function increases by a fixed percentage on every increment of x.

Compound interest calculation are a type of exponential growth function.

An exponential decay function is a function whose value decreases by a fixed percentage on every increment of x.

Radioactivity and half life are the example of exponential decay functions.

Both the exponential growth and decay functions defined as:
f (x) = a (1 + r)^x

Here r can be positive or negative depending on whether the function is growth or decay function.

It is the percent change in decimal form and a is a constant and is the vertical intercept of the graph.

The exponential growth function is an increasing function while the exponential decay function is a decreasing function.

We have thus explained the exponential growth and decay functions along with their definitions and characteristics.

 

Page 164  Exercise 25  Problem 27

Question 27.

A body initially had a temperature of 80.5°F, while the room temperature was 60°F. After one hour, the body temperature decreased to 78.5°F. Calculate the percentage decrease of the difference between the body temperature and the room temperature in one hour.

1. Calculate the initial difference (DI) between the body temperature and the room temperature.
2. Calculate the final difference (DF) between the body temperature and the room temperature after one hour.
3. Determine the change (C) in the difference between the initial and final temperatures.
4. Compute the percentage decrease (P) from the initial difference (DI) to the final difference (DF).

Answer:

We have to calculate the percentage decrease of the difference between the body and the room temperature in one hour.

The temperature earlier was 80.5° F while the room temperature was 60° F

The temperature became 78.5°F

The difference initially between the body and the room temperature is given by

D_I =  80.5−60 

D_I = 20.5

The final difference is given by

D_F = 78.5−60

D_F = 18.5

Change in the initial and final difference

C = 20.5−18.5

C = 2

Percentage change from DI to DF will be given by

P = \(\frac{C}{D_I} \times 100\)

P = \(\frac{2}{20.5} \times 100\)

P = 9.76

The percent change is P = 9.76 %

The percentage change between the difference of the two body temperature and the room temperature is 9.76 %

 

Page 164   Exercise 25  Problem 28

Question 28.

Using the table provided, which shows the decrease in body temperature as a difference from the room temperature, estimate the time of death. The percentage decrease in the difference of the temperature has been calculated to be 9.76%.

Here is the table showing the decrease in body temperature over time:

∴ \(\begin{array}{|l|l|l|}
\hline \text { Time (hours) } & \text { Body Temperature }\left({ }^{\circ} \mathrm{F}\right) & \text { Difference }\left({ }^{\circ} \mathrm{F}\right) \\
\hline 0 & 80.5 & 20.5 \\
\hline 1 & 78.5 & 18.5 \\
\hline 2 & 76.8 & 16.8 \\
\hline 3 & 75.1 & 15.1 \\
\hline 4 & 73.5 & 13.5 \\
\hline 5 & 71.9 & 11.9 \\
\hline 6 & 70.3 & 10.3 \\
\hline
\end{array}\)

Given that at h=6 (6 AM) the body temperature was nearly 80.5°F (slight difference due to rounding off the temperature at each step), and that the time of death was estimated to be 6 hours prior:

1. Explain how the difference in body temperature helps estimate the time of death.
2. Determine the estimated time of death based on the given table and calculate the percentage of decrease in the difference.
3. Verify the consistency of the estimated time of death with the given data.

Answer:

We have to prepare a table showing the decrease in body temperature as difference of the body temperature and estimate the time of death.

Note that we calculated the percentage decrease in the difference of the temperature to be 9.76%

The table is shown below

We can see that the at h = 6 was the midnight as that was the time when the temperature was nearly 80.5 ,(slight difference due to rounding off the temperature at each step).

Thus time of death was 6 hours prior and hence the death was at 6 PM in the evening

We have prepared the table of time against difference of the body temperature and the room temperature, the estimated time of death is 6 PM

 

Page 167  Exercise 26  Problem 29

Question 29.

The population of rabbits in a park grows at a rate of 11% every year. Initially, there were 100 rabbits in the park.

1. Write the exponential function representing the growth of the rabbit population.
2. Calculate the population of rabbits after 5 years using the exponential function.
3. Determine the population of rabbits after 10 years.

Answer:

We have to write an exponential function for the growth of rabbits at the rate of 11 % every year.

The exponential function is written as,  f(x)  =  a(1+r)^x

Here the constant a will be 100 as there are originally 100
rabbits in the park, and r is the percentage increase in the decimal form.

The value r will be the percentage growth in decimal form, the decimal form is given by

11 %  =  \(\frac{11}{100}\)

11 % = 0 .11

Thus the function will be written as

f(x) = 100(1 + 0.11)^x

f(x) = 100 × (1.11)^x

The exponential function for the rabbits in the park with a growth percent of 11 will be written as f(x) = 100 × (1.11)^x

 

Page 167  Exercise 27  Problem  30

Question 30.

Using the given table, determine whether the function is an exponential growth or decay function by calculating the percentage change in y for each unit increase in x.

The table is as follows:

1. Calculate the percentage change in y from x = −1 to x = 0.
2. Calculate the percentage change in y from x=0 to x = 1.
3. Calculate the percentage change in y from x = 1 to x = 2.
4. Determine if the function represents exponential growth or decay based on the percentage changes.
5. State the percentage rate of decay.

Answer:

We have to check using the given table whether the given function is an exponential growth or decay function:

If the value increases/decreases by a constant percentage it will be a exponential growth/decay function respectively.

From x  =  −1 to x = 0

The value of y changes from 160 to 40

The percentage change is :

P =  \(\frac{40-160}{160} \times 100\)

we get percentage change as −75 %

From x = 0to x = 1

The value of y changes from 40 to 10

The percentage change is:

P = \(\frac{10-40}{40} \times 100\)

We get percentage change as −75 %

From x = 1 to x = 2

The value of y changes from 10 to 2.5

The percentage change is :

P = \(\frac{2.5-10}{10} \times 100\)

The percentage change is − 75 %

Since the percentage change is constant and is in negative the function is an exponential decay function.

The function is an exponential decay function according to the given table and the percentage rate of decay is 75 %

Big Ideas Math Integrated Math  Chapter 4 Maintaining Mathematical Proficiency

 

Page 90  Exercise 1  Problem 1

Question 1.

Given the graph below, identify the coordinates of point A.

1. Determine the x-coordinate of point A by finding the perpendicular distance from the y-axis.
2. Determine the y-coordinate of point A by finding the perpendicular distance from the x-axis.
3. Write the ordered pair that corresponds to point A.

Answer:

we will find the x-coordinate of a point & the y-coordinate of a point by getting perpendicular distances from the axis.

Point A

The x-coordinate of A: 2

The y-coordinate of A: 6

The ordered pair corresponds to point A is (2,6)

The ordered pair corresponds to point A is (2,6)

 

Page 90  Exercise 2  Problem 2

Question 2.

Given the graph below, identify the coordinates of point E.

1. Determine the x-coordinate of point E by finding the perpendicular distance from the y-axis.
2. Determine the y-coordinate of point E by finding the perpendicular distance from the x-axis.
3. Write the ordered pair that corresponds to point E.

Answer:

We will find the x-coordinate of a point & the y-coordinate of a point by getting perpendicular distances from the axis.

point E

The x-coordinate of E: 0

The y-coordinate of E: − 4

The ordered pair corresponds to point E is (0,−4)

The ordered pair corresponds to point E is (0,−4)

 

Page 90  Exercise 3  Problem 3

Question 3.

Given the graph below, identify which points are located in Quadrant III of the Cartesian coordinate system.

1. List all the points from the graph.
2. Determine which points are located in Quadrant III.
3. Write the coordinates of the point(s) that are located in Quadrant III.

Answer:

Cartesian coordinates system

We will check which points in the given graph is in Quadrant III

Points in III Quadrant

F is only in III Quadrant

F is only in III Quadrant

 

Page 90  Exercise 4  Problem 4

Question 4.

Given the graph below, identify which points are located in Quadrant IV of the Cartesian coordinate system.

1. List all the points from the graph.
2. Determine which points are located in Quadrant IV.
3. Write the coordinates of the point(s) that are located in Quadrant IV.

Answer:

Cartesian coordinates system

We will check which points in the given graph is in Quadrant IV

Points in IV Quadrant

D is only in IV Quadrant

D is only in IV Quadrant

 

Page 90  Exercise 5  Problem 5

Question 5.

Given the graph below, identify which points are located on the negative x-axis of the Cartesian coordinate system.

1. List all the points from the graph.
2. Determine which points are located on the negative x-axis.
3. Write the coordinates of the point(s) that are located on the negative x-axis.

Answer:

Cartesian coordinates system

We will check which points are located on the negative x-axis

Points are located on the negative x-axis

G is the only point located on the negative x-axis

G is the only point located on the negative x-axis

 

Page 90  Exercise 6  Problem 6

Question 6.

Solve the equation x – y = -12 for the variable y.

Answer:

Given

x − y = −12

By using the isolation of variable y of the given equation, we will solve the equation for y.

x−y = −12

Subtracting x to both sides

x − y − x = −12−x

−y = − 12− x

Dividing by -1 on both sides

y = 12 + x

Solution of x − y = −12 for  y = 12 + x

 

Page 90  Exercise 7  Problem 7

Question 7.

Solve the equation 8x + 4y = 16 for the variable y.

Answer:

Given

8x + 4y = 16

By using the isolation of variable y of the given equation, we will solve the equation for y.

8x + 4y = 16

Dividing by 4 on both sides

\(\frac{8x+4y}{4}=\frac{16}{4}\)

2x + y = 4

Subtracting 2x  from both sides

2x + y −2x = 4 − 2x

y = 4 − 2x

Solution of  8x + 4y = 16 for   y = 4 − 2x

 

Page 90  Exercise 8  Problem 8

Question 8.

Solve the equation 3x – 5y + 15 = 0 for the variable y.

Answer:

Given

3x−5y + 15 = 0

By using the isolation of variable y of the given equation, we will solve the equation for y.

3x − 5y + 15 = 0

Adding 5y on both sides

3x − 5y + 15 + 5y = 0 + 5y

3x + 15 = 5y

Dividing by 5 on both sides

\(\frac{3x+15}{5}\)= y

Interchanging both sides

y = \(\frac{3x+15}{5}\)

Solution of 3x−5y+15=0 for y = \(\frac{3x+15}{5}\)

 

Page 90  Exercise 9  Problem 9

Question 9.

Solve the equation 0 = 3y – 6x + 12 for the variable y.

Answer:

Given

0 = 3y−6x+12

By using the isolation of variable y of the given equation, we will solve the equation for y.

0 = 3y − 6x + 12

Subtracting 3y from both sides

0 − 3y = 3y − 6x + 12 − 3y

−3y = −6x + 12

Dividing by −3 both sides

\(\frac{−3y}{−3}\)=\(\frac{−6x+12}{−3}\)

y = 2x − 4

Solution of 0 = 3y − 6x + 12 for y = 2x − 4

 

Page 91 Exercise 10  Problem 10

Question 10.

Given two points (0,-1) and (2,3) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line. Finally, plot the line and identify its slope and y-intercept.

Answer:

Given

(0,-1) and (2,3)

From the given graph, we can find the slope of the line where 2 points are known using slope & given point we will find both intercept of line

The slope of the line from points (0,−1) & (2,3)

m = \(\frac{−1−3}{0−2}\)

m = 2

y = 2x + c

Putting point (2,3) in the equation

3 = 2 × 2 + c

c = −1

Equation of line y = 2x − 1

Plot

y-Interceptc = −1 , Slope m = 2, Liney = 2x−1

 

Page 91 Exercise 10  Problem 11

Question 11.

Given two points (0,2) and (4,-2) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line. Finally, plot the line and identify its slope and y-intercept.

Answer:

Given

(0,2) and (4,-2)

From the given graph, we can find the slope of the line where 2 points(0,2)&(4,−2) are known using slope & given point we will find both intercepts of the line

The slope of the line from points (0,2) & (4,−2)

m = \(\frac{−2−2}{4−0}\)

m = −1

y = −x + c

Putting point(0,2) in the equation

2 = 0 + c

c = 2

Equation of line y = −x + 2

Plot

y-Intercept c = 2 , Slope m = −1, Line y = −x + 2

 

Page 91 Exercise 10  Problem 12

Question 12.

Given two points (−3,3) and (3,−1) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line. Finally, plot the line and identify its slope and y-intercept.

Answer:

Given

(−3,3) and (3,−1)

From the given graph, we can find the slope of the line where 2 points (−3,3) & (3,−1)are known using slope & given point we will find both intercept of line

The slope of the line from points (−3,3)&(3,−1)

m = \(\frac{−1−3}{3+3}\)

m = \(\frac{−4}{6}\)

m = \(\frac{−2}{3}\)

y= \(\frac{−2}{3}\) x + c

From(3,−1)

−1 = \(\frac{−2}{3}\) (3) + c

Cancellation to 3

−1= −2+c

c = 2−1

c = 1

y = \(\frac{−2}{3}\)x+1

Plot

y-Intercept c=1, Slope m = \(\frac{−2}{3}\), Line y = \(\frac{−2}{3}\) x + 1

 

Page 91  Exercise 10  Problem 13

Question 13.

Given two points (4,0) and (2,−1) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line. Finally, plot the line and identify its slope and y-intercept.

Answer:

Given

(4,0) and (2,−1)

From the given graph, we can find the slope of the line where 2 points(4,0)&(2,−1) are known using slope and given point we will find both intercepts of line

The slope of the line from points (4,0) & (2,−1)

Slope m = \(\frac{−1−0}{2−4}\)

m = \(\frac{1}{2}\)

y=\(\frac{1}{2}\)x + c

From point (4,0)

0 = \(\frac{1}{2}\)(4) + c

c = −2

y = \(\frac{x}{2}\) − 2

Plot

y-Intercept c = −2, Slope m = \(\frac{1}{2}\), Line y = \(\frac{x}{2}\) − 2

 

Page 92  Exercise 11  Problem 14

Question 14.

Given two points (0,20) and (2000,80) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

Given

(0,20) and (2000,80)

From the given graph, we can find the slope of the line where 2 points (0,20) & (2000,80)are known using slope & given point we will find both intercepts of line

The slope of the line from points (0,20) & (2000,80)

m = \(\frac{3}{100}\)

y-Intercept

c = 20

Using these

y = \(\frac{3}{100}\) x+20

Equation of a given line y = \(\frac{3}{100}\) x+20

 

Page 94  Exercise 12  Problem 15

Question 15.

Find the equation of a line given its slope m = 2 and y-intercept c = −3

Answer:

Given

m = 2 and y-intercept c = −3

Using slope & y-intercept we will find the equation of the line

y = mx + c

Putting m & c values

y = 2x − 3

Equation of the line y = 2x − 3

 

Page 94 Exercise 12 problem 16

Question 16.

Find the equation of a line given its slope m = −3 and y-intercept c = 7.

Answer:

Given

m = −3 and y-intercept c = 7.

Using slope & y-intercept we will find the equation of the line

y = mx + c

Putting m & c values

y =−3x + 7

Equation of the line  y = −3x + 7

 

Page 94  Exercise 13  Problem 17

Question 17.

Find the equation of a line given its slope \(m = /frac{1}{3}\) and y-intecept c = 2.

Answer:

Given

\(m = /frac{1}{3}\) and y-intecept c = 2.

Using slope & y-intercept we will find the equation of the line

y = mx + c

Putting m & c values

y = \(\frac{1}{3}\) x + 2

Equation of the line y = \(\frac{1}{3}\) x + 2

 

Page 94  Exercise 14  Problem 18

Question 18.

Given two points (0,0) and (2,4) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (0,0) & (2,4)

Slope  m = \(\frac{4−0}{2−0}\)

m = 2

y = 2x + c

Using (0,0)

c = 0

y = 2x

Equation of the line in slope-intercept form y = 2x

 

Page 94 Exercise 15 Problem 19

Question 19.

Given two points (0,5) and (3,−4) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (0,5) & (3,-4)

Slope  m = \(\frac{−4−5}{3−0}\)

m = −3

y = −3x + c

Using point (0,5)

5 = 0 + c

c = 5

y = −3x + 5

Equation of the line in the slope-intercept form y = −3x + 5

 

Page 94  Exercise 16  Problem 20

Question 20.

Given two points (−2,−3) and (0,−2) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points(-2,-3) & (0,-2)

Slope  m = \(\frac{−2+3}{0+2}\)

m = \(\frac{1}{2}\)

y = \(\frac{1}{2}\)x + c

Using point (0,-2)

c = −2

y = \(\frac{1}{2}\) x−2

Equation of the line in slope-intercept form y = \(\frac{1}{2}\) x−2

 

Page 94 Exercise 17 Problem 21

Question 21.

Given two points (0,3) and (4,0) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (0,3) & (4,0)

Slope m = \(\frac{−3}{4}\)

y = \(\frac{−3}{4}\)x+c

Using (0,3)

c = 3

y = \(\frac{−3}{4}\) x + 3

Equation of the line in slope-intercept form y = \(\frac{−3}{4}\) x + 3

 

Page 95  Exercise 18  Problem 22

Question 22.

Given two points (2,1) and (0,−7) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of the y-intercept by satisfying that point

Given points (2,1) & (0,-7)

Slope m = \(\frac{−7−1}{0−2}\)

m = \(\frac{−8}{−2}\)

m = 4

y = 4x+c

Using point (0,-7)

c = −7

y = 4x − 7

Equation of the line in slope-intercept form y = 4x − 7

 

Page 95  Exercise 19 Problem 23

Question 23.

Given two points (0,2) and (4,3) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (0,2) & (4,3)

Slope m = \(\frac{3−2}{4}\)

m = \(\frac{1}{4}\)

y = \(\frac{1}{4}\)x + c

Using point (0,2)

c = 2

y= \(\frac{1}{4}\) x+2

Equation of the line in slope-intercept form y = \(\frac{1}{4}\) x + 2

 

Page 95  Exercise 20  Problem 24

Question 24.

Given two points (8,0) and (0,8) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of the y-intercept by satisfying that point

Given points (8,0) & (0,8)

Slope m = \(\frac{−8}{8}\)

m = −1

y = −x + c

Using (0,8)

c = 8

y = −x + 8

Equation of the line in slope-intercept form y = −x + 8

 

Page 95  Exercise 21  Problem 25

Question 25.

Given two points (0,3) and (2,−5) on a graph, find the slope of the line passing through these points. Using the slope and one of the points, determine the y-intercept and write the equation of the line.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (0,3) & (2,-5)

Slope m = \(\frac{−5−3}{2−0}\)

m = −4

y = −4x + c

Using point (0,3)

c = 3

y = −4x + 3

Equation of the line in the slope-intercept form y = −4x + 3

 

Page 95  Exercise 22  Problem 26

Question 26.

Given the linear function f(x)= c + dx and the following conditions:

1. f(0) = 5
2. f(9) = -4

Determine the values of the constants c and d. Write the final equation of the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

f(x) = c + dx

Given f(0) = 5

f(0) = c

c = 5

f (9) = c + 9d

−4 = 5 + 9d

d = \(\frac{−9}{9}\)

d = −1

f(x) = 5 − x

A linear function f with the given values, f(x) = 5 − x

 

Page 95  Exercise 23  Problem 27

Question 27.

Given the linear function f(x)= c + dx and the following conditions:

1. f(0) = 10
2. f(7) = -4

Determine the values of the constants c and d. Write the final equation of the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

Given f(0) = 10

f(0) = c

c = 10

f(7) = c + 7d

−4 = 10 + 7d

d = \(\frac{−14}{7}\)

d = −2

f(x) = 10−2x

A linear function f with the given values f(x) = 10 − 2x

 

Page 95  Exercise 24  Problem 28

Question 28.

Given the linear function f(x)= c + dx and the following conditions:

1. f(0) = 2
2. f(-2) = -2

Determine the values of the constants c and d. Write the final equation of the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

f(x) = c + dx

Given f(0) = 2

f(0) = c

c = 2

f(−2) = c−2d

−2 = 2 −2d

d = \(\frac{−4}{−2}\)

d = 2

f(x) = 2 + 2d

A linear function f with the given values f(x) = 2 + 2d

 

Page 95  Exercise 25  Problem 29

Question 29.

An electrician charges a fixed initial fee and an hourly rate for their services. The total cost function f(x) is given by f(x)=c+dx, where x is the number of hours worked.

Given the following conditions:

1. The initial fee (when x=0) is 50.
2. After 4 hours of work, the total cost is 190.

Determine the values of the constants c and d. Write the final linear model that represents the total cost as a function of the number of hours worked.

Answer:

f(x) = c + dx in this f(x) is electrician charges function of hours of work x

We will put given conditions in the linear function f(x) = c + dx by which we will get values of constants (c,d)

f(x) = c + dx  , At x = 0 initially

f(0) = c

50 = c

After 4 hours of work

f(4) = c + 4d

190 = 50 + 4d

4d = 140

d = 35

f(x) = 50 + 35x

A linear model that represents the total cost as a function of the number of hours worked. f(x) = 50 + 35x

 

Page 95  Exercise 25  Problem 30

Question 30.

An electrician charges a fixed initial fee and an hourly rate for their services. The total cost function f(x) is given by f(x)= 50 + 35x, where x is the number of hours worked.

1. Identify the slope (or gradient) of the linear function.
2. Determine the electrician’s charge per hour.

Answer:

In the function that we find d is the charges per hour which is the gradient(slope) of the linear function

Linear model

In this slope or gradient is 35

The electrician charge per hour = 35

The electrician charge per hour = 35

Page 96  Exercise 26  Problem 31

Question 31.

Given the slope m and a point (x₁,y₁) through which a line passes, use the point-slope form of the equation of a line to find the equation of the line.

1. The slope m=4
2. The point (x₁,y₁) = (2,3)

Write the equation of the line using the point-slope form y −y₁ =m(x−x₁).

Answer:

Given slope & point, we will put them in the equation of the line when one point & slope is given

y − y₁  =  m(x−x₁)  by putting slope & one point values in this equation we get the equation of line

Equation of line when slope & one point is given y−y₁ =  m(x−x₁)

 

Page 96  Exercise  27  Problem 32

Question 32.

Given the slope m and a point (x₁,y₁) through which a line passes, use the point-slope form of the equation of a line to find the equation of the line.

1. The slope m = \(\frac{1}{2}\)
2. The point (x₁,y₁) = (3,2)

Answer:

The slope is given & from the given graph we will get the points & use them to find the equation by putting them in the slope-point form of the equation

Slope m = \(\frac{1}{2}\)

Given point (3,2)

Using the slop-point form of the equation

y−y₁ = m(x−x₁)

y−2 = \(\frac{1}{2}\) (x−3)

Simplifying  2y − 4 = x−3

2y  =  x + 1

Equation of the line 2y = x + 1

 

Page 96  Exercise 28   Problem 33

Question 33.

Given the slope m=−2 and a point (−4,6) through which the line passes, use the point-slope form of the equation of a line to find the equation of the line. Then simplify the equation to the standard form.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation

Slope m =−2

Given point (-4,6) using the slop-point form of the equation

y−y₁ = m(x−x₁)

y−6 = −2(x + 4)

Simplifying

y−6 = −2x−8

y + 2x + 2 = 0

Equation of the line y + 2x + 2 = 0

 

Page 96  Exercise 29  Problem 34

Question 34.

Given the slope m and a point (x₁,y₁) through which the line passes, use the point-slope form of the equation of a line to find the equation of the line.

1. Find the equation of the line given the following conditions:
   • Slope: m = 3
   • Point: (2,−1)
2. Find the equation of the line given the following conditions:
   • Slope: m = \(-\frac{1}{2}\)
   • Point: (4,5)

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equatio

Slope is m

Given point (x₁,y₁)

Equation of the line when one point & slope is given

y − y₁ = m(x−x₁)

Equation of the line y−y₁ = m(x − x₁)

 

Page 97  Exercise 30  Problem 35

Question 35.

Given the slope m=25 representing the savings rate in dollars per month and a point (4,175) representing the savings after 4 months, use the point-slope form of the equation to find the equation of the line that models the savings over time.

1. Write a point-slope from the equation y – y₁ = m(x-x₁) using the given slope and points
2. Simplify the equation to the slope-intercept form.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation

Given slope gradient of saving is $25 / month

m = 25

Given point (4,175)

y−y₁= m(x−x₁)

y−175 = 25(x−4)

Simplifying

y − 175 = 25x−100

y = 25x + 75

where y is saving & x is number of months

The equation that represents the balance A after x months. y = 25x + 75

 

Page 97  Exercise 30  Problem 36

Question 36.

Given the slope m=4 and the y-intercept 175, find the equation of the line in slope-intercept form and plot it on a graph to verify if it matches the given Plot.

Answer:

The slope is given & from the given graph we will get the point & use them to find the equation by putting them in the slope-point form of the equation.

We will plot equation on the graph & match with given plot

y = 4x + 175

Plotted graph is same as given

 

Page 97  Exercise 31  Problem 37

Question 37. Given the slope

m = 3 and a y-intercept = 175, use the slope-intercept form of the equation to find the equation of the line that models the given scenario.

1. Identify the slope and y-intercept:
   • Given: m = 4
   • y-intercept = 175
2. Write the slope-intercept form of the equation y = mx + b using the given slope and y-intercept.
3. Plot the equation on a graph and verify if it matches the given plot.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation.

y−y₁ = m(x−x₁) by putting slope & one point values in this equation we get the equation of line

Equation of line when slope & one point is given  y−y₁ = m(x−x₁)

 

Page 97   Exercise  31  Problem 38

Question 38.

Given the slope m = 5 and a point (0,0) which is the origin, use the point-slope form of the equation to find the equation of the line.

1. Identify the slope and point:
   • Given: m = 5
   • (x₁,y₁) = (0,0)
2. Write the point-slope form of the equation y-y₁ = m(x-x₁) using the given slope and point.
3. Simplify the equation to the slope-intercept form.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation

Let’s assume m = 5 & given point is the origin

Equation of the line when one point & slope is given

y−y₁ = m(x−x₁)

y−0 =  5(x−0)

y = 5x

An equation of a line when you are given the  Slope m = 5 and a Point(0,0) on the line  y = mx

 

Page 99  Exercise  32  Problem 39

Question 39.

Given the slope m = -1 and a point (-2,1), use the point-slope from of the equation to find the equation of the line.

1. Identify the slope and pont:
   • Given: m = -1
   • (x₁,y₁) = (-2,1)
2. Write the point-slope form of the equation y−y₁ = m(x−x₁) using the given slope and point.
3. Simplify the equation to the standard form.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation

Given slope m = −1

Point(-2,1) equation of the line when one point & slope is given

y−y₁ =  m(x−x₁)

y−1 = −(x+2)

y = −x−1

Equation of a line  y = −x−1

 

Page 99   Exercise 32  Problem 40

Question 40.

Given the slope m = \(\frac{4}{3}\) and a point (-2,1), use the point-slope form of the equation to find the equation of the line.

1. Identify the slope and point:
   • Given: m = \(\frac{4}{3}\)
   • (x₁,y₁) = (-2,1)
2. Write the point-slope form of the equation y−y₁ = m(x−x₁) using the given slope and point.
3. Simplify the equation to the standard form.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation

Given slope

m = \(\frac{4}{3}\)

Point(-2,1) equation of the line when one point & slope is given

y−y₁ = m(x−x₁)

y−1 = \(\frac{4}{3}\) (x+2)

3y−3 = 4x + 8

3y = 4x + 11

Equation of a line 3y = 4x + 11

 

Page 99  Exercise 33  Problem 41

Question 41.

Given the slope m = \(\frac{1}{2}\) and a point (-2,1), use the point-slope from of the equation to find the equation of the line.

1. Identify the slope and pont:
   • Given: m = \(\frac{1}{2}\)
   • (x₁,y₁) = (-2,1)
2. Write the point-slope form of the equation y−y₁ = m(x−x₁) using the given slope and point.
3. Simplify the equation to the standard form.

Answer:

The slope is given & from the given graph we will get the point & use them to find equation by putting them in the slope-point form of equation

Given slope

m = − \(\frac{−1}{2}\)

Point(-2,1) equation of the line when one point & slope is given

y−y₁ =  m(x−x₁)

y−1 = −\(\frac{−1}{2}\) (x+2)

2y−2 = −x−2

2y = −x

Equation of line 2y = −x

 

Page 99   Exercise 34   Problem 42

Question 42.

Given the points (1, 2) and (4, -1), find the equation of the line in slope-intercept form.

1. Calculate the slope m using the given points \(m=\frac{y_2-y_1}{x_2-x_1}\) where (x₁,y₁) = (1,2) and (x₂,y₂) = (4,-1)
2. Write the equation of the line using the point-slope from y-y₁ = m(x-x₁) and simplify it to slope-intercept from y = mx + c.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (1,2) & (4,-1)

Slope m = \(\frac{2+1}{1−4}\)

m = −1

y = −x+c using point (1,2)

2 = −1+c

c = 3

y = −x + 3

Equation of the line in slope-intercept form y = −x + 3

 

Page 99  Exercise 35  Problem 43

Question 43.

Given the points (−4,1) and (4,−1), find the equation of the line in slope-intercept form.

1. Calculate the slope m using the given points: \(m=\frac{y_2-y_1}{x_2-x_1}\) where (x₁,y₁) = (-4,1) and (x₂,y₂) = (4,-1)
2. Write the equation of the line using the point-slope from y-y₁ = m(x-x₁) and simplify it to slope-intercept from y = mx + c.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of the y-intercept by satisfying that point

Given points (−4,1) & (4,−1)

m = \(\frac{1+1}{−4−4}\)

m = \(\frac{−1}{4}\)

y = \(\frac{−1}{4}\) x+c

Using point (4,-1)

−1 = \(\frac{−1}{4}\) × 4 + c

c = 0

y = \(\frac{−1}{4}\) x

Equation of the line in slope-intercept form y = \(\frac{−1}{4}\) x

 

Page 99  Exercise 36  Problem 44

Question 44.

Given points (-1,-9) and (1,-3), find the equation of the line in slope-intercept form.

1. Calculate the slope m using the given points: \(m=\frac{y_2-y_1}{x_2-x_1}\) where (x₁,y₁) = (-1,-9) and (x₂,y₂) = (1,-3)
2. Write the equation of the line using the slope-intercept form y = mx + c and determine the value of c by substituting one of the given points.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point

Given points (-1,-9) & (1,-3)

Slope m = \(\frac{−3+9}{1+1}\)

m = 3

y = 3x + c

Using point (1,-3) −3 = 3 × 1 + c

c = −6

y = 3x − 6

Equation of the line in slope-intercept form y = 3x − 6

 

Page 99  Exercise 37  Problem 45

Question 45.

Given the points (1,2) and (2,0), find the equation of the line in slope-intercept form.

1. Calculate the slope m using the given points: \(m=\frac{y_2-y_1}{x_2-x_1}\) where (x₁,y₁) = (1,2) and (x₂,y₂) = (2,0)
2. Write the equation of the line using the point-slope from y-y₁ = m(x-x₁) and simplify it to slope-intercept from y = mx + c.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of the y-intercept by satisfying that point

Given points (1,2) & (2,0)

Slope m = \(\frac{2−0}{1−2}\)

m = −2

y = −2x + c

Using point (2,0)

0 = −2 × 2 + c

c = 4

y = −2x + 4

Equation of the line in the slope-intercept form y = −2x + 4

 

Page 100  Exercise 38  Problem 46

Question 46.

Given the linear function f(x)= c+dx and the following conditions:

1. f(1) = 7
2. f(-2) = 1

Find the values of the constants c and d and write the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

We will solve 2 equation to get 2 unknowns (c,d)

f(x) = c + dx

Using given conditions

f(1) = c + d

7 = c + d

f(−2) = c−2d

1 = c−2d

Solving both equations d = 2 & c = 5

f(x) = 5 + 2x

A linear function f with the given values f(x) = 5+2x

 

Page 100  Exercise 39  Problem 47

Question 47.

Given the linear function f(x)= c+dx and the following conditions:

1. f(−1) = 2
2. f(3) = 3

Find the values of the constants c and d and write the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

We will solve 2 equation to get 2 unknowns (c,d)

f(x) = c + dx

Using given conditions

f(−1) = c−d

2 = c−d

f(3) = c + 3d

3 = c + 3d

Solving both equations

d = \(\frac{1}{4}\) &c = \(\frac{9}{4}\)

f(x)= \(\frac{9}{4}\)+\(\frac{1}{4}\)x

A linear function f with the given values f(x)= \(\frac{9}{4}\)+\(\frac{1}{4}\)x

 

Page 100  Exercise 40  Problem 48

Question 48.

Given the linear function f(x)= c+dx and the following conditions:

1. f(0) = -2
2. f(4) = -1

Find the values of the constants c and d and write the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

We will solve 2 equation to get 2 unknowns (c,d)

f(x) = c + dx

Using given conditions

f(0) = c

−2 = c

f(4) = c + 4d

−1 = −2 + 4d

d = \(\frac{1}{4}\)

f(x) = −2  + \(\frac{1}{4}\)x

A linear function f with the given values f(x) = −2+\(\frac{1}{4}\)x

 

Page 100  Exercise 41  Problem 49

Question 49.

Given the linear function f(x)= c+dx and the following conditions:

1. f(3) = 5
2. f(2) = 6

Find the values of the constants c and d and write the linear function.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d).

We will solve 2 equation to get 2 unknowns (c,d).

f(x) = c + dx

Using given conditions

f(3) = c + 3d​

5 = c + 3d

f(2)= c + 2d

6 = c + 2d

Solving both equations

d = −1 & c =  8

f(x) = 8−x

A linear function f with the given values f(x) = 8−x

 

Page 100  Exercise 42  Problem 50

Question 50.

Given a set of points (−3,−98) and (−1,18), determine if the data in the table can be modeled by a linear equation. Follow these steps:

1. Find the slope (m) of the line passing through the given points.
2. Use the slope and one of the points to find the y-intercept (c).
3. Write the equation of the line in slope-intercept form.
4. Check if the equation satisfies another point from the data table to confirm if the data can be modeled by a linear equation.

Answer:

We will get the slope from the given points & put the slope value in the line equation.

By using the known slope equation we use one point to get the value of y-intercept by satisfying that point.

After getting we will check whether given data satisfy the equation, if yes then the data in the table can be modeled by a linear equation

Equation from points (-3,-98) & (-1,18)

Slope

m = \(\frac{18+98}{−1+3}\)

m = 58

y = 58x + c

From point (-1,18)

18 =−58 + c

y = 58x + 76

y = 58x + 76

For 0

y = 58 × 0 + 76

y = 76 not on line data in the table can not be modeled by a linear equation

Data in the table can not be modeled by a linear equation

 

Page 100  Exercise 43  Problem 51

Question 51.

Given the conditions, we need to create a linear function D(h) representing the miles driven after h hours. We are given that the gradient of the distance (d) is 60 miles per hour, and at h=3, the distance D=265 miles.

1. Determine the linear function D(h) that represents the miles driven after h hours.
2. Calculate the odometer reading after 7 hours of continuous driving.

Answer:

We will put given conditions in the linear function by which we will get values of constants (c,d)

After using values of h we can get D corresponding h using the function

D(h) = c + dh

Where given gradient of distance d = 60

At h = 3 D=265

Using these D(h) = c + 60h

265 = c + 60 × 3

c = 85

Function D(h) = 85 + 60h

D(h) = 85 + 60h

At h = 7

D(h) = 85 + 60 × 7

D(7) = 505

Linear function D that represents the miles driven after h hours   D(h) = 85 + 60h , The odometer read after 7 hours of continuous driving  D(7) = 505