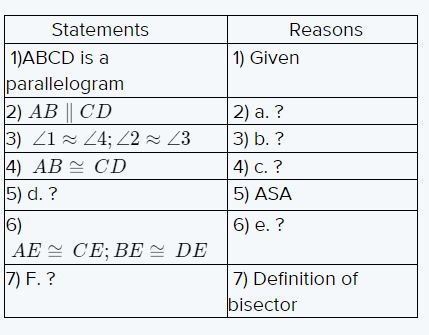
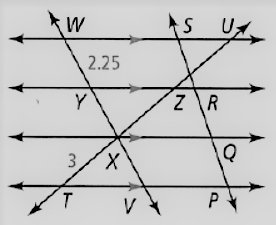
Savvas Learning Co

Savvas Learning Co Geometry Student Edition Chapter 9 Transformations Exercise 9.1 Translations

 

Page 548  Exercise 1  Problem 1

Given: △JPT→ΔJ′P′T′

To find – The image of P

To find the image of \(\overline{T J}\).

In geometry, a translation is the shifting of a figure from one place to another without rotating, reflecting or changing its size.

Given that , △JPT → △J′P′T′

In translation, it is valid to list the points of the preimage and image in the same order, where we use the notation prime to mark the preimage.

Therefore, we see that in △JPT mark of the point P is in the second place, and in the △J′P′T′
in the second place is the mark of the point P′ , so we have that the image of P is point P′.

Considering what we said in the previous step, we see that the image of is \(\overline{T J}\) line \(\overline{T′ J′}\) .

The image of P is point P′, The image of \(\overline{T J}\) is line \(\overline{T′ J′}\).

 

Page 548  Exercise 2  Problem 2

Given: (x ,y)  →   (x − 3, y − 4)

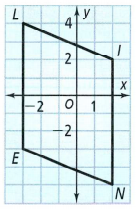
To graph the image of NILE for the translation (x ,y) → (x − 3,y − 4)

Using the method of graphing.

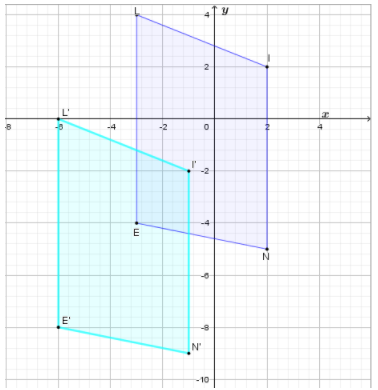
The given image of NILE is

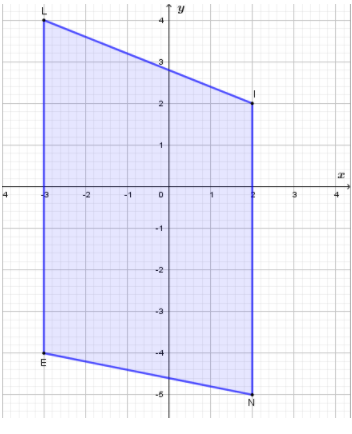
Identify the coordinates for each vertex:

N(2,−5) , I(2,2), L(−3,4), E(−3,−4)

Use the translation rule that is given to us to find the coordinates of each vertex of the image.

Our translation rule is ,  (x ,y) → (x − 3,y − 4)

For point N the image is, (2,−5 ) → (2 − 3, −5 − 4)

When we simplify, the image is point N′ (−1,−9).

Identify the coordinates for each vertex:

N(2,−5), I(2,2), L(−3,4), E(−3,−4)

Use the translation rule that is given to us to find the coordinates of each vertex of the image.

Our translation rule is

(x ,y) → (x − 3,y − 4)

For point N the image is: (2,−5) → (2 − 3,−5 − 4)

When we simplify, the image is point N′ (−1,−9).

For point I the image is: (2,2) → (2 − 3, 2 − 4)

When we simplify, the image is point I′ (−1,−2).

For point L the image is: (−3,4) → (−3 − 3, 4 − 4)

When we simplify, the image is point L′ (−6,0).

For point E the image is: (−3,−4)→(−3−3,−4−4)

When we simplify, the image is point E′(−6,−8).

To graph the image, first we graph points: N′,I′,L′ and E′.

Then draw \(\overline{N’ I’}\), \(\overline{I’ L’}\), \(\overline{L’ E’}\), \(\overline{E’ N’}\).

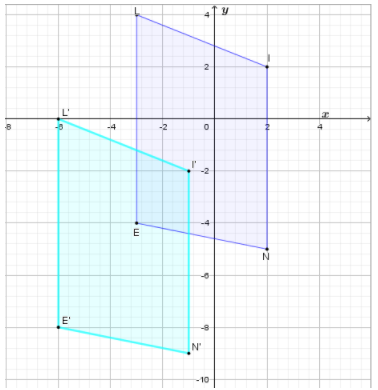
The image of NILE for the given translation is

 

Page 548  Exercise 3  Problem 3

Given: Point H(x ,y) moves 12 units left and4 units up

The given graph is

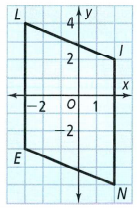
To find – The rule that describes this given translation.

In geometry, a translation is the shifting of a figure from one place to another without rotating, reflecting or changing its size.

Given point H(x ,y).

This point moves 12 units to the left and 4 units up.

Move it 12 units to the left

It is like we subtract 12 from x coordinate, and when we move it4 units up it is like we add 4 to y coordinate.

Based on the previous step, the translation rule is (x ,y)→(x − 12,y + 4).

The translation rule is (x ,y) → (x − 12,y + 4).

 

Page 548  Exercise 4  Problem 4

Given:

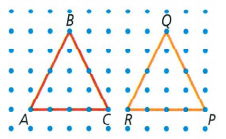
To explain and correct her error.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Given a sketch

Friend says that transformation △ABC → △PQR is a translation.

The image of A is R, image of B is Q , image of C is P.

Based on that, the transformation that is translation is , △ABC → △RQP

A friend’s mistake is that for a transformation that is rotation, he said it is translation.

Friend’s mistake is that for a transformation that is rotation, he said it is translation.

 

Page 548  Exercise 5  Problem 5

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

To write translation (x ,y) → (x + 1,y − 3) as a composition of a horizontal and vertical translation.

First, the(x ,y) translates to (x + 1,y)

This represents a horizontal translation.

Then, (x + 1, y) translates to (x + 1,y − 3)

This represents a vertical translation.

The(x ,y) translates to (x + 1, y).

This represents horizontal translation. (x + 1, y) translates to (x + 1,y − 3). This represents vertical translation.

 

Page 549  Exercise 6  Problem 6

Given:

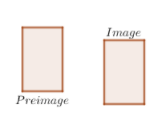
A sequence of transformations is a set of translations, rotations, reflections, and dilations on a figure.

Based on the sketch, we see that preimage and image are congruent.

Therefore, this transformation is isometry.

The given preimage and image are congruent, so this transformation is isometry.

 

Page 549  Exercise 7  Problem 7

Given:

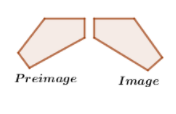
A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Based on the sketch.

The preimage and image are congruent, so this transformation is isometry.

The preimage and image are congruent, so this transformation is isometry.

 

Page 549  Exercise 8  Problem 8

Given:

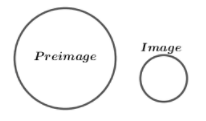
A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Based on the sketch.

The preimage and image are not congruent, because we have a change in size.

Therefore, this transformation is not isometry.

The preimage and image are not congruent, because we have a change in size so the transformation is not isometry.

 

Page 549  Exercise 9  Problem 9

Given:

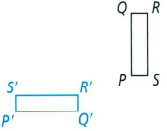
To choose an angle or point from the preimage and name its image.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

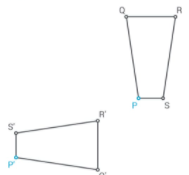
The image of the point p from the preimage is p​′

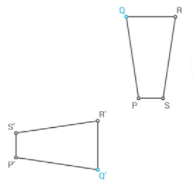
The image of the point Q from the preimage is Q′

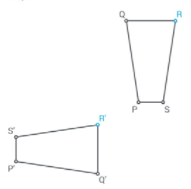
The image of the point R from the preimage is R′

The image of the point S from the preimage is S′

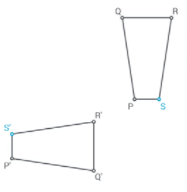
The image of the point p from the preimage is p′, The image of the point Q from the preimage is Q′, The image of the point R from the preimage is R′, The image of the point S from the preimage is S′.

 

Page 549 Exercise 9  Problem 10

Given:

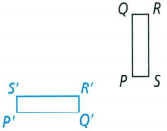
To list all pairs of corresponding sides.

Using the method of geometrical transformation.

The matching side to \(\overline{P Q}\) is \(\overline{P’ Q’}\)

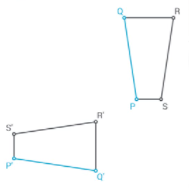
The matching side to \(\overline{Q R}\) is \(\overline{Q’ R’}\)

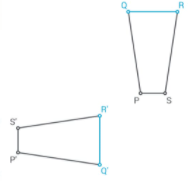
The matching side to \(\overline{R S}\) is \(\overline{R’ S’}\)

 

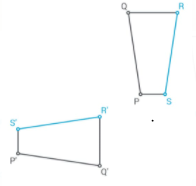
The matching side to \(\overline{S P}\) is \(\overline{S’ P’}\)

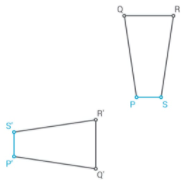
The matching sides are

\(\overline{P Q}\) and \(\overline{P’ Q’}\)

\(\overline{Q R}\) and \(\overline{Q’ R’}\)

\(\overline{R S}\) and \(\overline{R’ S’}\)

\(\overline{S P}\) and \(\overline{S’ P’}\).

 

Page 549  Exercise 10  Problem 11

Given: (x ,y) → (x − 2,y + 5)

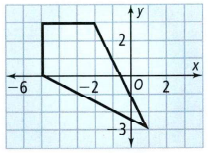
To graph the image of each figure under the given translation.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

The given a preimage, a rectangle, whose vertices we will mark with A,B,C and D

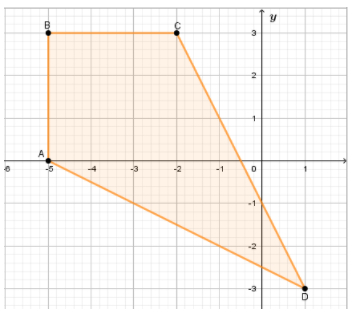
To identify the coordinate of each vertex

A(−5,0), B(−5,3), C(−2,3), D(1,−3)

Use the translation rule that is given to us to find the coordinates of each vertex of the image.

Our translation rule is (x ,y) → (x − 2, y + 5)

For point A the image is: (−5,0) → (−5 − 2, 0 + 5)

When we simplify, the image is point A′(−7,5).

For point B the image is: (−5,3) → (−5 − 2,3 + 5)

When we simplify, the image is point B′(−7,8).

For point C the image is: (−2,3) → (−2 − 2,3 + 5)

When we simplify, the image is point B′ (−4,8).

For point D the image is: (1,−3) → (1 − 2,−3 + 5)

When we simplify, the image is point D′ (−1,2).

To graph the image, first we graph points: A′ , B’, C’, and D’

The draw \(\overline{A^{\prime} B^{\prime}}, \overline{B^{\prime} C^{\prime}}, \overline{C^{\prime} D^{\prime}} \text { and } \overline{D^{\prime} A^{\prime}}\)

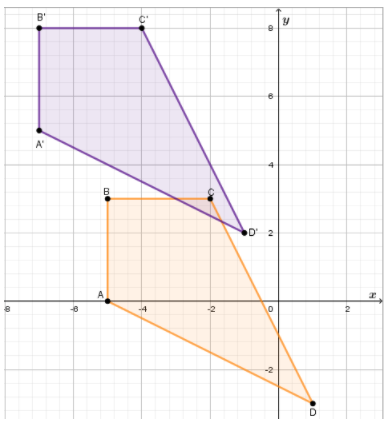
The graph of the image of each figure under the given translation is

 

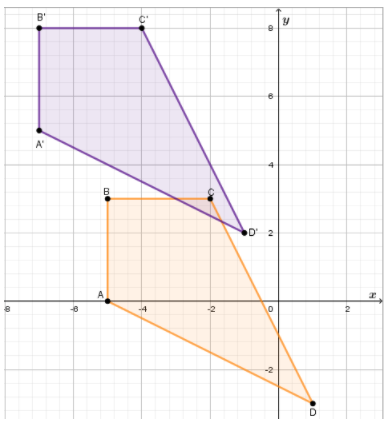
Page 549  Exercise 11  Problem 12

Given:

To write a rule to describe each translation.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

The vertices of the preimage are A(−7,−5) ,B(−3,−5) and C(−3,1).

Compare the r = \(\sqrt{(x-4)^2+(y-0)^2+(z-z)^2}\)  and y coordinates of the points A(−7,−5) and its image A′ (−6,−6).

The r = \(\sqrt{(x-4)^2+(y-0)^2+(z-z)^2}\) coordinate of the image A′ is 1 unit right from the point A’−6−(−7) = 1

It follows that the horizontal change is: x ⟶ x + 1

The y coordinate of the image A′ is 1 unit down from the point A ,−6 − (−5) = −1

It follows that the vertical change is: y ⟶ y − 1

The rule for the translation of a point (x ,y) 1 unit right and 1 unit down is

T_<1,_−1> (x ,y) = (x + 1,y − 1)

The rule should be valid for every vertex.

T_<1,_−1>(x ,y) = (x + 1, y − 1)

Check if the rule is valid for B(−3,−5) and its image B′ by inserting x = −3 and y = −5

T_<1,_−1> (−3,−5) = (−3 + 1, −5 − 1)

Calculate the coordinates

T<1,−1> (−3,−5) = (−2,−6)

Since the image B′ has coordinates (−2,−6) , it follows that the rule is valid for this vertex.

T<1,−1>(x ,y) = (x + 1,y − 1)

Check if the rule is valid for C(−3,1) and its image B′ by inserting x = −3 and y = 1

T_<1,_−1>(−3,1) = (−3 + 1,1 − 1)

Calculate the coordinates

T_<1,_−1>(−3,1) = (−2,0)

Since the image C′ has coordinates (−2,0), it follows that the rule is valid for this vertex.

The translation rule is T_<1,_−1>(x ,y).

The translation rule is T_<1,_−1>(x ,y).

 

Page 549  Exercise 12 Problem 13

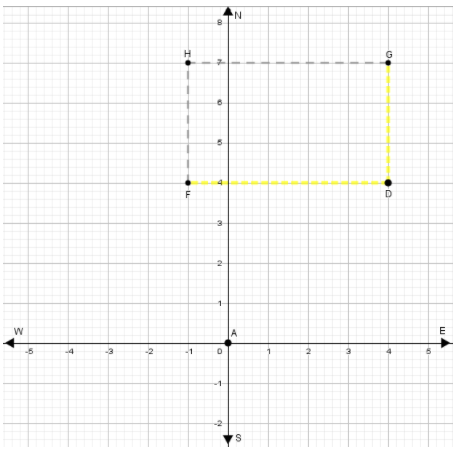
Given: Walk 4 blocks east and 4 blocks north to the Wells Fargo History Museum. 

Walk 5 blocks west and 3 blocks north to the Cable Car Barn Museum.

To find where is the Cable Car Barn Museum in relation to your hotel.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Based on the given data, Point A(0,0) represents the position of the hotel

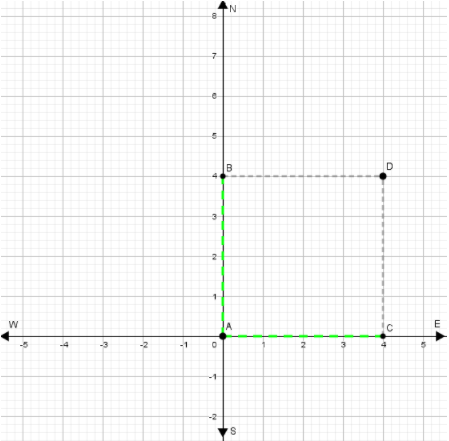
 When you go 4 blocks east (which is represented by AC ) and 4 blocks north (which is represented by AB) we see that we get to the point D(4,4)which represents the position of Wells Fargo History Museum.(0,0) → (0 + 4,0 + 4)

When we simplify, we get D(4,4), as we said.

Then we from point D move 5blocks west (which is represented by DF) and 3 blocks west (which is represented by DG) and we see that we get to the point  H(−1,7) which represents the position of Cable Car Barn Museum.(4,4) → (4  − 5 ,4 + 3)

When we simplify, we get H(−1,7), as we said.

To find the Cable Car Barn Museum in relation to hotel, that is, we need to see where point H is in relation to point A.

When we go east 1 block (which is represented by HI ) and 7 blocks south (which is represented by HJ ), we get to point A.

(−1,7)→(−1 + 1,7 − 7)

When we simplify, we get A(0,0), as we said.

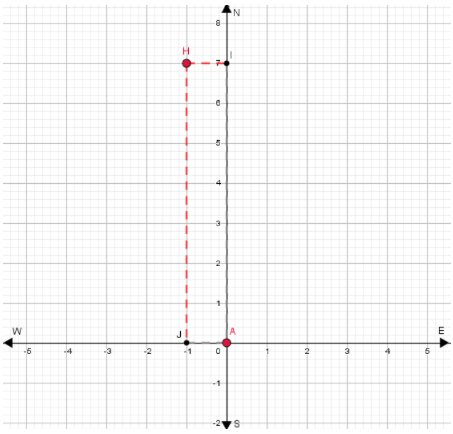
Based on the previous step, we see that the Cable Car Barn Museum is 1 block west and 7 blocks north in relation to hotel.

The Cable Car Barn Museum is 1 block west and 7 blocks north.

 

Page 550  Exercise 13  Problem 14

Given:  hey leave their home in Enid, Oklahoma, and drive to Tulsa, which is 107 mi east and 18 mi south of Enid.

From Tulsa, they go to Norman,83 mi west and 63 mi south of Tulsa.

To find – Where is Norman in relation to Enid.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Based on the given data, Point O(0,0) represent the position of home, Enid.

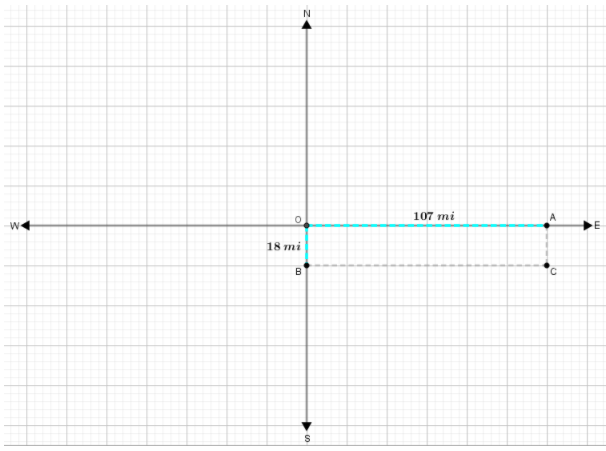
When you go 107mi east (which is represented by OA ) and 18 mi south (which is represented by OB) we see that we get to the point C(107,−18) which represents the position of Tulsa.

(0,0)→(0 + 107,0 − 18) When we simplify, we get C(107, − 18)

Then we from point C move 83 mi west (which is represented by CD ) and 63 mi south (which is represented by CF) and we see that we get to the point G(24,−81)which represents the position of Norman. (107,−18) → (107 − 83,− 18 − 63)

When we simplify, we get G (24, −   81)

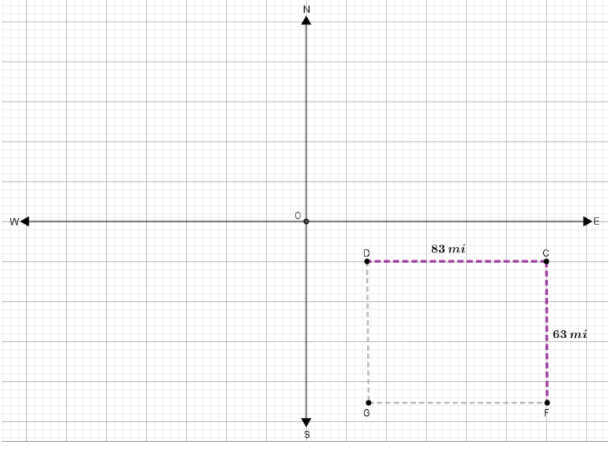
To find where is the Norman in relation to Enid, that is, we need to see where point G is in relation to point O.

When we go west 24mi (which is represented by GH ) and 81 mi north (which is represented by GI), we get to point O.

(24 ,− 81) → (24 − 24, −81 + 81)

When we simplify, we get O(0,0)

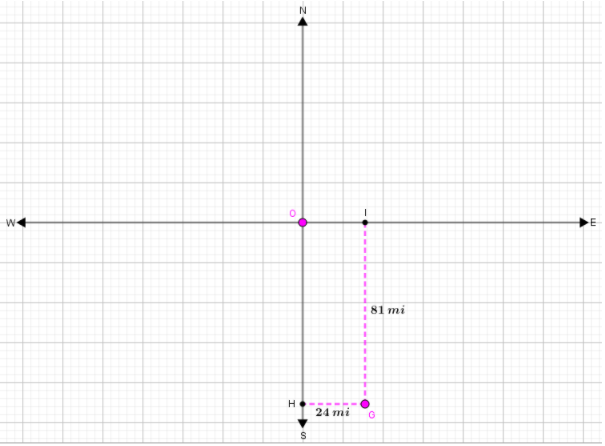
Based on the previous step, the Norman is 24 mi east and 81 mi south in relation to Enid.

The Norman is 24 mi east and 81 mi south in relation to Enid.

 

Page 550  Exercise 14  Problem 15

Given :

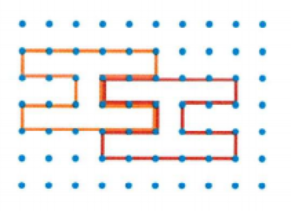
Write a rule that describes the translation.

The given translation is

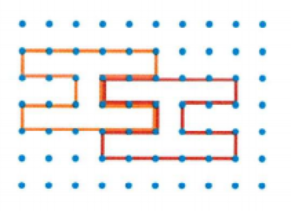
Here the orange figure is the translation image of the red figure

Hence the translation rule is (x ,y) → (x − 3,y + 1)

Therefore the required rule for the translation is (x ,y) n→ (x − 3,y + 1).

 

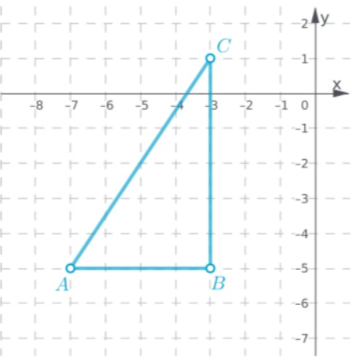
Page 550   Exercise 15   Problem 16

Given: △MUG has coordinates  M(2,−4), U(6,6) , and G(7,2).

A translation maps point M to M′ (−3,6).

To find the coordinates of U′ and G′ for this translation.

To find how can you use a graph to help you visualize the problem.

To find how can you find a rule that describes the translation.

Based on the given data, the graph is

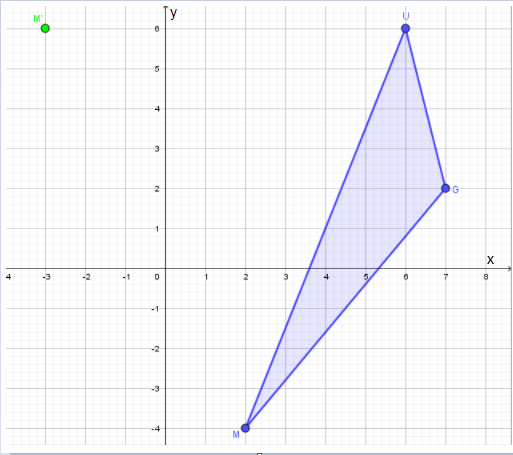
Given △MUG and that translation maps point  M(2,−4) to point M′ (−3,6).

Our assignment is to find the coordinates of U′ and G′ , that is, the coordinates of the points that are images of points U and G.

In order to find this coordinates, we will use points M and M′ to find the translation rule.

Then we will use the rule to find required coordinates.

Let’s look at the sketch.

If we move 5 units left from point M (Which is represented by MA ) and 10 units up from point M ( Which is represented by MB ) we see that we would reach to the point M′.

So, the x coordinate is reduced by 5 (Because we are going left) and the y coordinate is increased by 10

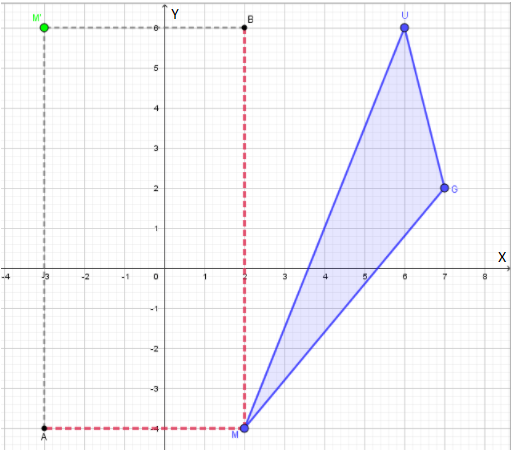
Based on the previous step, our translation rule is

(x ,y)→ (x − 5, y+  10)

For point U(6,6) the image is: (6,6) → (6 − 5,6 + 10)

When we simplify, the image is point U′ (1,16).

For point G(7,2) the image is: (7,2) → (7 − 5, 2 + 10)

When we simplify, the image is point G′ (2,12).

So, we have, U′ (1,16) G′(2,12)

Sketch a graph and enter the data given we can see how a point is translated (whether it goes left or right, up or down) or if we have a translation rule we can use it to find preimages.

When we have an image and a preimage, sketch a graph and observe the movement of the point that is the image to the point of the image that is the preimage.

Depending on whether the point moves left or right, up or down and how much, we conclude how much the coordinates of the point that represents the image decrease or increase, and that is how we find the translation rule.

The coordinates of U′ (1,16) and  G′(2,12)

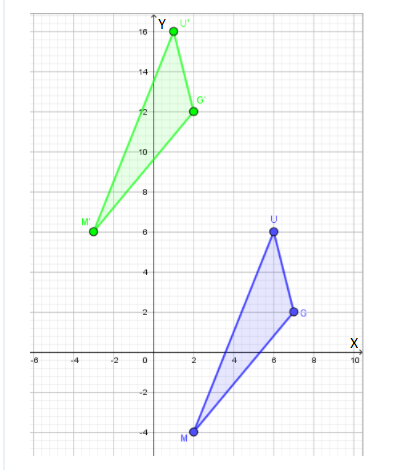
Sketch a graph and enter the data given we can see how a point is translated (whether it goes left or right, up or down) or if we have a translation rule we can use it to find preimages.

When we have an image and a preimage, sketch a graph and observe the movement of the point that is the image to the point of the image that is the preimage.

Depending on whether the point moves left or right, up or down and how much.

 We conclude how much the coordinates of the point that represents the image decrease or increase, and that is how we find the translation rule.

 

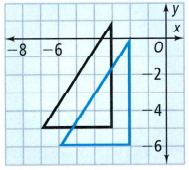
Page 550  Exercise 16  Problem 17

Given: (x ,y) → (x − 3,y − 5)

To use the rectangle and (x ,y)→ (x + 3,y + 1) to draw a box.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Given a trapeze, whose vertices we will mark with A,B,C and D

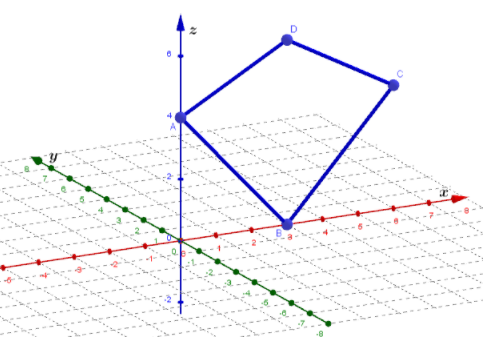
The coordinates of the points A,B,C and D with respect to the two-dimensional coordinate system x Or  y are

A(0,0), B(3,0), C(6,0), D(3,0)

The translation rule is

(x ,y) → (x − 3,y − 5)

To find the images of A,B,C and D.

For point A(0,0) the preimage is: (0,0) → (0 − 3,0 − 5)

When we simplify, the preimage is point A′(−3,−5).

For point B(3,0) the preimage is: (3,0) → (3 − 3,0 − 5)

When we simplify, the preimage is point B′ (0,−5).

For point C(6,0) the preimage is: (6,0) → (6 − 3,0 − 5)

When we simplify, the preimage is point C′(3,−5).

For point D(3,0) the preimage is: (3,0) → (3 − 3, 0 − 5)

When we simplify, the preimage is point D′(0,−5).

Points A and Care in the plane z = 4,point Bis in the plane z = 0, point Dis in the plane z = 6, and that is why we switch to three-dimensional space.

So we find the points: A′(−3,−5,4) , B′(0,−5,0), C′(3,−5,4), D′(0,−5,6)

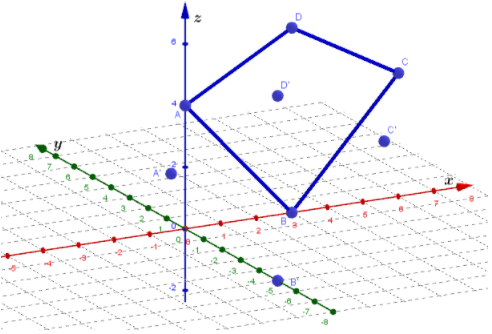
To draw \(\overline{A A^{\prime}}, \overline{B B^{\prime}}, \overline{C C^{\prime}} \text { and } \overline{D D^{\prime}} \text {. }\)

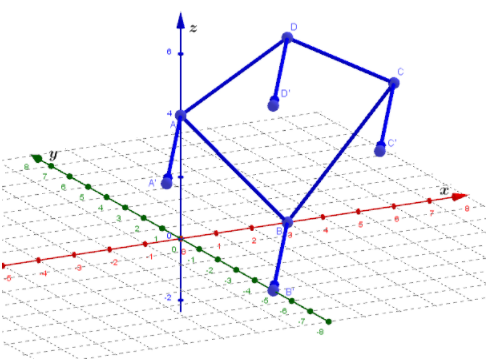
To connect points and we have our three-dimensional figure

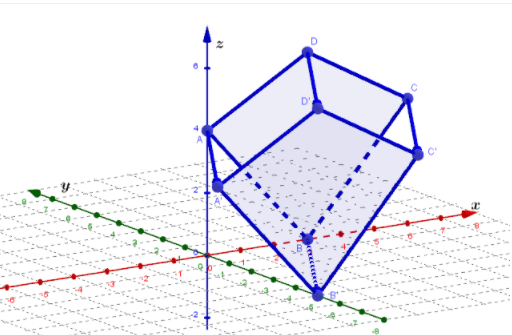
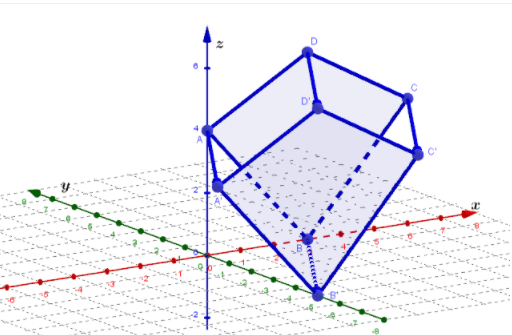
Using the rectangle and (x ,y) → (x + 3,y + 1) the box is

 

Page 550  Exercise 17  Problem 18

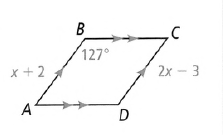
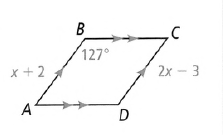
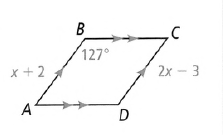
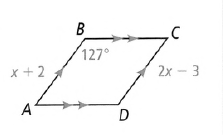
Draw a parallelogram ABCD

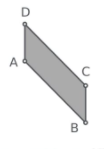
Draw a reflection line on the right of the parallelogram that is parallel to one of the sides.

Reflect points A,B,C, and D.

Draw a parallelogram A′B′C′D′.

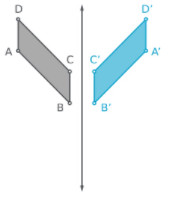
Translate parallelograms ABCD and A′B′C′D′ downwards for a little bit more than BC.

Draw another two parallelograms and Repeat transformations to make a pattern.

The translations are

 

 Page 550  Exercise 18  Problem 19

Given: Translation (x ,y) → (x + 5, y + 7) maps △MNO onto △M′ N′O′

To determine translation rule maps △M′N′O′ onto △MNO.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

In the assignment we are given translation, (x ,y) → (x + 5, y + 7)

This translation maps △MNO onto △M′N′O′.

For example, take some random triangle, like one on the sketch below.

We then used the given translation and we sketched the image, △M′N′O′.

For example, at point O, we see that we have moved7units up ( which is represented by OA) and 5 units to the left ( which is represented by OB ) and we reached to the point O′.

If we want to get back from the point O′ to the point O we need to move 7 units down ( which is represented by O′B) and 5units to the right ( which is represented by O′A) and we reached to the point O.

If we checked for the remaining two points, we would get the same.

The graph is

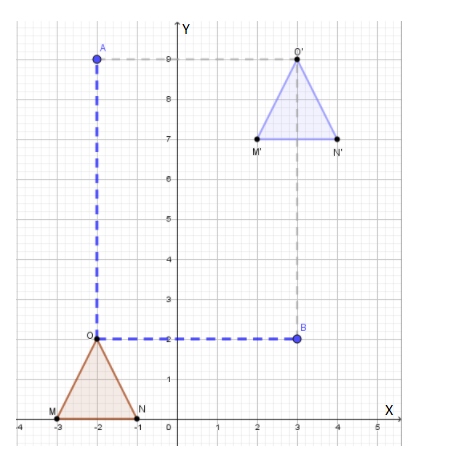
The translation that maps △M′N′O′ onto △MNO is (x ,y) → (x − 5,y − 7).

The translation that maps △M′N′O′ onto △MNO is (x ,y) → (x − 5,y − 7).

 

Page 550  Exercise 19  Problem 20

Given:

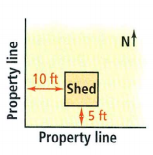
To describe how to move the shed to comply with the law.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Given

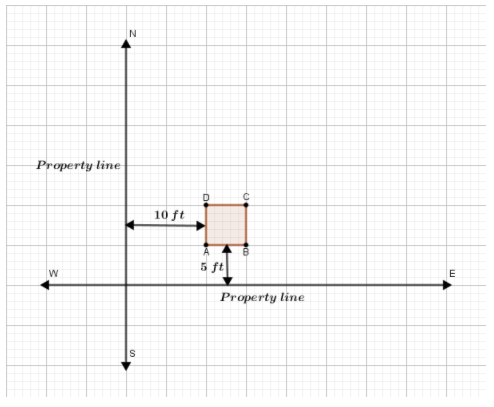
If we move points A,B,C and D5 ft east ( to the right) and 10ft north (upwards), we would get to the points

To mark with A′,B′,C′ and D′

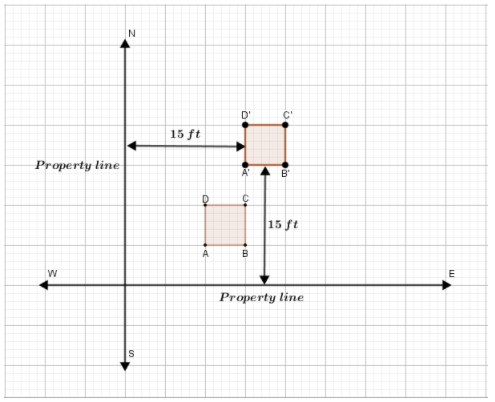
We see that □ A′B′C′D′ satisfies the law.

Used to satisfy the law is the translation of the shed.

The rule of the mentioned translation is (x ,y) → (x + 5, y + 10).

The rule of the mentioned translation is( x ,y)→(x + 5,y + 10).

 

Page 550  Exercise 20  Problem 21

Given: (x ,y) → (x + 5, y + 7) followed by (x ,y) → (x − 9, y − 2).

The program makes the letter W by connecting the points 1, 2, (2,0), 3, 2, (4,0) and (5,2)

To find the points does the program connect to make the last W.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

Given that when program connects to points (1,2),(2,0)(3,2)(4,0) and (5,2) a letter W appears.

Our assignment is to find the point that program connects to, to make the last appearance of the letter W.

The given translations are, (x ,y) → (x + 5,y + 7), (x ,y) → (x − 9 ,y − 2)

The problem can solve in two ways.

The first way is to use the first translation and find the point where the letter W is repeated for the first time, and then use the second translation to that point that we found and find the point where W appears for the last time.

The second way is to present these two translations as one translation in the following way.

Use this translation: (x ,y) → (x + 5, y + 7).

Have (x + 5,y + 7) and we use the second translation on this point so we have, (x + 5,y + 7) → (x + 5 − 9,y + 7 − 2)

When we simplify, we have (x − 4, y + 5).

The new translation that leads us directly to the point that connected with our program shows the last W is, (x ,y) → (x − 4, y + 5)

We will mark point (1,2) with A.

For this point find the required point in both ways.

If we move 5 unit to the right ( which is presented with AF ) and 7 ,units up ( which is presented with AG ) we would reach point A₁ , where letter W appears the first time.

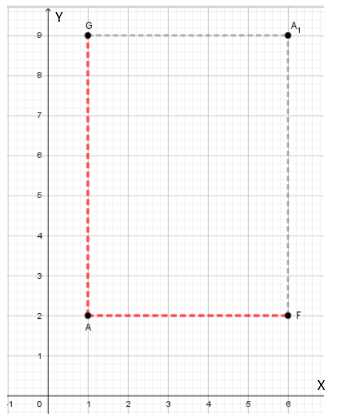
(1,2) → (1 + 5,2 + 7), When we simplify, we get  A₁ (6,9).

At point A₁ . move 9 unit to the left ( which is presented with A₁H ) and 2 units down ( which is presented with A₁ I) we would reach point A′, where letter W appears the second time.

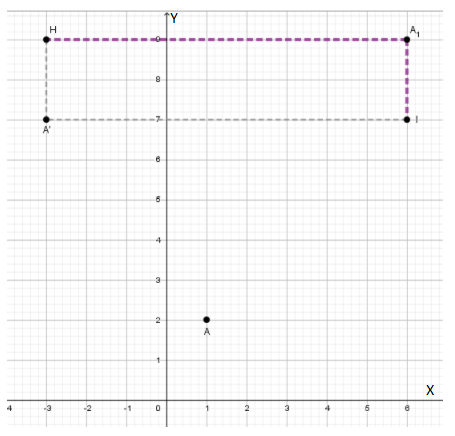
(6,9) → (6 − 9,9 − 2) , When we simplify, we get,  A′(−3,7).

At point A₁ . move 9 unit to the left ( which is presented with A₁ H) and 2 units down ( which is presented with A₁I) we would reach point A, where letter W appears the second time .(6,9) → (6 − 9, 9 − 2)

When we simplify, we get: A′ (−3,7).

If we use the new translation that leads us directly from point A(1,2) to required point, we would have, (1,2) → (1 − 4, 2 + 5)

Letter W shows the second time when point A′ (−3,7) is connected to the program.

We see that it is easier for us to use the second way to find the desired point, so we will use only the second way for the other points.

We will mark point (2,0) with B.

We use the new translation, that is, we move 4 unit to the left ( which is presented with BF₁ ) and 5 units up ( which is presented with BG₁ ) and we reach to the point B′ , where letter W appears the second time.

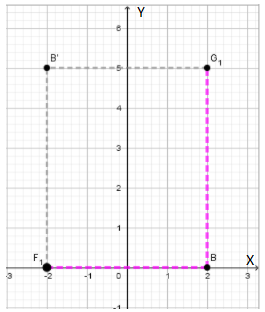
(2,0) → (2 − 4,0 + 5)

When we simplify, we get:  B′(−2,5).

Letter W shows the second time when point B′ (−2,5) is connected to the program

We will mark point (3,2) with C. 

We use the new translation, that is, we move 4 unit to the left ( which is presented with CG₂ ) and 5 units up (which is presented with CF₂ m) and we reach point C′ , where letter w appears the second time.

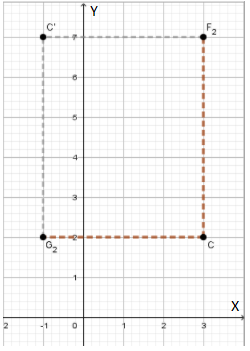
(3,2) → (3 − 4,2 + 5)

When we simplify, we get: C′ (−1,7).

Letter W shows the second time when point C′ (−1,7) is connected to the program.

 We will Mark point(4,0) with D .

We use the new translation, that is, we move 4 unit to the left ( which is presented with DF₃ ) and 5 units up (which is presented with DG₃) and we reach point D′ , where letter W appears the second time.

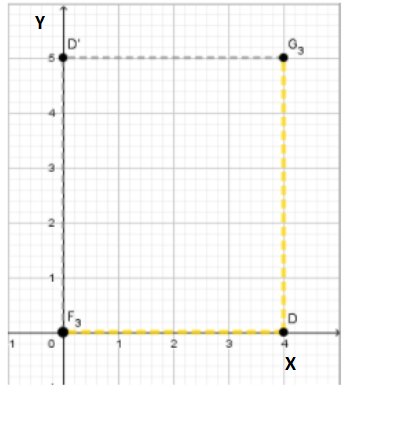
(4,0) → (4 − 4,0 + 5)

When we simplify, we get: D′ (0,5).

Letter W shows the second time when point D′ (0,5) is connected to the program.

We will mark point (5,2) with E.

We use the new translation, that is, we move 4 unit to the left ( which is presented with EG₄) and 5 units up (which is presented with EF₄) and we reach point E′, where letter W appears the second time.

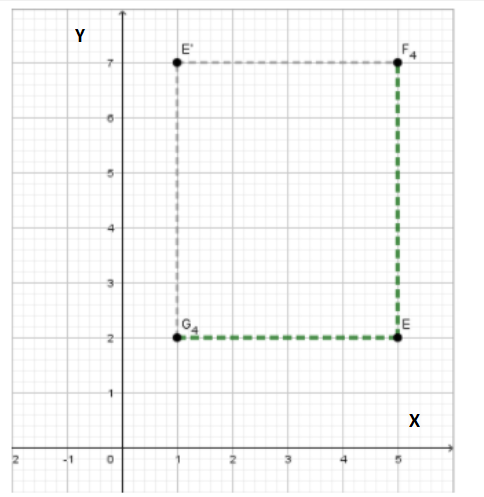
(5,2) → (5 − 4, 2 + 5)

When we simplify, we get: E′ (1,7).

Letter W shows the second time when point E′ (1,7) is connected to the program.

The required points are

A′(−3,7), B′(−2,5), C′(−1,7), D′(0,5), E′(1,7)

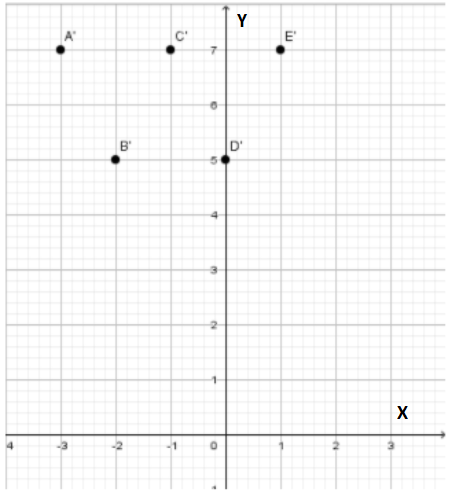
The points does the program connect to make the last W,(−3,7),(−2,5),(−1,7),(0,5)

 

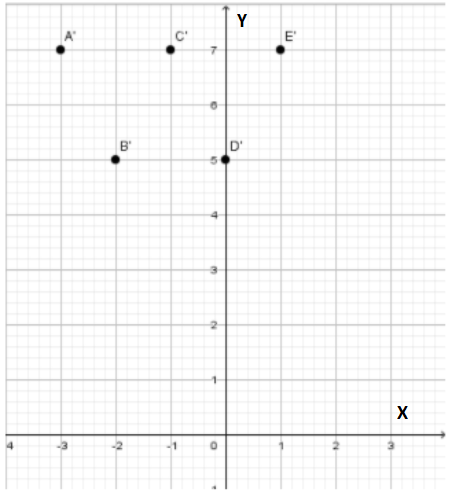
Page 551  Exercise 21  Problem 22

Given:

To write three different translation rules for which the image of △JKL has a vertex at the origin.

A transformation is a general term for four specific ways to manipulate the shape and/or position of a point, a line, or geometric figure.

The coordinates of the vertices of △KLM are, K(−4,1) , L(−2,1) , M(−4,4)

The image of a triangle to have one vertex at the origin, we observe which translation would satisfy the condition that, for example, the image of vertex L, which we will mark with L′ has coordinates (0,0) (which would mean that this point represents the origin).

Consider the point K.

If move 4 units to the right (which is presented with KA) and 1 unit down (which is presented with KB) we would reach to the point K′.

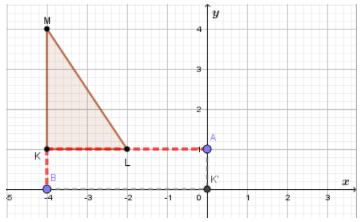
(−4,1)→(−4 + 4,1 − 1)

When we simplify, we have: K′ (0,0).

So, we have translation, (x ,y) → (x + 4,y − 1)

If we use translation (x ,y) → (x + 4, y−  1) to find image of △KLM we would get

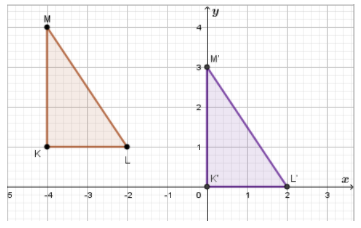
Use this translation, the image △K′L′M′ has one vertex at the origin, vertex K′.

Consider the point L.

If we move 2 units to the right (which is presented with LA₁) and 1 unit down (which is presented with LB₁) we would reach to the point L₁′.

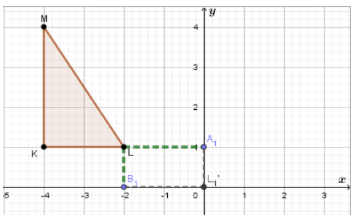
(−2,1) → (−2 + 2,1 − 1)

When we simplify, we have: L₁′(0,0).

So, we have translation, (x ,y) → (x + 2, y − 1)

If we use translation (x ,y) → (x + 2, y − 1) to find image of △KLM we would get

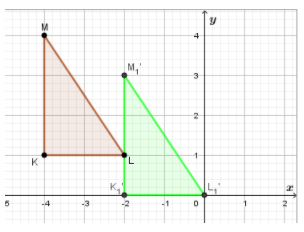
Use this translation, the image△K₁′L₁′M₁′has one vertex at the origin, vertex L₁′.

Consider the point M.

If we move 4 units to the right (which is presented with MA₂) and 4 unit down (which is presented with MB₂) we would reach to the point M₂′.

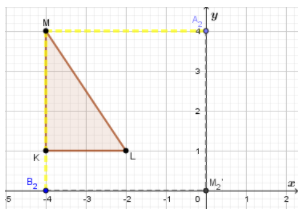
(−4,4) → (−4 + 4,4 − 4)

When we simplify, we have: M’₂(0,0)

So, the translation,(x ,y) → (x + 4,y − 4).

If we use translation (x ,y) → (x + 4, y − 4)to find image of △KLM

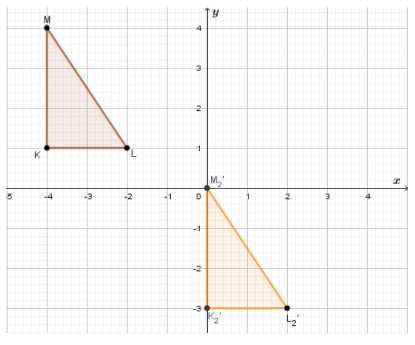
Use this translation, the image △K′₂L′₂M‘₂ has one vertex at the origin, vertex M′₂.

The required translations are, (x ,y) → (x + 4, y − 1)

(x ,y) → (x + 2, y − 1)

(x ,y) → (x + 4, y − 4)

The translations are

(x, y) → (x + 4, y − 1)

(x,y) → (x + 2, y − 1)

(x, y) → (x + 4, y − 4)

 

Page 551  Exercise 22  Problem 23

Given: (x ,y) → (x + 2, y + 5) followed by (x ,y) → (x − 4, y + 9).

To find a translation that has the same effect as each composition of translations.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

We have translations

(x ,y) → (x + 2,y + 5)

(x ,y) → (x − 4, y + 9)

Our assignment is to find a translation that would bring us to the same point that the composition of these translations would bring us to.
Suppose we have a point A(x ,y).

If we use translation (x , y) → (x + 2, y + 5) for point A, we would get

A(x , y) → (x + 2, y + 5)

When we simplify, we have: A₁ (x + 2, y + 5).

Now apply translation (x ,y) → (x − 4, y + 9) to point A₁, we have

(x + 2,y + 5) → (x + 2 − 4, y + 5 + 9)

When we simplify, we have: A′ (x − 2, y + 14)

Based on the previous step, the required translation is

(x ,y) → (x − 2, y + 14).

The translation is (x,y) → (x−2, y + 14).

 

Page 551  Exercise 23  Problem 24

Given: (x ,y) → (x + 12, y + 0.5) followed by (x, y) → (x + 1, y − 3).

To find a translation that has the same effect as each composition of translations.

A translation is a geometric transformation that moves every point of a figure, shape or space by the same distance in a given direction.

We have translations

(x ,y) → (x + 12, y + 0.5)

(x ,y) → (x + 1,y − 3)

Our assignment is to find a translation that would bring us to the same point that the composition of these translations would bring us to.

Suppose we have a point A(x ,y).

If we use translation (x ,y) → (x + 12, y + 0.5) for point A we would get

A(x ,y) → (x + 12, y + 0.5)

When we simplify, we have: A₁ (x + 12, y + 0.5).

When we now apply translation (x ,y) → (x + 1, y − 3)to point A1, we have

(x + 12, y + 0.5) → (x + 12 + 1, y + 0.5 − 3)

When we simplify, we have: A′(x + 13, y − 2.5)

Based on the previous step, we see that required translation is,(x ,y) → (x + 13, y − 2.5).

The translation is( x ,y)→ (x + 13, y − 2.5).

 

Page 551  Exercise 24  Problem 25

Given : △ABC has vertices A(−2,5), B(−4,−1), and C(2,−3).

To Find – The images of the midpoints of the sides of △ABC are the midpoints of the sides of △A′B′C′ for the translation (x ,y) → (x + 4, y + 2).

Translation means the displacement of a figure or a shape from one place to another.

In translation, a figure can move upward, downward, right, left or anywhere in the coordinate system.

In translation, only the position of the object changes, its size remains the same.

Based on the data from the assignment we have △ABC

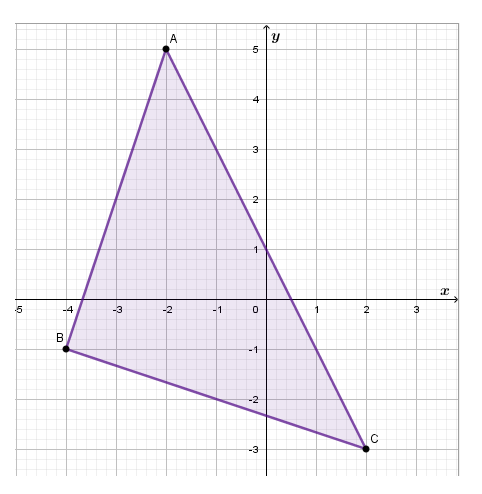
Our assignment is to prove that midpoints of sides \(\overline{A B}\) ,\(\overline{B C}\) and \(\overline{C A}\) are preimages of the sides of triangle △A′B′C′ , which represents the image of △ABC.

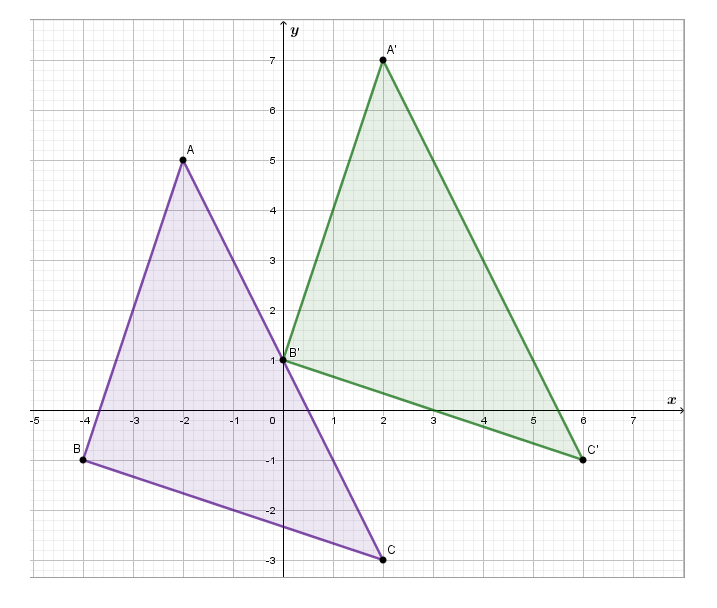
First we will use translation (x ,y)→(x + 4, y + 2) to find the image of △ABC.

For point A(−2,5) the image is: (−2,5) → (−2 + 4, 5 + 2)

When we simplify, the image is point A′ (2,7).

For point B(−4,−1) the image is: (−4,−1) → (−4 + 4,−1 + 2)

When we simplify, the image is point B′(0,1).

For point C(2,−3) the image is: (2,−3) → (2 + 4,−3 + 2)

When we simplify, the image is point C′(6,−1).

The image of △ABC is △A’B’C’.

To find the midpoints of the sides of a triangle we use:

In order to find the midpoint M of the line segment from point K(x₁,y₁) to point L(x₂ ,y₂ ) we use

⇒  M \(\left(\frac{x_1+x_2}{2}, \frac{y_1+y_2}{2}\right)\)

We find midpoint D of , \(\overline{A B}\) where A(−2,5),B(−4,−1)in the following way

⇒ \(D\left(\frac{-2+(-4)}{2}, \frac{5+(-1)}{2}\right)\)

Simplify

⇒ D \(\left(\frac{-6}{2}, \frac{4}{2}\right)\)

Simplify , D(-3,2)

We find midpoint E of BC \(\overline{B C}\) where B(−4,−1),C(2,−3)in the following way

⇒  E \(\left(\frac{-4+2}{2}, \frac{-1+(-3)}{2}\right)\)

Simplify 

⇒  E\(\left(\frac{-2}{2}, \frac{-4}{2}\right)\)

Simplify , E(-1,2)

We find midpoint F of \(\overline{C A}\) where C(2,−3),A(−2,5)in the following way

⇒  F\(\left(\frac{2+(-2)}{2}, \frac{-3+5}{2}\right)\)

Simplify

⇒  F \(\left(\frac{0}{2}, \frac{2}{2}\right)\)

Simplify , F (0,1)

We find midpoint D′ of \(\overline{A’ B’}\)where A′(2,7),B′(0,1)in the following way

D’\(\left(\frac{2+0}{2}, \frac{7+1}{2}\right)\)

Simplify

⇒  D’\(\left(\frac{2}{2}, \frac{8}{2}\right)\)

Simplify, D’ (1,4)

We find midpoint E′ of \(\overline{B’ C’}\) where B′ (0,1),C′(6,−1)in the following way

E′\(\left(\frac{0+6}{2}, \frac{1+(-1)}{2}\right)\)

Simplify

⇒  E’ \(\left(\frac{6}{2}, \frac{0}{2}\right)\)

Simplify , E'(3,0)

We find midpoint F′ of \(\overline{C’ A’}\), where C′ (6,−1),A′(2,7) in the following way

⇒    F′\(\left(\frac{6+2}{2}, \frac{-1+7}{2}\right)\)

Simplify

⇒   F′\(\left(\frac{8}{2}, \frac{6}{2}\right)\)

Simplify , F′(4,3)

Now we sketch all mid point that found and draw triangles △DEF is △D’E’F’

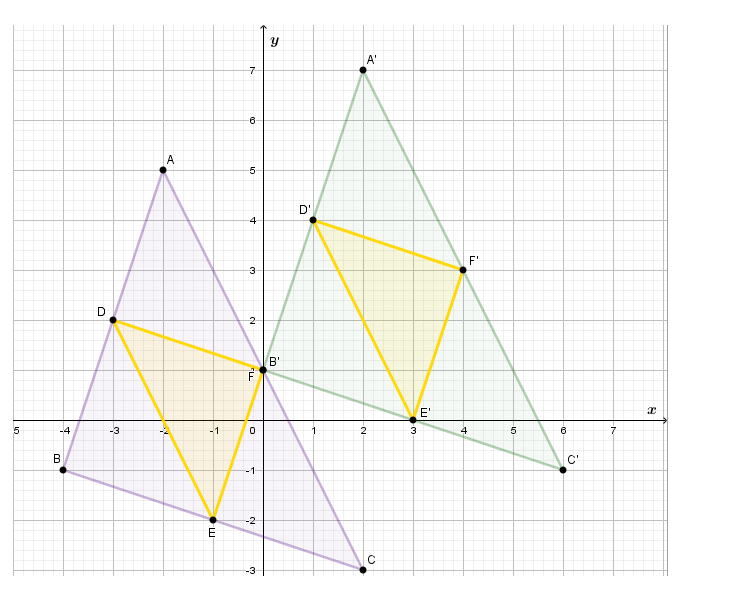
To prove what we are required to, we will show that .

When we apply a given translation to points D, E and F, we obtain points D′,E′ and  F′.

For point D(−3,2), which presents the midpoint of\(\overline{A B}\)

The image is (−3,2) → (−3 + 4,2 + 2)

When we simplify, the image is point D′(1,4), which we have shown to be the midpoint of \(\overline{A’ B’}\)

For point E (−1,−2), which presents the midpoint of \(\overline{B C}\) , 

The image is  (−1,−2) → (−1 + 4,−2 + 2)

When we simplify, the image is point E′(3,0), which we have shown to be the midpoint of \(\overline{B’ C’}\) .

For point F(0,−1), which presents the midpoint of \(\overline{C A}\)

The image is (0,1) → (0 + 4,1 + 2)

When we simplify, the image is point F′(4,3), which we have shown to be the midpoint of \(\overline{C’ A’}\).

It is shown that the images of the midpoints of the sides of △ABC are the midpoints of the sides of △A′B′C′ for the translation (x ,y) → (x + 4, y + 2).

 

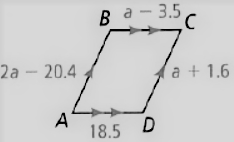
Page 551  Exercise 25  Problem 26

Given:

To Find –  How to use translations to draw a parallelogram.

Method used : Translation method.

A translation moves a shape up, down or from side to side but it does not change its appearance in any other way.

Translation is an example of a transformation.

Our assignment is to explain how to use translation to draw parallelogram.

We know that parallelogram is a quadrilateral with parallel and congruent opposite sides.

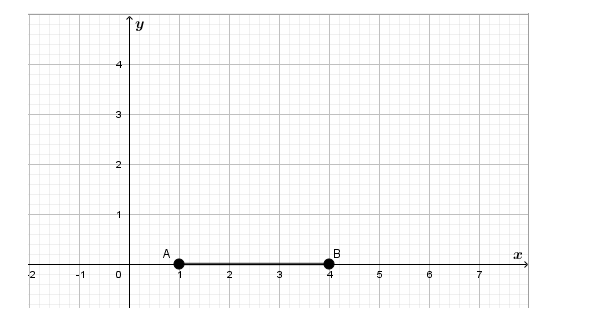
Suppose we have segment \(\overline{A B}\)

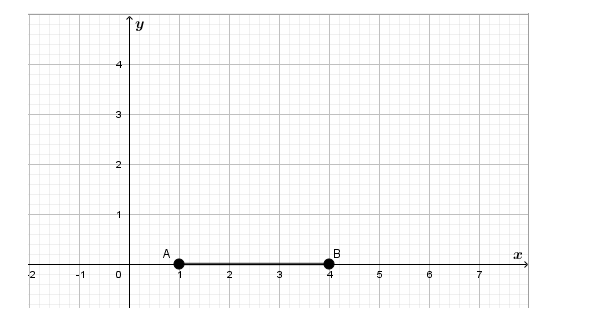
Now we want to use the translation to get the opposite parallel line \(\overline{A, B’}\).

The translation that satisfies this conditions is.

For example: (x ,y) → (x + 2, y + 4)

First let’s observe point A.

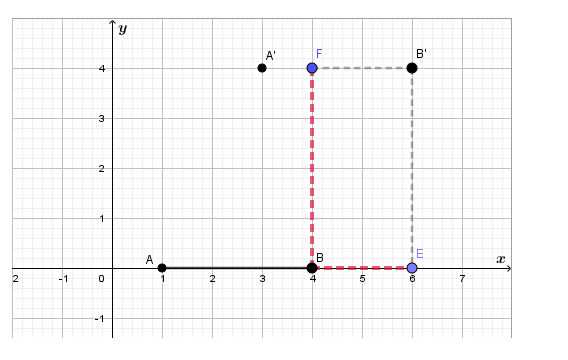
If we move 2 units to the right (which is presented with AC) and 4 units up (which is
presented with AC) we would reach to the point A’.

Now we observe point B.

If we move 2 units to the right (which is presented with BE) and 4 units up(which is Presented with BF) we would reach to the point B’.

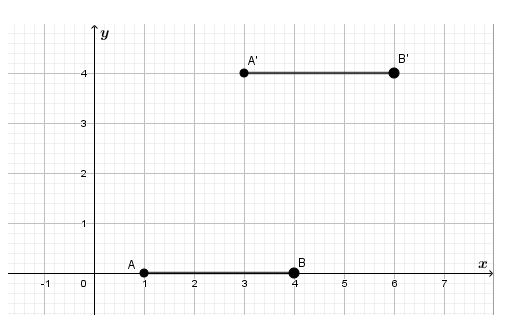
We now have \(\overline{A B}\) and \(\overline{A’ B’}\) which are parallel and congruent.

To sketch a parallelogram, it is enough to connect the sides AA’ and BB’.

When we connect AA’ and BB’ , we have parallelogram ABB’ A’

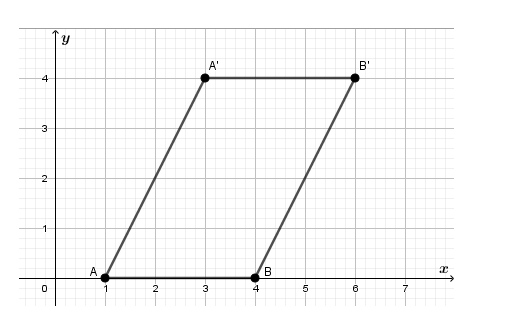
The above steps we have explained how to draw a parallelogram using translation.

Savvas Learning Co Geometry Student Edition Chapter 9 Transformations Exercise

 

Page 541  Exercise 1  Problem 1

The given two triangle are congruent.

To complete the congruence statement for the given pair △ABC≅.

Two triangles are said to be congruent if all three corresponding sides are equal and all the three corresponding angles are equal

Congruent triangles have congruent corresponding sides.

Triangles share the side \(\overline{A C}\).

Corresponding side to \(\overline{A B}\) is \(\overline{A D}\)

Since the triangles are congruent , it follows that  ⇒ \(\overline{A B}\) ≅ s \(\overline{A D}\)

Corresponding side to \(\overline{C B}\) is \(\overline{C D}\)

Since the triangles are congruent , it follows that \(\overline{C B}\) ≅ \(\overline{C D}\)

The congruence statement is

⇒ △ABC ≅ △ADC

The congruence statement is △ABC ≅ △ADC

 

Page 541  Exercise 2  Problem 2

The given two triangle are congruent.

To complete the congruence statement for the given pair △ABC≅.

Two triangles are said to be congruent if all three corresponding sides are equal and all the three corresponding angles are equal

Congruent triangles have congruent corresponding sides.

Corresponding side to \(\overline{A C}\) is \(\overline{L K}\).

Since the triangles are congruent, it follows that  ⇒ \(\overline{A C}\) ≅ \(\overline{L K}\)

Corresponding side to \(\overline{C B}\) is \(\overline{K J}\)

Since the triangles are congruent, it follows that  ⇒ \(\overline{C B}\) ≅ \(\overline{K J}\)

Corresponding  side to \(\overline{B A}\) is \(\overline{J L}\).

Since the triangles are corresponding, it follows that  ⇒ \(\overline{B A}\) ≅ \(\overline{J L}\)

The congruence statement is  ⇒ △ABC≅△LJK

The congruence statement is △ABC≅△LJK

 

Page 541  Exercise 3  Problem 3

The given two triangle are congruent.

To complete the congruence statement for the given pair △ABC≅.

Two triangles are said to be congruent if all three corresponding sides are equal and all the three corresponding angles are equal

Congruent triangles have congruent corresponding sides.

Corresponding side to \(\overline{A C}\) is \(\overline{R S}\).

Since the triangles are congruent, it follows that ⇒ \(\overline{A C}\) ≅ \(\overline{R S}\).

Corresponding side to \(\overline{A B}\) is \(\overline{R T}\).

Since the triangles are congruent, it follows that  ⇒ \(\overline{A B}\) ≅ \(\overline{R T}\).

Corresponding side to \(\overline{B C}\) is \(\overline{T S}\).

Since the triangles are congruent, it follows that  ⇒ \(\overline{B C}\) ≅ \(\overline{T S}\)

The congruence statement is ⇒△ABC ≅ △RTS

The congruence statement is △ABC ≅ △RTS

 

Page 541  Exercise 4  Problem 4

The given two triangle are congruent.

To complete the congruence statement for the given pair △ABC≅

Two triangles are said to be congruent if all three corresponding sides are equal and all the three corresponding angles are equal

Congruent triangles have congruent corresponding sides and angles.

Corresponding side to \(\overline{A B}\) is \(\overline{H L}\).

Since the triangles are congruent, it follows that ⇒ \(\overline{A B}\) ≅\(\overline{H L}\)

Corresponding side to \(\overline{B C}\) is \(\overline{H C}\).

Since the triangles are congruent, it follows that ⇒ \(\overline{B C}\) ≅ \(\overline{H C}\).

Corresponding side to \(\overline{A C}\) is \(\overline{C L}\).

Since the triangles are congruent, it follows that ⇒ \(\overline{A C}\) ≅ \(\overline{C L}\)

The congruence statement is  ⇒ ΔABC ≅ △LHC

The congruence statement is ΔABC ≅ △LHC

 

Page 541  Exercise 5  Problem 5

The given regular polygon is decagon.

To find – The measure of an angle of the given regular polygon.

The measure of interior angle of a regular n-polygon is given by  (n−2)⋅180° .

A regular decagon is a regular polygon with 10 sides.

Substitute n=10 into the formula from the Polygon Interior Angles Sum Theorem to calculate the sum of the measures of the angles

⇒  (10−2)⋅180

Calculate the sum of the angles:
​
​⇒  (10−2 ) ×  180

⇒  1440°

​Since the angles of a regular polygon are all equal in measure, divide the sum by the number of angles to find the measure of one angle:

⇒  \(\frac{1440}{10}\)

Divide the numbers  ⇒ 144°

Therefore, the measure of the interior angle of a regular decagon is 144°.

The measure of the interior angle of a regular decagon is 144°.

 

Page 541  Exercise 6  Problem 6

The given regular polygon is 18 -polygon.

To find – The measure of an angle of the given regular polygon.

The measure of interior angle of a regular n-polygon is given by   \(\frac{(n-2) \cdot 180^{\circ}}{n}\)

The formula for the measure $m$ of an interior angle of a regular n-polygon is   m = \(\frac{(n-2) \cdot 180^{\circ}}{n}\)

Since the given regular polygon is an n = 18 into the equation. m = \(\frac{(18-2) \cdot 180^{\circ}}{18}\)

Calculate the value of m:
​
​m = \(\frac{(18-2) \times 180}{18}\)

m = 160

⇒ 160°

​The measure of an interior angle of an 18 – polygon is 160°.

 

Page 541  Exercise 7  Problem 7

The given quadrilateral is rectangle.

To find whether a diagonal of the given quadrilateral always, sometimes, or never produces congruent triangles.

Two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other.

The rectangle ABCD is a parallelogram with four right angles.

The diagonal \(\overline{A C}\) is separating the rectangle ABCD into two right triangles △ABC and △ADC

The diagonal AC is the hypotenuse of both triangles △ABC and △ADC.

The leg \(\overline{A B}\) is congruent with \(\overline{C D}\).

Since conditions for the Hypotenuse-Leg (HL)

Theorem are met, triangles △ABC and △ADC are congruent.

It follows that the diagonal of any rectangle will split the rectangle into two right, congruent triangles.

The diagonal of the rectangle will always separate the rectangle into two congruent triangles.

The diagonal of the rectangle will always separate the rectangle into two congruent triangles.

 

Page 541  Exercise 8  Problem 8

The given quadrilateral is isosceles trapezoid.

To find whether a diagonal of the given quadrilateral always, sometimes, or never produces congruent triangles.

Two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other.

The diagonal AC is separating the isosceles trapezoid ABCD into two triangles △ABC and △ADC.

The diagonal AC is the side of both triangles △ABC and △ADC.

The leg h \(\overline{A D}\) is congruent with \(\overline{B C}\).

Since the bases AB and CD never have equal length, the triangles will never be congruent.

The diagonal of the isosceles trapezoid will never separate the trapezoid into two congruent triangles.

The diagonal of the isosceles trapezoid will never separate the trapezoid into two congruent triangles.

 

Page 541  Exercise 9  Problem 9

The given quadrilateral is kite.

To find whether a diagonal of the given quadrilateral always, sometimes, or never produces congruent triangles.

Two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other.

Kite has 2 diagonals.

The diagonal \(\overline{A C}\) is separating the kite ABCD into two triangles △ABC and △ADC.

The diagonal\(\overline{A C}\) is the side of both triangles △ABC and △ADC.

The side \(\overline{A C}\) is congruent with \(\overline{A D}\) . The side \(\overline{B C}\) is congruent with \(\overline{D C}\) .

Since conditions for the Side-Side-Side Postulate are met, triangles △ABC and △ADC are congruent.

The diagonal \(\overline{B D}\) is separating the kite ABCD in to two triangles △ABD and △BCD.

If the legs of triangle △BCD are not the same length as the legs of triangle △ABD , the triangles are not congruent.

The diagonal of the kite will sometimes separate the kite into two congruent triangles.

The diagonal of the kite will sometimes separate the kite into two congruent triangles.

 

Page 541  Exercise 10  Problem 10

To find whether a diagonal of the given quadrilateral always, sometimes, or never produces congruent triangles.

Two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other.

The diagonal AC is separating the parallelogram ABCD into two triangles △ABC and △ADC . The diagonal \(\overline{A C}\) is the side of both triangles △ABC and △ADC.

The side \(\overline{A B}\) is congruent with \(\overline{C D}\) . The side \(\overline{A D}\) is congruent with \(\overline{B C}\).

Since conditions for the Side-Side-Side Postulate are met, triangles △ABC and △ADC
are congruent.

It follows that the diagonal of any parallelogram will split the parallelogram into two congruent triangles.

The diagonal of the parallelogram will always separate the parallelogram into two congruent triangles.

The diagonal of the parallelogram will always separate the parallelogram into two congruent triangles.

 

Page 541  Exercise 11  Problem 11

The given length of a wall in the blueprint is 2.5in.

To find the length of the actual wall.

The given scale of a blueprint is 1in. = 20ft

The length of a wall in the blueprint is  ⇒ 2.5in

Substitute scale1in.=20ft to convert to actual length  ⇒ 2.5 × 20ft

Multiply the numbers  ⇒ 2.5 × 20

⇒  50

The actully length of the well is 50 ft.

 

Page 541  Exercise 12  Problem 12

The given entrance is wide.

To find the blueprint length of an entrance that is 5ft wide.

The given scale of a blueprint is 1in.=20ft

Let w be the width of the door on the image.

Using the scale and given width, write the proportion for the width w:

⇒  \(\frac{1}{20}\) = \(\frac{w}{5}\)

Calculate the value of w:

⇒  \(\frac{1}{20}\) = \(\frac{w}{5}\)

⇒  w = 0.25

The width of the door on the image is 0.25in.

The width is 0.25in

The width is 0.25in.

 

Page 541  Exercise 13  Problem 13

Given: A mirror and a person

To find if you raise your right hand, which hand appears to be raised in your reflection and If you are standing 2ft from the mirror, how far away from you does your reflection appear to be?

If you raise your right hand, left hand appears to be raised in your reflection If you are standing 2ft from the mirror, your reflection appear to be at a distance 4ft of from you

Therefore ,If you raise your right hand, left hand appears to be raised in your reflection If you are standing 2ft from the mirror, your reflection appear to be at a distance 4ft of from you.

 

Page 541 Exercise 14 Problem 14

Given:   

The minute hand of a clock rotates as the minutes go by.

To find –  That what point of the minute hand stays fixed as the hand rotates

The back end of the minute hand or the beginning part of the minute hand stays fixed as the hand rotates

The back end of the minute hand or the beginning part of the minute hand stays fixed as the hand rotates.

 

Page 541  Exercise 15  Problem 15

Given:  

The pupils in your eyes dilate in the dark

To find  – That what it means to dilate a geometric

To dilate a geometric, means to change the size of a given figure, without changing its shape.

For example: An equilateral triangle of side 3 units can be dilated to an equilateral triangle with side 9 units.

Therefore, Dilation is the process of increasing the size of an object while maintaining its shape.

 

Page 541  Exercise 16  Problem 16

The given that an interior designer used a repeating pattern when planning the tiling of a bathroom floor.

To describe your thinking about a tiling is in geometry.

A repeating pattern is a type of pattern where the rule just keeps on repeating over and over.

In real life, tiles used in floors should have the same pattern for it to be aesthetically pleasing.

Tiles are connected side by side to fill a certain floor area.

In geometry , tilling means a repeating patter of shapes that completely fills a plane.

Savvas Learning Co Geometry Student Edition Chapter 8 Right Triangles And Trigonometry Exercise 8.1 The Pythagorean Theorem And Its Converse

 

Page 495 Exercise 1 Problem 1

Given:

To find – The value of the variable.

Using the Pythagorean theorem find the value of the given variable.

We have

x² = 35² + 12²

x² = 1225 + 144

x²  = 1369

x = \(\sqrt{1369}\)

x = 37

The value is x = 37.

 

Page 495  Exercise 2  Problem 2

Given:

To find – The value of the variable.

Using the Pythagorean theorem find the value of the given variable.

We have

x² = 9² + 7²

x² = 81 + 49

x = \(\sqrt{130}\)

x ≈ 11.40

The value is x ≈ 11.40

 

Page 495  Exercise 3  Problem 3

Given:

To find – The value of the variable.

Using the Pythagorean theorem find the value of the given variable.

We have

x² =  5² − 3²

x²  = 25 − 9

x = \(\sqrt{16}\)

x = 4

The value is x = 4.

 

Page 495 Exercise 4 Problem 4

Given:

To find – The value of the variable.

Using the Pythagorean theorem find the value of the given variable.

We have

x² = 13² − 11²

x² = 169 − 121

x = \(\sqrt{48}\)

x = 4\(\sqrt{3}\).

The value is  x = 4\(\sqrt{3}\).

 

Page 495  Exercise 5  Problem 5

Given: Describe the conditions that a set of three numbers must meet in order to form a Pythagorean triple.

To find –  The condition for a Pythagorean triple.

The condition that must be met for three numbers to form a Pythagorean triple is that the sum of the squares of the two numbers should be equal to the square of the third number.

The condition that must be met for three numbers to form a Pythagorean triple is that the sum of the squares of the two numbers should be equal to the square of the third number.

 

Page 495  Exercise 6  Problem 6

Given:

To find – Describe the error.

Friend made a mistake in choosing the hypotenuse. Instead of 30,34 should have been chosen for the hypotenuse.

Choose  34  as hypotenuse, and 16,30 for legs.

We have

16² + 30² = 34²

256 + 900 = 1156

1156 = 1156

The friend made a mistake in choosing the hypotenuse. Instead of 30,34 should have been chosen for the hypotenuse. We see that the sum of the squares of the lengths of the legs is equal to the square of the hypotenuse. So the triangle is a right-angled triangle.

 

Page 495  Exercise 7  Problem 7

Given:

To find – The value of the variable.

Using the Pythagorean theorem find the value of the given variable.

We have

x² = 16² + 30² 

x²  = 256 + 900

x² = 1156

x = \(\sqrt{1156}\)

x = 34

The value is x = 34.

 

Page 495  Exercise 8  Problem 8

Given: A right-angle triangle

To Find – The value of x

We have, a or leg₁ = 16 and b or leg₂ = 12

So by applying the Pythagorean theorem

​⇒   a² + b²  = x²

⇒   16² + 12² =  x²

⇒   256 + 144 = x²

⇒   400 = x²

​⇒  \(\sqrt{400}\)

=  \(\sqrt{x^2}\)  (Take positive square root)

⇒  x =  20

So the length of the hypotenuse or the value of x is 20.

The length of the hypotenuse (value of x) of a right-angle triangle with the other two sides being 16 and 12 is 20.

 

Page 495  Exercise 9  Problem 9

Given: A right-angle triangle

To Find – The value of x

We have, a or leg₁  = 65 and b or leg₂  = 72

So by applying the Pythagorean theorem

​⇒  a²  + b² =  x²

⇒  65² + 72² =  x²

⇒   4225 + 5184  =  x²

⇒  9409 = x²

⇒  x = 97    (Take positive square root)

So the value of x is 97.

The length of the hypotenuse (value of x) of a right-angle triangle with the other two sides being 65 and 72 is 97.

 

Page 495 Exercise 10 Problem 10

Given: A right-angle triangle

To Find – The value of x

We have, a or leg₁ = 15 and b or leg₂ =  8

So by applying the Pythagorean theorem

⇒  a²  + b² = x²

⇒  15² + 8² = x²

⇒  225 + 64 = x²

⇒  289 = x²

​⇒  x = 17 (Take positive square root)

So the value of x is 17.

The length of the hypotenuse (value of x) of a right-angle triangle with the other two sides being 15 and 8 is 17.

 

Page 495  Exercise 11  Problem 11

Given: A set of numbers- 4, 5, 6

To Find – Does the set of numbers form a Pythagorean triple

We have, a set of whole numbers- 4, 5, 6

Let, a = 4, b = 5, c = 6

Substituting the value of a, b, and c in a² + b² = c²

​⇒   4² + 5² = 6² 

⇒  16 + 25 = 36

⇒  41 ≠ 36
​
As L.H.S. is not equal to R.H.S., therefore, the sets 4, 5, 6 do not satisfy the equation a² + b²  = c²

So the set 4, 5, 6 is not a Pythagorean triple.

The set of numbers 4, 5, 6 is not a Pythagorean triple as it does not satisfy the equation a² + b²  = c².

 

Page 495  Exercise 12  Problem 12

Given: A set of numbers- 10, 24, 26.

To Find – Does the given set of numbers form a Pythagorean triple

We have, a set of numbers- 10, 24, 26

Let, a = 10,b = 24, c = 26

Substituting the values of a, b, c in the equation a² + b²  = c²

​⇒  a² + b²  = c² 

⇒  10²  + 24²  = 26²

⇒  100 + 576 = 676

⇒  676 = 676
​
As L.H.S. is equal to R.H.S. therefore, the set of numbers 10, 24, 26 satisfy the equation a² + b² = c²

Hence, the set of numbers 10, 24, 26 is a Pythagorean triple.

The set of numbers 10, 24, 26 is a Pythagorean triple because it satisfies the equation a² + b² = c²

 

Page 495  Exercise 13  Problem 13

Given: A set of numbers- 10, 24, 26.

To Find – Does the given set of numbers form a Pythagorean triple

We have, a set of numbers- 10, 24, 26

Let, a = 10, b = 24, c = 26

Substituting the values of a, b, and c in the equation a² + b² = c²

​⇒  a²  + b²  = c²

⇒  10² + 24² = 26²

⇒  100 + 576  =  676

⇒  676 = 676
​
As L.H.S. is equal to R.H.S., therefore, the set of numbers 10, 24, 26 satisfies the equation a² + b² = c²

Hence, the set of numbers 10, 24, 26 is a Pythagorean Triple.

The set of numbers 10, 24, 26 is a Pythagorean triple because it satisfies the equation a²+ b² = c².

 

Page 496  Exercise 14  Problem 14

Given: A set of numbers- 15, 20, 25

To Find – Does the given set of numbers form a Pythagorean triple

We have, a set of numbers- 15, 20, 25

Let, a = 15,b = 20,c = 25

Substituting the values of a, b, c in the equation a² + b² = c²

​⇒   a² + b²  = c²

⇒  15² + 20² =  25²

⇒  225 + 400 = 625

⇒  625 = 625
​
As L.H.S. is equal to R.H.S., therefore, the set of numbers 15, 20, 25 satisfies the equation a² + b² = c²

Hence, the set of numbers 15, 20, 25 is a Pythagorean Triple.

The set of numbers 15, 20, 25 is a Pythagorean Triple because it satisfies the equation  a² + b² = c²

 

Page 496  Exercise 15  Problem 15

Given: A right-angle triangle with two sides

To Find – The value of x

In the given right-angle triangle

Base (a) = x units

Height (b) = 4 units

Hypotenuse (c) = 6 units

(leg₁ )²+(leg₂)² = (Hypotenuse)²               (Pythagorean theorem)

Substitute the values of a, b, c in the Pythagorean Theorem

​⇒   a² + b² = c²

⇒   x ²+ 4²  = 6²

⇒    x² + 16 = 36

⇒   x² + 16 − 16 = 36 − 16

⇒     x² = 20

⇒    x = \(\sqrt{20}\)

⇒    x = \(\sqrt{4(5)}\)

⇒   x = 2\(\sqrt{5}\)

The value of x in the simplest radical form is 2\(\sqrt{5}\).

 

Page 496  Exercise 16  Problem 16

Given: A right-angle triangle with two sides

To Find – The value of x in simplest radical form

In the given right-angle triangle

Base (a) = x units

Height (b) = 4 units

Hypotenuse (c) = 7 units

Substituting the values in the Pythagorean theorem

(leg1)² + (leg2)² =(Hypotenuse)²

​⇒   x² + 4² = 7²

⇒   x² + 16 = 49

⇒   x²  + 16 − 16 = 49 − 16

⇒   x² = 33

⇒   x = \(\sqrt{33}\)

​The value of x in the simplest radical form is \(\sqrt{33}\).

 

Page 496  Exercise 17  Problem 17

Given: A right-angle triangle with two sides

To Find  –  The value of x in simplest radical form

In the given right-angle triangle

leg₁ = 16, leg₂ = x,  Hypotenuse = 19

Substituting the values in the Pythagorean Theorem
(leg₁)² +(leg₂)² = (Hypotenuse)²

​⇒   16²  + x² = 19²

⇒   256 + x² = 361

⇒   x² = 361 − 256

⇒   x²  = 105

⇒  x = \(\sqrt{105}\)

​The value of x in the simplest radical form is \(\sqrt{105}\).

 

Page 496  Exercise 18  Problem 18

Given: A right-angle triangle

To Find –  The value of x in simplest radical form

In the given right-angle triangle

leg₁ = x, leg₂ = x, Hypotenuse = 6

Substituting the values in the Pythagorean Theorem

​⇒   (leg₁)²  + (leg₂ )² = (Hypotenuse)

⇒   x²  + x² = 6²

⇒  2x² = 36

⇒   \(\frac{2 x^2}{2}=\frac{36}{2}\)

⇒   x² = 18

⇒   x = \(\sqrt{18}\)

⇒   x  =  \(\sqrt{9(2)}\)

⇒   x =  3\(\sqrt{2}\)

The value of x in the simplest radical form is 3\(\sqrt{2}\)

 

Page 496  Exercise 19  Problem 19

Given: A right-angle triangle

To find – The value of x in the simplest radical form

In the given right-angle triangle

leg₁ = 5, leg₂ = x , Hypotenuse = 10

Substituting the values in the Pythagorean Theorem

​⇒  (leg1)² + (leg2)² = (Hypotenuse)²

⇒   5² + x²  = 10²

⇒   25 + x²  = 100

⇒   25 − 25 + x² = 100 − 25

⇒    x² = 75

⇒    x = \(\sqrt{75}\)

⇒    x = \(\sqrt{25(3)}\)

⇒   x = 5\(\sqrt{3}\)

The value of x in the simplest radical form is 5\(\sqrt{3}\).

 

Page 496  Exercise 20  Problem 20

​Given: Length of the ladder is 15 -ft, Base of the ladder from the house is 5 -ft

To Find –  Height on the house that the ladder reach

As the ladder is leaning against the house so the height of the ladder will be the hypotenuse, i.e., 15 − ft.

The base of the ladder is 5 ft from the house, so the base will be  5 ft.  b = 5ft

Let the height on the house that the ladder reach be x ft.

So according to the Pythagorean Theorem

​⇒   Base²+ Height² =  Hypotenuse²

⇒   5² + x² = 152

⇒   25 + x² = 225

⇒  x² = 225 − 25

⇒  x = \(\sqrt{200}\)

⇒  x = 14.142
​
⇒  x = 14.1ft (Roud off to nearest tenth)

Hence the height on the house that the ladder reach is 14.1 ft.

When a 15 − ft ladder leans against the house and the base of the ladder is 5 − ft from the house then the height on the house that the ladder reach is 14.1 ft.

 

Page 496 Exercise 21 Problem 21

Given: A 24 m long walkway forms a diagonal of a square playground.

To Find –  Length of a side of the playground

As the walkway divides the square into two right-angle triangles, and the walkway (Diagonal) is 24 m long so the Pythagorean Theorem can be applied.

As each side of a square is equal so let leg₁  = leg₂ = x meters and the walkway will be the hypotenuse.

Substituting the values in the Pythagorean Theorem

⇒  (leg₁)² + (leg₂)² = (hypotenuse)²

⇒    x² + x² = 24²

⇒   2x² = 576

⇒   \(\frac{2 x^2}{2}=\frac{576}{2}\)

⇒   x²  = 288

⇒   x = \(\sqrt{288}\)

⇒   x≈16.97
​
⇒  x≈17.0 meters (Round off)

Hence, the length of each side of the playground is approximately 17 meters.

If a 24 m walkway forms one diagonal of a square playground then, the length of a side of the playground is approximately 17 meters.

 

Page 496  Exercise 22  Problem 22

Given: A triangle

To Find – If the triangle is a right-triangle

Hypotenuse = 25, leg₁  = 24, leg₂ = 8

For checking, if the triangle is a right triangle apply the Pythagorean theorem

​⇒  (leg₁)² + (leg₂ )² = (Hypotenuse)²

⇒   24² + 8² = 25²

⇒  576 + 64 = 625

⇒   640 ≠ 625
​
As L.H.S. is not equal to R.H.S., therefore it does not satisfy the Pythagorean Theorem and hence it is not a right triangle.

The triangle is not a right triangle because it does not satisfy the Pythagorean theorem, 24² + 8² ≠25².

 

Page 496  Exercise 23  Problem 23

Given: The triangle.

To Find – If the triangle is a right-angled triangle or not.

Using the Pythagoras theorem, we will find if the triangle is a right-angled triangle.

Consider the triangle

Pythagoras theorem states that the square of the hypotenuse is equal to the sum of the squares of other sides.

Let,​c = 65, a = 56 , b = 33

​Now, using Pythagoras theorem:

​c² = 65²

c² = 4225
​
And,​a² + b² = 56² + 33²

a² + b² = 4225.
​
Since,c² = a2 + b²  the triangle is a right-angled triangle.

The triangle is a right triangle.

 

Page 496  Exercise 24  Problem 24

Given: The lengths of the sides of a triangle are 4,5,6.

To Find – The type of triangle.

In order to determine the type of triangle, we need to observe the inequality of the Pythagoras theorem

Consider the lengths of the three sides of a triangle , 4,5,6

Now, if  a² + b² = c² the triangle is a right angle, if a² + b² > c² triangle is acute, if a² + b² < c² triangle is obtuse.

Now, the highest length is the hypotenuse.

So, ​a = 4 , b = 5 , c = 6

​So, a² + b² = 4² + 5²

a² + b² =16 + 25

a² + b² = 41

​c² = 6²

c² = 36.

As a² + b² >c² the triangle is an acute triangle.

 

Page 496  Exercise 25  Problem 25

Given: The length of the sides of a triangle is  0.3,0.4,0.6 .

To Find – The type of triangle.

In order to determine the type of triangle, we need to observe the inequality of the Pythagoras theorem.

Consider the lengths of the three sides of a triangle  , 0.3,0.4,0.6.

Now, if a2 + b2 = c2 the triangle is a right triangle, if a² + b² > c² triangle is acute, if a² + b² < c² triangle is obtuse.

The highest length is the hypotenuse. So,​a = 0.3 b = 0.4 c = 0.6
​
Now,​a² + b² = 0.3² + 0.4²

a² + b² = 0.25 and c² = 0.62

c² = 0.36
​
As a² + b² <c²  the triangle is an obtuse triangle.

The triangle with lengths with sides 0.3,0.4,0.6 is an obtuse triangle.

 

Page 496  Exercise 26  Problem 26

Given: The length of the sides of a triangle is11,12,15.

To Find – The type of triangle.

In order to determine the type of triangle, we need to observe the inequality of the Pythagoras theorem.

Consider the sides of the triangle , 11,12,15.

Now, if a²  + b²  = c² the triangle is a right-angle triangle, if a²  +b² > c²  triangle is acute, if a²+ b²  <c²  triangle is obtuse.

The highest length is the hypotenuse. So,​a = 11 b = 12 c = 15

So,​a² + b² = 11² + 12²

a² + b² = 121 + 144

a² + b² = 265.
​
And,​c²= 15²

c² = 225

As a²  + b² > c² the triangle is an acute triangle.

The triangle with lengths of sides 11,12,15 is an acute triangle.

 

Page 496  Exercise 27  Problem 27

Given: The length of the sides of a triangle is \(\sqrt{3}\),2,3.

 To find – The type of triangle.

In order to determine the type of triangle, we need to observe the inequality of the Pythagoras theorem.

Consider the sides of the triangle ,  \(\sqrt{3}\),2,3.

Now, if a² + b² = c² triangle is a right angle triangle, if a² + b² > c² the triangle is an acute triangle and if a² + b² < c² the triangle is an obtuse triangle.

The highest length in a triangle is the hypotenuse.

So, a =   \(\sqrt{3}\) , b= 2, c= 3

Now, a² + b² = 7 and

​c² = 3²

c² = 9

As a² + b² < c²  the triangle is an obtuse triangle.

The triangle with the sides  \(\sqrt{3}\) ,2,3 is an obtuse triangle.

Savvas Learning Co Geometry Student Edition Chapter 8 Right Triangles and Trigonometry Exercise

 

Page 487  Exercise 1  Problem 1

Given: 0.5858 = \(\frac{24}{x}\)

To Find: We have to solve the given expression.

Using algebraic identities.

Given

​0.5858 = \(\frac{24}{x}\)

x = \(\frac{24}{0.5858}\)

0.5858

∴ x = 40.9696
​
The value of x is 40.9696.

 

Page 487  Exercise 2  Problem 2

Given: 0.8572 = \(\frac{5271}{x}\)

To Find – We have to solve the given expression.

Using algebraic identities.

Given

​0.8572 = \(\frac{5271}{x}\)

0.8572x = 5271

x = \(\frac{5271}{0.8572}\)

∴ x = 6149.0900
​
The value of x is 6149.0900.

 

Page 487  Exercise 3  Problem 3

Given: 0.5 = \(\frac{x}{3x+5}\)

To Find –  We have to solve the given expression.

Using algebraic identities.

Given , 0.5 = \(\frac{x}{3x+5}\)

1.5x + 2.5 = x

1.5x − x + 2.5 = 0

0.5x + 2.5 = 0

0.5x = −2.5

x = −2.5

0.5

∴ x = −5

The value of x is −5.

 

Page 487  Exercise 4  Problem 4

Given: \(\overline{C D}\) ∥ \(\overline{A B}\)

To Find –  We have to prove the given pair of triangles as similar.

Using the theorems and postulates of similarities.

Given in the diagram \(\overline{C D}\) ∥ \(\overline{A B}\)

Therefore

\(\overline{C E}\) = \(\overline{E B}\)

\(\overline{A E}\) = \(\overline{E D}\)

Therefore, the given pair of triangles are similar.

We have proved that the given pair of triangles are similar.

 

Page 487  Exercise 5  Problem 5

Given: A pair of triangles.

To Find – We have to prove the given pair of triangles as similar.

Using the theorems and postulates of similarities.

From the given diagram
​
\(\frac{21}{27}\) = \(\frac{14}{18}\)

∴ \(\frac{7}{9}\) = \(\frac{7}{9}\)

And , \(\frac{21}{9.6}\) = \(\frac{14}{6.4}\)

∴ \(\frac{7}{3.2}\) = \(\frac{7}{3.2}\)

And , \(\frac{27}{9.6}\) = \(\frac{18}{6.4}\)

∴ \(\frac{9}{3.2}\) = \(\frac{9}{3.2}\)

Therefore, the given pair of triangles are similar.

By proving the ratio of the corresponding sides of the triangles are same we have proved that the given pair of triangles are similar.

 

Page 487  Exercise 6  Problem 6

Given: \(\overline{J K}\) ⊥ \(\overline{M L}\)

To Find –  We have to prove the given pair of triangles as similar.

Using the theorems and postulates of similarities.

In the given diagram given that

Given  \(\overline{J K}\) ⊥ \(\overline{M L}\)

From the diagram we get

tan M =  \(\frac{15}{21}\)

M = tan^-1(\(\frac{5}{7}\))

∠M = 35.5°

tan L = \(\frac{20}{28}\)

L = tan^-1(\(\frac{5}{7}\))

∴ ∠L = 35.5°

Therefore ∠M = ∠L

The given pair of triangles are similar by ASA property of similarity.

By ASA property of similarity we have proved that the given pair of triangles are similar.

 

Page 487  Exercise 7  Problem 7

Given: A diagram of a ΔABCb with right ∠C and altitude \(\overline{C D}\)

To Find – We have to find the value of x.

Using the rules of the right angles.

From the given diagram we get

​BD = 16

DA = 9
​
​tan∠B = tan∠ACD

⇒  \(\frac{16}{x} – {9}{x}\)

⇒  \(\frac{7}{x}\) = 0

∴ x = 7

​In ΔABC the value of x is 7.

 

Page 487  Exercise 8  Problem 8

Given: A diagram of aΔABC with right ∠C and altitude \(\overline{C D}\)

To Find –We have to find the value of x.

Using the rules of the right angles.

From the given diagram we get

AB = 32 [Given]

BC = 16 [Given]

∠D = 90° [Given]

​Cos∠ABC = cos∠DBC

\(\frac{16}{32-x}\) = \(\frac{x}{16}\)

32x − x² = 256

x² − 32x + 256 = 0

x² − 16x − 16x + 256 = 0

x(x − 16) − 16(x − 16) = 0

(x−16)(x−16) = 0

∴ x = 16
​
In ΔABC the value of x is 16.

 

Page 487  Exercise 9  Problem 9

Given: A diagram of a ΔABC with right ∠C and altitude \(\overline{C D}\)

To Find – We have to find the value of x.

Using the rules of the right angles.

From the diagram we get

AD = 4 [Given]

DB = 9 [Given]

∠D = 90°[Given]

∠C = 90° [Given]

​cos∠DAC = cos∠BAC

\(\frac{4}{x}\) = \(\frac{x}{13}\)

x² =  52

∴ x = 7.211
​
In ΔABC the value of x is 7.211.

 

Page 487  Exercise 10  Problem 10

Given:  The triangle

To find – The value of x.

We will use geometric form and calculate the value of x

The geometric means is as follows:

\(\frac{d}{b}\) = \(\frac{b}{e}\)

\(\frac{x+16}{15}\) = \(\frac{15}{x}\)

x(x + 16) = 225x

x² + 16x − 225x = 0

x = 0,209

The value of x in △ABC with right ∠C and altitude is 209 \(\overline{C D}\)

 

Page 487  Exercise 11  Problem 11

We have to describe how might you describe an angle of elevation in geometry.

We observe that the angle of elevation is the angle between the horizontal line of sight and the line of sight up to an object.

An angle of elevation is the angle between the horizontal to a line of sight to the object when looking up.

In geometry it is the angle of elevation is the angle between the horizontal line of sight and the line of sight up to an object.

An angle of elevation in geometry is the angle of elevation is the angle between the horizontal line of sight and the line of sight up to an object.

 

Page 487  Exercise 12  Problem 12

We have to describe how to calculate the magnitude of a line segment in the coordinate plane.

For a line segment AB containing the points, A,B the magnitude is the length of the line segment.

|AB| = \(\sqrt{\left(x_1-x_2\right)^2+\left(y_1-y_2\right)^2}\)

The magnitude of a line segment in the coordinate plane can be calculated by the distance between the two points containing the formula and taking the magnitude

 

Page 487  Exercise 13  Problem 13

We have to what does the prefix indicate in these words and what geometric figure do you think is associated with the phrase trigonometric ratio.

We observe that tri represents three in quantity so so the geometric figure associated with the phrase trigonometric ratio is a triangle.

The geometric figure is a triangle.

The prefix tri indicates three and the geometric figure do you think is associated with the phrase trigonometric ratio is triangle.

Savvas Learning Co Geometry Student Edition Chapter 7 Similarity Exercise 7.1 Ratios And Proportions

 

Page 436  Exercise 1  Problem 1

We are given that a cellphone is 46mm wide and stands 84mm long.

We are required to find the ratio of the width to its length.

Here, we will divide the width by the length to get the answer.

From the given information, we have

​​L = 84mm
​
W = 46mm
​
Since both the measures are in the same units, we find the ratio as

​Ratio = \(\frac{W}{L}\)

=  \(\frac{46 \mathrm{~mm}}{84 \mathrm{~mm}}\)

=  \(\frac{23}{42}\)

Hence the required ratio is, 23:42.

​When, to the nearest millimeter, a cell phone is 84mm long and 46mm wide, the ratio of the width to the length is 23:42.

 

Page 436  Exercise 2  Problem 2

We are given that two angle measures are in the ratio 5:9.

We are required to write expressions for the two angle measures in terms of the variable x.

Here, we will equate the given ratio to the variable to get the expression.

Let us equate the given ratio of angles to the variable, to get

\(\frac{5}{9}\) = x

Now, multiplying both sides by 9, we get

\(\frac{5}{9}\) × 9 = x × 9

5 = 9x
​
Which is the required expression.

When two angle measures are in the ratio 5:9, expressions for the two angle measures in terms of the variable x is 5 = 9x.

 

Page 436  Exercise 3  Problem 3

We are given the proportion \(\frac{20}{z}\)= \(\frac{5}{3}\).

We are required to solve the given proportion.

Here, we will find the value of the variable involved to solve the proportion.

First we will cross multiply the numbers in the proportion, to get

​20 × 3 = 5 × z

60 = 5z
​
Now, dividing throughout the equation by 5, we get

\(\frac{5z}{5}\) = \(\frac{60}{5}\)

z = 12

​The solution of the given proportion \(\frac{5z}{5}\) = \(\frac{60}{5}\) is z = 12 , obtained using cross multiplication and simple arithmetic operations.

 

Page 436  Exercise 4  Problem 4

We are given the proportion \(\frac{a}{7}\) = \(\frac{13}{b}\)

We are required to find the ratio that completes the equivalent proportion

Here, we will use cross multiplication to the given proportion to get to the answer.

From the given proportion, using cross multiplication, we can write that

⇒  \(\frac{a}{7}\) = \(\frac{13}{b}\)

a × b = 13 × 7
​
Multiplying both sides of the equation by \(\frac{1}{13×b}\),we get

​a × b × \(\frac{1}{13×b}\)

= 13 × 7 × \(\frac{1}{13×b}\)

⇒  \(\frac{a}{13}\) = \(\frac{7}{b}\)

The ratio which completes the given equivalent proportion is given by \(\frac{a}{13}\) = \(\frac{7}{b}\), which is obtained from the given value \(\frac{a}{7}\) = \(\frac{13}{b}\)

 

Page 436  Exercise 4  Problem 5

We are given the proportion\(\frac{a}{7}\) = \(\frac{13}{b}\).

We are required to find the ratio that completes the equivalent proportion

Here, we will use subtraction from the given proportion to get to the answer.

The ratio which completes the given equivalent proportion is given by.  \(\frac{a-7}{7}=\frac{13-b}{b}\), which is obtained from the given value . \(\frac{a}{7}\) = \(\frac{13}{b}\)

 

Page 436  Exercise 4  Problem 6

We are given the proportion \(\frac{a}{7}\) = \(\frac{13}{b}\).

We are required to find the ratio that completes the equivalent proportion

Here, we will take reciprocal of the given proportion to get to the answer.

For the given proportion

\(\frac{a}{7}\) = \(\frac{13}{b}\)

We can take the reciprocal to get

\(\frac{7}{a}\) = \(\frac{b}{13}\)

Which is the required equivalent proportion.

The ratio which completes the given equivalent proportion is given by \(\frac{7}{a}\) = \(\frac{b}{13}\) , which is obtained from the given value  \(\frac{7}{a}\) = \(\frac{b}{13}\)

 

 Page 436  Exercise 5  Problem 7

In the given question, we have been given that the length of the sides of the triangle are in the extended ratio 3:6:7.

We need to find two possible sets of side lengths, in inches, for the triangle.

First, we will assume the length side and then put it into the expended ratio.

Let us assume that x be the length of the sides of the triangle.

Then we get the length of the sides of the triangle as 3x,6x,7x.

Let we assume x = 1 , then the sides of the triangle will be 3(1),(6(1),7(1) = 3,6,7(all in inches).

Let we assume x = 2 , then the sides of the triangle will be 3(2),6(2),7(2)=6,12,14 (all in inches).

So, the two sets will be 3,6,7​ and​ 6,12,14 (all in inches).

The lengths of the sides of a triangle are in the extended ratio 3:6:7 then two possible sets of side lengths, in inches, for the triangle will be: 3in,​6in,​7in 6in,​12in,​14in

 

Page 436  Exercise 6  Problem 8

In the given question, we have been given the solution of the proportion shown below.

We need to tell the error done in the solution of the proportion.

We will do the proportion and then multiply numbers diagonally to find the error.

Here we will find the proportion of \(\frac{7}{3}\) = \(\frac{4}{x}\).

We will multiply the numbers diagonally, we will get

​7x = 12

x = \(\frac{12}{7}\)

So the correct answer is x = \(\frac{12}{7}\).

And the error is in multiplying the number diagonally.

The correct solution is x = \(\frac{12}{7}\) not \(\frac{28}{3}\)  which is the error in the solution of the proportion shown.

 

Page 436  Exercise  7  Problem 9

We have been asked that what is a proportion that has means 6​and ​18 and extremes 9​ and ​12.

Since we know that when the proportion is stated with colons, the means are the two words that are closest together and when the proportion is stated with colons.

The extremes are the words in the proportion that are the farthest apart.

Thus the proportion will be 9:6 = 18:12.

As the first and the last number in a proportion are extremes and the middle two are the means.

A proportion that has means 6​ and ​18 and extremes 9 ​and ​12 is 6:9 = 18:12.

 

Page 436  Exercise 8  Problem 10

In the question, we have been given that the height of a table tennis net is 6​in. and the height of a tennis net is 3​ft.

We have been asked to write the ratio of the first measurement to the second measurement.

We will convert the height into inches and then divide them.

We have been given that the height of a table tennis net is 6​in and the height of a tennis net is 3​ft.

We know that 1​ft = ​12​in.

Thus, we will get ​3​ft ​= 3 × 12​in = 36​in

Now for the ratio, we will divide the height, we will get 6​in

36​in = \(\frac{1}{6}\)

= 1:6
​
So, the ratio is 1:6.

The ratio of the first measurement to the second measurement of the height of a table tennis net is 6​in and the height of a tennis net is 3​ft is 1:6.

 

Page 436  Exercise 9  Problem 11

We have been given that a baseball team played 154 regular-season games and the ratio of the number of games they won to the number of games they lost was \(\frac{5}{2}\)

We have been asked that how many games did they win and also how many games did they lose.

We will assume x and then use the given ratio to find the result.

Given to us that a baseball team played 154 regular-season games and the ratio of the number of games they won to the number of games they lost was 5:2.

Let the number of games be x, then the number of games they won will be 5x and the number of games they lose will be 2x.

Then we can write

​5x + 2x = 154

7x = 154

x = \(\frac{154}{7}\)

x = 22
​
Then the number of games they won will be 5 × 22 = 110.

And the number of games they lose will be 2 × 22 = 44.

A baseball team played 154 regular-season games and the ratio of the number of games they won to the number of games they lost was \(\frac{5}{2}\) then 110 games did they win and 44 games did they lose.

 

Page 436  Exercise 10  Problem 12

We have been given that the measures of two supplementary angles are in the ratio 5:7

We need to find the measure of the larger angle.

By using the sum of supplementary angles, we will find the result.

The measure of two supplementary angles is in the ratio 5:7.

Let us assume the measure of angle be x.

Then we can write 5x + 7x = 180°

Solving it, we will get

​12x = 180

x = \(\frac{180}{12}\)

x = 15
​
Then the measure of angles will be

​5 × 15 = 75

7 × 15 = 95
​
So, the measures of the angle of the larger angle is 95°.

The measures of two supplementary angles are in the ratio 5:7 then the measure of the larger angle is 95°.

 

Page 436  Exercise 11  Problem 13

In the given question, we have been given a proportion \(\frac{1}{3}\)= \(\frac{x}{12}\).

We have e  been asked to solve the given proportion.

Using cross multiplication, we will solve it.

Given to us a proportion \(\frac{1}{3}\)= \(\frac{x}{12}\).

By using the cross-multiplication, we can write

​1 × 12 = 3 × x

12 = 3x

\(\frac{12}{3}\) = x

4 = x  or  x = 4
​
So, the solution of the given proportion is x = 4.

The solution of the given proportion \(\frac{1}{3}\)= \(\frac{x}{12}\) is x = 4

 

Page 436  Exercise 12 Problem 14

From the given question, we have a proportion \(\frac{9}{5}\)= \(\frac{3}{x}\).

We have e been asked to solve the given proportion.

Using cross multiplication, we will solve it.

Given to us a proportion \(\frac{9}{5}\)= \(\frac{3}{x}\).

By using the cross-multiplication, we can write

​9 × x = 3 × 5

9x = 15

x = \(\frac{15}{9}\)

x = \(\frac{5}{3}\)

So, the solution of the given proportion is x = \(\frac{5}{3}\)

The solution of the given proportion \(\frac{9}{5}\)= \(\frac{3}{x}\) is x = \(\frac{5}{3}\)

 

Page 436  Exercise 13  Problem 15

In the given question, we have been given a proportion \(\frac{4}{x}\)= \(\frac{5}{9}\).

We have e been asked to solve the given proportion.

Using cross multiplication, we will solve it.

Given to us a proportion \(\frac{4}{x}\)= \(\frac{5}{9}\)

By using the cross-multiplication, we can write

​4 × 9 = 5 × x

36 = 5x

\(\frac{36}{5}\) = x
​
So, the solution of the given proportion is \(\frac{36}{5}\).

The solution of the given proportion \(\frac{4}{x}\)= \(\frac{5}{9}\) is \(\frac{36}{5}\).

 

Page 436  Exercise 14  Problem 16

We have been given a proportion \(\frac{y}{10}\)= \(\frac{15}{25}\).

We have e been asked to solve the given proportion.

Using cross multiplication, we will solve it.

Given to us a proportion \(\frac{y}{10}\)= \(\frac{15}{25}\).

By using the cross-multiplication, we can write

​y × 25 = 15 × 10

25y = 150

y = \(\frac{150}{25}\)

y = 6

So, the solution of the given proportion is 6.

The solution of the given proportion \(\frac{y}{10}\)= \(\frac{15}{25}\).

 

Page 436  Exercise 15  Problem 17

In this question, we are given the proportion \(\frac{9}{24}\) = \(\frac{12}{n}\)

We need to solve each proportion.

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the proportion \(\frac{9}{24}\) = \(\frac{12}{n}\)

We will solve by cross multiplication , we get the value of n

\(\frac{9}{24}\) = \(\frac{12}{n}\)

12 × 24 = 9 × n

\(\frac{288}{9}\) = n

32 = n or n = 32

The solution of the given proportion \(\frac{9}{24}\) = \(\frac{12}{n}\) after solving using cross multiplication got the value of n = 32.

 

Page 436  Exercise 16  Problem 18

In this question, we are given the proportion \(\frac{11}{14}\) = \(\frac{b}{21}\).

We need to solve each proportion.

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the proportion \(\frac{11}{14}\) = \(\frac{b}{21}\)

We will solve by cross multiplication, we get the value of b.

\(\frac{11}{14}\) = \(\frac{b}{21}\)

11 × 21 = 14 × b

\(\frac{231}{14}\) = b

16.5 = b

​The solution of the given proportion \(\frac{11}{14}\) = \(\frac{b}{21}\) after solving using cross multiplication got the value of b = 16.5.

 

Page 436  Exercise 17  Problem 19

In this question, we are given the proportion \(\frac{3}{5}\) = \(\frac{6}{x+3}\)

We need to solve each proportion.

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the proportion \(\frac{3}{5}\) = \(\frac{6}{x+3}\)

We will solve proportion using cross multiplication, we get the value of x

\(\frac{3}{5}\) = \(\frac{6}{x+3}\)

(x + 3)3 = 6 × 5 3x + 9 = 30
​
After simplifying, we get

​3x = 30 − 9

x = \(\frac{30−9}{3}\)

x = \(\frac{21}{3}\)

x = 7

​The solution of the given proportion \(\frac{3}{5}\) = \(\frac{6}{x+3}\) after solving using cross multiplication got the value of x = 7.

 

Page 436  Exercise 18  Problem 20

In this question, we are given the proportion \(\frac{y+7}{9}=\frac{8}{5}\)

We need to solve each proportion.

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the proportion \(\frac{y+7}{9}=\frac{8}{5}\)

We will solve by cross multiplication, we get the value of y

⇒  \(\frac{y+7}{9}=\frac{8}{5}\)

(y + 7)5 = 8 × 9

5y + 35 = 72
​
After solving, we get

​5y = 72 − 35

5y = 37

y = \(\frac{37}{5}\)

y = 7.4

​The solution of the given proportion \(\frac{y+7}{9}=\frac{8}{5}\) after solving using cross multiplication got the value of y = 7.4.

 

Page 436  Exercise 19  Problem 21

In this question, we are given the proportion \(\frac{5}{x-3}=\frac{10}{x}\)

We need to solve each proportion.

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the proportion \(\frac{5}{x-3}=\frac{10}{x}\)

We will solve by cross multiplication, we get the value of x

⇒ \(\frac{5}{x-3}=\frac{10}{x}\)

5 × x = 10(x − 3)

5x = 10x − 30

30 = 10x − 5x

​After solving, we get

​30 = 5x

\(\frac{30}{5}\) = x

6 = x or x = 6
​
The solution of the given proportion \(\frac{5}{x-3}=\frac{10}{x}\)after solving using cross multiplication got the value of x = 6.

 

Page 437  Exercise 20  Problem 22

In this question, we are given the diagram \(\frac{a}{b}=\frac{3}{4}\)

We need to complete each statement \(\frac{b}{4}=\frac{?}{?}\)

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the following information

⇒  \(\frac{a}{b}=\frac{3}{4}\)

To find the value of \(\frac{b}{4}\) we must multiply the numbers diagonally, we get

⇒  \(\frac{a}{b}=\frac{3}{4}\)

4 × a = 3 × b
​
Now we need to divide the equation with 4, we get
​
\(\frac{4a}{4}\) = \(\frac{3b}{4}\)
​
a = \(\frac{3b}{4}\)

And now with 3, we get

​\(\frac{a}{3}=\frac{3 b}{4 \times 3}\)

⇒ \(\frac{a}{3}=\frac{b}{4}\)

The solution of the given expression \(\frac{a}{b}\) = \(\frac{3}{4}\) using multiply the numbers diagonally is\(\frac{b}{4}=\frac{a}{3}\).

 

Page 437  Exercise 21  Problem 23

In this question, we are given the diagram \(\frac{a}{b}=\frac{3}{4}\)

We need to complete each statement \(\frac{7}{4}=\frac{?}{?}\)

We multiply the numerator of the first fraction with the denominator of the second fraction and the numerator of the second fraction with the denominator of the first fraction.

We are given the following information

⇒ \(\frac{a}{b}=\frac{3}{4}\)

To find the value of \(\frac{7}{4}\)

We need to add1 to both sides of the equation, we get

⇒ \(\frac{a}{b}=\frac{3}{4}\)

⇒  \(\frac{a}{b}+1=\frac{3}{4}+1\)

⇒  \(\frac{a+b}{b}=\frac{3+4}{4}\)

⇒  \(\frac{a+b}{b}=\frac{7}{4}\)

The solution of the given expression \(\frac{a}{b}=\frac{3}{4}\) by adding1 to both sides of the equation is \(\frac{7}{4}=\frac{a+b}{b}\).

Savvas Learning Co Geometry Student Edition Chapter 7 Similarity Exercise

 

Page 429  Exercise 1  Problem 1

In this question, we have been given a diagram and an angle ∠1.

We need to find the measure of an angle.

By using Supplementary angles, we will calculate the result.

When two angles add up to 180 degrees, they are considered to be supplementary angles.

A straight line is formed by the intersection of two angles, but the angles do not have to be the same.

So, we find the angle by subtracting 110 to the 180 degrees

​∠1 + 110° = 180°

∠1 = 180° − 110°

∠1 = 70°

​By using Supplementary angles, the measure of the given angle ∠1 is 70°

 

Page 429  Exercise 2  Problem 2

In this question, we have been given a diagram and an angle ∠2.

We need to find the measure of an angle.

By using Supplementary angles, we will calculate the result.

When two angles add up to 180 degrees, they are considered to be supplementary angles.

A straight line is formed by the intersection of two angles, but the angles do not have to be the same.

Since the previous exercise 1 , we have the value ∠1=70°.

So, we find the angle by subtracting 70 to the 180 degrees

​∠2+70° = 180°

∠2 = 180° − 70°

∠2 = 110°
​
By using Supplementary angles, the measure of the given angle ∠2 is 110°.

 

Page 429  Exercise 3  Problem 3

In this question, we have been given a diagram and an angle ∠3.

We need to find the measure of an angle.

By using Supplementary angles, we will calculate the result.

When two angles add up to 180 degrees, they are considered to be supplementary angles.

A straight line is formed by the intersection of two angles, but the angles do not have to be the same.

So, we find the angle by subtracting 110 to the 180 degrees

​∠3 + 110° =  180°

∠3 = 180°− 110°

∠3 = 70°

By using Supplementary angles, the measure of the given angle ∠3 is 70°.

 

Page 429  Exercise 4  Problem 4

In this question, we have been given a diagram and an angle ∠4.

We need to find the measure of an angle.

By using Supplementary angles, we will calculate the result.

When two angles add up to 180 degrees, they are considered to be supplementary angles.

A straight line is formed by the intersection of two angles, but the angles do not have to be the same.

Since the previous exercise 2, we have the value ∠2 = 110°.

So, we find the angle by subtracting110 to the 180 degrees

​∠4 + 110° = 180°

∠4 = 180° − 110°

∠4 = 70°
​
By using Supplementary angles, the measure of the given angle∠4 is 70°.

 

Page 429  Exercise 5  Problem 5

In this question, we have been given ΔPAC ≅ ΔDHL.

We need to complete the congruence statement.

By using the properties of Congruence of Triangles, we will calculate the result.

We have given the two congruent triangles ΔPAC and ΔDHL.

The two triangles are said to be congruent if all three sides of one triangle are comparable to the corresponding three sides of the second triangle by SSS using rule
​
PA = DH

AC = HL

PC = DL
​
Therefore, \(\overline{P C} \cong \overline{D L}\).

By using the properties of Congruence of Triangles, the complete congruence statement is \(\overline{P C} \cong \overline{D L}\).

 

Page 429  Exercise 6  Problem 6

In this question, we have been given ΔPAC ≅ ΔDHL.

We need to complete the congruence statement.

By using the properties of Congruence of Triangles, we will calculate the result.

The two triangles are said to be congruent if any two sides and the angle included between the sides of one triangle are equivalent to the corresponding two sides and the angle between the sides of the second triangle by using SAS rule.

​PA = DH

AC = HL
​
Therefore, ∠H ≅ ∠A

By using the properties of Congruence of Triangles, the complete congruence statement is ∠H≅∠A

 

Page 429  Exercise 7  Problem 7

In this question, we have been given ΔPAC ≅ ΔDHL.

We need to complete the congruence statement.

By using the properties of Congruence of Triangles, we will calculate the result.

We have given the two congruent triangles  ΔPAC and ΔDHL

The two triangles are said to be congruent if any two angles and the side included between the angles of one triangle are comparable to the corresponding two angles and sides included between the angles of the second triangle by using ASA rule.

From the previous Page 429  Exercise 6 Problem 6  we have the angle ∠A ≅ ∠H, PC ≅ DL

Therefore, ∠PCA ≅ DLH

By using the properties of Congruence of Triangles, the complete congruence statement is ∠PCA ≅ DLH.

 

Page 429  Exercise 8  Problem 8

In this question, we have been given ΔPAC ≅ ΔDHL.

We need to complete the congruence statement.

By using the properties of Congruence of

Triangles, we will calculate the result.

Since all the sides of the ΔDHL when we find the congruence for ΔHDL so, the triangles are congruent by SSS rule.

The two triangles are said to be congruent if all three sides of one triangle are comparable to the corresponding three sides of the second triangle.

Therefore, ΔHDL ≅ ΔAPC

By using the properties of Congruence of Triangles, the complete congruence statement is ΔHDL ≅ ΔAPC.

​
Page 429  Exercise 9  Problem 9

In this question, we have been given a triangle.We need to write a congruence statement for each pair of triangles.

By using the SSS rule, we will calculate the result.

The two triangles are said to be congruent if all three sides of one triangle are comparable to the corresponding three sides of the second triangle.

We have given the two triangles ΔHUG and ΔBUG in which we have given three equal sides

​HU = BU

HG = BG

UG=UG​(same​side)

Therefore, ΔHUG ≅ ΔBUG by SSS rule

A congruence statement for the pair of triangles is “The two triangles are said to be congruent if all three sides of one triangle are comparable to the corresponding three sides of the second triangle”. by SSS rule the triangles are congruent ΔHUG ≅ ΔBUG.

 

Page 429  Exercise 10  Problem 10

In this question, we have been given a triangle, and the value of

​BC = 12

EF = 4.7

We need to find the value of BF and DE.

By using the properties of the triangle, we will calculate the result.

As we know from the triangle diagram the side BC = BF + CF and BF = CF so to calculate the value of BF we substitute the value

​12 = BF + BF

12 = 2BF

\(\frac{12}{2}\)= BF

6 = BF or BF = 6
​
We know the value of EF which is equal to DE

By using the properties of the triangle, the value of BF is 6 . the value of EF is 4.7

 

Page 429  Exercise 11  Problem 11

In this question, we have been given a triangle, and the value of

​BC = 12

EF = 4.7
​
We need to find the value of AD and AC.

By using the properties of the triangle, we will calculate the result.

We can calculate the value of BE by Pythagoras theorem by substituting the values from the previous  Page 429  Exercise 10  Problem 10 in triangle ΔBEF

​BE² = BF² − EF²

BE² = 62 − 4.72

BE² = 13.91

BE = 3.72
​
Now, we calculate the value of AD by Pythagoras theorem by substituting the values from the previous  Page 429  Exercise 10  Problem 10  in triangle ΔADE

​AD² = 4.72 − 3.72

AD² = 4.72 − 3.72

AD = \(\sqrt{8.4}\)

AD = 2.9
​
By using the properties of the triangle, the value of BE is 3.72  the value of AD is 2.9.

 

Page 429  Exercise 12  Problem 12

In this question, we have been given an artist sketches a person.

She is careful to draw the different parts of the person’s body in proportion.

We need to find the meaning of proportion in this situation By using the proportion, we will calculate the result.

The meaning of proportion in this situation is the proper or suitable relationship between the size, shape, and orientation of different portions of anything

An artist creates a portrait of a person. She takes great care to draw the various sections of a person’s physique in proportion. The meaning of proportion in this situation is the proper or suitable relationship between the size, shape, and orientation of different portions of anything.

 

Page 429  Exercise 13  Problem 13

In this question, we have been given Siblings often look similar to each other.

We need to find the two geometric figures to be similarBy using the Geometric figures, we will calculate the result.

As we know Geometric figures are similar if they have the same shape but not necessarily the same size.

Siblings are frequently mistaken for one another. Geometric figures are similar if they have the same shape but not necessarily the same size.

 

Page 429  Exercise 14  Problem 14

In this question, we have been given a road map that has a scale on it that tells us how many miles are equivalent to a distance of 1 inch on the map.

We need to estimate the distance between the two cities on the map.

By using the Distance, we will calculate the result.

We first measure the distance between the two cities in inches, then using the given scale, we convert the distance to miles.

On a road map, there is a scale that shows how many miles are comparable to 1 inch on the map. To measure the distance between the two cities in inches, then using the given scale, convert the distance to miles.

Savvas Learning Co Geometry Student Edition Chapter 6 Polygons And Quadrilaterals Exercise 6.2 Properties of Parallelograms

 

Page 363  Exercise 1  Problem 1

Given: The parallelogram as 

To find –  The value of m∠A.

We will be using the properties of the parallelogram to find the above asked.

From the figure, we have ∠B = 127°.

Now as the opposite angles of the parallelogram are equal so we have 

∠B = ∠D

∠A = ∠C
​
By angle sum property we have

​∠A + ∠B + ∠C + ∠D = 360°

∠A + 127o + ∠A + 127°

=  360°

2∠A + 254°  =  360°

2∠A  =  106°

∠A  =  \(\frac{63}{2}\)

∠A  =  53°

The value of m∠A is 53°.

 

Page 363  Exercise 2  Problem 2

Given: The parallelogram as 

To find –  The value of m∠D.

We will be using the properties of the parallelogram to find the above asked.

From the given figure we have ∠B = 127°.

Now as the opposite angles of the parallelogram are equal so, we have 

∠B = ∠D

∠D = 127°

​The value of m∠D is 127°.

 

Page 363  Exercise 3  Problem 3

Given: The parallelogram as 

To find  – The value of x.

We will be using the properties of the parallelogram to find the above asked.

From the given figure, we have ​AB = x + 2

CD = 2x − 3.

Mow in a parallelogram the opposite sides are equal so, we have 

​AB = CD

x + 2 = 2x − 3

2x − x = 3 + 2

x = 5

The value of x is 5.

 

Page 363  Exercise 4  Problem 4

Given: The parallelogram as 

To find – The value of AB.

We will be using the properties of the parallelogram to find the above asked.

Now as we have that ABCD is a parallelogram, so the opposite sides are parallel and equal, that is AB = CD.

So, we have :

​x + 2 = 2x − 3

2x − x = 3 + 2

⇒  x = 5
​
From the given figure, we have AB = x + 2.

And we have x = 5, which gives

​AB = x + 2

AB = 5 + 2

AB = 7

​The value of AB is 7.

 

Page 363  Exercise 5  Problem 5

Given: The figure as 

To find – The value of ED,FD.

We will be using the theorem of parallel lines and the transversal dividing the lines.

From the given figure, we have

​AB = BC

= 8   and  FE = 12

Since, AB = BC so we know by theorem 6−7 that is three or more parallel lines cut off congruent segments on one transversal then they cut off congruent segments on every transversal.

So we have

⇒  ​FE = ED

ED = 12

​⇒ FD = FE + ED

= 12 + 12

= 24
​
The value of ED = 12 and FD = 24.

 

Page 363  Exercise 6  Problem 6

If we know one measure of angle, we can find the other three angles by using the angle sum property of a quadrilateral, which says that the sum of all the angles is 360o

Also, in the parallelogram the opposite angles are equal so, we can easily find the measure of three angles if we have the measure of one angle.

For example if in a parallelogram one angle is x , then the opposite angle will also be x and suppose the adjacent angle to x is y , so the opposite angle will also be y.

So by angle sum property we have x + x + y + y = 360°.

So if we have one known value of angle of a parallelogram, then we can find the other three by using angle sum property of the quadrilateral and by using the property of parallelogram that opposite angles are equal.

 

Page 363  Exercise 7  Problem 7

We may have the difference between quadrilateral and parallelogram written as -Quadrilaterals are polygons with four sides while parallelogram is a special type of a quadrilateral that has some special properties.

The four sides of quadrilateral cannot be equal or parallel but in a parallelogram the opposite sides are equal and parallel.

The angles in a Quadrilateral may vary and may not be all equal but in a parallelogram the opposite angles are equal.

The diagonals of a parallelogram divides the parallelogram into two congruent triangles, but this may not be necessary in a quadrilateral.

The diagonals of a parallelogram bisect each other but this is not necessary in a quadrilateral.

The difference between quadrilateral and parallelogram is given below :

⇒  Quadrilaterals are polygons with four sides while parallelogram is a special type of a quadrilateral that has some special properties.

⇒  The four sides of quadrilateral cannot be equal or parallel but in a parallelogram the opposite sides are equal and parallel.

⇒  The angles in a Quadrilateral may vary and may not be all equal but in a parallelogram the opposite angles are equal.

⇒  The diagonals of a parallelogram divides the parallelogram into two congruent triangles, but this may not be necessary in a quadrilateral.

⇒  The diagonals of a parallelogram bisect each other but this is not necessary in a quadrilateral.

 

Page 363  Exercise 8  Problem 8

Consider the figure given to us as

Now we are given that

​PR ≅ RT

QS = 5cm
​
PT and QV ae transversals of the lines PQ, RS,TV.

The classmate has applied the following theorem

Theorem: If three ( or more) parallel lines cut off congruent segments on one transversal, then they cut off congruent segments on every transversal.

Since, the lines PQ,RS and TV are not given to be parallel, therefore, the statement QV = 10 may not be correct.

The statement given by our classmate that is QV = 10 may not be correct because we are not given any lines to be parallel.

 

Page 364  Exercise 9  Problem 9

Given: We have the parallelogram with one angle as 53° and one as x°

The figure for the same is given below

To find – The value of x.

We will be using the properties of parallelogram to find the value of the given variable.

We have one angle of parallelogram as 53° as the opposite angles are equal in a parallelogram so the opposite angle will also be 53°.

Similarly, we have two angles measuring as x°.

By angle sum property of a quadrilateral we have

​53 + x + 53 + x = 360

2x + 106 = 360

2x = 360 − 106

2x = 254

x = \(\frac{254}{2}\)

x = 127°

​The value of x is given as 127°.

 

Page 364  Exercise 10  Problem 10

Given: We have the parallelogram with two angles given as 113° and x°.

The figure for the same is given below

To find –  The value of x.

We will be using the concept of properties of parallelogram to find the value of the unknown variable.

We are given one angle as 113°.

As opposite angles in parallelogram are equal to the other opposite angle will be 113°

Similarly, we have two angles as x°.

By angle sum property of quadrilateral, we have

​113 + x + 113 + x  =  360

2x + 226 = 360

2x = 134

x = \(\frac{134}{2}\)

x = 67°

​The value of x is given as 67°.

 

Page 364  Exercise 11  Problem 11

Given: The figure of the parallelogram is

To find –  We have to find the value of x.

We will analyze the given data and then apply the condition to solve the problem.

Given, The figure of the parallelogram is

As we now that, the opposite angles of a parallelogram are equal.

Thus , ​∠D = ∠B , ∠A = ∠B

​The angle sum property of a parallelogram tells that

​∠A + ∠B ∠C + ∠D = 180°

80° + x°+ 80° + x° = 180°

2x = 180° − 160°

x =\(\frac{20^{\circ}}{2}\)

x = 10°

Hence, the value of x = 10°.

The value of x in given parallelogram is 10°.

 

Page 364  Exercise 12  Problem 12

Given: The figure of the parallelogram is

To find – We have to find the value of x.

We will analyze the given data and then apply the condition to solve the problem.

Given, The figure of the parallelogram is

As we now that, the opposite angles of a parallelogram are equal.

Thus , ​∠D = ∠B , ∠A = ∠C

​The angle sum property of a parallelogram tells that

​∠A + ∠B + ∠C + ∠D = 180°

62° + x + 62° + x = 180°

2x  = 180° − 124°

x  =  \(\frac{56^{\circ}}{2}\)

x = 28°

Hence, the value of x  =  28°.

The value of x in given parallelogram is 28°.

 

Page 364  Exercise 13  Problem 13

Given: The figure of the parallelogram is

To find –  We have to complete the given table.

We will analyze the given data and then apply the condition to solve the problem.

Given, The figure of the parallelogram is

Since, ABCD is a parallelogram, which means.

Its opposite sides are parallel

Thus, AB ∥ CD

And ∠1  =  ∠4  (Alternate Interior angle Property)

Similarly ∠2 = ∠3

Since, opposite sides of a parallelogram are equal.

Thus, AB ≈ CD

In triangle AED & BEC

∠1  =  ∠4     (Alternate Interior Angle Property)

AD  =  BC   (Opposite Sides of a parallelogram are equal)

∠AED  =  ∠BEC   (Vertically Opposite Angle Property)

Hence, by ASA Congruency ΔAED ≅ ΔBEC.

The diagonals of a parallelogram bisects each other.

Thus, ​AE = CE, BE = DE

The bisector is a ray or a segment which divides the geometry in two identical halves.

In the parallelogram ABCD ,AC and BD bisects each other at E.

 

Page 364  Exercise 14  Problem 14

Given: The side lengths given are

​PT = 2x

TR = y + 4

QT = x + 2

TS = y
​
The figure of the parallelogram is

To find – We have to find the value of x and y.

We will analyze the given data and then apply the condition to solve the problem.

Given,  The side lengths given are

​PT = 2x

TR = y + 4

QT = x + 2

TS = y
​
The figure of the parallelogram is

Since, the diagonals of a parallelogram bisects each other.

So, PT = TR , QT = TS
​
Thus, the equation obtained are

2x = y + 4 and x + 2 = y

On solving the above equations, we get

​2x = (x + 2) + 4

2x − x = 4 + 2

x = 6

⇒  y = 6 + 2

⇒  y = 8
​
Hence, the value of  x = 6 and y = 8.

The value of x and y in given parallelogram is 6 and 8.

 

Page 364  Exercise 15  Problem 15

Given: The given sides are​

PT = x + 2

TR = y

QT = 2x

TS = y + 3
​
The figure of the parallelogram is

To find – We have to find the value of x and y.

We will analyze the given data and then apply the condition to solve the problem.

Given, The given side lengths are

​PT = x + 2

TR = y

QT = 2x

TS = y + 3
​
The figure of the parallelogram is

Since, the diagonals of a parallelogram bisects each other.

​PT = TR

QT = TS
​
So, the equations obtained are x + 2 = y and 2x = y + 3

On solving the above equations, we get

​2x = (x + 2) + 3

2x − x = 2 + 3

x = 5

⇒  y = 5 + 2

⇒  y = 7

Hence, the value of x = 5 and y = 7.

The value of x and y in given parallelogram is 5 and 7.

 

Page 364   Exercise 16  Problem 16

Given: The relation between the segments are PQ = QR = RS

The diagram given is

To find –  We have to find the length of XZ.

We will analyze the given data and then apply the condition to solve the problem.

Given, The side length given are

PQ = QR = RS

The diagram given is

Since, the if a line cuts the parallel lines of pair of three or more, then

Those parallel lines will divide the cutting lines in equal segments.

So, XT = XZ = ZU

As it is given that, the length of the segment is

XT = 3

Thus , ​XT = XZ

⇒  XZ = 3
​
Hence, the value of XZ = 3.

The value of XZ in the given figure is 3.

 

Page 364  Exercise 17  Problem 17

Given: The relation given is PQ = QR = RS

The diagram given is

To find – We have to find the length of TU.

We will analyze the given data and then apply the condition to solve the problem.

Given, The relation given is

PQ = QR = RS

The diagram given is

Since, the if a line cuts the parallel lines of pair of three or more, then

Those parallel lines will divide the cutting lines in equal segments.

So , XT = XZ = ZU

As it known that, the length of the segment XT = 3

Thus, TU = XT + ZX + ZU

TU = 3(XT)

TU = 3(3)

TU = 9
​
Hence, the value of TU = 9.

The value of TU in the given figure is 9.

 

Page 364  Exercise 18  Problem 18

Given:  The relation given is  PQ = QR = RS

The diagram given is

To find – We have to find the length of XV.

We will analyze the given data and then apply the condition to solve the problem.

Given, The relation given is

PQ = QR = RS

The diagram given is

Since, the if a line cuts the parallel lines of pair of three or more, then

Those parallel lines will divide the cutting lines in equal segments.

So, WY = YX = XV

As we know that, the length of the segment is

WY  =  2.25

Thus ,WY = XV

⇒  XV  =  2.25
​
Hence, the value of  XV  =  2.25.

The value of XV in the given figure is 2.25.

 

Page 364  Exercise 19  Problem 19

Given: The given relation is PQ = QR = RS

The diagram given is

To find –  We have to find the length of YV.

We will analyze the given data and then apply the condition to solve the problem.

Given, The given relation is, PQ = QR = RS

The diagram given is

Since, the if a line cuts the parallel lines of pair of three or more, then

Those parallel lines will divide the cutting lines in equal segments.

So , WY = YX = XV

As we know that, the length of the segment is

WY = 2.25

Thus , ​YV  =  YX + XV

YV  =  2XV

YV  =  2WY

YV  =  2(2.25)

YV  =  4.5
​
Hence, the value of YV = 4.5.

The value of YV in the give figure is 4.5.

 

Page 364  Exercise 20  Problem 20

Given: The given relation is PQ = QR = RS

The diagram given is

To find –  We have to find the length of WV.

We will analyze the given data and then apply the condition to solve the problem.

Given, The given relation is, PQ = QR = RS

The diagram given is

Since, the if a line cuts the parallel lines of pair of three or more, then

Those parallel lines will divide the cutting lines in equal segments.

So, WY = YX = XV

As we know that, the length of the segment is

WY = 2.25

Thus , ​WV = WY + YX + XV

WV  =  3WY

WV  =  3(2.25)

WV  =  6.75
​
Hence, the value of WV = 6.75.

The value of WV in the given figure is 6.75.

 

Page 364  Exercise 21  Problem 21

Given: The parallelogram given is

To find –  We have to find the values of each variables in the given parallelogram.

We will analyze the given data and then apply the condition to solve the problem.

Given, The parallelogram given is

As we now that, the opposite angles of a parallelogram are equal.

Thus , ∠A = ∠C

So, the angles are related as

4a − 4  =  2a + 30

4a − 2a  =  30 + 4

2a  =  34

a  =  \(\frac{34}{2}\)

a  =  17
​
Hence, the value of a  =  17.

The value of a in the given parallelogram is 17.

 

Page 365  Exercise 22  Problem 22

Given: The parallelogram given is

To find – We have to find the values of x and y and we have to find the relation between the angles.

We will analyze the given data and then apply the condition to solve the problem.

Given, The parallelogram given is

As we now that, the opposite angles of a parallelogram are equal.

So, ∠D = ∠B, ∠A = ∠C

​Thus, the angles are related as y = 3x, ∠C = 3y

​As we know the angle sum property of a parallelogram

​∠A + ∠B + ∠C + ∠D = 360°

3y + 3x + 3y + y = 360°

3y + y + 3y + y = 360°

8y  =  360°

y = \(\frac{360^{\circ}}{8}\)

y  =  45°

⇒   x = \(\frac{45^{\circ}}{3}\)

⇒   x = 15°

Hence, the value of x = 15°  and y = 45°.

The relation between angles in the given parallelogram is y = 3x and ∠C = 3y and the values of x andy are 15° and 45°.

 

Page 365  Exercise 22  Problem 23

Given: The parallelogram given is

To find – We have to find the values of x and y and also have to tell which variable is going to be solved first.

We will analyze the given data and then apply the condition to solve the problem

Given, The parallelogram given is

As we now that, the opposite angles of a parallelogram are equal.

So, ∠D = ∠B , ∠A = ∠C
​
Thus, the angles are related as y = 3x, ∠C = 3y
​
As we know the angle sum property of a parallelogram

​∠A + ∠B + ∠C + ∠D  =  360°

3y + 3x + 3y + y  =  360°

3y + y + 3y + y  =  360°

8y  =  360°

y = \(\frac{360^{\circ}}{8}\)

y  =  45°

⇒  x = \(\frac{45^{\circ}}{3}\)

⇒  x  =  15°

​Hence, the value of x  =  15° and y = 45°.

The variable first going to be solve is y m and the values of x and y are 15° and 45°.

 

Page 365  Exercise 23  Problem 24

Given: The parallelogram given is

To find – We have to find the length of given parallelogram.

We will analyze the given data and then apply the condition to solve the problem.

Given, The parallelogram given is

Since, the length of opposite sides of parallelogram are equal.

So, AD = BC , AB = CD
​
Thus, the sides are related as

​18.5  =  a − 3.5

a  =  18.5 + 3.5

a = 22

​The length of sides are

​CD = a + 1.6

⇒  22 + 16

⇒  38
​
​BC  =  a − 3.5

⇒  22.0 − 3.5

⇒  18.5
​
​AB = 2a − 20.4

⇒  2(22.0) − 20.4

⇒  44.0 − 20.4

⇒  23.6

Hence, the length of sides are​

CD = 38

BC = 18.5

AB = 23.6

AD 18.5.

​The length of sides of the given parallelogram are CD = 38, BC = 18.5, AB = 23.6, AD = 18.5.

 

Page 365  Exercise 24  Problem 25

Given: The parallelogram given is

To find – We have to find the value of a angle measure of given parallelogram.

We will analyze the given data and then apply the condition to solve the problem.

Given, The parallelogram given is

As we now that, the opposite angles of a parallelogram are equal.

So, ∠G = ∠K ∠H = ∠J

​Thus, the angles are related as

​20a + 30 = 17a + 48

20a − 17a = 48 − 30

3a = 18

a = 6
​
The angle measures are

​∠G  =  (20a + 30)°

⇒  (20(6) + 30)°

⇒  150°

​∠H = 5a°

⇒  5(6)°

⇒  30°

​∠J = ∠H

⇒ ∠J = 30°

​∠K = ∠G

⇒  ∠K = 150°

Hence, the angle measure are​

∠G = 150°

∠H = 30°

∠J = 30°

∠K = 150°.

​The values of angle measure of given parallelogram is​ ∠G = 150°, ∠H = 30°, ∠J = 30°, ∠K = 150°.

Savvas Learning Co Geometry Student Edition Chapter 6 Polygons And Quadrilaterals Exercise 6.1 The Polygon – Sum Theorems

 

Page 356  Exercise 1  Problem 1

Given:  We have given a regular decagon.

To find –  Measures of an interior angle and an exterior angle of a regular decagon.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides and the polygon exterior angle sum theorem.

We are given a regular decagon, that is the number of sides are n = 10.

The measure of an exterior angle of a decagon is given by

\(\frac{360^{\circ}}{10}\) = 36°

The sum of interior angles is given by:

​S  =  180 (n − 2)

S  =  180 (10 − 2)

S  =  180 (8)

S  =  1440°

So, we have the measure of an interior angle as

\(\frac{1440^{\circ}}{10}\) = 144°

The measures of an interior angle is 144° and an exterior angle is 36° of a regular decagon

 

Page 356  Exercise 2  Problem 2

An equiangular polygon means a polygon with the same measure of each interior angle.

We can draw an equiangular polygon that is not equilateral means it will have all the angle of same measure but not all the sides of same measure, that is a rectangle.

From the given figure, of the rectangle we may se that it has all the angles as right angle but not all the sides are of same measure.

Yes, we can draw an equiangular polygon that is not equilateral. For example we may draw a rectangle, that has all the angles as right angles but not all the sides of same measure.

 

Page 356  Exercise 3  Problem 3

We have the given figure as 

We know that an exterior angle is an angle which is formed by one of the sides of any closed shape structure such as polygon and the extension of its adjacent side.

From the given figure, we may see that the exterior angle of ∠1 can be given by ∠2,∠4

We see that ∠1,∠2 makes a linear pair and ∠1,∠4 also make linear pair. So the measure of the exterior angle ∠2, ∠4 can be given by 180° −∠1.

The exterior angles of ∠1 are given by ∠2, ∠4.

The measure of these angles can be given by 180° −∠1 as both the exterior angle makes a linear pair with the given interior angle.

 

Page 356  Exercise 4  Problem 4

Given:  We have given a 35 – polygon.

To find –  The sum of interior angles.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

We have the given polygon with total number of sides as n = 35.

The sum of interior angles of a polygon can be given by

S = 180 (n − 2), where n is the number of sides.

So, we have

​S  =  180 (35 − 2)

S  =  180 (33)

S  =  5940°

The sum of the interior angle measures of the given polygon is 5940°.

 

Page 356  Exercise 5  Problem 5

Given:  A  14 − polygon.

To find –  The sum of interior angles.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

We have the given polygon with total number of sides as n = 14.

The sum of interior angles of a polygon can be given by

S = 180 (n−2), where n is the number of sides.

So, we have

​S  =  180 (14 − 2)

S  =  180 (12)

S  =  2160°

The sum of the interior angle measures of the given polygon is 2160°.

 

Page 356   Exercise 6   Problem 6

Given: The regular polygon as

To find – The measure of one interior angle in regular polygon.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

From the given figure, we have the number of sides of the given regular polygon as n = 8.

The sum of all interior angles is given by:

​S  =  180 (n − 2)

S  =  180 (8 − 2)

S  =  180 (6)

S  =  1080°

Measure of one interior angle is given by

=  \(\frac{1080^{\circ}}{8}\)

=  135°

The measure of one interior angle is 135° in the given regular polygon.

 

Page 356  Exercise 7  Problem 7

Given: The figure as showing a quadrilateral as follows

To find –  The missing angles.

We will be using the concept of sum of all interior angles to find the measure of missing angle.

We are given a quadrilateral with angle measures as – 2h°, 2h°, h°, h°.

By angle sum property of quadrilateral, we have:

​2h + h + 2h + h = 360°

6h = 360°

h = 60°

So we have

​2h  =  2 (60°)

​2h  =  120°

h  =  \(\frac{120^{\circ}}{2}\)

h  =  60°

​The angle measure of the given quadrilateral are 120°,120°,60°, 60.

 

Page 356  Exercise 8  Problem 8

Given: The figure representing a pentagon as follows 

To find –  The missing angles.

We will be using the concept of sum of all interior angles to find the measure of missing angle.

​We are given a pentagon with some angle measures as – 117°,100°,105°,115°,x°.

By angle sum property of a pentagon, we have:

117° + 100° + 105° + 115° + x  =  540°

x + 437° = 540°

x  =  540° − 437°

x  =  103°

The measure of missing angle is 103° for the given pentagon.

 

Page 356  Exercise 9  Problem 9

Given: We have given a pentagon.

To find  – The measure of an exterior angle of the given regular polygon.

We will be using the concept of polygon exterior angle-sum theorem.

We are given a pentagon, that means the number of sides are n = 5.

By polygon exterior angle sum theorem, we have that the measure of an exterior angle of a regular polygon is the ratio of the whole angle by the number of sides of the polygon.

So, we have the measure of an exterior angle of given polygon as

\(\frac{360^{\circ}}{5}\)  =  72°

The measure of an exterior angle is 72° of the given pentagon.

 

Page 356  Exercise 10  Problem 10

Given:  We have given a 36 −polygon.

To find  – The measure of an exterior angle of the given regular polygon.

We will be using the concept of polygon exterior angle-sum theorem.

We are given a 36 − polygon, that means the number of sides are n =  36.

By polygon exterior angle sum theorem, we have that the measure of an exterior angle of a regular polygon is the ratio of the whole angle by the number of sides of the polygon.

So, we have the measure of an exterior angle of given polygon as

\(\frac{360^{\circ}}{36}\) = 10°

The measure of an exterior angle is 10o of the given 36 –  polygon.

 

Page 356  Exercise 11  Problem 11

Given: We have given a 100 − polygon.

To find –  The measure of an exterior angle of the given regular polygon.

We will be using the concept of polygon exterior angle-sum theorem.

We are given a 100 − polygon, that means the number of sides are  n   = 100.

By polygon exterior angle sum theorem, we have that the measure of an exterior angle of a regular polygon is the ratio of the whole angle by the number of sides of the polygon.

So, we have the measure of an exterior angle of given polygon as

\(\frac{360^{\circ}}{100}\) = 3.6°

The measure of an exterior angle is 3.6° of the given 100 − polygon.

 

Page 356  Exercise 12  Problem 12

Given: The sum of interior angles of a polygon is given as 180°.

To find – The number of sides of the polygon.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

We are given the sum of interior angles of the polygon as S = 180°

By using the formula for finding the sum, we have:

S = 180 (n−2), where is n the number of sides.

So we have

​180 = 180 (n − 2)

1  =  n − 2

n  =  2 + 1

n  =  3
​
The number of sides are 3 when the sum of interior angles is given as 180° for a polygon.

 

Page 356  Exercise 13 Problem 13

Given:  The sum of interior angles of a polygon is given as 1080°.

To find –  The number of sides of the polygon.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

We are given the sum of interior angles of the polygon as S = 1080°.

By using the formula for finding the sum, we have:

S = 180 (n − 2), where n is the number of sides.

So we have

​1080 = 180 (n − 2)

\(\frac{1080}{180}\) = n − 2

6 = n − 2

n = 6 + 2

n = 8

​The number of sides are 8 when the sum of interior angles is given as 1080° for a polygon.

 

Page 356  Exercise 14  Problem 14

Given:  The sum of interior angles of a polygon is given as 1980°.

To find – The number of sides of the polygon.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

We are given the sum of interior angles of the polygon as 1980°.

By using the formula for finding the sum, we have:

S = 180 (n − 2), where nis the number of sides.

So we have

​1980 = 180 (n − 2)

\(\frac{1980}{180}\) = n − 2

11 = n − 2

n = 11 + 2

n = 13
​
The number of sides are 13 when the sum of interior angles is given as 1980° for a polygon.

 

Page 356  Exercise 15  Problem 15

Given: The sum of interior angles of a polygon is given as 2880°.

To find – The number of sides of the polygon.

We will be using the concept of finding the sum of interior angles of the polygon with a given number of sides.

We are given the sum of interior angles of the polygon as 2880°.

By using the formula for finding the sum, we have:

S = 180 (n − 2), where n is the number of sides.

So we have

​2880 = 180 (n − 2)

\(\frac{2880}{180}\) = n − 2

16 = n − 2

n = 16 + 2

n = 18
​
The number of sides are 18 when the sum of interior angles is given as 2880° for a polygon.

 

Page 357  Exercise 16  Problem 16

We know that Polygon is a representation of the surface and is formed using a collection of lines.

An polygon that can is equilateral but not equiangular can be given by a rhombus.

As a rhombus have all equal sides, but the angles of the rhombus are not equal.

We may sketch the rhombus as follows:

The sketch of an equilateral polygon that is not equiangular is a rhombus that is given below: 

 

Page 357   Exercise 17   Problem 17

We are given that a triangle has two congruent interior angles and an exterior angle that measures 100°.

So, we may draw the diagram for the triangle as:

Now, here we see that the two interior angles that are congruent to each other are given by y, and other interior angle is given by x.

Diagram thus helps us to find the values of the missing angle in any polygon.

The diagram helps us to find the missing angles in a polygon, which is only possible if we have the diagram with the given values of angles and the missing values as variables.

 

Page 357  Exercise 17  Problem 18

We are given a triangle has two congruent interior angles and an exterior angle that measures 100°

Also, we have the diagram for the given triangle as follows:

Now by angle sum property of a triangle we know that the sum of all the interior angles of a triangle is always 180°.

We can also verify this by the formula S = 180(n − 2), where n is the number of sides and we will get :

​S = 180 (3 − 2)

S = 180° (1)

S =  180°

The sum of the angle measures in a triangle is always 180°.

​

Page 357  Exercise 18  Problem 19

Given: The figure as

To find  – The value of each variable.

We will be using the angle sum property of the polygon and the linear pair property to find the missing values.

From the given figure as, we see that z,110o form a linear pair, so we have :

z +110° = 180°

z = 180° − 110°

z = 70°

Now by angle sum property of a quadrilateral, we have:

​z + y + 100° + 87° =  360°

70° + y + 100°+ 87° = 360°

y + 257°  = 360°

y = 360° − 257°

y = 103°

The value of variable are ​y = 103°, z = 70°.

 

​Page 357  Exercise 19  Problem 20

Given: The figure as 

To find –The value of each variable.

We will be using the angle sum property of the polygon and the linear pair property to find the missing values.

We know by polygon exterior angle sum property that the sum of all exterior angles is 360°, so here we have:

​z + (z − 13°) + (z + 10°) = 360°

z + z + z − 13°+ 10° =  360°

3z = 360° + 3

z  =  \(\frac{363}{3}\)

z  =  121°

​Now, we that each each interior angle makes linear pair with the exterior angle, so we have:
​
z + x = 180°

x = 180° − z

x = 180° − 121°

x = 59°

As, (z − 13° ) makes linear pair with w so we have:

​z − 13° + w = 180°

w = 180° − 13° − 121°

w = 46°

Again (z​ + 10o) makes a linear pair with y, so we have:

​z + 10° + y = 180°

y = 180° − 10°− 121°

y = 49°

​The values of the variables are :- ​x = 59° ,y = 49° ,z = 121° ,w = 46°

Savvas Learning Co Geometry Student Edition Chapter 6 Polygons And Quadrilaterals Exercise

 

Page 349  Exercise 1  Problem 1

Given: The figure as 

To find  – The value of x.

We will be using the concept of co-interior angles to find the value of the unknown variables.

From the given figure, we see that since the opposite sides of the given parallelogram are equal, so the angles formed are co-interior angles.

As co-interior angles are supplementary, so we have:

​(3x − 14) + (2x − 16) = 18

5x − 30 = 180

5x = 180 + 30

5x = 210

x = \(\frac{210}{5}\)

x = 42°

​The value of x = 42°

 

Page 349  Exercise 2  Problem 2

Given: The figure as 

To find –  The value of x.

We will be using the concept of corresponding angles to find the value of unknown variable.

From the given figure, we have the lines parallel to each other, so the corresponding angles will be equal.

So, we have

​5x  = 176 − 3x

5x + 3x  =  176

8x = 176

x  =  \(\frac{176}{8}\)

x  =  22°

​The value of x = 22°.

 

Page  349  Exercise 3  Problem 3

We have the given figure as

From, the figure, we see that we have been given a quadrilateral with four sides and one diagonal.

Also, we see that ∠ABC = ∠BCD.

Now, we know that if the lines are parallel then the corresponding angles are equal.

Here, the corresponding angles are equal, which means that the lines AB ∥ CD

The line AB is parallel to CD.

 

Page 349  Exercise 4  Problem 4

Given: The figure as

To check  –  If AB ∥ CD.

We will be using the concept of corresponding angles, to check the above asked.

Now, we know that AB is parallel to CD if the corresponding angles formed by these lines are equal, that is if

∠CDF = ∠FAB

Let us consider the ΔCFD by angles sum property, we have:

​2x + 4x + 3x = 180

9x = 180

x =  \(\frac{180}{9}\)

x  =  20°

So we have

∠CDF = 4x

=   4 × 20

=   80°

∠FAB = (3x + 18)

=  3 × 20 + 18

=  60 + 18

=  78°

As ∠CDF ≠ ∠FAB, thus the lines are not parallel.

The line AB is not parallel to CD.

 

Page 349  Exercise 5  Problem 5

Given: The figure as

To check:  If AB ∥ CD.

We will be using the concept of co-interior angles to check if the two lines are parallel or not.

From the figure, we have the pair of vertically opposite angles as

​2x + 11 = 3x − 9

3x − 2x = 11 + 9

x = 20°

So, now we know that AB ∥ CD if the co-interior angles are supplementary

​3x − 9 = 3 × 20 − 9

=  60 − 9

=  51°

6x + 9 = 6 × 20 + 9

=  120 + 9

=  129°

​This gives

(3x − 9) + (6x + 9) = 180

51°+ 129° = 180°

180° = 180°

Which is true.

Thus, the lines are parallel.

The line AB is parallel to CD.

 

Page 349  Exercise 6  Problem 6

Given:  The lines as

⇒  ​y = −2x

⇒  y − 2x + 4
​
To check: Is the lines are parallel, perpendicular or neither.

We will be calculating the slope of each line and check if they are equal than the slopes are parallel and if the product of the slopes is −1 then they are perpendicular.

We have the line as

y = −2x

Now, comparing the equation with the slope-intercept form y = mx + c gives the slope as:

m₁ = −2

The other line is

y = −2x + 4

Now, we have the slope for this as

m₂ = −2

This implies

m₁ = m₂

Thus, the lines are parallel.

The given lines ​y = −2x, y = −2x + 4 are parallel.

 

Page 349  Exercise 7  Problem 7

Given: The lines as

⇒ ​y  =  \(\frac{−3}{5}\)  x + 1

⇒  y  = \(\frac{5}{3}\) x − 3
​
To check:  Is the lines are parallel, perpendicular or neither.

We will be calculating the slope of each line and check if they are equal than the slopes are parallel and if the product of the slopes is −1 then they are perpendicular

We have the line as

⇒  ​y = \(\frac{−3}{5}\)  x + 1

This gives us the slope as m₁ = \(\frac{−3}{5}\)

Now the other line is given as

⇒  y = \(\frac{5}{3}\) x − 3

So we have the slope as m₂  =  \(\frac{5}{3}\)

Now since the two slopes are not equal this means the lines are not parallel.

Now, we have

m₁ m₂  = \(\frac{−3}{5}\) × \(\frac{5}{3}\)

= −1

Thus, the lines are perpendicular.

The given lines ​y = \(\frac{−3}{5}\) x + 1 , y =\(\frac{5}{3}\) x − 3 are perpendicular.

 

Page 349  Exercise 8  Problem 8

We are given the following figure as

From the given figure, we see that we have a parallelogram with two triangles as  ΔABC, ΔADC

Now, in the two triangles we see that the opposite sides of the parallelogram are equal and the one is the diagonal that is common between the two triangles.

That means, the two triangles are congruent using the SSS congruence rule.

The postulate or theorem that makes each pair of triangles congruent in the given figure is the Side-Side-Side (SSS) congruence rule.

 

Page 349   Exercise 9   Problem 9

We are given the following figure as

From the given figure, we see that we have two triangles.

The base of both the triangles are given to be equal and the perpendicular is common in both the triangles.

Now, we see that the angle formed by the perpendicular is right angle angle in both the triangles, which gives that hypotenuse must be equal in both the triangles.

Thus, both the triangles are congruent by Right angle-Hypotenuse-Side (RHS) rule.

The postulate or theorem that makes each pair of triangles congruent is Right angle-Hypotenuse-Side (RHS) congruence rule.

 

Page 349  Exercise 10  Problem 10

We are given the figure ass

 

From the given figure, we see that we have two triangles.

The base of both the triangles are given to be equal and the angle formed on the base is right angle.

Since in both the triangles, angles are equal and length of both the angles are equal, this implies that the other leg of the angle must be equal.

Thus, both the triangles are congruent by Side Angle Side (SAS) rule.

The postulate or theorem that makes each pair of triangles congruent is Side-Angle-Side (SAS) congruence rule.

 

Page 349  Exercise 11  Problem 11

The word or the term “equilateral” means having all its sides of the same length.

For Example: An equilateral triangle, which means that the triangle where all the sides are of same measure or the same length.

Similarly, if we think about an equilateral polygon, we know that it is such a kind of polygon where all the sides of the it are equal.

Suppose let us take and example of polygon that is – a pentagon. The figure given below have all the sides of same measure, thus forming a pentagon with five equal sides.

The word “equilateral” means having all its sides of the same length. The equilateral polygon is the polygon where all the sides are of same length or measure.

 

Page 349  Exercise 12  Problem 12

Now, we see that a Kite is a flat shape with straight sides. The kite is a quadrilateral with four sides.

Kite is diamond shape quadrilateral.

The geometrical characteristics of the kite are given below:

⇒  Two pair of adjacent sides are equal

⇒  The intersection of the diagonals of a kite form 90o (right) angles.  This means that they are perpendicular.

⇒  The longer diagonal of a kite bisects the shorter one.  This means that the longer diagonal cuts the shorter one in half.

⇒  The angles between the two pairs of equal adjacent sides are equal to each other.

A kite can be defines as a diamond shaped quadrilateral. The geometrical characteristics of the kite are as follows :- there are two pair of adjacent sides that are equal, the diagonals are perpendicular to each other, the longer diagonal bisects the shorter diagonal and the angles between the two pair of adjacent sides are equal.

 

Page 349  Exercise 13  Problem 13

We know that when a team wins two consecutive gold medals, it means they have won two gold medals in a row.

This gives us the meaning of the term or the word “consecutive” as following each other continuously without interruption.

If we talk about a quadrilateral, then two consecutive angle will be one after the other, which means if one angle is ∠A, then the consecutive angle can be given by its adjacent angle ∠B
and so on.

Thus, the consecutive angle in a quadrilateral are the angles following each other without the interruption of any other angle.

The consecutive angle in a quadrilateral are the angles following each other without the interruption of any other angle.

Savvas Learning Co Geometry Student Edition Chapter 5 Relationships Within Triangles Exercise 5.1 Midsegments Of Triangles

 

Page 288  Exercise 1 Problem 1

From the given figure we can see that \(\overline{N O}\) is parallel to \(\overline{J K} \)

The segment \(\overline{N O}\) is parallel to \(\overline{J K} \).

 

Page 288  Exercise 2  Problem 2

Given: Length of the side of the triangle.

To Find – Length of NM.

Use the relationship between the length of a midsegment and the length of the side of a triangle.

We have,LK = 46

Using the relationship

Length of a midsegment = \(\frac{1}{2}\) (length of the third side)

NM  =  \(\frac{1}{2}\)(46)

NM = 23

The length of the midsegment NM is 23.

 

Page 288  Exercise 3  Problem 3

A line segment that connects two midpoints of the sides of a triangle is called a midsegment.

It is a line that is drawn from one side to another, parallel to the other side.

A midsegment connects two mid points of the side of a triangle.

 

Page 288  Exercise 4  Problem 4

The two noncollinear segments in the coordinate plane have the same slope, which means that the segments are neither perpendicular nor intersecting.

Thus, the two segments are parallel to each other which is the reason that the slopes of both the noncollinear segments are the same.

The two noncollinear segments are parallel to each other in the coordinate plane.

 

Page 288  Exercise 5  Problem 5

In the given figure, we know that NP = PT.

Which means that P is the midpoint of the side NT.

On the other hand, there is no information if OL is equal to LT, so we can say that L is not the midpoint of the side OT.

Thus, we can say that the conclusion given by the student is not correct and PL is not parallel to NO.

The error in the student’s reasoning is that L is not the midpoint of the side OT.

 

Page 288  Exercise 6  Problem 6

Given: A segment of the triangle.

To Find – The segment that is parallel to the given segment.

Use the triangle midsegment theorem.

We have the given segment, AB of the triangle.

Using the triangle midsegment theorem, AB ∥ FE.

The segment parallel to the segment AB is the segment FE.

 

Page 288  Exercise 7  Problem 7

Given: A segment of the triangle.

To Find – The segment that is parallel to the given segment.

Use the triangle midsegment theorem.

We have the given segment,BC of the triangle

Using the triangle midsegment theorem, BC ∥ GF

The segment that is parallel to the segment BC is the segment GF.

 

Page 288  Exercise 8  Problem 8

Given: A segment of the triangle.

To Find – The segment that is parallel to the given segment.

Use the triangle midsegment theorem.

We have the given segment,EF of the triangle ABC.

Using the triangle midsegment theorem, EF ∥ AB

The segment parallel to the segment EF is the segment AB.

 

Page 288  Exercise 9  Problem 9

Given: A segment of the triangle ABC.

To Find – The segment parallel to the given segment.

Use the triangle midsegment theorem.

We have the given segment,CA of the triangle ABC.

Using the triangle midsegment theorem, CA ∥ EG

The segment parallel to the segment CA is the segment EG.

 

Page 288  Exercise 10  Problem 10

Given: A segment of the triangle ABC.

To Find –  The segment parallel to the given segment.

Use the triangle midsegment theorem.

We have the segment,EG of the triangle FGE

Using the triangle midsegment theorem, EG ∥ CA

The segment parallel to the segment EG is the segment CA.

 

Page 288  Exercise 11  Problem 11

Given: Midpoints and lengths of the sides of the ΔTUV.

To Find – The length of HE.

Use the triangle midsegment theorem.

We have

UV = 80

TV = 100 and HD = 80 also,E,D and H are the midpoints of ΔTUV

Using the triangle midsegment theorem

HE = \(\frac{1}{2}\) VU

HE = \(\frac{1}{2}\) (80)

HE = 40

The length of HE is 40 units.

 

Page 288  Exercise 12  Problem 12

Given: Midpoints and lengths of the sides of the ΔTUV.

To Find –  The length of ED.

Use the triangle midsegment theorem.

We have

UV = 80, TV = 100 and HD = 80 also E,D and H are the midpoints of the sides of the ΔTUV

Using the triangle midsegment theorem

ED = \(\frac{1}{2}\) TV

ED = \(\frac{1}{2}\) (100)

ED = 50

The length of ED is 50 units.

 

Page 288  Exercise 13  Problem 13

Given: HD = 80

To Find  –  The length of TU

In triangle TUV by midpoint theorem.

​HD = \(\frac{1}{2}\) TU

80 = \(\frac{1}{2}\) TU

TU = 160

​The value of TU = 160

 

Page 288  Exercise 14  Problem 14

Given: HD = 80

To Find – The length of TE

In triangle TUV by mid point theorem.

​HD = \(\frac{1}{2}\) TU

80 = \(\frac{1}{2}\) TU

TU = 160

TU = 2 TE

TE = \(\frac{TU}{2}\)

TE = \(\frac{160}{2}\)

TE = 80

The value of TE = 80

 

Page 288  Exercise 15  Problem 15

Given:  A diagram

To Find – The value of x

In the triangle by midpoint theorem, segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side

SO,  x = \(\frac{1}{2}\) × 26

⇒  x = 13
​
The value of x = 13

 

Page 288  Exercise 16  Problem 16

Given: A diagram

To Find  – The value of x

In the triangle by midpoint theorem, segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side

SO,  5x = \(\frac{1}{2}\) × 45

⇒  x  = 4.5

The value of x = 4.5

 

Page 288  Exercise 17  Problem 17

Given: A diagram

To Find – The value of x

In the triangle by midpoint theorem, segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side

So​, 6x = \(\frac{72}{2}\)

⇒  x  =  6
​
The value of x = 6

 

Page 289  Exercise 18  Problem 18

Given: A diagram

To Find – The value of x

In the triangle by midpoint theorem, segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side

So ​x − 4  =  \(\frac{1}{2}\) × 17

⇒  x  =  \(\frac{17}{2}\) + 4

⇒  x  =  12.5
​
The value of x = 12.5

 

Page 289  Exercise 19  Problem 19

Given: A diagram

To Find –  The value of x

In the triangle by midpoint theorem, segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side

So​ x + 2 =   \(\frac{1}{2}\)  × 38

⇒ x = 19 − 2

⇒  x = 17

​The value of x = 17

 

Page 289  Exercise 20 Problem 20

Given: A diagram

To Find –  The value of x

In the triangle by midpoint theorem, segment joining two sides of a triangle at the midpoints of those sides is parallel to the third side and is half the length of the third side.

So, ​5x − 4 = \(\frac{1}{2}\) × 8

⇒  5x = 8

⇒  x = \(\frac{8}{5}\)

⇒  x = 1.6
​
The value of x = 1.6.

 

Page 289  Exercise 21  Problem 21

Given: The number of strides is shown in the triangle and the average stride is 3.5 ft

To Find  – The length of the longest side of the triangle.

In the triangle, the longest side will be in which maximum strides are paddled so the maximum number of strides is 250

The length of the longest side ​= 3.5 × 250

⇒  875ft
​
The length of the longest side is 875ft

 

Page 289  Exercise 21  Problem 22

Given: The sides of the triangle.To find: Distance to be paddled across the lake.

Let ,a,b, c be sides of the triangle.

a = 80 + 80 = 160  (Say)

b = 250  (Say)

c = 150 + 150 = 300 (Say)

Distance to be paddled across the lake = The perimeter of the triangle × 3.5

=  (a + b + c) × 3.5

=  (160 + 250 + 300) × 3.5

=  710 × 3.5

=  2485 ft

The distance to be paddled across the lake is 2485ft.

 

Page 289  Exercise 22  Problem 23

Given: The following triangular face of the Rock and Roll Hall of Fame in Cleveland, Ohio, that is isosceles is provided with congruent red segments and the length of the base is 229 feet 6 Inches or 229 ft 6 in.

Converting the given measurement of the base into inches 229 ft 6 in

=  (229 × 12) + 6 in

=  2748  +  6 in

=  2754 in

The red segments divide the legs into four congruent parts and the white segment divides each leg into two congruent parts. The white segment is a midsegment of the triangular face of the building, so its length is half the length of the base.

So, the length of the white segment

=  \(\frac{2754}{2}\)

=  1377 in

Converting to feet and inches

Length of the white segment

=   \(\frac{1377}{12}\) ft

=   114. 75 ft

=   114 ft (0.75 × 12) in

=  114 ft 9 in

The length of the white segment is 114 ft 9in.

 

Page 289  Exercise 23  Problem 24

Given: A figure.

To find –  m ∠ QPR

S is the mid-point of QP

T is the mid-point of QR

Using the mid-point theorem, we get  ST ∥ PR

QP is a transversal and  ST ∥ PR

Therefore,∠QPR = ∠QST  (Corresponding angles)

∠QPR = 40°  (Since,∠QST  =  40°)

Using the mid-point theorem,m ∠QPR = 40°.

 

Page 289  Exercise 24  Problem 25

Given: The coordinates of the vertices of a triangle  EFG.

To find – The coordinates of H & J.

Since, H is the mid-point of EG

Therefore, coordinates of

H = \(\left(\frac{1+3}{2}, \frac{2-2}{2}\right)\)

H = (2,0)

Since, J is the mid-point of FG

Therefore, coordinates of

J = \(\left(\frac{5+3}{2}, \frac{6-2}{2}\right)\)

J = (4,2)

The coordinates of H = (2,0) and of J = (4,2).

 

Page 289  Exercise 24  Problem 26

Given:  H,  J are the mid-points of the sides EG, FG respectively.

To prove – HJ ∥ EF

Since, H,J are the mid-points of the sides EG, FG respectively.

Therefore, using the mid-point theorem we get, HJ ∥ EF

Using the mid-point theorem we get, HJ ∥ EF

 

Page 289  Exercise 24  Problem 27

Given: H,J are the mid-points of the sides EG, FG respectively.

To prove –  HJ = \(\frac{1}{2}\) EF

Since, H,J  are the mid-points of the sides EG,FG, respectively.

Therefore, using the mid-point theorem we get

HJ = \(\frac{1}{2}\) EF

Using the mid-point theorem we get,HJ = \(\frac{1}{2}\) EF

 

Page 289  Exercise 25  Problem 28

Given: X,Y are the mid-points of the sides UV, UW respectively.m∠UXY = 60

To find – m∠V

Since, X, Y are the mid-points of the sides UV, UW respectively.

Therefore, using the mid-point theorem we get, XY ∥ VW

Hence,∠V = ∠UXY (Corresponding angles)

m∠V = 60 (Since m∠UXY = 60)

According to the question,m∠V = 60°.

 

Page 289  Exercise 26  Problem 29

Given: X,Y are the mid-points of the sides UV,UW respectively.

To find –  m∠UYX

Since ,X,Y are the mid-points of the sides UV, UW respectively.

Therefore, using the mid-point theorem we get, XY ∥ VW

Hence,∠UYX = ∠W (Corresponding angles)

m∠UYX = 45 (Since m∠W = 45)

According to the given condition, m∠UYX = 45°