Integral Transformations Exercise 7(b)
1. Evaluate \(\oint_C\)(3x+4y) dx+(2x-3y) dy where C is a circle x2+y2=4
Solution:
Given \(\oint_C(3 x+4 y) d x+(2 x-3 y) d y\). Here \(\mathbf{P}=3 x+4 y, \mathbf{Q}=2 x-3 y\)
By Green’s theorem \(\int_C \mathbf{P} d x+\mathbf{Q} d y=\iint_S\left(\frac{\partial \mathbf{Q}}{\partial x}-\frac{\partial \mathbf{P}}{\partial y}\right) d x d y\)
= \(\iint_S(2-4) d x d y=-2 \int_S d \mathrm{~A}=-2 \mathrm{~A}=-2 \pi(2)^2=-8 \pi\)
2. Find \(\oint_C\)(x2-2xy)dx+(x2y+z)dy around the boundary C of the regeion defined by y2= 8x and x=2 by Green’s theorem.
Solution:
Given \(\oint\left(x^2-2 x y\right) d x+\left(x^2 y+2\right) d y\) .
P = \(x^2-2 x y, \mathrm{Q}=x^2 y+2\)
By Green’s theorem the given integral
= \(\iint_S\left(\frac{\partial \mathbf{Q}}{\partial x}-\frac{\partial \mathbf{P}}{\partial y}\right) d x d y=\iint_S(2 x y+2 x) d x d y\)
For the region \(y^2=8 x\) and x=2
x varies from 0 to 2 . y varies from \(-\sqrt{8 x}\) to \(\sqrt{8 x}\).
⇒ \(\int_{x=0}^2 \int_{y=-\sqrt{8 x}}^{\sqrt{8 x}} 2 x(y+1) d x d y=\int_0^2 2 x\left[\frac{y^2}{2}+y\right]_{-\sqrt{8 x}}^{\sqrt{8 x}} d x=+8 \sqrt{2} \int_0^2 x^{3 / 2} d x=\frac{128}{5}
\)
3. Find the area bounded by one arc of the cycloid x=a(θ-sin). y=a(1-cosθ), a>0 and the axis.
Solution:
The area of the curve \(\mathbf{A}=\frac{1}{2} \oint_C(x d y-y d x)\)
Now \(x=a(\theta-\sin \theta), y=a(1-\cos \theta) \theta\) varies from 0 to \(2 \pi\).
∴ A = \(\frac{1}{2} \int_{\mathcal{C}}^{2 \pi}\left[a(\theta-\sin \theta) a \sin \theta-a^2(1-\cos \theta)^2\right] d \theta\)
= \(\frac{a^3}{2} \int_0^{2 \pi} \theta \sin \theta-\frac{a^2}{2} \int_0^{2 \pi} 2(1-\cos \theta) d \theta\)
= \(\frac{a^2}{2}[-\theta \cos \theta+\sin \theta]_0^{2 \pi}-2 \frac{a^2}{2}[\theta-\sin \theta]_0^{2 \pi}=3 \pi a^2\)
4. Find the area bounded by the hypocycloid x2/3+y2/3=a2/3, a>0 [Hint: Take x=a cos3 θ,y= a sin3θ ].
Solution:
Here x=a cos3 θ, y= asin3 θ and varies from 0 to π/2
A = \(\int_C x d y-y d x=\int\left(a \cos ^3 \theta\right) \cdot\left(3 a \sin ^2 \theta \cos \theta\right)+\left(a \sin ^3 \theta\right)\left(3 a \cos ^2 \sin \theta\right) d \theta\)
= \(3 a^2 \cdot 2 \int_0^{\pi / 2} \sin ^2 \theta \cos ^2 \theta d \theta=\frac{3}{8} \pi a^2\)
5. Find \(\oint_C\)(3x2– 8y2)dx+ (4y-6xy) dy by Green’s theorem where C is the boundary defined by x=0, y=0, x+y=1.
Solution:
P = \(3 x^2-8 y^2, \mathbf{Q}=4 y-6 x y\)
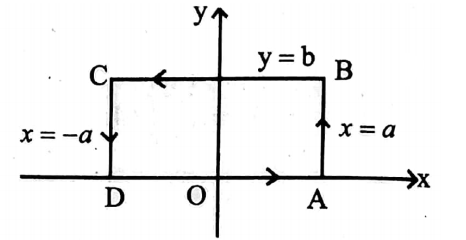
⇒ \(\frac{\partial \mathbf{P}}{\partial y}=-16 y \quad \frac{\partial \mathbf{Q}}{\partial x}=-6 y\)
⇒ \(\int_C \mathbf{P} d x+\mathbf{Q} d y=\iint_S\left(\frac{\partial \mathbf{Q}}{\partial x}-\frac{\partial \mathbf{P}}{\partial y}\right) d x d y\)
= \(\int_{x=0}^1 \int_{y=0}^{1-x}(10 y) d y d x=10 \int_0^1\left[\frac{y^2}{2}\right]_0^{1-x} d x=5 \int_0^1(1-x)^2 d x=\frac{5}{3}\)
6. Find \(\oint_C\)(2x2-y2) dx+(x2+y2) dy where C is the boundary of the surface in the xy-plane enclosed by the x-axis and the circle x2+y2=1.
Solution:
⇒ \(\frac{\partial \mathbf{P}}{\partial y}=-2 y \quad \frac{\partial \mathbf{Q}}{\partial x}=2 x\)
= \(\iint_S\left(\frac{\partial \mathbf{Q}}{\partial x}-\frac{\partial \mathbf{P}}{\partial y}\right) d x d y=\int_{x=-1}^1 \int_{y=0}^{\sqrt{1-x^2}}(2 y+2 x) d x d y\)
= \(\int_{-1}^1\left[2 x y+y^2 \int_{y=0}^{\sqrt{1-x^2}} d x=\int_{-1}^1\left[2 x \sqrt{1-x^2}+1-x^2\right] d x\right.\)
= \(\left[-\frac{2}{3}\left(1-x^2\right)^{3 / 2}+x-\frac{x^3}{3}\right]_{-1}^1=\frac{4}{3}\)
7. Find \(\oint_C\)(x2+y2)dx+3xy2 dy whre C is the circle x2+y2=4 in xy-plane.
Solution:
⇒ \(\int \int\left(\frac{\partial \mathbf{Q}}{\partial x}-\frac{\partial \mathbf{P}}{\partial y}\right) d x d y=\iint_S\left(3 y^2-2 y\right) d x d y\)
= \(\int_{x=-2}^2 \int_{y=-\sqrt{4-x^2}}^{\sqrt{4-x^2}}\left(3 y^2-2 y\right) d y d x=\int_{-2}^2\left[y^3-y^2\right]_{-\sqrt{4-x^2}}^{\sqrt{4-x^2}} d x\)
= \(2 \int_{-2}^2\left(4-x^2\right)^{3 / 2} d x=2.2 \int_0^2\left(4-x^2\right)^{3 / 2} d x\)
= \(64 \int_0^{\pi / 2} \cos ^4 \theta \cdot d \theta=64 \cdot \frac{3}{4} \cdot \frac{1}{2} \cdot \frac{\pi}{2}=12 \pi\)
