Vector Calculus 4th Edition Chapter 7 Surface Integrals and Vector Analysis
Page 467 Problem 1 Answer
Given the X(s,t)=(s2−t2,s+t,s2+3t)
To find the a normal vector to this surface at the point(3,1,1)=X(2,−1)
Using the method of cross product of vector.
We have givenX(s,t)=(s2−t2,s+t,s2+3t)
First we calculate
dX/ds=(2s,1,2s)
dX/dt=(−2t,1,3)
Now, we have to find the value of these factor for(s,t)=(2,−1)
Two vector we obtain are(4,1,4) and(2,1,3)
Now, normal vector is given as cross product of these two vector:
(4,1,4)×(2,1,3)=(−1,−4,2)
The normal vector to this surface at the point(3,1,1)=X(2,−1) is given by(−1,−4,2).
Page 467 Problem 2 Answer
Given the parametrized surface X(s,t)=(s2−t2,s+t,s2+3t)
To find the an equation for the plane tangent to this surface at the point(3,1,1)
Using the formula for equation of tangent.
The formula for equation of tangent line is given point(a,b,c) is n(x−a,y−b,z−c)=0
Where n denote normal vector.
Hence our equation become
Read and Learn More Susan Colley Vector Calculus Solutions
(−1,−4,2)×(x−3,y−1,z−1)=0 where is(3,1,1)
Expand everything to expand the equation x+4y−2z−5=0
An equation for the plane tangent to this surface at the point (3,1,1) is x+4y−2z−5=0
Page 467 Problem 3 Answer
Given:X(s,t)=(5+2cost)coss,(5+2cost)sins
To find an equation for the plane tangent to the torus at point
(5−√3/√2,5−√3/√2,1)
Using the method of equation of tangent.
The given parameterization of surface is(5+2cost)coss,(5+2cost)sins,2sint
To find the value of s,t
we have to find the partial derivatives of the given parameterization.
Dϕ/ds=(−(5+2cost)sins,(5+2cost)coss,0)
dϕ/dt=(−2sintcoss,−2sintsins,2cost)
Take the cross product of derivatives above to obtain the formula for the normals,t
(−(5+2cost)sins,(5+2cost)coss,0).(−2sintcos,−2sintsins,2cost)
Plugging the value of s,t we get,
n=√3−5/√2(√3,√3,−√2)
Now we get equation of tangent as
√3x+√3y−√2z−5√6+4√2=0
The equation of tangent is given by √3x+√3y−√2z−5√6+4√2=0
Page 468 Problem 4 Answer
Given: The parametrized surface X(s,t)=(s,s2+t,t2)
To find the graph for this surface−2≤s≤2,−2≤t≤2
Using the method of graph.
The graph is given by
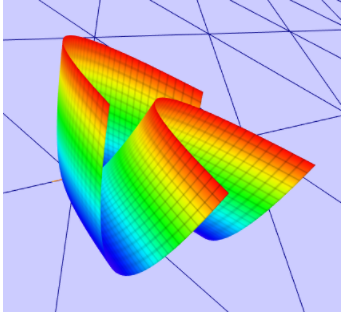
The graph is given by
Page 468 Problem 5 Answer
Given: The parametrized surface isX(s,t)=(s,s2+t,t2).To find the is the surface smooth.
Using the method of vector.
We haveX(s,t)=si^+s2+tj^+t2k^
The surface is smooth if and only if N≠0⃗
Ts=∂x/∂si^+∂y/∂sj^+∂z/∂sk^ And
Tt=∂x/∂ti^+∂y/∂tj^+∂z/∂tz^ Now,
⃗Ts=0i^+1j^+2tk^And
Tt=1i^+2sj^+0k^
Hence,N=Ts×Tt
N=4sti^+2tj^−1k^
Since,k component of normal vector never be zero so the surface is smooth.
Yes,the surface is smooth.
Page 468 Problem 6 Answer
Given: The parametrized surface isX(s,t)=(s,s2+t,t2)
To find an equation for the tangent plane at the point(1,0,1) using the method of tangent plane equation.
We have N=4sti^+2tj^−1k^
Put value of s=1andt=−1
We get,N=−4i^−2j^−1k^
Hence the tangent plane equation is given by
(−4,−2,−1).(x−1,y−0,z−1)=0
−4(x−1)−2y−1(z−1)=0
The tangent plane equation equation is given by−4(x−1)−2y−1(z−1)=0
Page 468 Problem 7 Answer
Given: Equation of formz=f(x,y)
To find the parametrized surface of Exercise1
Using the method of vector.
We look at the parameterization given to us in exercise 1.
Since,x=s2−t2 andy=s+t
We want to express x overy then and we do this with:
x=y(s−t)
With this, now the goal is to isolatet and s in term of x,y
We can reform above equation as
X/y=s−t
From this and second coordinate in parametrized we have
2s=y+x/y and 2t=y−x/y
Now, write down z in term of newly expressed s,t
We have,
z=1/4(y+x/y)2+3/2(y−x/y)
The parametrized surface of Exercise1 is given by 1/4(y+x/y)2+3/2(y−x/y)
Page 468 Problem 8 Answer
Given: S be the surface parametrized bex=scost,y=ssint,z=s2
To find an equation for the tangent plane at the point(1,√3,4)’Using the method of cross product of vector.
The parametrized surface is given as ϕ(s,t)=(scost,ssint,s2)
Partial derivative of the parametrization
dϕ/ds=(cost,sint,2s)
dϕ/dt=(−ssint,−scost,0)
Normal is given with cross product
dϕ/ds×dϕ/dt=(−2s2cost,−2s2sint,s)
Now, recall that surface surface is smooth at every point except origin.
Hence, equation of tangent plane is given as
(x−1,y−√3,z−4).(−4,−4√3,2)=02x+2√3y−z−4=0
Yes, the point S is smooth and the equation of tangent plane is given as2x+2√3
y−z−4=0
Page 468 Problem 9 Answer
Given: S be the surface parametrizedx=scost,y=ssint,z=s2 wheres≥0,0≤t≤2π
To find the sketch the graph of S and recognize S as a familiar surface.Using the method of graph.
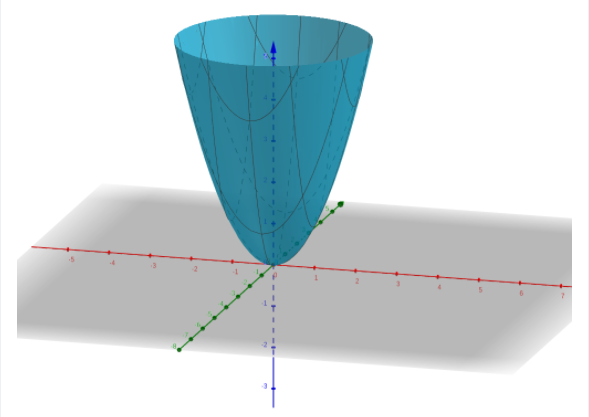
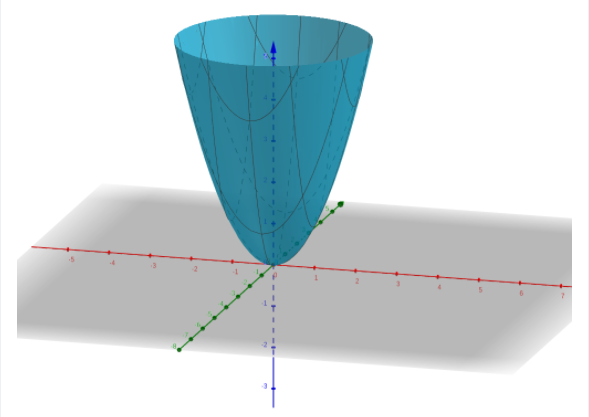
Using the geogebra and we plot the surface as paraboloid.
The surface is paraboloid.
Page 468 Problem 10 Answer
Given S be the surface parametrized byx=scost,y=ssint,z=s2 wheres≥0,0≤t≤2π
To find equation of formz=f(x,y)
Using the method of normal vector.
From parametrization we conclude that
z=x2+y2
This due the fact that we easily cancel out the term with sine and cosine once we square them.
The equation of formz=f(x,y) is z=x2+y2
Page 468 Problem 11 Answer
Given: S be the surface of parameterized byx=scost,y=ssint,z=s2
To find the Using answer in part(c), discuss whether S has a tangent plane at every point.
Using the method of vector and surface integral.
It does. For any point on surface expect the origin we can use the same process as in part(a).
At the origin the tangent plane is the planez=0.
Yes,S has a tangent plane at every point.
Page 468 Problem 12 Answer
Given: The parametrized surface is given asX(s,t)=(2sinscost,3sinssint,coss) where0≤s≤π,0≤t≤2π
To find the parametrized surface is ellipsoid.
Using the method of surface integral.
Simply try fitting the parametrized of the given surface into the ellipsoid equation.
The general ellipsoid equation is given by, ax2+by2+cz2=1
Now we simplify to find the value of a,b,c for which the parametrized satisfy.
a(2sinscost)2+b(3sinssint)2+c(coss)2=1
From this we have,a=1/4,b=1/9,c=1
The equation of ellipsoid is given by x2/4+y2/9+z2=1
Page 468 Problem 13 Answer
Given: The coordinate curve, when t=t0, is a circle of radiusa+bcost0
To find the torus of example 5 is a circle.Using the method of surface integral.
When we fixt=t0 the parametrization only depend on s.
Hence the new parametrization is a single variable function.
γ(s)=((a+bcost0)coss,(a+bcost0)sins,bsint0)
You can write this as
γ(s)=((a+bcost0)coss,(a+bcost0)sins,(0,0,bsint0))
This is circle with radiusa+bcost0
displayed from the origin on point(0,0,bsint0)
The parametrized as a function of a single variable to confirm the result.
Page 468 Problem 14 Answer
Given The surface in R3 parametrized byX(r,θ)=(rcosθ,rsinθ,θ),r≥0,−∞<θ<∞
To find the r-coordinate curve Method used for vector calculus
(a) When θ=π/3, the r-coordinate curve is given by(r/2,r√3/2,π/3) where r≥0.
This is the ray y=√3/x where x≥0 and z=π/3.
In general, the r-coordinate curve when θ=θ0
0 is a ray in the z=θ0 plane.
The solution is simpler than the following four cases make it seem.
If cosθ0≠0then y=(tanθ0)x
where x≥0
if cosθ0>0and x≤0.
If cosθ0=0,
Then the ray is x=0 with y≥0 if sinθ0>0 and y≤0 if sinθ0<0.
If cosθ0=0 then the ray is x=0
with y≥0 if sinθ0 >0 and y≤0 if sinθ0<0.
Page 468 Problem 15 Answer
Given The surface in R3
parametrized by X(r,θ)=(rcosθ,rsinθ,θ),r≥0,−∞<θ<∞
To find the θ-coordinate curve Method used for vector calculus
When r=1 the θ-coordinate curve is the helix (cosθ,sinθ,θ).
In general, when r=r0 the θ-coordinate curve is the helix (r0cosθ,r0sinθ,θ)
The θcoordinate curve in the helix is: (r0cosθ,r0sinθ,θ)
Page 468 Problem 16 Answer
Given The surface in R3
parametrized by X(r,θ)=(rcosθ,rsinθ,θ),r≥0,−∞<θ<∞
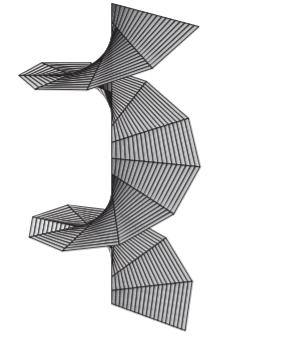
To find the graph of the helicoidMethod used for vector calculus
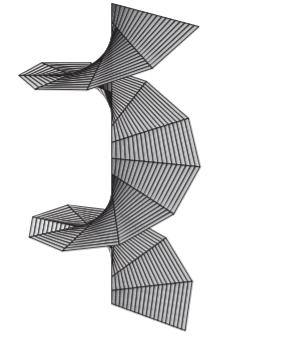
(c) You can see that the helicoids are made up of the helices that are the θ-coordinate curves
The graph of the helicoid
Page 468 Problem 17 Answer
Given the equation form of f(x,y)=√4−(x−2)2−(y+1)2
To find an equation for the plane tangent to it at the pointMethod used for vector calculus
(a) First we consider the sphere as the graph of the function f(x,y)=√4−(x−2)2−(y+1)2.
The partial derivatives are fx=−(x−2)√4−(x−2)2−(y+1)2,fy=−(y+1)√4−(x−2)2−(y+1)2.
So, fx(1,0,√2)=1/√2 and fy(1,0,√2)=−1/√2.
By Theorem 3.3 of Chapter 2, z=f(a,b)+fx(a,b)(x−a)+fy(a,b)(y−b).
In this case, this is z=√2+(1/√2)(x−1)−(1/√2) y, or equivalently −x+y±√2 z=1
An equation for the plane tangent to it at the point (1,0,√2) by considering the sphere as the graph of the function f(x,y)=√4−(x−2)2−(y+1)2 is: z=√2+(1/√2)(x−1)−(1/√2)y, or equivalently −x+y±√2 z=1
Page 468 Problem 18 Answer
Given the equation form of F(x,y,z)=(x−2)2+(y+1)2+z2
To find an equation for the plane tangent to it at the pointMethod used for vector calculus
(b) Now we look at the sphere as a level surface of F(x,y,z)=(x−2)2+(y+1)2+(z)2.
The gradient ∇F(x,y,z)=2(x−2,y+1,z) and,Therefore, ∇F(1,0,√2)=(−2,2,2,√2).
By formula (5) of Section 2.6, the tangent plane is given by 0=∇F(1,0,√2).(x−(1,0,√2))=(−2,2,2,√2)⋅(x−(1,0,√2).
This too is equivalent to −x+y+√2z=1.
an equation for the plane tangent to it at the point (1,0,√2) by considering the sphere as a level surface of the function ∇F(x,y,z)=2(x−2,y+1,z) is: −x+y+√2 z=1.
Page 468 Problem 19 Answer
Given the sphere as the surface parametrized by X(s,t)=(2sinscost+2,2sinssint−1,2coss)
To find an equation for the plane tangent to it at the pointMethod used for vector calculus
(c) Now we’ll use the results of this section.
Considering the z-component, We see 2coss=√2
so cos s=√2/2.
Considering the y- and x-components, 2sinssint=1 and sinscost=−1.
Thus we have that s=π/4 and t=3π/4.
Also Ts(s,t)=(2cosscost,2cosssint,−2sins) and
Tt(s,t)=(−2sinssint,2sinscost,0).
A normal vector to the sphere at the specified point is
N(π/4,3π/4)=Ts (π/4,3π/4)×Tt(π/4,3π4)
N(π/4,3π/4)=(−1,1,−√2)×(−1,−1,0)
N(π/4,3π/4)=(−√2,√2,2)
The tangent plane is given by(−√2,√2,2).(x−(1,0,√2))=0
Which is also equivalent to−x+y+√2z=1.
An equation for the plane tangent to it at the point (1,0,√2) by considering the sphere as the surface parametrized by X(s,t)=(2sinscost+2,2sinsint−1,2coss) is: −x+y+√2z=1.
Page 468 Problem 20 Answer
Given the cylinder x2+z2=4 lying between y=−1 and y=3
To find represent the given surface as a piecewise smooth parametrized surface.
Method used for vector calculus.
Imagine the cylinder as a trace of the circle which has its origin slid between (0,−1,0) and (0,3,0).
From here, this gives us the idea how to parametrize such object.
If y=0 our cylinder is just a circle in x,z plane.
The radius of the circle is 2 hence the parametrization becomes:
Υ(t)=(2cost,0,2sint),t∈[0,2Π]
We want to allow the circle to slide, so we vary the y coordinate.
Final parametrization of the cylinder is:ϕ(t,s)=(2cost,s,2sint),t∈[0,2Π],s∈[−1,3]
Parametrization of the cylinder is: ϕ(t,s)=(2cost,s,2sint),t∈[0,2Π],s∈[−1,3]
Page 468 Problem 21 Answer
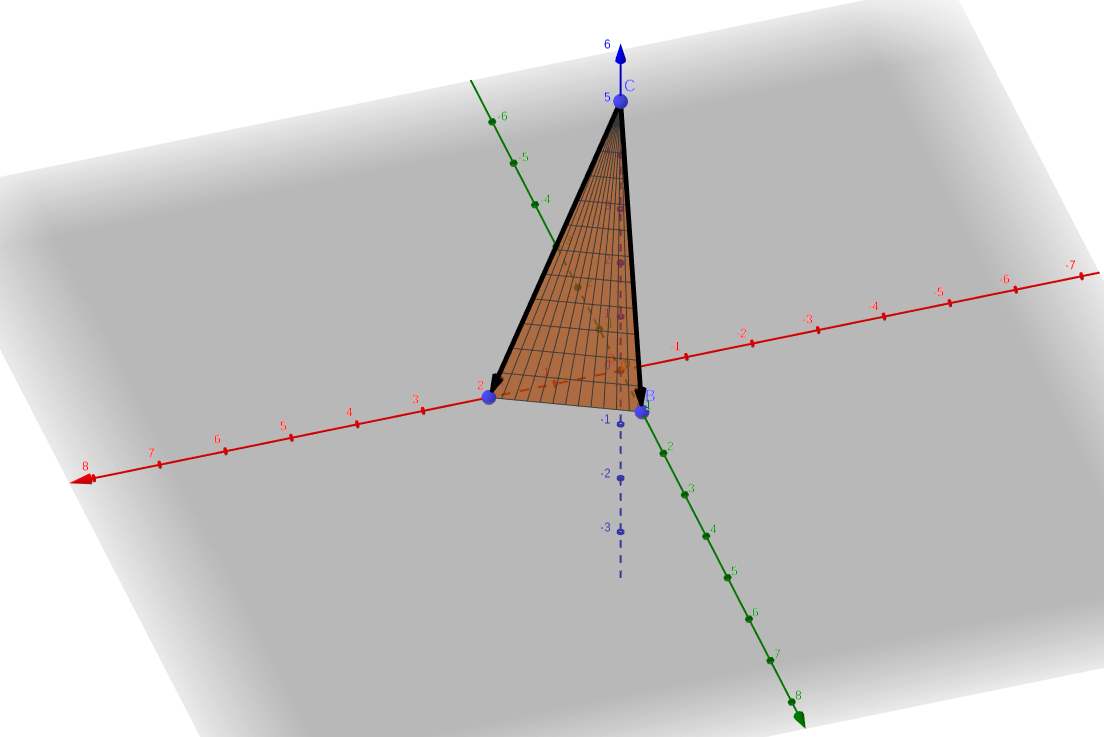
Given The closed triangular region in R3 with vertices (2,0,0),(0,1,0) and (0,0,5)
To find represent the given surface as a piecewise smooth parametrized surface.
Method used for vector calculus.
Easiest way to parametrize the triangle is to consider it as a convex combination of its sides.
To do it, first draw two segments from the point (0,0,5) to the points (2,0,0) and (0,1,0).
Next, we parametrize these segments on such way that they both start at (0,0,5)
and end at their respective end points.
We obtain two curves:
Υ1(t)=(2t,0,5−5t),t∈[0,1]
Υ2 (t)=(0,t,5−5t),t∈[0,1]
The triangle can be viewed as a convex combination of these two curves, hence define the surface parametrization as:
ϕ(t,s)=(1−s)Υ1(t)+Υ2(t),t,s∈[0,1]
The final parametrization is:
ϕ(t,s)=(2t−2ts,ts,5−5t−5s+5ts+5s−5ts)
ϕ(t,s)=(2t−2ts,ts,5−5t)
The image of the area is below.
From there one can see where the idea from the solution comes in.
Every line in the interior of the tringle is a linear combination of its two sides.
Parametrization of triangle is: (2t−2ts,ts,5−5t)
Page 467 Problem 22 Answer
Given the point (1,−1,−1) and the parametrized surface X(s,t)=(s3,t3,st).To find the equation for the parametric surface on the point (1,−1,−1)
Using the partial derivation.
Partial derivative of X=(s3,t3,st) with respect to s
dX/ds=(3s2,0,t)
Partial derivative of X=(s3,t3,st) with respect to t
dX/dt=(0,3t2.s)
Cross multiplication of dX
Ds and dX/dt
(3s2,0,t)×(0,3t2,s)=(3,−3,9)
Now, the required equation of the plane
3(x−1)+(−3)(y+1)+9(z+1))=0
⇒3x−3y+9z+3=0
⇒x−y+3z=−1
The required equation is x−y+3x=−1
Page 467 Problem 23 Answer
Given the parametric plane X(s,t) on (1,−1,−1)
To find whether the curve was plane or not.Using the normal of the plane
The surface is smooth , if its normal vector is non-zero at origin.
Normal vector
(3s3,−3t3,9st) at t=0 and s=0
⇒(0,0,0)
So the surface is not plane.
The surface is not smooth as its normal vector is zero at origin.
Page 467 Problem 24 Answer
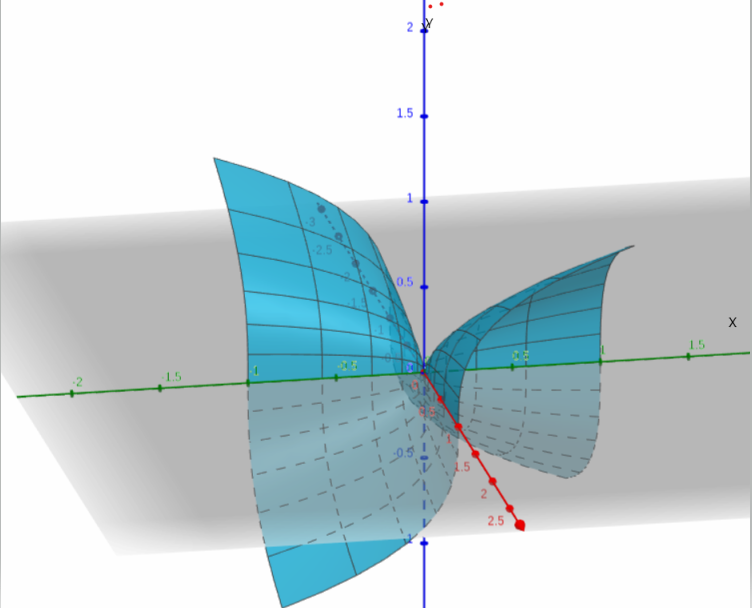
Given the parametric plane X(s,t) and condition −1≤t≤1 and −1≤s≤1.
To find the surface along the given condition.Using plotting of parametric curves.
The surface made under given conditions is
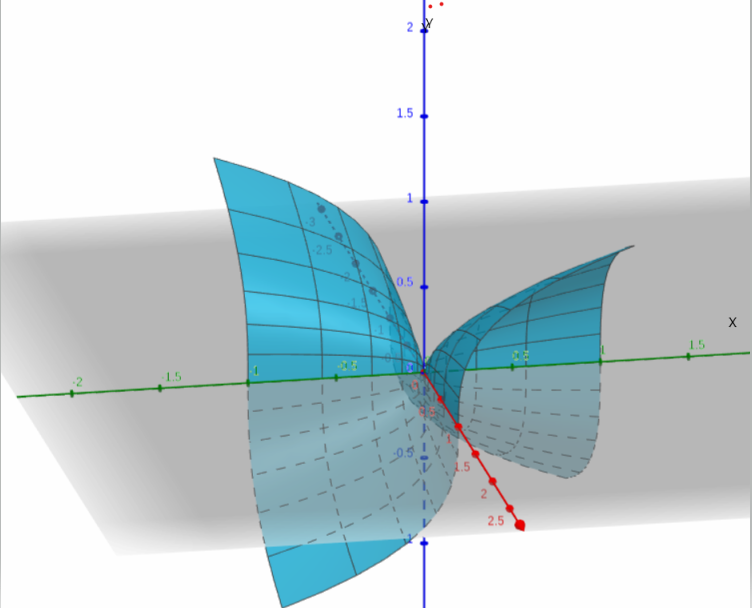
The required graph is
Page 467 Problem 25 Answer
Given the parametrized surface X(s,t) and the equation z=(xy)1/3
To find that X(s,t) at t,s∈[−1,1] ,also shows z=(xy)1/3
Method used for plotting the surfaces on same graph.
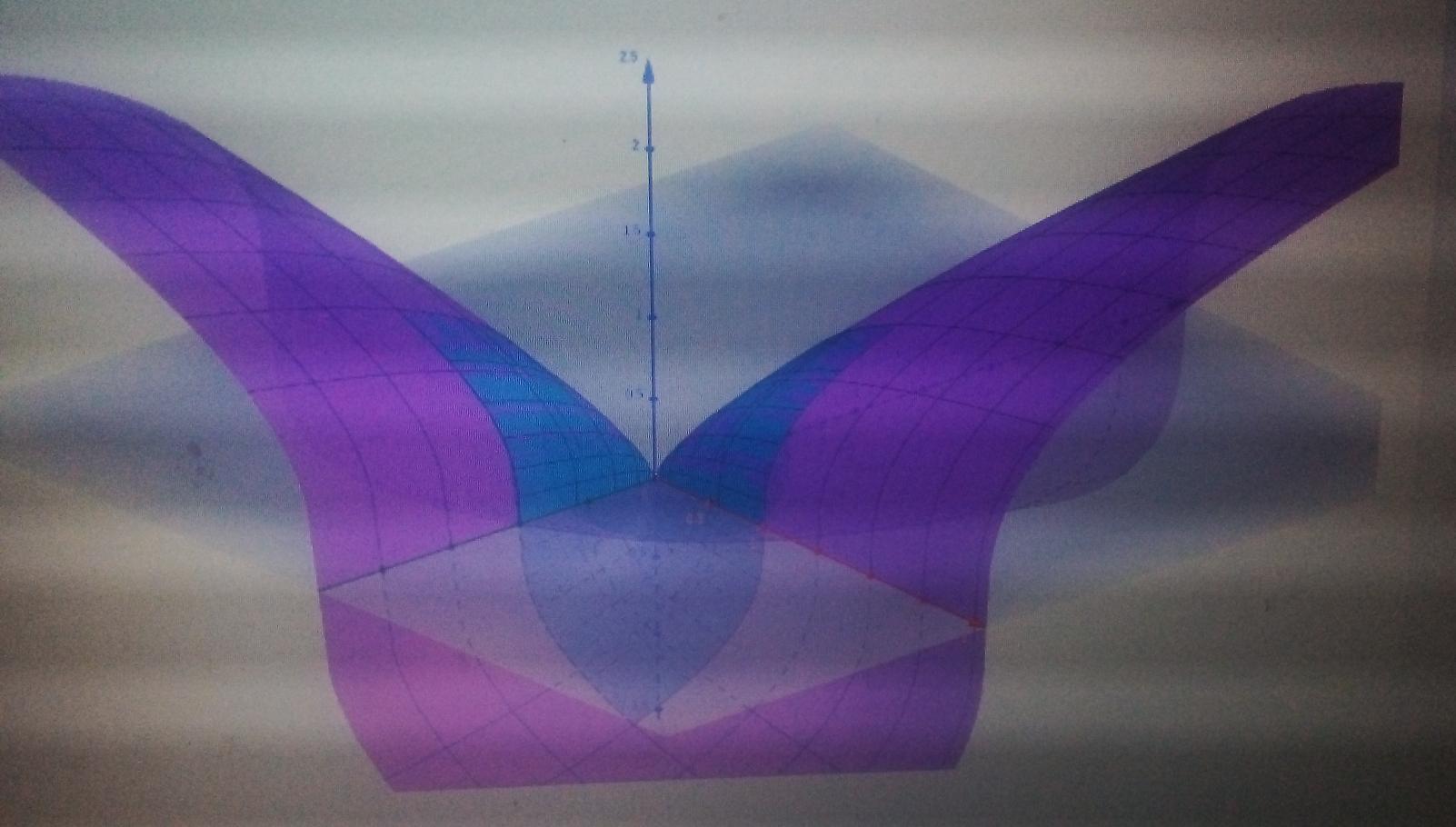
The graph plotted for X(s,t)=(s3,t3,st) at s,t∈[−1,1] and the equationz=(xy)1/3
Here, numerically if x=s3 and y=t3,
Then z=(s3⋅t3)1/3z=st
Which satisfies X(s,t)=(s3,t3,st)
The graph of z=(xy)1/3 also include the graph of X(s,t)